Fractions: A Primary School Teacher’s Guide

Here we’ve collected all our best articles and resources to help you teach fractions effectively. This information is predominantly aimed at the fraction concepts pupils learn during years 3 to 6 in primary school (Key Stage 2). 

If you’re looking for higher level content then our GCSE revision guide on fractions is the place to start. In this article, we’ll explain the basics of KS2 fractions, including the different types of fractions and how these fit into the primary school national curriculum.

What is a fraction?

A fraction is any part of a group, number, or whole. More specifically, it’s a mathematical expression that represents the division of one whole number by another. For example, the fraction \frac{1}{5} represents 1 divided by 5 or 1 parts out of 5 parts. 

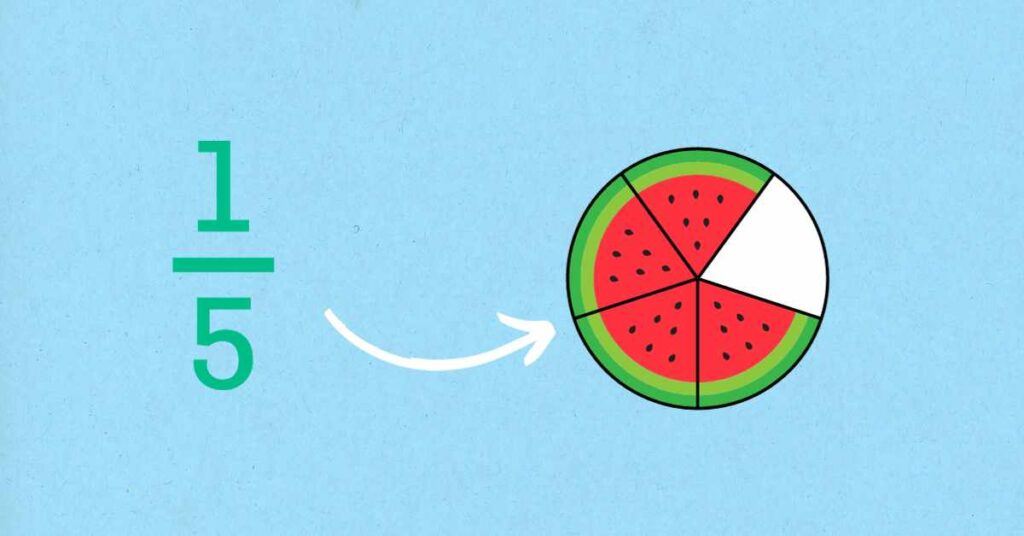
A fraction has two parts: the numerator and denominator. These consist of a numerator (the top number) and a denominator (the bottom number), separated by a vinculum or fraction bar. In essence, a fraction tells us how many parts of a whole we have.

What is the fraction of a whole?

When we talk about a fraction of a whole, we’re referring to how many pieces a whole is divided into and how many of those pieces we’re considering. For example, if an apple is cut into four equal parts and you have one, then you have a fraction of the whole apple, which is \frac{1}{4} . 

If the number 20 is the whole and you share it into four equal parts, one part is \frac{1}{4} which in this case would be 5.

The basics of fractions

Denominator

The denominator is the number below the bar. The denominator is the bottom part of a fraction. It tells you the total number of equal parts that make up a whole. For example, in the fraction \frac{3}{4} , the denominator is 4. This means that the whole is divided into 4 equal parts.

Numerator

The numerator is the top part of a fraction. It represents the number of equal parts you have or are considering. Using the same fraction example, \frac{3}{4} , the numerator is 3, which means you have 3 out of the 4 equal parts that make the whole.

Whole number

A whole number is an integer that is not a fraction or a decimal. Whole numbers can be represented as fractions by placing them over a denominator of 1. For example, the whole number 5 can be represented as the fraction \frac{5}{1} .

Decimal

A decimal is another way to represent a fraction, where the denominator is a power of 10. You can convert a fraction to a decimal by dividing the numerator by the denominator. For example, to convert \frac{3}{4} to a decimal, you would divide 3 by 4, giving you 0.75.

Another way to calculate this, is to convert \frac{3}{4} into hundredths, by multiplying both the denominator and the numerator by 25. This gives the fraction \frac{75}{100} which is easier to convert into a decimal than \frac{3}{4} .

Types of fractions

Improper fractions 

Improper fractions have a numerator larger than its denominator. For example, \frac{5}{3} is an improper fraction. These types of fractions often appear in advanced calculations and real-world scenarios.

Proper fractions 

A proper fraction has a numerator smaller than its denominator, like \frac{3}{4} . Proper fractions are often used to represent a part of a whole, such as a slice of pizza or a piece of fruit.

Mixed numbers

A mixed number combines a whole number with a fraction. For example, 2 \frac{1}{3} is a mixed number, comprising the whole number 2 and the fraction \frac{1}{3} . Mixed numbers are particularly useful in everyday scenarios like cooking or carpentry, where you might deal with whole units and fractions simultaneously.

Unit fractions 

A unit fraction is a fraction that contains a numerator of 1, such as \frac{1}{4} or \frac{1}{5} . These fractions are the building blocks for other fractions and are often the first type of fraction taught to pupils.

Non-unit fractions 

Unlike unit fractions, non-unit fractions have a numerator larger than 1, like \frac{3}{4} or \frac{5}{6} . Pupils will encounter this type of fraction typically when finding fractions of an amount. Here children are taught to first calculate the unit fraction of the amount and multiply this by the numerator.

For example, when finding \frac{5}{6} of 24, pupils will begin by finding \frac{1}{6} of 24, which is 4.

This then needs to be multiplied by 5, to calculate \frac{5}{6} of 24, which is 20.

Equivalent fractions

Equivalent fractions are fractions that represent the same value, even though they may look different.

For example, \frac{1}{2} is equivalent to \frac{2}{4} or \frac{3}{6} .

Understanding equivalent fractions is crucial for simplifying calculations and solving problems.

Multiples and factors

In the context of fractions, multiples and factors refer to the common divisors and multiples of the numerator and denominator. The highest common factor (HCF) is the largest number that the numerator and the denominator can be divided by, while the lowest common multiple (LCM) is the smallest number that both numbers are factors of.

For example, if the fraction is \frac{12}{20} , the HCF of 12 and 20 is 4, and the LCM is 60.

Reducing a fraction to its simplest form involves dividing both the numerator and denominator by their HCF. In this case, dividing 12 and 20 by 4 gives the simplest form of the fraction: \frac{3}{5} .

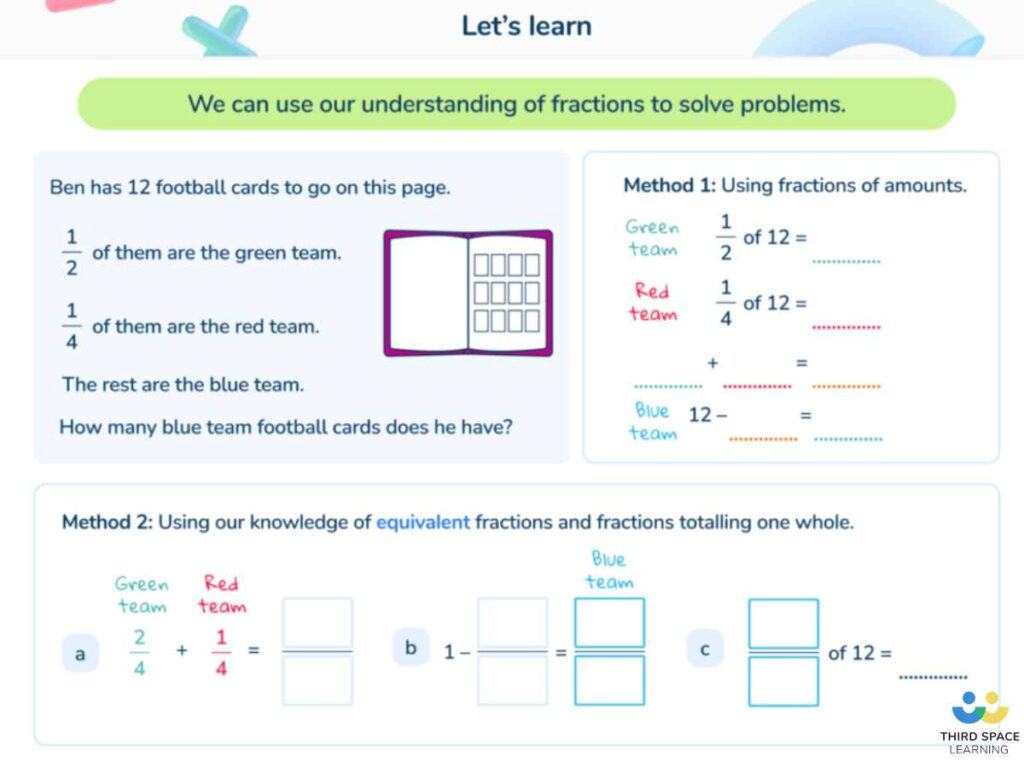
Common denominator

A common denominator refers to a shared multiple of the denominators of two or more fractions. Once you know how to add fractions or how to subtract fractions, you need to find the lowest common denominator (the smallest multiple they share) to ensure that the fractions are compatible for these operations.

Operations involving fractions

Multiplying fractions

In terms of knowing how to multiply fractions, you simply multiply the numerators (top numbers) together and the denominators (bottom numbers) together. Here’s how:

1. Multiply the numerators: numerator x numerator

2. Multiply the denominators: denominator x denominator

3. Simplify the resulting fraction if possible. For example, to multiply \frac{2}{3} and \frac{3}{4} , you’d calculate: \frac{(2 x 3)}{(3 x 4)} = \frac{6}{12} = \frac{1}{2}

Dividing fractions

In terms of knowing how to divide fractions, you’ll perform the following steps:

1. Invert the second fraction (swap its numerator and denominator)

2. Then multiply the two fractions (as explained above)

For example, to divide \frac{2}{3} by \frac{4}{5} :

1. Invert the second fraction: \frac{5}{4}

2. Multiply the resulting fractions: \frac{(2 x 5)}{(3 x 4)} = \frac{10}{12} = \frac{5}{6}

Adding fractions

To add fractions, you first need to find a common denominator:

1. Find the lowest common multiple (LCM) of the denominators

2. Convert both fractions to equivalent fractions with the LCM as their denominator

3. Add the numerators

4. Simplify the resulting fraction if necessary

For example, to add \frac{2}{3} and \frac{3}{4} :

1. LCM (3, 4) = 12

2. Convert: \frac{8}{12} and \frac{9}{12}

3. Subtract numerators: 9 - 8 = 1

4. Simplified fraction: \frac{1}{12}

How do fractions relate to other areas of maths at primary school?

Fractions are a fundamental concept in mathematics, connecting to various other topics, including percentages, decimal equivalents, and beyond. It’s worth noting that times tables are an essential skill which pupils must first possess when it comes to fractions.

Once your pupils have grasped times tables, they can start applying their foundational knowledge to comparing fractions. In this section, we will explore the relationships between fractions, decimals, and percentages.

Percentages

Percentages are a powerful way to express ratios, especially when dealing with proportions. You can think of a percentage as a fraction with a denominator of 100. For example, if you have three-fifths of something, you can convert this fraction to a percentage:

Three-fifths = \frac{3}{5} = \left(\frac{3}{5}\right)× 20 = \frac{60}{100} = 60%

By converting fractions to a percentage, you can easily compare fractions that differ and communicate information more effectively.

Decimal equivalents

Just as fractions can be expressed as percentages, so too can they be represented as decimals. In Key Stage 2, children are taught to convert fractions to decimals, by first calculating the equivalent fraction with a denominator of 10 or 100. Once this has been done, it is much easier to convert the fraction into a decimal.

Begin by calculating how many tenths \frac{3}{5} is equivalent to:

Three-fifths = \frac{3}{5} = \frac{6}{10} = 0.6

You can also express decimals as fractions by considering their place value. For example, 0.6 means there are 6 tenths, which is written as \frac{6}{10} :

0.6 + \frac{6}{10}

Converting between fractions, decimals, and percentages is an important skill, enabling you to approach problems in diverse ways and connect different representations of numbers.

When working with fractions in algebra, it is important to be comfortable with operations involving fractions. For example, when solving equations involving fractions, it can be helpful to find a common denominator or convert the fractions to decimals or percentages.

Fractions also have their place on the number line, as they fill the gaps between the integers. By understanding the relative positions of fractions on the number line, you can compare them more easily and use them in tasks such as estimation and approximation.

In summary, fractions are an essential part of mathematics, connecting to percentages, decimal equivalents, algebra, and more. By mastering the use of fractions in different contexts, you can enhance your problem-solving skills and communication of mathematical ideas.

When do pupils learn about fractions in the national curriculum?

In the UK, pupils are introduced to the concept of fractions during their primary school education. As a part of the National Curriculum, pupils start learning about fractions in Year 1, at the age of five or six.

Fractions in year 1

In Year 1, pupils begin to grasp the basics of fractions by understanding the concept of halves and quarters. They learn to recognise and find half of a shape, object, or quantity. Additionally, they explore how to divide quantities into two equal parts and recognise that each part is a half.

Fractions in year 2 

As pupils progress to Year 2, they build upon their understanding of fractions and start learning about thirds. They continue to work with simple fractions, practising to find non-unit fractions of small numbers, such as half, quarter, and third of a set of objects or quantities.

Fractions in year 3 and year 4

In Years 3 and 4, pupils delve deeper into fractions by learning about unit and non-unit fractions and equivalent fractions. They are taught how to compare and order fractions with the same denominator, add and subtract fractions with the same denominator, and find the fractions equivalent to a whole number.

Fractions in year 5 and year 6

By Years 5 and 6, pupils have a more in-depth knowledge of fractions and further explore mixed numbers, improper fractions, and equivalent fractions. They also learn how to add and subtract fractions with different denominators, multiply simple fractions, and divide proper fractions by whole numbers.

The pace children learn fractions varies considerably. With fractions concepts being quite abstract, it is essential to provide them with the appropriate support and resources to consolidate their understanding. 

Read more: 24 Fraction Questions for Year 6: Complete with Answers

Fractions in real life

We regularly encounter fractions in our everyday lives. Let’s explore some real-life situations where you may use fractions.

Imagine you are cooking a recipe that requires cutting up vegetables. You need to serve 5 people, and the recipe calls for half a carrot per person. To determine the total amount of carrots, you could count in halves until you’ve counted 5 halves, or multiply \frac{1}{2} by 5, which equals \frac{5}{2} or 2 and a half carrots.

When shopping, you can often find discounts on products with fractions like “Save \frac{1}{3} on the regular price!”. To find out how much you’ll save on a product, you would calculate one-third of £15, by dividing £15 by 3. From this you can work out that \frac{1}{3} of £15 is £5, therefore a \frac{1}{3} discount would be a  £5 reduction of the original price, making the new price £10.

Consider a sports competition where you and your friends participate in a relay race. Your team of five people sets a relay distance where each team member covers four-fifths (\frac{4}{5}) of a mile. To find out the total distance your team will run, simply multiply the per person distance (\frac{4}{5}) by the number of participants, in this case, 5. The result is 4 miles.

When your parents are working out how much money they have to spend, they might allocate percentages of their income to various expenses. For example, three-fifths (60%) of their income could go to essentials like rent and groceries, and the remaining two-fifths (40%) to savings, entertainment, or other things.

As you can see, fractions play a significant role in many aspects of your daily life. By understanding their value and how to use them, you can become more confident and knowledgeable in various real-life situations involving fractions.

3 fractions worked examples with answers

In this section, we will explore three worked examples of common fractions problems, with detailed solutions to help improve your understanding.

Example 1: Simplifying fractions

Consider the fraction \frac{18}{24} . To simplify this fraction, follow these steps:

1. Identify the highest common factor (HCF) of the numerator and denominator. In this case, the HCF of 18 and 24 is 6.

2. Divide both the numerator and denominator by the HCF. Here, 18 ÷ 6 = 3 and 24 ÷ 6 = 4 .

So, the simplified fraction is \frac{3}{4} .

Example 2: Adding fractions

Suppose you want to add the following fractions: \frac{4}{5} and \frac{2}{15} .

1. Determine the lowest common multiple (LCM) of the denominators. In this case, the LCM of 5 and 15 is 15.

2. Convert the fractions to equivalent fractions with the LCM as the common denominator. \frac{4}{5} becomes \frac{(4 × 3)}{15} , or \frac{12}{15} . \frac{2}{15} remains the same.

3. Add the numerators and keep the common denominator. \frac{12}{15} + \frac{2}{15} = \frac{(12 + 2)}{15} .

Hence, the sum of the fractions is \frac{14}{15} .

Example 3: Multiplying fractions

Let’s say you need to multiply the fractions \frac{3}{8} and \frac{5}{14} .

1. Multiply the numerators together. 3 × 5 = 15.

2. Multiply the denominators together. 8 × 14 = 112.

Now, you have the fraction \frac{15}{112} . Since 15 and 112 have no common factors apart from 1, the result is already in its simplest form.

These examples should provide a greater insight into how to solve various fractions problems. Remember, it’s important to know how to simplify fractions when necessary and take care to find common denominators when adding or subtracting fractions.

5 practice fraction questions

Here are five practice fraction questions:

1. What is the sum of \frac{3}{4} and \frac{1}{8} ?
To solve this, you need to find a common denominator. 8 is the lowest common denominator. You can convert the fractions as follows:
    • \frac{3}{4} becomes \frac{6}{8}
    • \frac{1}{8} remains the same
Now you can add the fractions: \frac{6}{8} + \frac{1}{8} = \frac{7}{8} . So, the sum is \frac{7}{8} .

2. Simplify the fraction \frac{24}{40} to its lowest terms.
You can simplify a fraction by dividing both the numerator and denominator by their HCF (HCF). The HCF of 24 and 40 is 8. Divide both numbers by 8:
    • 24 ÷ 8 = 3
    • 40 ÷ 8 = 5
The simplified fraction is \frac{3}{5} .

3. What is the product of \frac{5}{6} multiplied by \frac{3}{5} ?
To multiply fractions, multiply the numerators together and the denominators together:
\frac{(5 × 3)}{(6 × 5)} = \frac{15}{30} . Now you must simplify the fraction by dividing both the numerator and denominator by their HCF, which is 15:
    • 15 ÷ 15 = 1
    • 30 ÷ 15 = 2
The product of \frac{5}{6} and \frac{3}{5} is \frac{1}{2} .

4. How do you divide \frac{3}{4} by \frac{1}{2} ?
To divide fractions, you need to multiply the first fraction by the reciprocal of the second fraction:
\frac{3}{4} ÷ \frac{1}{2} = \frac{3}{4} × \frac{2}{1} = \frac{(3 × 2)}{(4 × 1)} = \frac{6}{4}
Now simplify the fraction by dividing both the numerator and the denominator by their GCD, which is 2:
    • 6 ÷ 2 = 3
    • 4 ÷ 2 = 2
The quotient of \frac{3}{4} divided by \frac{1}{2} is \frac{3}{2} .

5. Calculate \frac{3}{5} of 35.
First, calculate \frac{1}{5} of 35 by dividing 35 by 5 = 7.
If \frac{1}{5} of 35 is 7, \frac{3}{5} of 35 must be 3 × 7 = 21 .

Additional resources

Fraction games and word questions

Fraction games are a great way to make learning fun, interactive, and engaging for your pupils. It allows pupils to consolidate their knowledge and practically apply what they know about fractions to the games. Read our fractions games blog for some suggestions of fraction games to use with your pupils, which can be easily adapted to fit the needs of your pupils and requires minimal resources. We also have a series of fraction word problems for your pupils to apply fractions to real-life scenarios.

Fractions worksheets

These worksheets cover various aspects of fractions, ranging from basic concepts to complex problems with answers always included:

• Year 1 Fractions Independent Recap Worksheets 
• Year 2 Unit Fractions Worksheet 
• Year 3 Equivalent Fractions Worksheet 
• Year 4 Subtract Fractions Worksheet 
• Year 5 Fractions of Amounts Worksheet 
• Year 6 Ordering Fractions, Decimals and Percentages Worksheet 

Read more

• How To Explain KS2 Fractions
• How To Teach Fractions Key Stage 2: Maths Bootcamp