Teaching Division KS2: A Guide For Primary School Teachers From Year 3 To Year 6

Division in Key Stage 2 forms the last part of the ‘Four Operations’ – the four core mathematical concepts all pupils should know. This blog will help you ensure all your pupils, from Year 3 to Year 6, can approach division with the confidence they need to succeed. 

Division in KS2 maths builds on what has been learnt at KS1. By the end of Year 2 students at the expected standard will be able to ‘recall and use multiplication and division facts for the 2, 5 and 10 multiplication tables, including recognising odd and even numbers.’

For teachers in Year 3, it is always a good idea to ensure that the students know these very well. By that, I do not simply mean that they can parrot the division facts at you, but that they truly understand how to represent these facts both with concrete representations, verbal explanations and through other pictorial methods.

Students who are not able to do this, yet who are able to verbally tell you the division facts, have a very shallow understanding of division and in order for them to progress onto more difficult problem solving activities, deepening their conceptual understanding of division is a must at Year 3. 

The national curriculum places division within the same section as multiplication for Years 3-5 and places it within a section called ‘Four Operations’ in Year 6. The parts taken from the national curriculum relate only to those that specifically mention division. The other elements of those sections can be found in the blog on multiplication or addition and subtraction, where appropriate. 

Take a look at our guide to division for kids for KS1 & KS2 and our collection of division worksheets for Years 3-6.

Teaching division KS2 – Sharing, grouping and scaling

Division is the inverse of multiplication. As with the multiplication blog, the teaching of division contains three underlying structures, which, when coupled with division facts and conceptual understanding, will give students the best opportunity to be successful when solving problems related to division.

The three structures are sharing, grouping and scaling. While there is not enough time to explore these in depth, what follows will be just a short introduction to them.

Sharing and grouping

Sharing and grouping are the inverse of multiplication as repeated addition. Sharing is a structural concept that is introduced in KS1 and earlier where, as the name suggests, an amount is shared into equal groups.

For example, 12 apples are shared amongst 3 students. How many apples does each student get?

Here we know the whole amount or dividend (12 apples) and crucially, we know how the amount we are going to share into (3 – the divisor). Depending on where students are on their mathematical journey, they may repeatedly subtract by some number (1, 2, 3 or 4) from the amount into three piles which represent the three students. 

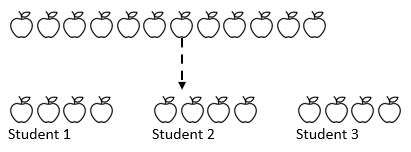
As we can see from the above, the whole amount has been shared into three equal groups.

Grouping is similar to sharing but what we know is slightly different when compared to sharing. In the sharing structure, we know the amount of groups that the sharing will take place. In the grouping structure, we know the amount in each group but not the number of actual groups that there are.

For example, 12 apples are shared in groups of 4. How many groups will there be? While this question is very similar to the one above, it now takes on the grouping structure as we know how many will go into each group but no longer know how many groups there will now be. 

As we know that there are 4 in each group, we can group 4 apples until we have covered all 12 to see that there are 3 groups.

Scaling

The final structure of division is scaling. While in multiplication we were scaling up a value, the division structure scales down. For example, Shannen has 6 pens and Jen has 3 times as fewer. How many pens does Jen have?

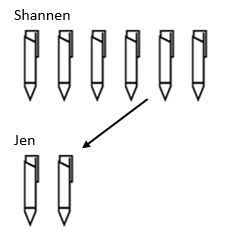
Here, we can see that to find the total amount of pens that Jen has, we had to take the amount that Shannen had and decrease it by a scale factor of 3 so that Jen ends up with 2 pens.

From experience, this is the hardest division structure for students to grasp and understand and is one that needs to be revisited in conjunction with scaling as multiplication as this is a crucial skill when converting measurements and currencies from a larger amount to a smaller amount, e.g. kilometres to miles.  

Division Year 3

In the national curriculum for maths in England, for each area of maths outlined, there is both a statutory element and a non-statutory element. The statutory element is as follows:

• Recall and use multiplication and division facts for the 3, 4 and 8 multiplication tables.
• Write and calculate mathematical statements for multiplication and division using the multiplication tables that they know, including for two-digit numbers times one-digit numbers, using mental and progressing to formal written methods.
• Solve problems, including missing number problems, involving multiplication and division, including positive integer scaling problems and correspondence problems in which n objects are connected to m objects. 

Non-statutory notes and guidance: 

• Pupils develop efficient mental methods, for example, using commutativity and associativity (for example, 4 × 12 × 5 = 4 × 5 × 12 = 20 × 12 = 240) and multiplication and division facts (for example, using 3 × 2 = 6, 6 ÷ 3 = 2 and 2 = 6 ÷ 3) to derive related facts (for example, 30 × 2 = 60, 60 ÷ 3 = 20 and 20 = 60 ÷ 3). 
• Pupils develop reliable written methods for multiplication and division, starting with calculations of two-digit numbers by one-digit numbers and progressing to the formal written methods of short multiplication and division. 
• Pupils solve simple problems in contexts, deciding which of the four operations to use and why. These include: measuring and scaling contexts (for example, four times as high, eight times as long etc.) and correspondence problems in which m objects are connected to n objects (for example, 3 hats and 4 coats, how many different outfits?; 12 sweets shared equally between 4 children; 4 cakes shared equally between 8 children). 

Division: Lesson ideas Year 3

This will not cover a lesson, but provide a guide as to how division can be taught with some conceptual understanding at this stage. A pre-requisite for this would be the teaching of related multiplication facts and times tables first before teaching any related division.

When thinking about structure, it is best at this part of students’ learning journey to stick with grouping and sharing. For the following examples, we will look at developing students understanding of dividing by 4. It will follow the Concrete-Pictorial-Abstract approach. 

It is often students’ understanding of number that can limit their progress in division. Particularly when it comes to grouping and sharing when they only take one away continuously from the whole.

From the outset of teaching division, teachers should lean on what students know already about even numbers and other number facts to progress students onto counting in whatever number may be appropriate.   

Counters can be used to demonstrate related division facts as students will have used these when building their conceptual understanding of multiplication. Questions such as, ‘How many groups of 4 are there?’ and ‘How many are there in each group?’ should be asked and the answers should relate to how this shows division. 

You can see how that would be repeated for the following:

When students are beginning to get confident with this, we can move on to other models such as Cuisenaire rods.

Here, the whole amount is represented in the top train and the purple rod (which, in this case, represents 4 and if you have read other blogs in this series, hopefully you will know how much of an advocate I am for their use in the classroom and so students will be aware of its value by then) is used to show the number of times groups of 4 can go into the total amount. 

Students can then move on to drawing out scenarios based on division calculations read by the teacher. For example, there are 24 buttons and they need to be grouped into 4. How many groups are there? Students could draw those buttons and group them into 4, etc.

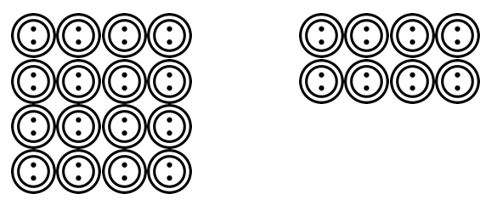
Once students have gotten used to being able to visualise these grouping or sharing division scenarios in a range of contexts, then they can move on to more abstract representations using mathematical symbols.

Division: Word problems Year 3

Word problems in Year 3 will commonly use the grouping or sharing structure.

There are 40 cakes and 10 students. How many cakes would each student get?

As we know how many groups there are (10), we need to work out the amount per group so this is a sharing structure. Without a strong knowledge of the 4 times table, students will struggle with the process of dividing as they will need to use this knowledge to get the answer 4.

Division: Reasoning and problem solving Year 3

For reasoning and problem solving, a good activity I find is to match a word problem to a calculation. For example:

Getting students to write down their explanations for each question is useful as it allows to see their thinking. The questions have been selected carefully so that the numbers in each question relate to the numbers on the question so students are relying on their mathematical skills to deduce the correct answer. 

Division Year 4

In the national curriculum for maths in England, for each area of maths outlined, there is both a statutory element and a non-statutory element. The statutory element is as follows:

• Recall multiplication and division facts for multiplication tables up to 12 × 12.
• Use place value, known and derived facts to multiply and divide mentally, including: multiplying by 0 and 1; dividing by 1; multiplying together three numbers.

Non-statutory notes and guidance:

• Pupils continue to practise recalling and using multiplication tables and related division facts to aid fluency. 
• Pupils practise mental methods and extend this to three-digit numbers to derive facts, (for example 600 ÷ 3 = 200 can be derived from 2 x 3 = 6). 
• Pupils practise to become fluent in the formal written method of short multiplication and short division with exact answers.
• Pupils solve two-step problems in contexts, choosing the appropriate operation, working with increasingly harder numbers. This should include correspondence questions, such as the numbers of choices of a meal on a menu, or three cakes shared equally between 10 children.

Division: Lesson ideas Year 4

For this lesson, I will look at how you can begin to teach short division using manipulatives alongside it to divide increasingly larger numbers.

Say we are going to teach students short division for the question 168 divided by 12.

We would normally write this in what is commonly referred to as the bus stop method. But what is interesting is that different places in the world would write it differently.

The reason for this is quite simple. What the ‘bus stop’ actually represents is a shortened way to represent a rectangle.

When we teach formal short division, what we are effectively saying is that you need to imagine a rectangle where the width is 12. The total area of the rectangle is 168 and we are attempting to find the missing length across the top. So how do we show this using manipulatives? Dienes blocks are a useful tool for this.

We know the total amount is 168. So we need to gather 168 worth of dienes using the smallest amounts of blocks possible. This is shown below.

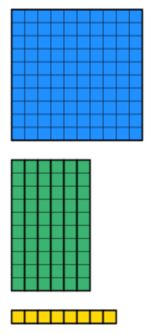
Looking back at the question, we know that the width is 12. That means we now need to manipulate the blocks so that we get a width of 12. Note that the rectangle needs to be solid and can have no missing parts. For example, the below would not be allowed despite there being 12 units going down the width. 

Instead, students can rotate two 10s blocks to get the following. Notice how there is 12 in each column and it is a solid object.

The final part the students must do is use the remaining blocks to create a solid rectangle while keeping the width 12. This could take some time but with practice, students do get quicker. After some time, students will produce this:

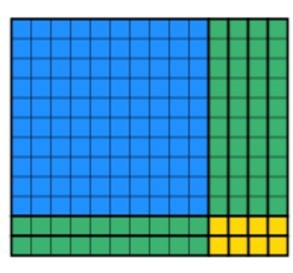
We can see that all 168 worth of blocks have been used up and that the width is still 12. To find the answer, students are required to count the length which in this case is 14. I have superimposed both methods below so you can see how they complement each other.

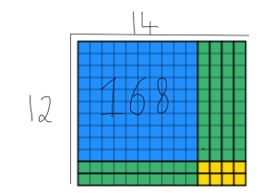
This is a useful strategy to teach students in Year 4 as there are massive repercussions in other areas of the curriculum that are already illuminated to students.  

Though they may not recognise it yet, by teaching short division like this they have already been introduced to perimeter and area – think how much teaching time can be saved when this connection is made. Not only that, but in secondary school, this approach transfers to algebraic thinking.

Division: Word problems Year 4

Students would be expected to draw on their knowledge of place value to solve division-based problems and so an appropriate word problem would be:

An orchard grows 4,800 apples in a season. They are placed in packs of 4 before going to the market. How many packs are there?

Here, students should use the fact that 4 x 12 = 48 and as 4,800 is a hundred times more than 48, 12 must also by multiplied by 100 to get 1,200

Division: Reasoning and problem solving Year 4

Is it always, sometimes or never true that the sum of three even numbers is divisible by 4?

This question gives students ample opportunity to experiment within the parameters of the question. Students who need more support can look at single digit even numbers and students could go up to whatever they feel comfortable with.

With open questions such as this, it is good to provide a minimum requirement for how many attempts students should make, otherwise they will often choose to do the bare minimum.

A quick ‘trick’ to know if a number is divisible by 4 is to look at the last 2 digits of the number and see if those are divisible by 4.

For example, if a child found the sum of 34, 36 and 38 and got 108, as a teacher I know that this number is divisible by 4 as the final two digits (08) make 8 and are divisible by 4. If a child chose 34, 36 and 48 to get 118, I know that this is not divisible by 4 as 18 – the final two digits of 118 – are not divisible by 4.

Division Year 5

In the national curriculum for maths in England, for each area of maths outlined, there is both a statutory element and a non-statutory element. The statutory element is as follows:

• Multiply and divide numbers mentally drawing upon known facts.
• Divide numbers up to 4 digits by a one-digit number using the formal written method of short division and interpret remainders appropriately for the context.
• Multiply and divide whole numbers and those involving decimals by 10, 100 and 1000.
• Solve problems involving multiplication and division including using their knowledge of factors and multiples, squares and cubes.
• Solve problems involving addition, subtraction, multiplication and division and a combination of these, including understanding the meaning of the equals sign. 
• Solve problems involving multiplication and division, including scaling by simple fractions and problems involving simple rates. 

Non-statutory notes and guidance: 

• Pupils interpret non-integer answers to division by expressing results in different ways according to the context, including with remainders, as fractions, as decimals or by rounding (for example, 98 ÷ 4 = 24 r 2 = 24 = 24.5 ≈ 25).
• Pupils use multiplication and division as inverses to support the introduction of ratio in year 6, for example, by multiplying and dividing by powers of 10 in scale drawings or by multiplying and dividing by powers of a 1,000 in converting between units such as kilometres and metres.

Division: Lesson ideas Year 5

Division in Year 5 progresses through the use of remainders in answers. For this activity, I will look at another use of manipulatives that teachers can use to teach 4 digit by 1 digit division.

If the question was 5,642 divided by 4, we could represent this using a place value chart and place value counters as we can see below.

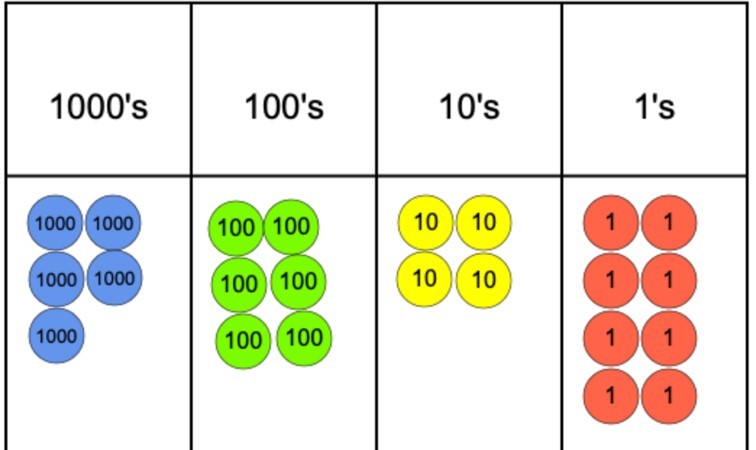
The next step would be to begin to group the counters into 4 as this is our divisor. However, as can be seen, we quickly come to a problem in the 1000’s column as we are able to group 4 of the counters but then we cannot group the remaining counter in a group of 4.

To combat this issue, the 1000 counter that has not been grouped can be exchanged for ten 100 counters. These can then be grouped into groups of 4 and this can happen without there being any 100s counters left over. From there, it is a simple case of then moving to the 10s and finally the 1s. 

As there are nine 1s counters, we can group 8 of them into 2 groups of 4 and claim that there is one left over*. To find the answer, all that is required is to count the number of groups made in each place value and use these to write the digits to create the final answer. In this case, the answer would be 1,412 remainder 1. 

*When you are looking at decimals, you can exchange the remaining 1s counter for ten 0.1 counters and carry on the division as described above. Students being familiar with this model is useful as it can help to demystify division with decimals which students can struggle with. This helps them see that it is a continuation of a process that they are already familiar with rather than something new.

Division: Word problems Year 5

When dividing in Year 5, students will come across scenarios where they will need to round their answer up in order for the answer to make sense in the context. An appropriate question that demonstrates this would be something like the following:

A school is planning a disco where they plan on offering 250ml of juice to each student. They can buy 2 litre bottles of juice. They are expecting 33 students to arrive so know that they will give out 8.25 litres of juice. How many bottles of juice will they need to buy?

A common answer is that students will say 4 and a quarter. However, as you cannot buy a quarter of a bottle, in this instance, the correct answer would be 5 bottles of juice would be needed to ensure that all students could partake. 

Division: Reasoning and problem solving Year 5

Getting students to create their own problems using a range of provided context can be a really challenging activity that gets the students thinking mathematically about context and division.

Students can then swap these questions they have created with a partner and then go about solving them and checking with the original question creator if they have the correct answer. 

Division Year 6

In the national curriculum for maths in England, for each area of maths outlined, there is both a statutory element and a non-statutory element. The statutory element is as follows:

• divide numbers up to 4 digits by a two-digit whole number using the formal written method of long division, and interpret remainders as whole number remainders, fractions, or by rounding, as appropriate for the context.
• divide numbers up to 4 digits by a two-digit number using the formal written method of short division where appropriate, interpreting remainders according to the context. 
• perform mental calculations, including with mixed operations and large numbers. 
• identify common factors, common multiples and prime numbers. 
• use their knowledge of the order of operations to carry out calculations involving the four operations. 

Non-statutory notes and guidance: 

• Solve problems involving addition, subtraction, multiplication and division.
• Use estimation to check answers to calculations and determine, in the context of a problem, an appropriate degree of accuracy. 

Division: Lesson Ideas Year 6

Sophie Bartlett (@_MissieBee) has created an excellent blog on teaching long division, detailing how she would teach long division for a year 6 class. As long division is the only new learning on division for Year 6, this is an ideal way to introduce the procedure of long division. 

Read more: Long Division Examples

Division: Word problems Year 6

A typical division word problem that a Year 6 student would come across would be:

A school raised £3,146 from a bake sale. 104 parents attended the event. If all parents spent an equal amount of money, how much did each parent spend?

Here, students would be expected to use the long division method to solve this question to get the answer £30.25.

Division: Reasoning and problem solving Year 6

With something procedural like long division, a good reasoning activity is to get students to find mistakes. These resources provide a large bank of such activities. As we can see from the question below, the student has done all the hard work but a careless error in counting the groups of 59 mean that the child believed 59 x 7 was 472 and not 59 x 8.

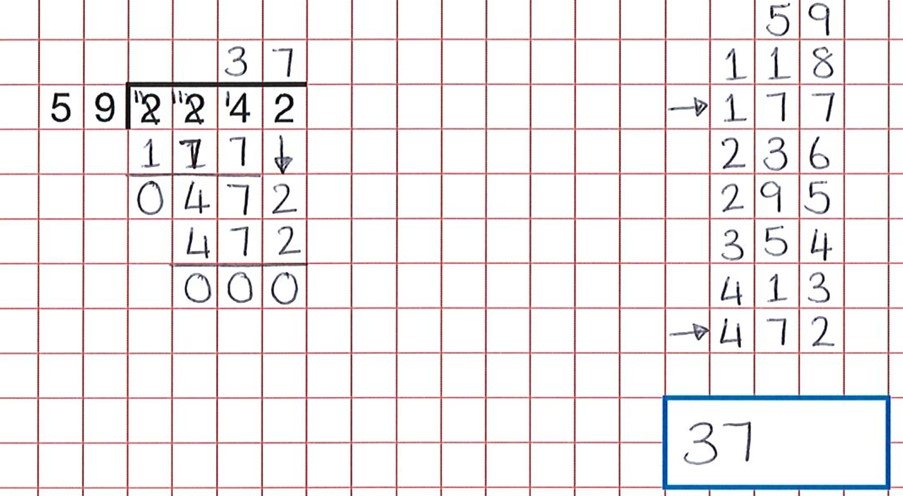
The correct answer would have been 38.

Division is a key maths topic, not just at KS2 but in maths as a whole. Hopefully this post has given you some good ideas to help your pupils approach it with confidence.

See also:

• Division Questions That Will Test Your Primary School Pupils
• Long Division Questions That Will Test All Pupils From Year 6 To Year 11
• Partitioning Explained For Primary School Parents And Kids
• How To Teach Division KS2: Maths Bootcamp