What Is A Prime Number? Explanation For Primary School Teachers, Parents & Children
A prime number is a number that can only be divided by itself without remainders. In this guide, we will explain what exactly this means, give you a list of prime numbers children need to know at primary school, and provide you with some practice questions to find prime numbers.
What is a prime number?
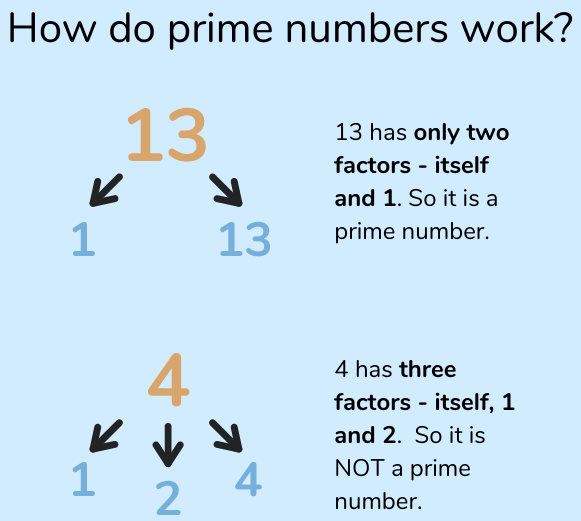
A prime number is a whole number greater than 1 with only two factors – itself and 1.
A prime number cannot be divided by any other positive integer without leaving a remainder, decimal or fraction.
Prime numbers are often seen as ‘building blocks’ by mathematicians in number theory. The fundamental theorem of arithmetic states that a composite number can be expressed as the product of prime numbers.
Examples of prime numbers
An example of a prime number is 13. That is because its only divisors are 1 and 13.
Dividing a prime number by another natural number results in numbers left over e.g. 13 ÷ 6 = 2 remainder 1.
15 is not an example of a prime number because it can be divided by 5 and 3 as well as by itself and 1. 15 is an example of a composite number because it has more than two factors.
Example of a Third Space Learning Year 5 prime numbers lesson slide

See also: Divisibility Rules
Prime Numbers Worksheets
FREE worksheets containing 29 prime numbers questions and answers for Year 5 and Year 6!
Download Free Now!What are the prime numbers?
Here is how to work out if a given number is a prime number or not, based on the properties of prime numbers.
What are the prime numbers?
- There are 8 prime numbers under 20: 2, 3, 5, 7, 11, 13, 17 and 19.
- The first 10 prime numbers are 2, 3, 5, 7, 11, 13, 17, 19, 23, 29.
- There are 25 prime numbers between 1 and 100.
- Prime numbers include large numbers and can continue well past 100.
- For example, 21,577 is a prime number.
List of prime numbers to 100
2, 3, 5, 7, 11, 13, 17, 19, 23, 29, 31, 37, 41, 43, 47, 53, 59, 61, 67, 71, 73, 79, 83, 89, 97
Notice that this list of prime numbers contains only odd numbers, apart from 2.
What is the smallest prime number?
2 is the smallest prime number. It also the only even prime number – all other even numbers can be divided by themselves, 1 and 2 at least, meaning they will have at least 3 factors.
What is the largest prime number?
Greek mathematician Euclid (one of the most famous mathematicians of the classical era), recorded a proof that there is no largest prime number among the set of primes. However, many scientists and mathematicians are still searching to find it as part of the Great Internet Mersenne Prime Search. The sieve of Eratosthenes formula is used, which is an ancient Greek algorithm for finding all prime numbers.
The largest known prime number (as of November 2020) is 282,589,933 − 1, a number that has 24,862,048 digits when written in base 10. Before then the largest known prime number was 277,232,917 − 1, having 23,249,425 digits.
By the time you read this, it might be even larger, but you can follow its progress on Wikipedia.
Common FAQs about prime numbers
What is a prime number in maths?
A prime number is a number that can only be divided by itself and 1 without remainders.
What are the prime numbers from 1 to 100?
The prime numbers from 1 to 100 are: 2, 3, 5, 7, 11, 13, 17, 19, 23, 29, 31, 37, 41, 43, 47, 53, 59, 61, 67, 71, 73, 79, 83, 89, 97.
Why is 1 not a prime number?
1 is not a prime number because it has only one factor, namely 1. Prime numbers need to have exactly two factors.
Why is 2 a prime number?
2 is a prime number because its only factors are 1 and itself.
Is 51 a prime number?
51 is not a prime number because it has 3 and 17 as divisors, as well as itself and 1. In other words, 51 has four factors.
Prime numbers in primary school
Prime numbers are not introduced in the UK until Year 5, along with ratios and square roots.
According to the national curriculum, Year 5 children should be taught to “know and use the vocabulary of prime numbers, prime factors and composite (non-prime) numbers to establish whether a number up to 100 is prime and recall prime numbers up to 19”.
In Year 6, children should be able to “identify common factors, common multiples and prime numbers”.
How are prime numbers used in the real world?
One of the most important uses for prime numbers is in cyber security – making information shared over the internet safer.
In order to encrypt (make secure) things like credit card details, medical records, and even some messaging services like WhatsApp, software engineers make algorithms using prime numbers.
By multiplying two very large prime numbers together (some companies use prime numbers that are hundreds of digits long!), we create an even larger number whose original factors (the two very large prime numbers) are only known to us. We then use this even larger number to encrypt our information.
If anyone else wants to discover what information we are sending, they have to find out what our original factors were. With prime numbers as long as the ones we have used, it could take them years or even decades of constant trial and error before they find even one. This kind of public-key cryptography ensures our information is kept safe.
Wondering how to explain other key maths vocabulary to your children? Check out our Primary Maths Dictionary, or try these primary maths terms:
Prime number questions
1) A square number and a prime number have a total of 22. What are the two numbers?
A: 9 and 13
2) Emma thinks of two prime numbers. She adds the two numbers together. Her answer is 36. Write all the possible pairs of prime numbers Emma could be thinking of.
A: 5 and 31, 7 and 29, 13 and 23, 17 and 19
3) Circle the two prime numbers – 29, 59, 39, 69, 29
A: 29 and 59
4) Write the three prime numbers which multiply to make 231.
A: 3 x 7 x 11
CHALLENGE QUESTION: Chen chooses a prime number. He multiplies it by 10 and then rounds it to the nearest hundred. His answer is 400. Write all the possible prime numbers Chen could have chosen.
A: 37, 41 or 43.
All of our resources for primary schools can be found in the Third Space Maths Hub
Create your own free Maths Hub account to access hundreds of free resources, guides and CPD videos. Premium Maths Hub access includes 36 weeks of Fluent in Five, Rapid Reasoning and Ready To Go Lesson Slides for years 1 to 6. It is available through a whole-school subscription or is included at no additional cost for schools using our online one to one maths tutoring.
Register free for the Third Space Maths Hub.
Enquire about Premium Maths Hub
DO YOU HAVE PUPILS WHO NEED MORE SUPPORT IN MATHS?
Every week Third Space Learning’s specialist primary maths tutors support thousands of students across hundreds of schools with weekly online 1 to 1 maths lessons designed to plug gaps and boost progress.
Since 2013 these personalised one to one lessons have helped over 150,000 primary and secondary students become more confident, able mathematicians.
Learn about the scaffolded lesson content or request a personalised quote for your school to speak to us about your school’s needs and how we can help.





