What Is An Improper Fraction: Explained for Primary School

Improper fractions and mixed numbers (a whole number with a fractional part) are introduced once children are secure in their understanding of proper fractions in Upper KS2. 

What is an improper fraction?

There are two main types of fractions: proper fractions, see fractions for kids (which include unit fractions and non-unit fractions) and improper fractions.

An improper fraction, sometimes also called a top-heavy fraction, is a number greater than one whole. The numerator of the fraction (top number) is therefore greater than the denominator of the fraction (bottom number). 

Examples of improper fractions

4⁄3, five halves, and 927⁄4 are all examples of improper fractions. Some improper fractions are actually equivalent to a whole number, such as 4⁄2 (four halves is the same as two wholes), but it can still be represented as an improper fraction. All whole numbers above 1 can be represented as improper fractions (e.g. 2 is the same as 4⁄2, which is an equivalent fraction to 6⁄3 and 16⁄8; 10 is the same as 70⁄7, 120⁄12, or 530⁄53).

When do children learn about improper fractions in the national curriculum?

Children first encounter improper fractions in UKS2. In Year 5, pupils must “recognise mixed numbers and improper fractions and convert from one form to the other and write mathematical statements > 1 as a mixed number [for example, 2⁄5 + ⅘ = 6⁄5 = 1 and ⅕]”. Whilst they are not explicitly referred to again in the NC, a solid understanding of improper fractions is beneficial when attempting more complex maths in Year 6.

How do improper fractions relate to other areas of maths?

Besides coming alongside mixed numbers (also known as mixed fractions), or perhaps when identifying decimal equivalents, children are unlikely to come across improper fractions in any other areas of maths. Some improper fractions may require simplifying to the lowest term, e.g. 10⁄4 = 5⁄2.

Improper fractions in real life

Mixed numbers are encountered more often in real life contexts and can have everyday use, more so than improper fractions. However, sometimes it may be useful to think of ideas in terms of improper fractions first: for example, if you’re holding a party for 13 people, and each person would eat half a pizza, you would need 13 equal pieces (or 13⁄2) of a pizza – which is in its simplest form, 6½ (the number of whole pizzas plus the fractional part). 

Worked examples for improper fractions

To convert an improper fraction to a mixed number, divide the numerator (in a division, this is also known as the dividend) by the denominator (also known as the divisor). The answer to this (also known as the quotient) becomes the whole number; the remainder (if there is one) becomes the numerator; the denominator (which was the divisor) remains the same.

For example, to convert 23⁄5 to a mixed number, step-by-step:

1. Divide 23 by 5.
2. 5 fits into 23 4 whole times, so the whole number is 4.
3. There is a remainder of 3, so the new numerator is 3 in the fraction part of the mixed number (the denominator remains the same as the original improper fraction). The answer is therefore 4⅗. 

This can be much more clearly visualised with a diagram such as a bar model:

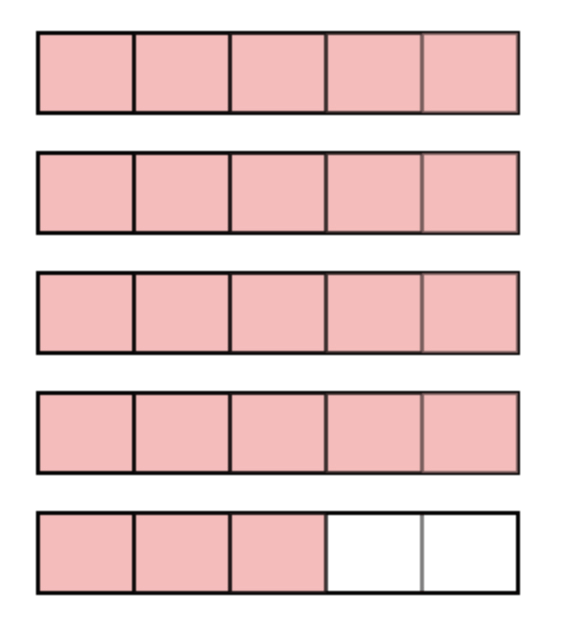
As shown above, twenty-three fifths can also be written as four wholes and three-fifths.

To convert a mixed number to an improper fraction, multiply the whole number by the denominator and add the numerator. The answer to this becomes the new numerator; the denominator remains the same. 

For example, to convert 2⅔ to an improper fraction step-by-step:

1. 2 (whole number) x 3 (denominator) = 6
2. 6 + 2 (numerator) = 8 (the new numerator). The answer is therefore 8⁄3.

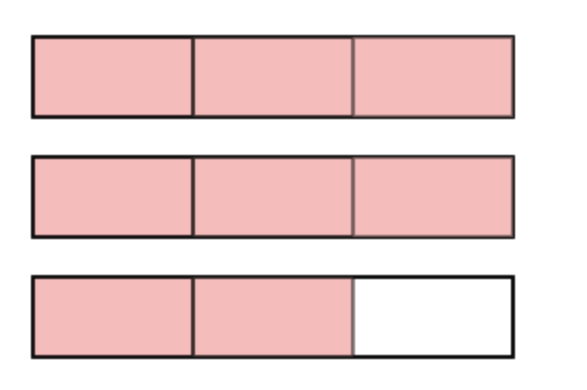
As shown above, two wholes and two-thirds can also be written as eight-thirds. 

As previously mentioned, whilst not explicitly referenced in the NC, converting to improper fractions may be useful when adding, subtracting and multiplying mixed numbers. 

5 Improper fractions practice questions and answers

1. Which improper fraction is equivalent to 6⅞?
   67⁄8     48⁄8     62⁄8     55⁄8     76⁄8     (2018 SATs)
   Answer: 55⁄8 
2. Convert 17⁄3 to a mixed number.
   Answer: 5⅔
3. Convert 2 and 5⁄9 to an improper fraction.
   Answer: 23⁄9
4. Which is the odd one out?
   2¾     23⁄4     11⁄4     1 + 7⁄4     3 – ¼
   Answer: 23⁄4 because the rest are all equivalent to 2¾
5. Which mixed number is equivalent to 37⁄6?
   3 and 6⁄7 6⅙    6 and 6⁄7     55⁄8     7 and 3⁄6
   Answer: 6⅙

For more explanations of primary maths topics, take a look at Third Space Learning’s Primary Maths Dictionary for Kids and Parents: A Guide To The Mathematical Terms Used In KS1 And KS2

Do you have pupils who need extra support in maths?
Every week Third Space Learning’s maths specialist tutors support thousands of pupils across hundreds of schools with weekly online 1-to-1 lessons and maths interventions designed to plug gaps and boost progress.

Since 2013 we’ve helped over 150,000 primary and secondary school pupils become more confident, able mathematicians. Learn more or request a personalised quote for your school to speak to us about your school’s needs and how we can help.

Primary school tuition targeted to the needs of each child and closely following the National Curriculum.