One to one maths interventions built for KS4 success

Weekly online one to one GCSE maths revision lessons now available

Learn more

In order to access this I need to be confident with:

Arithmetic Negative numbers

This topic is relevant for:

GCSE Maths Number Powers And Roots

Cube Numbers And Cube Roots

Cubes And Cube Roots

Here we will learn about cube numbers and cube roots including what a cube number is, what a cube root is, as well as how to cube a number and how to find the cube root of an integer. You’ll also learn how to solve problems by applying knowledge of cube numbers.

There are also cube numbers and cube roots worksheets based on Edexcel, AQA and OCR exam questions, along with further guidance on where to go next if you’re still stuck.

What is a cube number?

A cube number is found when we multiply an integer (whole number) by itself and then itself again. These are sometimes called ‘perfect cubes’.

We can cube numbers with decimals but we do not refer to these as cube numbers.
A given number / variable that is ‘cubed’ is multiplied by itself three times.

E.g.
4 × 4 × 4 can be written as 4³ and is spoken as “4 cubed” or “4 to the power of 3”

A cube number is found when we multiply an integer (whole number) by itself,

Here are the first 10 cube numbers:

\begin{align*} 1 \times 1 \times 1 &=1 \quad \quad \quad \quad \quad \quad \quad \quad \quad \text { Therefore } 1 \text { is a cube number } \\\\ 2 \times 2 \times 2&=8 \quad \quad \quad \quad \quad \quad \quad \quad \quad \text { Therefore } 8 \text { is a cube number } \\\\ 3 \times 3 \times 3&=27 \; \; \quad \quad \quad \quad \quad \quad \quad \quad \text { Therefore } 27 \text { is a cube number } \\\\ 4 \times 4 \times 4&=64 \; \; \quad \quad \quad \quad \quad \quad \quad \quad \text { Therefore } 64 \text { is a cube number } \\\\ 5 \times 5 \times 5&=125 \; \quad \quad \quad \quad \quad \quad \quad \quad \text { Therefore } 125 \text { is a cube number } \\\\ 6 \times 6 \times 6&=216 \; \quad \quad \quad \quad \quad \quad \quad \quad \text { Therefore } 216 \text { is a cube number } \\\\ 7 \times 7 \times 7&=343 \; \quad \quad \quad \quad \quad \quad \quad \quad \text { Therefore } 343 \text { is a cube number } \\\\ 8 \times 8 \times 8&=512 \; \quad \quad \quad \quad \quad \quad \quad \quad \text { Therefore } 512 \text { is a cube number } \\\\ 9 \times 9 \times 9&=729 \; \quad \quad \quad \quad \quad \quad \quad \quad \text { Therefore } 729 \text { is a cube number } \\\\ 10 \times 10 \times 10&=1000 \quad \quad \quad \quad \quad \quad \quad \quad \text { Therefore } 1000 \text { is a cube number } \end{align*}

A cube number can be represented as an array which forms the shape of a cube that has a length 3 units, width 3 units and depth 3 units.

E.g.

If we look at 3³, this is 3 × 3 × 3

Any cube number will form the shape of a cube.

What is a cube number?

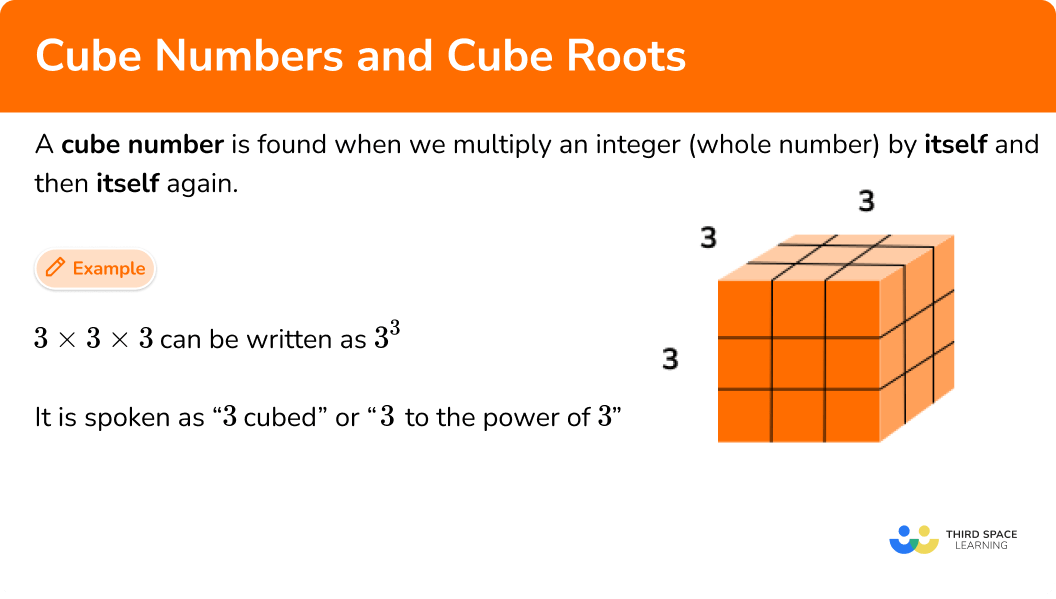
Perfect cubes

The cube number of an integer is also called a perfect cube.

We can cube numbers with decimal places but we do not refer to these as cube numbers or perfect cubes.

A given number or variable that is ‘cubed’ is multiplied by itself and this applies to decimals as to integers.

It’s relatively straightforward to work out the cube root of a perfect cube but it is a much harder process to work out decimal cube roots. You quickly end up working with surds and irrational numbers.

Cubing negative numbers

We can also cube negative numbers.
E.g.

\begin{array}{ll} (-5) \times(-5) \times(-5)=-125 & \quad \therefore(-5)^{3}=-125 \\\\ (-7) \times(-7) \times(-7)=-343 & \quad \therefore(-7)^{3}=-343 \end{array}

You will notice that when we cube a negative number we get a negative number.
This is because a negative number multiplied by a negative number multiplied by a negative number gives us a negative result.
Learn more by reviewing our lesson on negative numbers.

When we cube negative 5 we get the negative answer of the cube of positive 5.
This is true for all numbers (and variables) and means:

\[(5)^{3} \neq(-5)^{3}\]

What is a cube root?

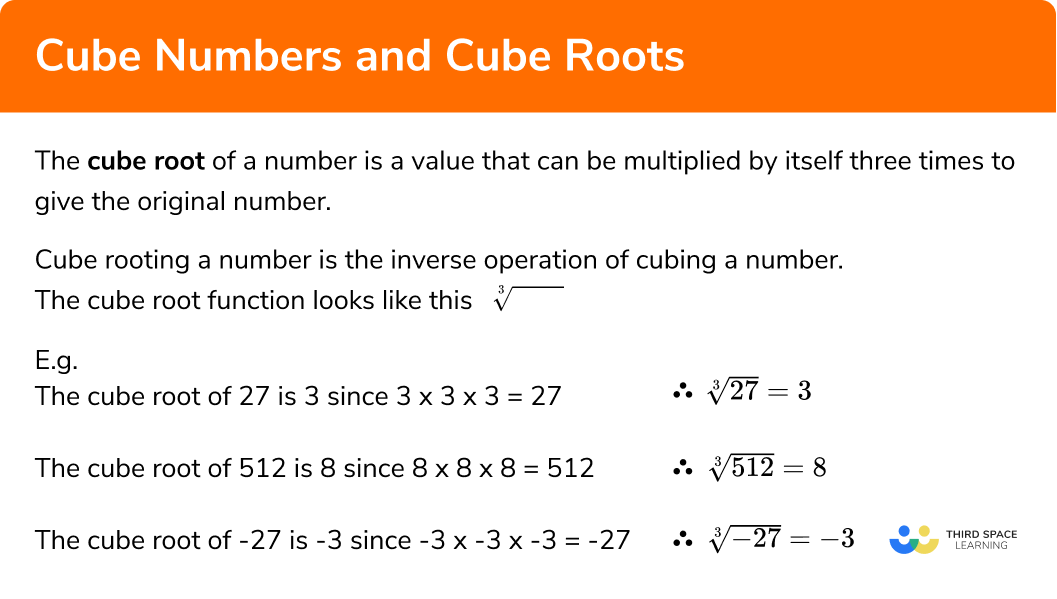
The cube root of a number is a value that can be multiplied by itself three times to give the original number.
A cube root is the inverse operation of cubing a number.
The cube root symbol looks like this ³√

When we cube a positive number we get a positive result and when we cube a negative number we get a negative result.
So the cube root of a positive number is also a positive number, and the cube root of a negative number is also a negative number.

E.g.

\begin{array}{l} \text { As } 3^{3}=27 \text { the cube root of } 27 \text { is } 3 & \quad \therefore \sqrt[3]{27}=3 \\\\ \text { As } 8^{3}=512 \text { the cube root of } 512 \text { is } 8 & \quad \therefore \sqrt[3]{512}=8 \\\\ \text { As} (-3)^{3}= -27 \text { the cube root of} -27 \text { is} -3 & \quad \therefore \sqrt[3]{-27}=-3 \end{array}

What is a cube root?

Cube numbers worksheet

Get your free cube numbers worksheet of 20+ questions and answers. Includes reasoning and applied questions.

DOWNLOAD FREE

Cube numbers worksheet

Get your free cube numbers worksheet of 20+ questions and answers. Includes reasoning and applied questions.

DOWNLOAD FREE

Related lessons on powers and roots

Cube numbers and cube roots is part of our series of lessons to support revision on powers and roots. You may find it helpful to start with the main powers and roots lesson for a summary of what to expect, or use the step by step guides below for further detail on individual topics. Other lessons in this series include:

Key words

Term

A single number (constant) or variable

E.g.
in the expression 4x − 7 both 4x and − 7 are terms

Coefficient

The number which the variable is being multiplied by

E.g.
in 2x³ the coefficient is 2

Integer

A whole number

E.g.
1, 7 or 1003

Index (also called exponent or powers)

The index number is the amount of times you multiply a number/variable by itself.

E.g.
the index number in 5³is 3

Note: the plural of index is indices

Note: you will see index number as a superscript

Base number

The number/unknown that is being multiplied by itself an amount of times

E.g.
the base number in 5³is 5 and in 2x³ the base number is x

Advanced vocabulary – only for Additional Maths, A-Level

Real numbers

Any positive or negative number is called a real number. Numbers that are not ‘real’ are called imaginary numbers. Integers, decimals, fractions are all examples of real numbers

E.g.
1, 2, 5 and 100 are all examples of a positive real numbers

-1, -2, -50 and -65.67 are all examples of a negative real number

Imaginary numbers

Numbers that are not real are called imaginary numbers, for example you will notice we cannot find the square root of a negative number (try it on a calculator), this because it is an imaginary number.. Numbers that contain an imaginary part and real part are called complex numbers.

Cube numbers and cube roots examples

Example 1

What is 5 cubed?

5 cubed means 5 × 5 × 5

So 5 cubed is 125

Example 2

What is 9 cubed?

9³ cubed means 9 × 9 × 9

So 9 cubed is 729

Example 3

What is the cube root of 64?

The cube root of 64 means what value can be multiplied by itself three times to give 64

\[\sqrt[3]{64} =4\]

How to use cube numbers and cube roots

Identify whether you need to cube or cube root the number/variable
Perform the operation
Clearly state the answer within the context of the question e.g. including units

Now you will focus on solving problems using your knowledge of cube numbers and cube roots.

Explain how to solve problems involving cube numbers and cube roots in 3 steps

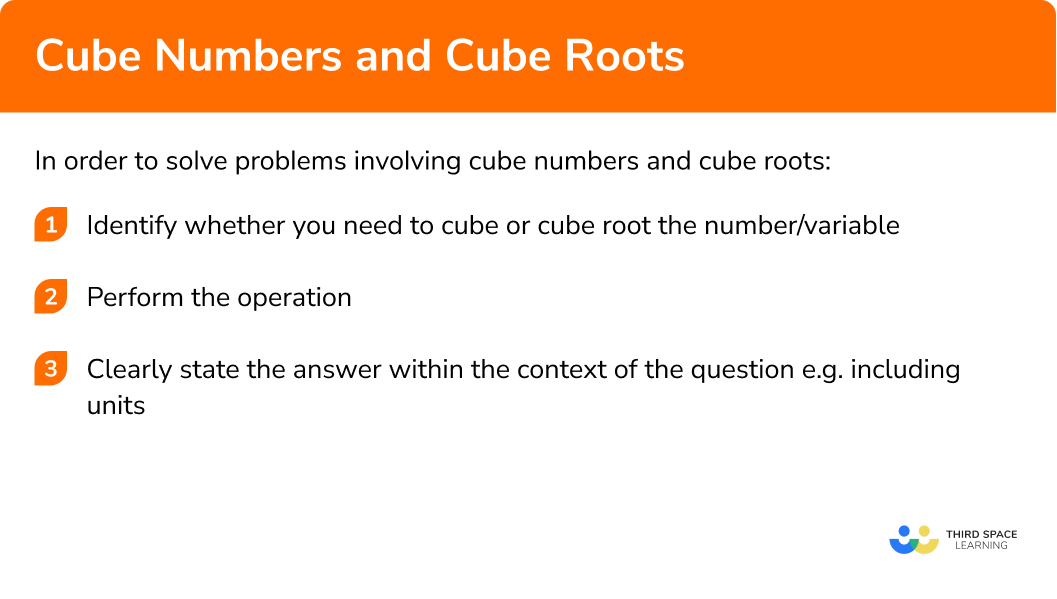
Cubes and cube roots problem examples

Example 4: knowledge of cube numbers

Danny says 2 cubed is 6. Why is Danny wrong? What mistake did he make?

Identify whether you need to cube or cube root the number/variable

The question focus is on cubing 2 or “2 cubed”

2 Perform the operation

\begin{array}{l} 2 \times 2 \times 2 =2^{3} =8 \end{array}

3 Clearly state the answer within the context of the question

Danny is wrong because 2 cubed is 8 not 6.

The mistake Danny made was he did ‘2 × 3’ not ‘2³’.

Example 5: problem solving with cube roots

Ava says the cube root of an integer is always smaller than the original number. Prove Ava is incorrect.

Identify whether you need to cube or cube root the number/variable

You are looking for a relationship between the cube root of an integer and the original number. It will help to write down your cube numbers and their cube roots.

Perform the operation

\begin{array}{l} \sqrt[3]{1000}=10 \\\\ \sqrt[3]{729}=9 \\\\ \sqrt[3]{512}=8 \\\\ \sqrt[3]{343}=7 \\\\ \sqrt[3]{216}=6 \\\\ \sqrt[3]{125}=5 \\\\ \sqrt[3]{64}=4 \\\\ \sqrt[3]{27}=3 \\\\ \sqrt[3]{8}=2 \\\\ \sqrt[3]{1}=1 \end{array}

Clearly state the answer within the context of the question

Ava is incorrect because

\[\sqrt{1}=1\]

The answer here is not smaller than the original number.

Example 6: solving problems involving cube numbers

The sum of two cube numbers is 72. Find the two cube numbers.

Identify whether you need to cube or cube root the number/variable

Remember sum means add. Therefore you are looking for two cube numbers that add together to make 72.

It will help here to list the cube numbers up to 72

Perform the operation

The Cube Numbers: 1 8 27 64

You now need to pick two of these numbers that when added together make 72

Clearly state the answer within the context of the question

The two cube numbers are 64 and 8.

Example 7: cube numbers within a 3D polygon

A cube has a volume of 125mm³. What is the length of one side?

Identify whether you need to cube or cube root the number/variable

The volume of a cube is found by multiplying the length, width and height together. For a cube the length, width and height are the same length.

Therefore you are looking for a number that when multiplied by itself three times (or cubed) is equal to 125.

Therefore you need to find the cube root of 125

Perform the operation

\[\sqrt[3]{125}=5\]

Clearly state the answer within the context of the question

Therefore the length of the cube is 5mm.

Example 8: cube numbers within a 3D polygon

Length of one side of a cube is 4mm. What is the volume of the cube?

Identify whether you need to cube or cube root the number/variable

The volume of a cube is found by multiplying the length, width and height together. For a cube the length, width and height are the same length.

Therefore you are are going to multiply the side length by itself three or ‘cube’ the length.

Therefore you need to cube 4

Perform the operation

\[{4^3}=64\]

Clearly state the answer within the context of the question

Therefore the volume of the cube is 64mm³.

Example 9

Lexi says “when you add three consecutive cube numbers, the answer is always odd.”

Is Lexi right? Explain your answer.

Identify whether you need to cube or cube root the number/variable

To prove Lexi wrong we only need to find one example where she is incorrect, this is sometimes known as proof by contradiction.

Therefore we are looking for 3 consecutive cube numbers that when added together are even. It will be helpful here to list the ‘main’ cube numbers.

Perform the operation

The cube numbers:

\[1, \quad 8, \quad 27, \quad 64, \quad 125, \quad 216, \quad 343, \quad 512, \quad 729, \quad 1000 \]

You are now looking for one example where three of these numbers when added together make an even number.

For example: 1 + 8 + 27 = 36

Clearly state the answer within the context of the question

Lexi is wrong because 1 + 8 + 27 = 36

Common misconceptions

Cube numbers

Incorrect understanding of cubing a number

E.g.
3³ = 27 not 9

Cube roots

Not recognising that a negative number cube rooted is negative

E.g.
$\sqrt[3]-8 = -2$

Practice cube numbers and cube roots questions

30

100

1000

10000

10^{3}=10 \times 10 \times 10 = 1000

72

6

108

21

6 \times 6 \times 6 = 216 \quad \text{ therefore } \quad \sqrt[3]{216} = 6

343

-343

21

-21

(-7)^{3} = (-7) \times (-7) \times (-7) = -343

0

1

3

-1

(-1) \times (-1) \times (-1) = -1 \quad \text{ therefore } \quad \sqrt[3]{-1}=-1

$8$ and $27$

$8$ and $57$

$1$ and $64$

$27$ and $38$

The cube numbers up to $65$ are $1, 8, 27, 64.$

The two that add up to $65$ are $1$ and $64.$

$170.7$ cm

$8$ cm

$256$ cm

$5.12$ cm

We find the volume of a cube by multiplying the length, the width and the height together.

These are all equal for a cube so we need to find a number that, when multiplied by itself three times, gives us $512.$

Therefore we need to find the cube root of $512.$

\sqrt[3]{512}=8

Cube numbers and cube roots GCSE questions

1. Work out the value of:

(a) $3^{3}$

(b) $5 \times 2^{3}$

(3 Marks)

Show answer

(a) $3 \times 3 \times 3$

$27$

(1)

(b) $5 \times 8$

$40$

(1)

$189$

(1)

2. Here is a list of numbers:

1000 \quad \quad 18 \quad \quad 8 \quad \quad 64 \quad \quad 7 \quad \quad 144 \quad \quad 1 \quad \quad 19

(a) List the cube numbers

(b) Which number is a square and cube number?

(2 Marks)

Show answer

(a) $1000, 8 , 64$ and $1$

(1)

(b) $64$

(1)

3. Find the value of:

(a) $\sqrt[3]{729}$

(b) $\sqrt[3]{-1}$

(3 Marks)

Show answer

(a) $9$

(1)

(b) $-1$

(1)

4. Simplify the following expression

5^{3} \times \sqrt[3]{x^{3}}

(2 Marks)

Show answer

125 x

Correct coefficient

(1)

Correct $x$ (or $x^{1}$ )

(1)

Learning checklist

You have now learned how to:

Calculate cube numbers up to 10 × 10 × 10

Use positive integer powers and their associated real roots

Recognise and use the cube numbers

Apply properties of cubes to a context

The next lessons are

Still stuck?

Prepare your KS4 students for maths GCSEs success with Third Space Learning. Weekly online one to one GCSE maths revision lessons delivered by expert maths tutors.