Converting fractions, decimals and percentages

One to one maths interventions built for KS4 success

Weekly online one to one GCSE maths revision lessons now available

Learn more

In order to access this I need to be confident with:

Equivalent fractions Ordering fractions Decimal number line To the power of

This topic is relevant for:

GCSE Maths Number FDP

Converting Fractions, Decimals and Percentages

Here we will learn about converting fractions, decimals and percentages, including how to define a fraction, a decimal and a percentage, convert between fractions, decimals and percentages and compare and order them.

There are also converting fractions, decimals and percentages worksheets based on Edexcel, AQA and OCR exam questions, along with further guidance on where to go next if you’re still stuck.

How to convert fractions, decimals and percentages

In order to compare fractions, decimals and percentages you need to be able to convert between them. On this page we will explore how to convert between fractions, decimals and percentages step by step with examples.

Here is a summary

To convert a fraction to a decimal divide the numerator by the denominator.
To convert a fraction to a percentage divide the numerator by the denominator and multiply by 100.
To convert a decimal to a percentage multiply the decimal by 100.
To convert a percentage to a decimal divide the percentage by 100.
To convert a percentage to a fraction divide the percentage by 100, write as a fraction, simplify the fraction
To convert a decimal to a fraction: write the decimal as a fraction by dividing by 1, convert the numerator to an integer (by multiplying by a multiple of 10), multiply the denominator by the same amount (to create an equivalent fraction), simplify the fraction.
To convert a recurring decimal to a fraction the calculation is more complex:

Assign the recurring decimal to an unknown value e.g x.
Multiply x (and the recurring decimal) by a base of 10 so you can eliminate the recurring part of the decimal by subtraction.
Subtract your values of x and respective recurring decimals.
Divide by the coefficient of x.
Simplify the fraction where possible.

Name 7 ways to convert between fractions, decimals and percentages

$Name 7 ways to convert between fractions, decimals and percentages$

Key facts about converting fractions, decimals & percentages

Fractions, decimals and percentages are different ways of expressing the same value

Fraction:

A fraction results from dividing one integer by another:
E.g.

\[\frac{1}{4} \]

means 1 ÷ 4, and is made up of a numerator (the top number) and a denominator (the bottom number).

The numerator refers to how many ‘parts’ we have and the denominator refers to how many ‘parts’ there are in total. So here we have 1 out of 4 parts.
We need to equate denominators when converting fractions.

When verbalising fractions we can refer to them in different ways:
E.g.

\[\frac{3}{8} \]

can be verbalised as “three out of eight” or “three eighths”.

Some fractions have specific names which you will already be familiar with:
E.g.

\[\frac{1}{4} \]

is known as “one quarter”.

Note: The line in a fraction is called the vinculum.

Note: Any value that can be written as a fraction is called a ‘rational number.’

Decimal:

Numbers containing a decimal point are often referred to as “decimals”.

E.g.
4.62 is a decimal number which can be read as “four point six two”

Note: The word decimals comes from the Latin decima meaning a tenth. This is why the numbers after the point represent ‘tenths’, ‘hundredths’ and so on.

Percentage:

A percentage represents a number out of 100 i.e. “per cent”.

E.g.
1% means 1 per 100,
55% means 55 per 100
122% means 122 per 100.

Note: The % symbol represents out of the 100. A much less common symbol is the ‘per mille symbol ‰ (out of 1000) and the ‘per then thousandth’ symbol ‱ *(out of 10000).

Converting Fractions, Decimals and Percentages

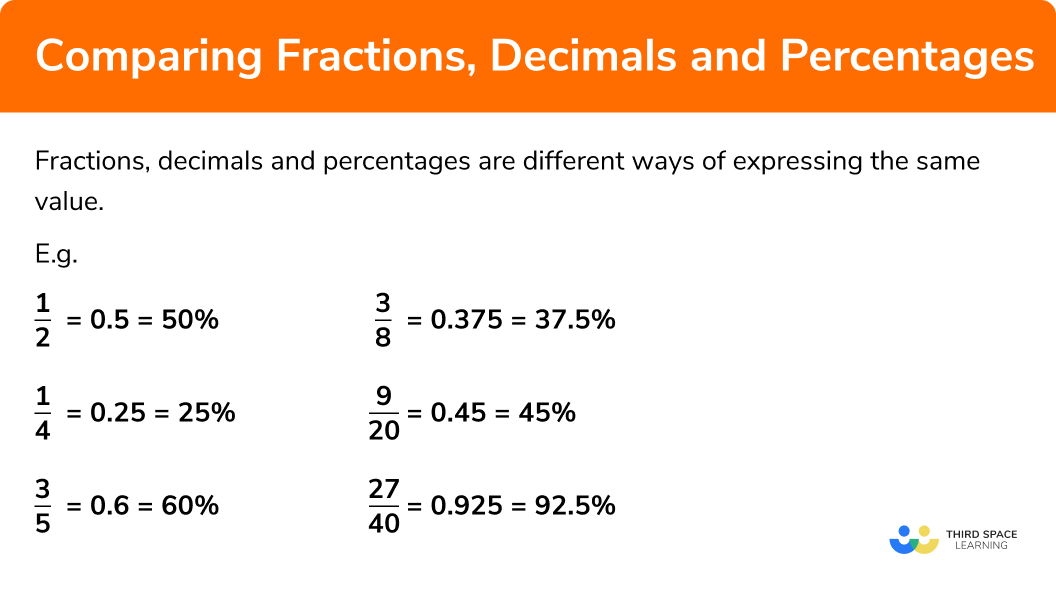
Listing in order fractions, decimals and percentages

Fractions, decimals and percentages are all equivalents of each other, so we can order them by converting them into the same form.

E.g.

Write these numbers in ascending order.

\[\frac{1}{4},\quad 0.19,\quad 0.3,\quad 26\%\,\quad \frac{1}{5}\]

Converting the fractions and decimals to percentages gives

\[\frac{1}{4}=25\%\,\quad 0.19=19\%\,\quad 0.3=30\%\,\quad \frac{1}{5}=20\%\]

Ascending means smallest to biggest, the smallest percentage is 19% = 0.19, the biggest percentage is 30% = 0.3.

In ascending order

\[\quad 0.19,\quad \frac{1}{5},\quad \frac{1}{4},\quad 26\%\,\quad 0.3\]

Let’s explore below how we can convert between fractions, decimals and percentages.

Converting fractions, decimals and percentages step by step

In order to compare fractions, decimals and percentages you need to be able to convert between them. Here we will explore step by step how to convert between the following:

Converting fractions to decimals.
Converting decimals to fractions.
Converting fractions to percentages.
Converting percentages to fractions.
Converting decimals to percentages.
Converting percentages to decimals.
Converting recurring decimals to fractions.

1. Convert a fraction to a decimal

In order to convert a fraction to a decimal:

Divide the numerator by the denominator.

E.g.

\[\frac{3}{4} \]

\[{3}\div 4\]

(Use a written method or a calculator)

\[{3}\div 4=0.75\]

Step by step guide: Fraction to decimals

2. Convert a decimal to a fraction

In order to convert a decimal to a fraction in its simplest form:

Write the decimal as a fraction by dividing by 1.
Convert the numerator to an integer (by multiplying by a multiple of 10).
Multiply the denominator by the same amount (to create an equivalent fraction).
Simplify the fraction.

E.g.

\begin{array}{l} 0.75 \\\\ =\frac{0.75}{1} \\\\ =\frac{0.75 \times 100}{1 \times 100} \\\\ =\frac{75}{100} \\\\ =\frac{75 \div 25}{100 \div 25} \\\\ =\frac{3}{4} \end{array}

Step by step guide: Decimals to fractions

3. How to convert a fraction to a percentage

In order to convert a fraction to a percentage:

Divide the numerator by the denominator.
Multiply by 100.

E.g.

\begin{array}{l} \frac{3}{4} \\\\ =3 \div 4 \\\\ =3 \div 4=0.75 \\\\ =0.75 \times 100 \\\\ =75 \% \end{array}

Or:

Convert the fraction so the denominator is 100 (not always possible).
Write as a percentage because it is ‘out of 100’.

E.g

\[\begin{array}{l} \frac{3}{4} \\\\ =\frac{75}{100} \\\\ =75 \% \end{array} \]

Step by step guide: Fractions to percentages

4. How to convert a percentage to a fraction

In order to convert a percentage to a fraction:

Divide the percentage by 100.
Write in fraction form.
Simplify the fraction if required.

E.g.

\begin{array}{l} 75 \% \\\\ =75 \div 100 \\\\ =0.75 \\\\ =\frac{75}{100} \\\\ =\frac{75 \div 25}{100 \div 25} \\\\ =\frac{3}{4} \end{array}

Step by step guide: Percentages to fractions

5. How to convert a decimal to a percentage

In order to convert a decimal to a percentage:

Multiply the decimal by a 100.

E.g.

\begin{array}{l} 0.75\\\\ =0.75 \times 100\\\\ =75 \% \end{array}

Step by step guide: Decimals to percentages

6. How to convert a percentage to a decimal

In order to convert a percentage to a decimal:

Divide the percentage by 100.

E.g.

\begin{array}{l} 75 \% \\\\ =75 \div 100 \\\\ =0.75 \end{array}

Step by step guide: Percentages to decimals

7. How to convert a recurring decimal to fraction

In order to convert a recurring decimal to fraction:

Assign the recurring decimal to an unknown value e.g x.
Multiply x (and the recurring decimal) by a base of 10 so you can eliminate the recurring part of the decimal by subtraction.
Subtract your values of x and respective recurring decimals.
Divide by the coefficient of x.
Simplify the fraction where possible.

E.g.

\begin{array}{l} 0.\dot{2}\dot{4} &= x \\\\ 24.\dot{2}\dot{4} &= 100x \\\\ 24 &= 99x \\\\ \frac{24}{99} &= x\\\\ \frac{8}{33} &= x \end{array}

Step by step guide: Recurring decimals to fractions

How to compare fractions, decimals and percentages

In order to compare fractions, decimals and percentages:

Convert all the values into the same form (i.e. fractions, decimals or percentages).
Note: Which one you convert to may differ based on the question and context.
– If working with fractions; convert so all fractions have a common denominator.
– If working with decimals; write all values in a vertical line ensuring the decimals places are aligned.
– If working with percentages; check they all are out of 100.
– If fractions compare the numerators (denominators must be equal).
– If decimals compare the units, tenths, hundredths etc.
– If percentages compare the value before the % sign.
Check you have answered the question e.g. are they in ascending order?
Write all values in their original form.

$Comparing fractions, decimals and percentages worksheet$

Comparing fractions, decimals and percentages worksheet

$Comparing fractions, decimals and percentages worksheet$

Get your free comparing fractions, decimals and percentage worksheet of 20+ questions and answers. Includes reasoning and applied questions.

DOWNLOAD FREE

$Comparing fractions, decimals and percentages worksheet$

Comparing fractions, decimals and percentages worksheet

$Comparing fractions, decimals and percentages worksheet$

Get your free comparing fractions, decimals and percentage worksheet of 20+ questions and answers. Includes reasoning and applied questions.

DOWNLOAD FREE

Key terminology for converting fractions, decimals and percentages

Integer
A whole number
E.g.
5 is an integer whilst 5.4 is not an integer.

Comparing
Examining the differences between two or more items/values.

Equivalent fraction
Fractions that are of the same value but shown differently
E.g.

\[\frac{2}{4}=\frac{1}{2}\]

Therefore,

\[\frac{2}{4} \quad \text{and} \quad \frac{1}{2}\]

are equivalent fractions.

Unit fraction
A fraction with 1 as its numerator is sometimes called a ‘unit fraction’:
E.g.

\[\frac{1}{7} \]

is a unit fractions because its numerator is 1.

Unlike denominators
Fractions with non-identical denominators are sometimes called unlike denominators. When comparing fractions we need a common denominator (sometimes called like denominators):
E.g.

\[\frac{1}{4} \quad \text{and} \quad \frac{1}{3}\]

are unlike denominators because the bottom numbers of each fractions are not the same.

Improper fraction
A fraction where the numerator is greater than the denominator
E.g.

\[\frac{7}{6} \]

Mixed number
A number made up of an integer and a fraction
E.g.

\[1 \frac{1}{6}\]

Convert
To change between one form and another
E.g.

\[50 \%=\frac{1}{2}=0.5\]

Recurring decimal
A decimal number with a digit (or group of digits) that repeats forever. The part that repeats can also be shown by placing dots over the first and last digits of the repeating pattern:
E.g.

\[\begin{aligned} &0 . \dot{3}=0.333… \\\\ &0 . \dot{2}\dot{4} = 0.24242424…\\\\ &0.\dot{1}23\dot{4} = 0.12342341234… \end{aligned}\]

Note: this are sometimes called repeating decimals

Ascending order
Increasing in value.

Descending order
Decreasing in value.

Converting and comparing fractions, decimals and percentages examples

Example 1: comparing fractions and decimals

Place these fractions and decimals in ascending order:

\[\frac{2}{3},\quad \quad 0.6,\quad \quad \frac{3}{6}\]

Convert all the values into the same form: fractions, decimals or percentages.

As the two of the values are all fractions so we can convert the single decimal to fraction form.

\[0.6\quad= \quad \frac{6}{10}\quad=\quad\frac{3}{5}\]

2Convert all the fractions so they have a common denominator.

As you are comparing fractions you need to find a common denominator for the three fractions, we can do this by finding the lowest common multiple of 3, 5 and 6. The lowest common multiple (lcm) of 3, 5 and 6 is 30.
Therefore the common denominator is 30.

Let’s now convert each fraction to have a denominator of 30:

\begin{array}{l} \frac{2}{3}=\frac{20}{30} \quad \quad \text{multiply the numerator and denominator by 10 } \\\\ \frac{3}{5}=\frac{18}{30} \quad \quad \text{multiply the numerator and denominator by 6 } \\\\ \frac{3}{6}=\frac{15}{30} \quad \quad \text{multiply the numerator and denominator by 5 } \end{array}

3Compare the numerators (denominators must be equal).

As the fractions now have a common denominator we can compare the different numerators:

\[\frac{20}{30}, \quad \quad \frac{18}{30}, \quad \quad\frac{15}{30}\]

4Check you have answered the question e.g. are they in ascending order?

The ascending order is:

\[\frac{15}{30}, \quad \quad \frac{18}{30}, \quad \quad \frac{20}{30}\]

5Write all values in their original form.

The fractions and decimals in ascending order are:

\[\frac{3}{6}, \quad \quad 0.6, \quad \quad \frac{2}{3}\]

Example 2: comparing decimals and percentages

Place these in descending order:

\[0.7, \quad 0.77, \quad 7\%, \quad 0.077\]

Convert all the values into the same form: fractions, decimals or percentages.

Three of the values are decimals and one is a percentage so we can convert the single percentage to a decimal.

\[ 7\%\quad=\quad 0.07\]

Write all values in a vertical line ensuring the decimals places are aligned.

The best way to compare decimals is to write all values in a vertical line ensuring the decimals places are aligned (see below):

\begin{aligned} &0 . 7\\\\ &0 . 77\\\\ &0 . 07\\\\ &0 . 077 \end{aligned}

Compare the units, tenths, hundredths etc.

Create a place value table and put each number in:

Units	Decimal Point	Tenths	Hundredths	Thousandths
0	.	7
0	.	7	7
0	.	0	7
0	.	0	7	7

Fill in the empty spaces with a zero:

Units	Decimal Point	Tenths	Hundredths	Thousandths
0	.	7	0	0
0	.	7	7	0
0	.	0	7	0
0	.	0	7	7

We can now easily compare the values.

Check you have answered the question

The descending order is:

\[0.770, \quad 0.700, \quad 0.077, \quad 0.070\]

Write all values in their original form.

The decimals and percentage in descending order are:

\[0.77, \quad 0.7, \quad 0.077, \quad 7\%\]

Example 3: comparing percentages and fractions

Place these in ascending order

\[\frac{14}{25}, \quad 54\%, \quad 5.4\%, \quad 56.4\%\]

Convert all the values into the same form: fractions, decimals or percentages.

Three of the values are percentages and one is a fraction so we can convert the single fraction to a percentage.

\[\frac{14}{25}\quad=\quad\frac{56}{100}\quad=\quad 56\%\]

With percentages; check they all are out of 100.

As all the percentages are shown with the % symbol it means they are all out of one hundred.

Compare the value before the % sign.

Because each percentage is out of 100 we can compare the value before the symbol.

\[56, \quad 54, \quad 5.4, \quad 56.4\]

We can see that 5.4 is the smallest value shown and 56.4 is the largest value shown

Check you have answered the question

The ascending order is

\[5.4, \quad 54, \quad 56, \quad 56.4\]

Write all values in their original form.

The ascending order is:

\[5.4\%, \quad 54\%, \quad\frac{14}{25}, \quad 56.4\%\]

Example 4: comparing fractions, decimals and percentages (simple)

Place these in ascending order:

\[0.5, \quad \frac{1}{3}, \quad 25 \%\]

Convert all the values into the same form: fractions, decimals or percentages.

The three values given are in different forms, you therefore need to write them in the same form. In this example we will use decimals as the common form but you could use fractions or percentages.

Let’s convert each value to a decimal

\begin{array}{l} \frac{1}{3} = 1 \div 3 = 0.333… = 0.\dot{3} \\\\ 25 \%=\frac{25}{100}=0.25 \end{array}

Write each out value

So the three values as decimals form are:

\[0.5, \quad 0.\dot{3}, \quad 0.25\]

Check you have answered the question

The values in ascending order are:

\[0.25, \quad 0.\dot{3}, \quad 0.5 \]

Write all values in their original form.

The values in ascending order are:

\[ 25 \%, \quad \frac{1}{3}, \quad 0.5,\]

Extra: try this questions again using fraction or percentages as your common form.

Example 5: comparing fractions, decimals and percentages

Place these in ascending order:

\[45.2 \%, \quad 0.463, \quad \frac{22}{50}, \quad \frac{4}{10}\]

Convert all the values into the same form: fractions, decimals or percentages.

The three values given are in different forms, you therefore need to write them in the same form. In this example we will use percentages as the common form but you could use decimals or fractions.

\begin{array}{l} 0.463=46.3 \% \\\\ \frac{22}{50}=\frac{44}{100}=44 \% \\\\ \frac{4}{10}=\frac{40}{100}=40 \% \end{array}

With percentages; check they all are out of 100.

As all the percentages are shown with the % symbol it means they are all out of one hundred.

Compare the value before the % sign.

Because each percentage is out of 100 we can compare the value before the symbol

\[45.2, \quad 46.3, \quad 44, \quad 40\]

We can see that 40 is the smallest value shown and 46.3 is the largest value shown

Check you have answered the question e.g. are they in ascending order?

The ascending order is:

\[40\%, \quad 44\%, \quad 45.2\%, \quad 46.3\%\]

Write all values in their original form.

The ascending order is:

\[\frac{4}{10}, \quad \frac{22}{50}, \quad 45.2 \%, \quad 0.463 \]

Extra: try this questions again using decimals or fractions as your common form

Common misconceptions

Converting

Incorrect conversion between fractions, decimals and percentages will result in the incorrect answer.

Comparing decimals

Not taking place value into account when comparing decimals will result in the incorrect answer.
E.g.
0.6 is larger than 0.072.

Comparing fractions

Not finding a common denominator when comparing and ordering fractions will result in the incorrect answer .

Ascending/descending

A common error is to confuse ascending and descending.

Practice comparing fractions, decimals and percentages questions

\frac{1}{2}

0.5

\frac{50}{100}

\frac{5}{100}

$\frac{5}{100}$ as a percentage is $5\%$ because $\%$ means out of $100$ .

Therefore $\frac{5}{100}$ ≠ $50\%$

\frac{2}{8}

\frac{25}{100}

4\%

0.25

$4\%$ is equal to $\frac{4}{100}$ which when simplified is $\frac{1}{25}$ ≠ $\frac{1}{4}$

\frac{2}{10}

2\%

\frac{20}{100}

0.200

$2 \%=\frac{2}{100}=0.02$ ≠ $0.2$

0.5

\frac{4}{6}

\frac{20}{30}

70\%

$70 \%=\frac{70}{100}=\frac{7}{10}=\frac{21}{30}$
$\frac{2}{3}=\frac{20}{30}$

Therefore, $\quad \frac{21}{30}>\frac{20}{30}$

Therefore, $\quad \frac{7}{10}>\frac{2}{3}$

Therefore, $\quad 70 \%>\frac{2}{3}$

0.3, \;\frac{31}{100}, \;32 \%

32\%, \;\frac{31}{100}, \;0.3

0.3, \;0.31, \;0.32

32 \%, \;0.3, \;\frac{31}{100}

\frac{31}{100} = 0.31\\

32 \% = 0.32 \\

0.3= 0.30\\

So the numbers in ascending order are $0.3, \frac{31}{100}, 32 \%$

0.89, \;\frac{8}{9}, \;90%

\frac{8}{9}, \;0.89, \;90 \%

90 \%, \;0.89, \;\frac{8}{9}

They are equivalent

\frac{8}{9} = 8 \div 9 = 0.888… = 0.\dot{8}\\

90\% = 0.90 \\

So the numbers in descending order are $90\%, 0.89, \frac{8}{9}$

Comparing fractions, decimals and percentages GCSE questions

1. Write these numbers in ascending order:

0.82, \quad \frac{4}{5}, \quad 85 \%, \quad \frac{2}{3}, \quad \frac{7}{8}

(3 marks)

Show answer

Attempt to convert all values to the same format with at least one conversion correct carried out.

(1)

Smallest and largest values:

$\frac{2}{3}$ – smallest

$\frac{7}{8}$ – largest

(1)

Correct order with all given in original form:

\frac{2}{3}, \quad \frac{4}{5}, \quad 0.82,  \quad 85 \%,  \quad \frac{7}{8}

(1)

2. Write these numbers in ascending order:

70 \%, \quad \frac{3}{4},\quad 0.6, \quad \frac{2}{3}

(3 marks)

Show answer

Attempt to convert all values to the same format with at least one conversion correct carried out.

(1)

Smallest and largest values:

$0.6$ – smallest

$\frac{3}{4}$ – largest

(1)

Correct order with all given in original form:

0.6, \quad \frac{2}{3}, \quad 70 \%, \quad \frac{3}{4}

(1)

3. Write these numbers in ascending order:

0.4, \quad \frac{7}{15},\quad 35 \%, \quad \frac{3}{7}

(3 marks)

Show answer

Attempt to convert all values to the same format with at least one conversion correct carried out.

(1)

Smallest and largest values:

$35 \%$ – smallest

$\frac{7}{15}$ – largest

(1)

Correct order with all given in original form:

$35 \%, \quad 0.4, \quad \frac{3}{7}, \quad \frac{7}{15}$

(1)

4. Jamaal received his scores for his recent tests:

– Art $\quad \quad \quad \quad \quad \quad \quad \frac{14}{25}$

– Biology $\;\quad \quad \quad \quad \quad 64 \%$

– German $\quad \quad \quad \quad \quad\; 49 \%$

– Sports Science $\quad \quad \quad \frac{25}{43}$

– Music $\quad \quad \quad \quad \quad \quad \frac{11}{15}$

– Physics $\quad \quad \quad \quad \quad\; 54 \%$

Arrange the subjects in order starting with the highest test score.

(3 marks)

Show answer

Attempt to convert all values to the same format. with at least one conversion correct carried out

(1)

Smallest and largest values:

German $49 \%$ – smallest

Art $\frac{14}{25}$ – largest

(1)

Correct order with all given (listed by subject):

Music, Biology, Sports Science, Art, Physics, German.

(1)

Learning checklist

You have now learned how to:

Order positive and negative integers, decimals and fractions.

Work interchangeably with terminating decimals and their corresponding fractions.

Ordering fractions, decimals and percentages.

Define percentage as ‘number of parts per hundred.

Work with percentages greater than 100%.

Compare fractions by using equivalent fractions.

Compare fractions with different denominators by finding a common denominator.

The next lessons are

Still stuck?

Prepare your KS4 students for maths GCSEs success with Third Space Learning. Weekly online one to one GCSE maths revision lessons delivered by expert maths tutors.