Teaching Geometry – Properties Of Shapes KS2: A Guide For Primary School Teachers From Year 3 To Year 6
In Key Stage 2, properties of 2-d shapes and properties of 3-d shapes are an important section of geometry. In this blog, Neil Almond provides his theories for teaching shape, help with lesson plans and plenty of teaching resources.
For some pupils, shape can be the topic they struggle with the most in KS2 maths, as it is so different to topics like place value which fall under Number. Even pupils who are fluent in numeracy may have difficulties up until upper KS2.
By looking back at the theory behind the subject and finding alternate ways to teach your lessons, both pupils who excel at shape and those who find it difficult will be able to find a deeper understanding.
Theory behind teaching geometry: properties of shapes
While there is no specific ‘theory’ as such to the teaching behind the property of shapes, there are some dos and don’ts that I feel are important from the outset, particularly in regard to resourcing for this subject. In too many classrooms, there will be a drawer labelled 2-d shapes that we believe to contain 2-d shapes like those below.

The issue here is that they are not 2-d. In the physical plane, where items can be manipulated, there exists exactly 3 dimensions. A piece of paper for example, despite its appearance, has a minuscule measurement of depth, and is therefore 3-d.
Every shape in that drawer is in fact a 3-d shape. Their purpose however is to represent 2-d shapes in an interactive way. Therefore, the teacher is left with two choices. Keep the label ‘2-d shapes’ but take out all the shapes and replace them with drawn shapes on a piece of paper or change the label to ‘3-d shapes used to represent 2-d shapes’.
The fact of the matter is that holding up one of these shapes and asking pupils to identify the 2-d shape you are holding is categorically wrong. Asking them what 2-d shape is represented by this 3-d shape is, however, perfectly permissible. It is for this reason that I recommend that 3-d shapes are taught before 2-d shapes.
When it comes to the lesson delivery of property of shape, leaning into variation theory can be a useful tool for educators to deploy. The application of variation theory is far more complicated and nuanced than even the best crafted worksheet could ever get across to pupils.
Rather, one of its possible uses involves the intellectual pursuit of skilful examples, where great care has been considered not only in the examples selected but the order in which they will be revealed..
It is very easy to get wrong but the rewards of getting variation theory correct are bountiful and shape, I believe, provides teachers who are interested in its application an easier route into how it works.
Those subscribed to Third Space Learning’s premium content can access a staff CPD powerpoint on variation theory and its application.
2D Shapes Worksheet Year 3
Download this FREE recognising 2D shapes Geometry worksheet for Year 3 pupils, from our Independent Recap collection.
Download Free Now!Geometry – properties of shapes KS2: Year 3
In the national curriculum for maths in England, for each area of maths outlined, there is both a statutory requirement and a non-statutory requirement. The statutory requirement is as follows:
- Draw 2-d shapes and make 3-d shapes using modelling materials; recognise 3-d shapes in different orientations and describe them
- Recognise angles as a property of shape or a description of a turn
- Identify right angles, recognise that 2 right angles make a half-turn, 3 make three-quarters of a turn and 4 a complete turn; identify whether angles are greater than or less than a right angle
- Identify horizontal and vertical lines and pairs of perpendicular and parallel lines
Unlimited maths tutoring for primary schools with Skye, our AI tutor
Built on the same principles, pedagogy and curriculum as our traditional tutoring but with more flexibility, reach and lower cost.
Join the schools already helping hundreds of primary pupils nationwide with Skye’s one to one maths tutoring
Find out moreThe non-statutory notes and guidance suggests:
Pupils’ knowledge of the properties of shapes is extended at this stage to symmetrical and non-symmetrical polygons and polyhedra. Pupils extend their use of the properties of shapes. They should be able to describe the properties of 2-d and 3-d shapes using accurate language, including lengths of lines and acute and obtuse for angles greater or lesser than a right angle.
Pupils connect decimals and rounding to drawing and measuring straight lines in centimetres, in a variety of contexts.

Properties of shapes Year 3 lesson ideas
It is here we can begin our first foray into what variation theory may look like in the classroom. To do this, we will examine the objective ‘identify horizontal and vertical lines and pairs of perpendicular and parallel lines.’
As has been a theme throughout this series, the objectives from the national curriculum do not equate to a lesson. It is quite right that objectives taken from the national curriculum are dissected into smaller, more manageable parts. Therefore, I will only be discussing the idea of parallel lines.
When thinking about the examples we will carefully select, it is best to consider the boundaries of the concept itself. By that, I mean when parallel lines are no longer parallel lines. In order to understand what parallel lines are, they must also know what they are not.
Secondly, we must also consider whether examples that do demonstrate the concept are shown and whether these are typical or not. For example, many pupils will have no issues in identifying the shape below as a square.

But will struggle to do the same for the following:

Often, this will be called a diamond despite it having all the properties of a square (the rotation of a shape does not alter the name of the shape). The reason for this struggle is that when ‘squares’ are shown to pupils they are often just shown the first example and not the second.
It is from these cues that we can build up standard and non-standard examples as well as non-examples to build up the concept of parallel lines. How best to introduce these examples is up to you and below are just some examples.
Standard example:

Non-example:

Standard example:
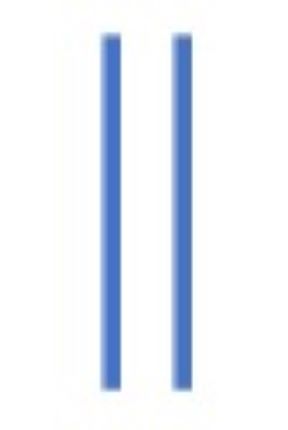
Non-example:

Non-standard example:

Already we can see here that the use of example and non-example demonstrates that parallel lines require more than one line.
Non-standard example:

Non-example:

Non-example:

Non-standard example:

Non-example:

When introducing each example, I would do so one at a time telling pupils whether each example does show parallel lines or not.
Once you have demonstrated a set that draws out differences, probe the pupils’ understanding of the examples with the questions ‘What is the same and what is different?’
The quality of conversation and the conjecturing that the pupils will make will be worth the time and effort put into constructing your sequence of examples and non-examples.
Properties of shapes Year 3 word problems
There would not be any standard ‘word problem’ that would look at the mathematics behind parallel lines that was so convoluted that it would hold any mathematical relevance.
Properties of shape Year 3 reasoning and problem solving
The most effective use of reasoning and problem solving when working with a concept and drawing on ideas of variation are sentence stems. For example, the following sentence stem could be used when looking at non-standard examples:
Despite the fact that______________, these are parallel lines because___________.

Despite the fact that these lines are not exactly vertical or horizontal, these are parallel lines because they are the same distance apart from each other and will never meet if extended.
Geometry – properties of shapes KS2: Year 4
In the national curriculum for maths in England, for each area of maths outlined, there is both a statutory requirement and a non-statutory requirement. The statutory requirement is as follows:
- Compare and classify geometric shapes, including quadrilaterals and triangles, based on their properties and sizes
- Identify acute and obtuse angles and compare and order angles up to 2 right angles by size
- Identify lines of symmetry in 2-d shapes presented in different orientations
- Complete a simple symmetric figure with respect to a specific line of symmetry
The non-statutory notes and guidance suggests:
Pupils continue to classify shapes using geometrical properties, extending to classifying different triangles (for example, isosceles triangle, equilateral triangle, scalene triangle) and quadrilaterals (for example, parallelogram, rhombus, trapezium).
Pupils compare and order angles in preparation for using a protractor and compare lengths and angles to decide if a polygon is regular or irregular.
Pupils draw symmetric patterns using a variety of media to become familiar with different orientations of lines of symmetry; and recognise line symmetry in a variety of diagrams, including where the line of symmetry does not dissect the original shape.
Properties of shapes Year 4 lesson ideas
The objective this will look at will be ‘Identify lines of symmetry in 2-d shapes presented in different orientations.’ In this objective, ‘orientation’ is the key word and here we can draw on variation theory again. The objective makes no reference if the shapes should be regular or irregular, so it is best to spread this over two days and tackle both. Ideally, mirrors will be provided for each pupil.
When using mirrors it is best to provide pupils with two types of questions. One where they are required to find a line of symmetry or denote whether an already given line represents a line of symmetry or not.
Once pupils are comfortable with this, they should then use their skill with a mirror to draw the other half of a shape. At the beginning stages, this should be done on isometric paper so that the drawings themselves can be as accurate as possible.
I would start with simple shapes and gradually, over a sequence of a couple of lessons, make the shapes harder by including irregular shapes. I would not, at this stage, expect pupils to complete a ‘picture’ only shape.
Properties of shapes Year 4 word problems
There would not be any standard ‘word problem’ that would look at the mathematics behind symmetry that was so convoluted that it would hold any mathematical relevance.
Properties of shapes Year 4 reasoning and problem solving
A great investigation for symmetry would be to dislodge the misconception that a circle only has one side.
To do this you can look at lines of symmetry of regular shapes. When pupils are aware that regular shapes have all equal sides and equal angles, they can then investigate what this means for lines of symmetry.
As long as you provide regular polygons, they will quickly see a pattern where the number of lines of symmetry are associated with the number of sides. A square has 4 lines of symmetry, a regular pentagon has 5 lines of symmetry etc.
Once pupils notice the pattern you can get them to conjecture about all manner of regular shapes.
At this point, I would ask about a circle. Pupils are often taught that a circle has one continuous side and so therefore, if our pattern holds true, should only have one line of symmetry.
A quick attempt of getting 30 pupils to find just one line of symmetry will, in more cases than not, provide at least two cases where pupils have drawn the one line in different places of the circle.
Here we can draw the distinction between a circle and a polygon (must have straight sides) and that there are actually an infinite number of lines of symmetry and this leads some mathematicians to conjecture that a circle also has an infinite number of sides.
Geometry – properties of shapes KS2: Year 5
In the national curriculum for maths in England, for each area of maths outlined, there is both a statutory requirement and a non-statutory requirement. The statutory requirement is as follows:
- Identify 3-D shapes, including cubes and other cuboids, from 2-D representations
- Know angles are measured in degrees: estimate and compare acute, obtuse and reflex angles
- Draw given angles, and measure them in degrees (°)
- Identify:
- Angles at a point and 1 whole turn (total 360°)
- Angles at a point on a straight line and half a turn (total 180°)
- Other multiples of 90°
- Use the properties of rectangles to deduce related facts and find missing lengths and angles
- Distinguish between regular and irregular polygons based on reasoning about equal sides and angles
The non-statutory notes and guidance suggests:
Pupils become accurate in drawing lines with a ruler to the nearest millimetre, and measuring with a protractor. They use conventional markings for parallel lines and right angles.
Pupils use the term diagonal and make conjectures about the angles formed between sides, and between diagonals and parallel sides, and other properties of quadrilaterals, for example using dynamic geometry ICT tools.
Pupils use angle sum facts and other properties to make deductions about missing angles and relate these to missing number problems.
Properties of shapes Year 5 lesson ideas
In this lesson, it will take its objective from being able to identify angles in a straight line and, as the non-statutory guidance says, ‘Pupils use angle sum facts and other properties to make deductions about missing angles and relate these to missing number problems’.
Prior to teaching this objective, it is important that the following have been learn by the pupils:
- Know angles are measured in degrees: estimate and compare acute, obtuse and reflex angles
- Draw given angles, and measure them in degrees (°)
Pupils should draw on their knowledge of right angles and know that 2 right angles next to each other will constitute a half turn and that a half turn is equal to 180 degrees.
Prior to this lesson, I would spend considerable time getting pupils to complete missing number type questions with two and three integers where the answer is equal to 180.
This would be done under the guise of regular fluency practice and I would not explain its true purpose at the time.
To begin with, I would ensure that all missing angles can be solved by measuring it with a protractor and expect the questions to be solved this way. Furthermore, the orientation of the questions would greatly vary so pupils get practice in using a protractor in a manner of different situations.
Once pupils have begun to grow comfortable with measuring, only then would I introduce questions not drawn to scale and where pupils will have to rely on their application of number to solve the missing angles. Once again, this would take place where there are 2 or 3 given angles that pupils would have to solve. It would be at this point that all the prior training of finding missing values that equal 180 would begin to make sense and ensure that this objective is taught as efficiently as possible.
Properties of shapes Year 5 reasoning and problem solving
Angles in a straight line allow for the retrieval of other number facts that have been taught previously. A common reasoning activity in my classroom involved pupils recollecting squared and cubed numbers to solve missing angles in a straight line. This worked in multiple ways.
First, questions such as the one below where angle b was a square number between 20-50.
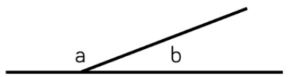
Pupils would then have to use their knowledge of square numbers and angles in a straight line to find all the possibilities.
B = 25 degrees A = 155 degrees
B = 36 degrees A = 144 degrees
B = 49 degrees A = 131 degrees
The other way it would be used would be with cubed numbers where A was an obtuse angle and also a cubed number. What are angles A and B?
Again, here pupils would have to recall their knowledge of cubed numbers and obtuse angles and know that there is only one possible outcome here. A must be 125 degrees as it is the result of 5 cubed and the only cubed number that is an obtuse angle. Therefore, angle b must be 155 degrees.
Geometry – properties of shapes KS2: Year 6
In the national curriculum for maths in England, for each area of maths outlined, there is both a statutory requirement and a non-statutory requirement. The statutory requirement is as follows:
- Draw 2-D shapes using given dimensions and angles
- Recognise, describe and build simple 3-D shapes, including making nets
- Compare and classify geometric shapes based on their properties and sizes and find unknown angles in any triangles, quadrilaterals, and regular polygons
- Illustrate and name parts of circles, including radius, diameter and circumference and know that the diameter is twice the radius
- Recognise angles where they meet at a point, are on a straight line, or are vertically opposite, and find missing angles
The non-statutory notes and guidance suggests:
- Pupils draw shapes and nets accurately, using measuring tools and conventional markings and labels for lines and angles.
- Pupils describe the properties of shapes and explain how unknown angles and lengths can be derived from known measurements.
- These relationships might be expressed algebraically for example, d = 2 × r; a = 180 − (b + c).
Properties of shapes Year 6 lesson ideas
Somewhat surprisingly, it is not until Y6 that we see the use of the net explicitly mentioned. A primary favourite is the deconstruction of 3d shapes into their component pieces to see how they are created.
It is quite common for this to be done earlier in school and so when Y6 teachers wish to look at this objective, it is important to extend pupils’ thinking.
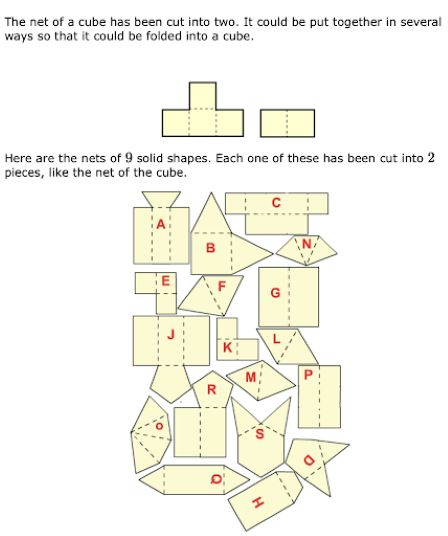
The joy of the above lesson would be to allow pupils to physically cut the shapes and attempt to match them up – though it would be possible to look at pupils’ special thinking by only using the 2D representations.
When modelling the activity, I would be sure to look at K and E as this is the most obvious pairing that pupils may go for. By using this as the model, we are getting pupils to extend their thinking as we are eliminating the easier option from the list.
The answers and the name of the shapes can be found below.
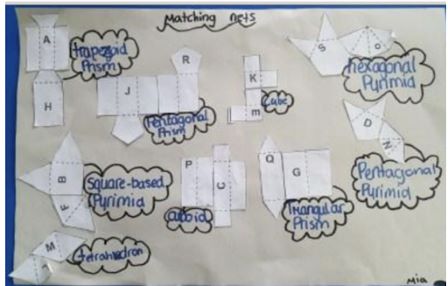
Properties of shapes Year 6 word problems
A simple true or false question can provide valuable insight into pupils’ understanding of shape. For example,
True or false?
In order to be regarded as a quadrilateral, a shape must have at least one right angle.
While pupils may know the answer is false, you can push this thinking on by getting pupils to draw some quadrilaterals that do not contain a right angle. These would include a parallelogram and a trapezium.
Looking for some more ideas of how to do this? You can find plenty of free resources and shapes worksheets on the Third Space Learning maths hub. You may also find our blog, What Are Vertices, Faces and Edges?, useful to pair alongside this topic!
For guidance on other KS2 subjects, check out the rest of the series:
- Teaching Decimals KS2
- Teaching Place Value KS2
- Teaching Fractions KS2
- Teaching Percentages KS2
- Teaching Statistics KS2
- Teaching Ratio and Proportion KS2
- Teaching Multiplication KS2
- Teaching Division KS2
- Teaching Addition and Subtraction KS2
- Teaching Geometry – Position, Direction and Coordinates KS2
Looking to get ahead on other KS2 maths topics?
We have the lowdown from expert primary teachers on all the trickiest KS2 maths concepts to teach, including teaching times tables, telling the time, as well as the long division method and the long multiplication method.
DO YOU HAVE PUPILS WHO NEED MORE SUPPORT IN MATHS?
Every week Third Space Learning’s specialist primary maths tutors support thousands of students across hundreds of schools with weekly online 1 to 1 maths lessons designed to plug gaps and boost progress.
Since 2013 these personalised one to one lessons have helped over 169,000 primary and secondary students become more confident, able mathematicians.
Learn about the scaffolded lesson content or request a personalised quote for your school to speak to us about your school’s needs and how we can help.