What Are Types of Triangles? Isosceles, Scalene, Equilateral And Right Angle Triangles: Explained For Primary School

Here you can find out about the different types of triangles, their properties, and how you can help children to understand triangles as part of their maths learning at home.

Students encounter different triangles as early as Year 1, but as they progress through school, the amount of knowledge that they need to know about different triangle types increases. 

They will need to know the names of these different types of triangles and their properties. They will also need to know how to use these properties when classifying triangles and solving problems, such as missing angles, by using and applying their understanding.

What is a triangle?

As the name suggests, a triangle is a shape that contains three angles and three edges. The angle measures, when added together, will result in a total of 180 degrees.

In the early stages of primary school, students will just need to know that any triangle will have three sides. 

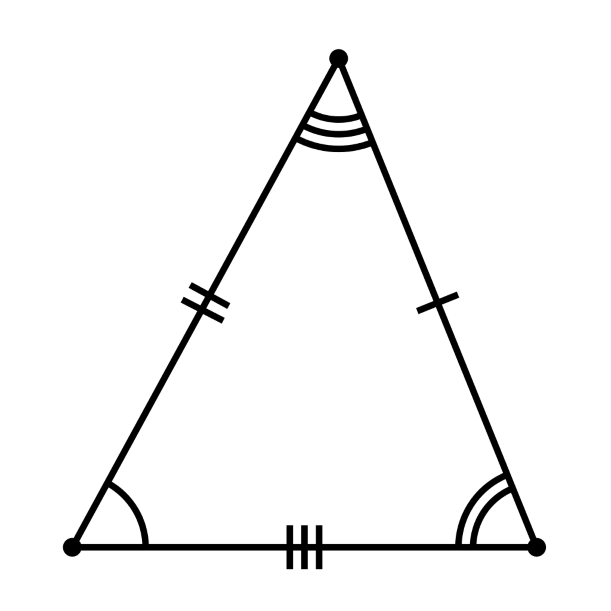
Types of triangles

The interior angles of a triangle can help to identify what type it is. For example, an equilateral triangle has 3 equal angles, while an isosceles triangle has two equal lengths.

However, no matter what types of angle a triangle has, they will always ad

What is an equilateral triangle?

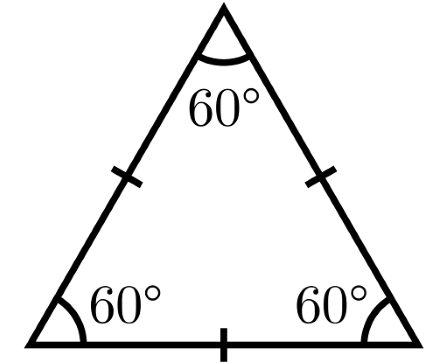
There is a hint within the name as to the properties of this triangle. An equilateral triangle is where the lengths of the sides of a triangle and all its interior angles are equal. 

As 180 degrees divided by 3 is 60, each angle will always be an acute angle of 60 degrees. Therefore an equilateral triangle is also an acute-angled triangle and an equiangular triangle

Note that an obtuse-angle triangle is a triangle with one obtuse angle – a triangle can only have a maximum of one obtuse angle, otherwise it would take on a different shape and the angles would not add up to 180.

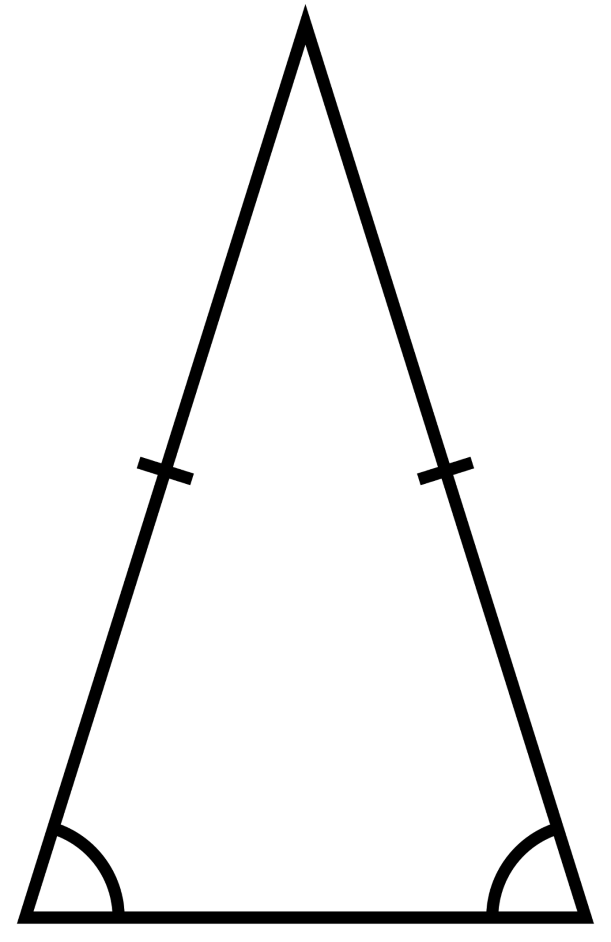
What is an isosceles triangle?

An isosceles triangle has two sides of equal length and two equal angles.

Isosceles comes from the latin meaning ‘equal legs’ and this can be an engaging way to get students to remember what an isosceles triangle is.

What is a scalene triangle?

A scalene triangle has no equal sides or angles. All sides of the triangle are different lengths.

However, the angles of the triangle will continue to add up to 180 degrees.

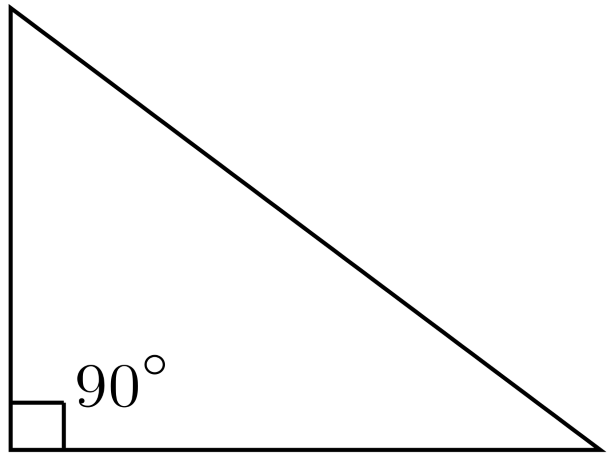
What is a right-angled triangle?

A right-angled triangle is, as the name implies, a triangle that has one angle of exactly 90 degrees opposite its longest side, or its ‘hypotenuse’.

For example, a right isosceles triangle has one angle of 90 degrees and two angles of 45 degrees.

It may be useful to introduce this term at this stage so that pupils are familiar with it when they come to studying the Pythagorean theorem in later years.

What is an acute triangle?

An acute triangle is a type of scalene or isosceles triangle where all angles are acute.

This means every angle is below 90 degrees.

For example, an acute scalene triangle is a triangle with no equal sides or angles where each angle is less than 90 degrees.

What is an obtuse triangle?

An obtuse triangle is a scalene or isosceles triangle where one angle is obtuse.

This means one angle is above 90 degrees, which will mean that the other two are acute angles.

When will my child learn about triangles in primary school?

Students first explicit introduction to triangles comes in Year 1. The national curriculum states that pupils should be taught to:

• Recognise and name common 2-D and 3-D shapes, including:
  •  2-D shapes [for example, rectangles (including squares), circles and triangles]
• [Non-statutory] Pupils handle common 2-D and 3-D shapes, naming these and related everyday objects fluently. They recognise these shapes in different orientations and sizes, and know that rectangles, triangles, cuboids and pyramids are not always similar to each other.

This progresses in Year 4 where students must learn the following:

• Compare and classify geometric shapes, including quadrilaterals and triangles, based on their properties and sizes.
• Non-statutory] Pupils continue to classify shapes using geometrical properties, extending to the classification of triangles(for example, isosceles, equilateral, scalene) and quadrilaterals (for example, parallelogram, rhombus, trapezium).

This progresses in Year 6 where students must learn the following:

• compare and classify geometric shapes based on their properties and sizes and find unknown angles in any triangles, quadrilaterals, and regular polygons.

How does this relate to other areas of mathematics?

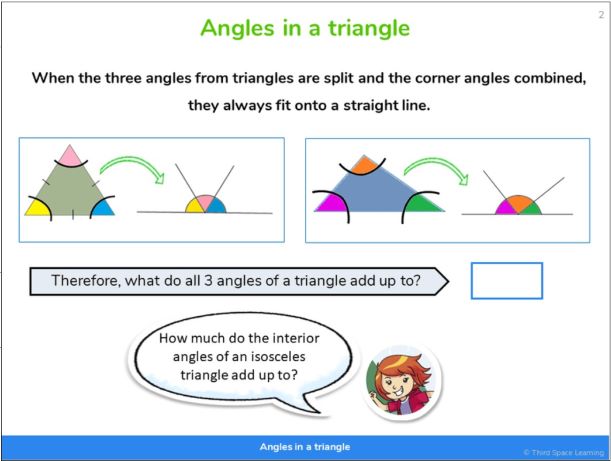
Students will also be asked to calculate the area of a triangle when they reach Year 6 and they may use some facts about triangles to assist them in this endeavour. 

Links may be made between the angles of a triangle and angles in a straight line being the equivalent to a half turn (180 degrees) and students may investigate why angles in a triangle are equal to the angles on a straight line.

There is also a link to 3D shapes, triangles, and vertices in Year 2 and 3.

How does this relate to real life?

Triangles are structurally the most stable geometric shape and used throughout the construction industry, even though you cannot see them on modern buildings. 

You may see some if you go across long bridges. Next time you are challenged to make a bridge out of spaghetti, try to incorporate triangles and you will be surprised how well it can hold up!

Triangles worked examples

1. Circle the isosceles triangle.

To answer this question, I need to know the different types of triangles as well as be fluent in my understanding of the properties of triangles. I need to know that an isosceles triangle has two lengths of their sides that are identical and look at the triangles to see if this is the case. From this, I can deduce that the top left triangle is the isosceles triangle

2. Find the missing angle.

For this question, I would have to know that angles in a triangle all add up to 180 degrees and that this is an equilateral triangle, so all angles must be equal. I can therefore deduce that the missing angle is 60 degrees.

3. Draw an isosceles triangle with two 80 degree angles.

To be successful here I would need to know how to use a protractor accurately. With this knowledge, I would then be able to draw a triangle where 2 angles are 80 degrees. This would look like the following:

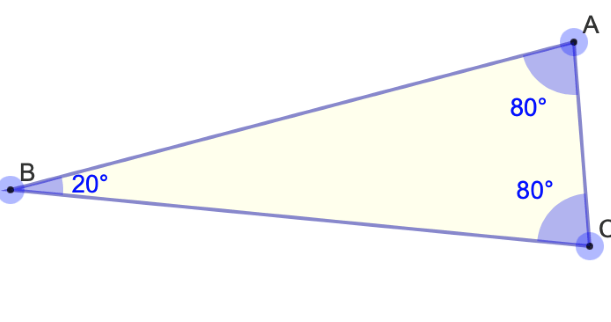
4. Find the missing angles.

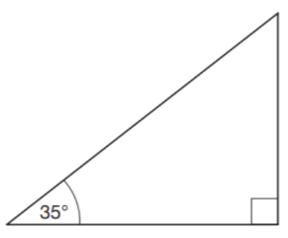
To find the missing angle, I need to know that there are 180 degrees in a triangle and that the square in the bottom right represents an angle of 90 degrees. From here, I can perform some calculations to find the missing angle. I will first subtract 90 from 180.

180 – 90 = 90

As I know the other angle is 35 degrees, I can subtract that from the 90.

90 – 35 = 55

The missing angle is 55 degrees.

Triangles example questions

1. What is a scalene triangle?

Answer: A scalene triangle has 3 unequal sides and no angles are the same

2. Draw an equilateral triangle 

Answer:

3. Find the value of Y in the triangle

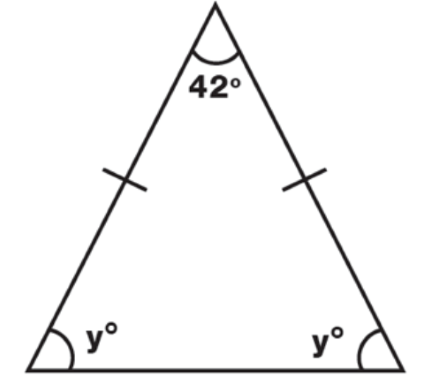
Answer: Y = 69 degrees

4. Circle the scalene triangle

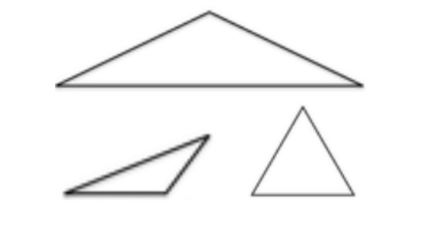
Answer: Bottom left is the scalene

Wondering about how to explain other key maths vocabulary to your children? Check out our Primary Maths Dictionary, or try these other blogs:

• What is Maths Mastery?
• What are 2d shapes? (Shape properties)
• What Are Angles? (acute, reflex, obtuse angles and more)

You can find plenty of triangles worksheets for primary school pupils on the Third Space Learning Maths Hub.