How to Teach Fractions KS2: Maths Bootcamp [3]
Want to know how to teach fractions to KS2 Maths pupils? You’ve found the right place!
This post is part of our interventions bootcamp series: designed to support Year 5 and 6 teachers and SATs booster group leaders achieve age related expectations with pupils who need that extra intervention.
It’s particularly aimed at those running interventions in school but is also relevant much more widely to your whole class teaching, supporting a mastery approach, to help you ensure each child is getting appropriate support and challenge.
Each post follows a similar structure:
- First you’ll diagnose where children are struggling with ‘the nuts and bolts’ of that area of Mathematics from the National Curriculum.
- Next you’ll track back to the different stages of understanding and examine what the misconception might be in detail.
- Finally we’ll give you strategies that can be used with whole classes, booster groups or alongside 1 to 1 interventions.
In this post on fractions, we help you solve problems such as:
- They keep multiplying diagonally when adding fractions.
- They just don’t seem to understand what the numerator and denominator are.
- They keep writing their answer as a fraction when they shouldn’t be.
Fraction Lessons Resource Pack
Plug gaps and help conquer common upper KS2 misconceptions in fractions
Download Free Now!The How to Teach KS2 Maths Interventions Bootcamp series
How to teach fractions KS2
How to teach place value year 5 and year 6
How to teach multiplication KS2
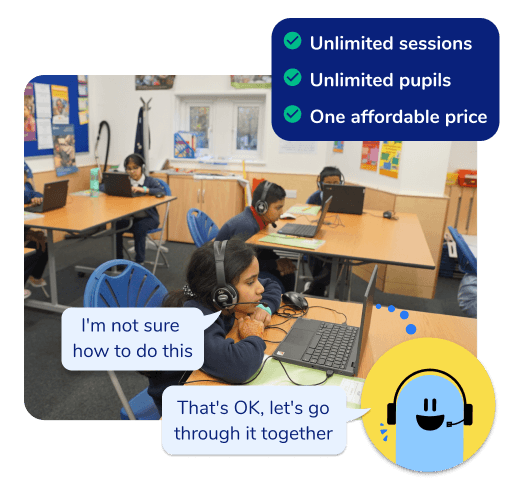
Unlimited primary maths tutoring with Skye, the voice-based AI maths tutor.
Built on the same principles, pedagogy and curriculum as our traditional tutoring but with more flexibility, reach and lower cost.
Join the schools already helping hundreds of primary pupils nationwide with Skye’s one to one maths tutoring
Watch Skye in actionDiagnosis: What are pupils’ key misconceptions with Fractions?
From the below children, find the best fit for those you are looking at. Then use this to guide where in the post to read for support:
Archie has, over time, learnt how to find a fraction of a number through repetition (such as 1/6 of 30). However, he struggles when finding 2/6 of 30. He has had lots of practice of fraction methods but hasn’t had much exposure to concrete or pictorial resources during his fractions learning. When presented with adding fractions he multiplies the numbers or adds diagonally. He has some concept of improper fractions but has no idea how to apply this in a problem context Stage: EMERGING.
Sehr can tell you about equal parts, what the denominator and numerator mean and can use this knowledge in the context of finding fractions of a number or shape. She begins to struggle when applying her understanding to adding and subtracting fractions or converting between mixed and improper fractions. Stage: DEVELOPING.
Holly struggles a little with multiplying and dividing fractions as she gets their methods mixed up with adding and subtracting fractions. She can find fractions of numbers and is beginning to apply her understanding in problem contexts but then makes errors and picks the wrong method to work out. Sometimes it seems as though she makes a ‘best guess’ at an answer rather than applying a method she is confident with. Stage: SECURING.
Reece finds it difficult to work out what method or process he should undertake when presented with calculations such as ‘find 1/5 of 20’. He can colour in fractions of a shape such as 3/7 of a shape with 7 equal parts but struggles when asked to colour 1/7 of a shape with 14 equal parts. Stage: PRE-BOOSTER: Reece’s needs are beyond the scope of a booster group or this post. He needs specifically targeted 1-to-1 support from a trained professional to catch him up to a point where a booster intervention could be considered. (If you are looking for 1-to-1 support, then read this review I wrote of Third Space Learning’s 1-to-1 Maths programme.)
For a more personalised diagnosis of pupils’ misconceptions in fractions download the free Fractions, Decimals and Percentages Diagnostic Quiz for years 5 and 6.
Misconceptions and strategies for ‘EMERGING’ in Fractions
For a child like Archie, he needs a concrete foundation built on the use of physical manipulatives and with fractions in particular, pictorial representations. Using a more visual approach, such as fraction games, will give him a greater understanding of what fractions are, which will in turn give him the knowledge to attempt nearer age-appropriate fraction questions.
Underpinning skills and concepts
What are fractions?
- Give children a piece of squared paper. Ask the how we can make halves out of the paper. Discuss simple different ways (folded in half vertically, horizontally, diagonally).
- Discuss how we know this is half (they fit onto each other etc). Push towards making the deduction that the 2 parts are all equal in size.
- Push children to thinking if there are other ways you could make half with the paper.
- Repeat but folding into 4 fourths or quarters.
- Children should realise that the foundation of everything else in fractions is that you have a whole and it is split into equal parts.
- Shade 1 part of the 4. Ask what fraction is shaded in. Write ¼ and discuss the terms numerator and denominator. As suggested explanation is that the denominator is the ‘name’ of the fraction. IE how many equal parts there are altogether. The numerator at this stage is whatever is shaded in. A better explanation is the numerator is whatever we are talking about/finding out. This could be what is shaded, what isn’t shaded or written as well as drawn.
- To secure understanding, ensure the children say ‘out of’ when reading the vinculum (horizontal line splitting the numerator from denominator), when looking at a written fraction. For example, 5/7 would be said by the children as “five out of seven”.
- Give children an example such as 1/6 where 1 equal part is shaded, discussing the denominator and numerator and what they show. Ask them what fraction is unshaded. The following answers show a lack of understanding that means children need more practical time, experience and examples such as given above:
- 1/5 (not understanding what the numerator and denominator mean or they are not applying this knowledge when looking at a fraction).
- 5 or 5/5 (can see that there are 5 equal parts unshaded but are not able to put this in the context of a written fraction)
- Once the children are able to see that there are 5/6 unshaded, put the 2 fractions alongside each other in both written and pictorial form (1/6 + 5/6). Ask them what fraction is shaded and unshaded. Children should be readily ably to see that there is 1 part shaded + 5 parts unshaded = 6 parts. They may struggle with the denominator, either saying the total is 6/1 or 6/12. Focus the children on saying the fraction as directed above: “one out of six are shaded and five out of six are unshaded) and looking at the pictorial fraction. Discuss how many parts we had to start with (6) and how many we have now (6). Write 6/6. Say the fraction. Ask children what has happened to the numerator (added) and denominator (stayed the same). Ask what this fraction is the same as (1 or 1 whole one).
- Try the children them with different fractions, withdrawing the pictorial support until the children can explain and see the fraction shown and the other fraction that makes a whole without support.
- Finally, to secure understanding that there must be equal parts, show the children a selection of fractions and shapes. Some of the shapes should be cut into equal parts, others not. Ask the children to find and shade a shape with (for example) 3/5.
Fractions of numbers
- Give the children the calculation ¼ of 12. Ask them what this means. Nudge them to saying there are 12 whole ones. Give the children 12 concrete manipulatives each (such as multilink). Conclude that if we are finding ¼ we will need to have 4 groups with the same amount in each because the denominator means the total amount of equal parts. Discuss that when we are finding a fraction of a number we will need to share and sharing is one way to divide. Give the children time to share out their 12 objects into 4 groups of equal size. Ask how many cakes are in each group (3). Write down ¼ of 12 = 3 then ask “Can anyone see anything in that calculation that looks familiar?”. The children should be able to see the multiplication family 3, 4, 12. Discuss this even if they don’t see it. IE we have found that 3 fits into 12, 4 times. Ask the children if there is a way we can re-write this calculation as a division statement (12 ÷ 4 = 3).
- Give 1 or 2 more examples but move on quickly to finding more than 1 part (e.g. ¾ of 12).
- Ask the children to split 12 into 4 equal sized groups again. This time ask what the calculation requires (3 out of 4). Push the children to seeing that they have 4 groups, so if we now need to find 3 of them we can count the amount in 3 of our 4 groups because we know they are the same size. Children making connections here should be able to multiply 3 (one group) by 3 (3 total groups) = 9. Use these children to explain how they can use multiplication in this step. Repeat for other fractions such as 3/5 and 4/7. Return to concrete manipulatives where children are not understanding the concept of find 1 part and multiplying it by the numerator.
When children like Archie can confidently explain what fractions are, what the numerator and denominator show and how to find fractions of shapes and numbers, they are ready to move on to the next stage of the booster – ‘DEVELOPING’.
Misconceptions and strategies for ‘DEVELOPING’ in Subtraction
Children like Sehr, who make errors when adding and subtracting fractions or converting between mixed and improper fractions, need to visualise the fractions more to eliminate those errors and develop a deeper understanding they can then apply in problem contexts.
Adding subtracting fractions
It is recommended to relate anything the children do not understand to slices of the same size cake.
- Give the children addition calculations with the same denominator such as 3/10 + 5/10. Note any children who are adding the denominator, multiplying or going diagonally. If any of these occurs, return to the content on adding fractions in ‘Emerging’, focusing on use of pictorial representations to ground their understanding. It is important children ‘see’ rather than are just taught to ‘add the numerators, keep the denominator the same’ as otherwise this will continue to lead to errors, particularly when introducing multiplying and dividing fractions. IE discuss how there are 3 parts of the cake eaten already and another 5 parts of the cake are also going to be eaten. So, in that same cake, how many parts have been eaten altogether and how many parts of the cake were there altogether.
- Repeat with subtractions such as 5/7 – 2/7. Ask how many equal parts the cake has (7) and if there were 5 parts left and you are giving out another 2, how many equal parts will remain? And how many did we have at the start again? 3 out of 7, 3/7.
- Keep referring children during this process for addition and subtraction that you are adding or subtracting with the numerator whereas the denominator remains constant because you are still looking at that same cake all the way through the calculation.
Ordering Fractions
- Children should already be able to quickly order fractions with the same denominator such as 5/10, 3/10, 10/10. Initially, when looking at fractions with differing numerators children should draw bar model pictorial representations one under the other to compare visually:
- When children are able to see that the smaller the denominator, the less equal parts, so the larger each of those parts are, challenge the children to compare and order fractions with a large degree of difference. For example, ordering ½, 3/20, 1/10, 7/8. Children can draw if necessary but should be able to see that 7/8 is the largest and ½ is the next largest. They should also be able to notice that 2 fractions have the same numerator and they can use this to help order the fractions (a pictorial version of ½ and 1/10 would help explain this to the children).
- When children are able to look at clearly different fractions and order them by just visualising them, they are ready to discuss finding the lowest common denominator. To keep things simple for the children, write and draw 1/3 and 1/6. We know that 1/3 is a greater amount than 1/6 but how much? Children should be able to see that 1/6 looks half the size of a 1/3. Draw lines on 1/3 so it is cut into 6. Ask what the 1/3 looks the same as. Write that as a fraction and ask what has happened to get from 1/3 to 2/6 (we have doubled both numbers). Explain that we have made the denominator the same (common) and that it is the lowest one we could do this with. Experiment with the same fractions with higher denominators to demonstrate (such as 18 so the children can see that in the worst case scenario, they can multiply the 2 numbers together).
- Explain that when we turned 1/3 into 2/6 we did so by starting with our denominator. We looked at the relationship between 3 and 6 and saw that 3 fitted into 6 twice. So we doubled our denominator. Explain that if we do this we also need to double our numerator. Refer to pictorial versions for this and other examples, including what to do when one denominator will not ‘fit’ into the other as 3 to 6 does (e.g. ¼ and 2/5)
- Give children experience of applying there understanding in adding and subtracting contexts, e.g. ¼ + 2/5 = 5/20 + 8/20=13/20.
Mixed and improper fractions
- Show children 8/8 drawn and written. Discuss what this means (one whole one). Now draw 2 more 8/8. Again, discuss that this means we have 3 whole ones. Discuss what we have if someone eats a slice of cake. We have 8/8 and 8/8 and 7/8. Discuss how this could be written (2 and 7/8). Spend time with examples linking the same sized numerator and denominator with whole numbers.
- Using the same example as above (3 whole ones), discuss how many pieces each cake has (8) and how many pieces we have altogether (24). Explain that we can also write this as 24/8. It is the same as 3 whole ones because each cake has 8 pieces. Again, remove one slice of cake. Ask the children what size this now leaves (23/8). Ask them if we can change this to a mixed fraction with whole numbers separate. Ask them how we could do this and nudge them to seeing that if a whole cake has 8 parts, we can take away 8 from 23 to put one cake separately at the side. We can then keep doing this until we have less than a whole cake left (less than 8/8). In this example we will have 2 whole ones and 7/8 left. Compare with the mixed fraction we had to begin this section with.
- Nudge the children to seeing that rather than taking ‘8’ away each time, which is repeated subtraction which is in turn related to division, we can simply see how many times 8 fits into 23 (twice) and that is how many whole ones we have. The remaining amount is the fraction we have left.
- Again, give the children experience of applying this understanding in addition and subtraction contexts such as 2 ½ + ¾.
Misconceptions and Strategies for ‘SECURING’ in Subtraction
Children like Holly may well need to recap some of the content of ‘Developing’ to eliminate errors when ‘Securing’ their understanding. However, it may well be sufficient for these children to recap in whole class contexts and focus their booster on the specifics that they really struggle with such as multiplying and dividing fractions. If after covering some of the content in this section, some children are struggling and making errors, return to the ‘Developing’ content.
Multiplying Fractions
- Discuss the fraction ¼ x 1/5. Ask what we know about multiplication (that it is repeated addition). Using an array, draw a whole one and split into 4 equal parts. Underneath, draw 4 more whole ones split into 4 equal parts. So the total rows are 5 to represent the fifths. Discuss that each side totals 1 whole one and that the area totals one whole as well. We can choose how to split up each side to help us.
- Discuss how many parts there are (20) so ¼ x 1/5 = 1/20. Discuss how this can be calculated by multiplying the denominators together. Ask how many parts we should shade in (1 because our numerators are both 1). Give other examples where you have to shade more than one in such as ¾ x 2/5. Where the amount shaded (numerator) is 6.
- Move children towards not needing arrays anymore, once they have made the link to just needing to multiply the denominators together and then multiply their numerators together before potentially simplifying the resulting fraction.
Read more: How to Simplify Fractions: A Primary School Guide
Dividing Fractions
- When going through the process of how to divide fractions by a whole number, use a bar model. For example, in ¼ ÷ 6, draw a bar that represents one whole one. Split it into 4 to represent each quarter. Underneath the first ¼, draw a comparison bar that splits into 6 (as we need to divide ¼ by 6). Continue the bar until all ¼ are split into 6. The total bars will show the equal parts (denominator) and one of the sections will show how many we are looking at (numerator). So ¼ ÷ 6 = 1/24.
- Repeat for calculations such as 6/7 ÷ 3 where the only difference is our numerator is not 1, it is 6. Draw a bar to represent 1. Split it into 7 equal parts. Under each part draw a comparative bar that is split into 3. Colouring 1 in will find 1/7 so we need to colour 6 in to find 6/7. This then gives us 6 out of 21 coloured in. This can then be simplified to 2/7.
- Once the children see that the link between the bars and multiplying the denominator by the whole number, the bars can be removed.
Problem Contexts
Problem contexts can be given during the booster with additional pictorial support where necessary but primarily this should be possible through whole class teaching and learning, providing the children are secure on the areas of fractions that are required to solve the problem.
If your pupils have gaps that still need plugging in the run up to SATs, don’t panic! Read our blog on how to get all your Year 6 to achieve 100 in Maths (be sure to follow this order of topics to revise) or get in touch with our schools team who can arrange a free demo of our one-to-one maths interventions.
Don’t forget to download your free subtraction resources
FREE Fractions Intervention Lessons for Years 5 and 6
FREE All Kinds of Fractions Word Problems
FREE Adding and Subtracting Fractions Gap Plugging Pack (Years 1 to 6)
FREE Multiplying and Dividing Fractions Gap Plugging Pack (Years 5 and 6)
FREE Fractions Decimals and Percentages Year 6 SATs Questions Pack
FREE All Kinds of Decimals and Percentages Word Problems
Further Reading
DO YOU HAVE STUDENTS WHO NEED MORE SUPPORT IN MATHS?
Skye – our AI maths tutor built by teachers – gives students personalised one-to-one lessons that address learning gaps and build confidence.
Since 2013 we’ve taught over 2 million hours of maths lessons to more than 170,000 students to help them become fluent, able mathematicians.
Explore our AI maths tutoring or find out about a primary school maths tutor for your school.



![How to Teach Fractions KS2: Maths Bootcamp [3]](https://thirdspacelearning.com/wp-content/uploads/2018/07/Fractions-intervention-pack-thumb.png)



