25 Fun Maths Problems For KS2 And KS3 (From Easy To Very Hard!)
Fun maths problems are one of the things mathematicians love about the subject; they provide an opportunity to apply mathematical knowledge, logic and problem solving skills all at once.
In this article, we’ve compiled 25 fun maths problems, each covering various topics and question types. They’re aimed at students in KS2 & KS3. We’ve categorised them as:
How should teachers use these maths problems?
Teachers could make use of these maths problem solving questions in a number of ways, such as:
- embed into a relevant maths topic’s teaching.
- settling tasks at the beginning of lessons.
- break up or extend a maths worksheet.
- keep students thinking mathematically after the main lesson has finished.
Some are based on real life or historical maths problems, and some include ‘bonus’ maths questions to help to extend the problem solving fun! As you read through these problems, think about how you could adjust them to be relevant to your students or to practise different skills.
These maths problems can also be used as introductory puzzles for maths games such as those introduced at the following links:
Need more support teaching reasoning, problem solving and planning for depth? Read here for free CPD for you and your team of teachers.
Maths word problems
1. Home on time – easy
Type: Time, Number, Addition
A cinema screening starts at 14:35. The movie lasts for 2 hours, 32 minutes after 23 minutes of adverts. It took 20 minutes to get to the cinema. What time should you tell your family that you’ll be home?
Answer: 17:50
2. A nugget of truth – mixed
Type: Times Tables, Multiplication, Multiples, Factors, Problem Solving
Chicken nuggets come in boxes of 6, 9 or 20, so you can’t order 7 chicken nuggets. How many other impossible quantities can you find (not including fractions or decimals)?
Answer: 1, 2, 3, 4, 5, 7, 8, 10, 11, 13, 14, 16, 17, 19, 22, 23, 25, 28, 31, 34, 37, or 43
There is actually a theorem which can be used to prove that every integer quantity greater than 43 can be ordered.
3. A pet problem – mixed
Type: Number, Problem Solving, Forming and Solving Equations, Simultaneous Equations, Algebra
Eight of my pets aren’t dogs, five aren’t rabbits, and seven aren’t cats. How many pets do I have?
Answer: 10 pets (5 rabbits, 3 cats, 2 dogs)
4. The price of things – mixed
Type: lateral thinking problem
A mouse costs £10, a bee costs £15, and a spider costs £20. How much does a duck cost?
Answer: £5 (£2.50 per leg)
Looking for more word problems, solutions and explanations? Read our article on word problems for primary school.
25 Fun Maths Problems - Printable
Download a printable version of these fun maths problems together with answers and mark scheme.
Download Free Now!Maths puzzles
5. A dicey maths challenge – easy
Type: Place value, number, addition, problem solving
Roll three dice to generate three place value digits. What’s the biggest number you can make out of these digits? What’s the smallest number you can make?
Add these two numbers together. What do you get?
Answer: In most cases, 1,089.
Bonus: Who got a different result? Why?
6. PIN problem solving – mixed
Type: Logic, problem solving, reasoning
I’ve forgotten my PIN. Six incorrect attempts locks my account: I’ve used five! Two digits are displayed after each unsuccessful attempt: “2, 0” means 2 digits from that guess are in the PIN, but 0 are in the right place.
What should my sixth attempt be?

Answer: 6347
7. So many birds – mixed
Type: Triangular Numbers, Sequences, Number, Problem Solving
On the first day of Christmas my true love gave me one gift. On the second day they gave me another pair of gifts plus a copy of what they gave me on day one. On day 3, they gave me three new gifts, plus another copy of everything they’d already given me. If they keep this up, how many gifts will I have after twelve days?
Answer: 364
Bonus: This could be calculated as 1 + (1 + 2) + (1 + 2 + 3) + … but is there an easier way? What percentage of my gifts do I receive on each day?
8. I 8 sum maths questions – mixed
Type: Number, Place Value, Addition, Problem Solving, Reasoning
Using only addition and the digit 8, can you make 1,000? You can put 8s together to make 88, for example.
Answer: 888 + 88 + 8 + 8 + 8 = 1,000
Bonus: Which other digits allow you to get 1,000 in this way?
Fraction problems
9. Quizzical – easy
Type: Fractions, Adding Fractions, Equivalent Fractions, Fractions to Percentages
4 friends entered a maths quiz. One answered \frac{1}{5} of the maths questions, one answered \frac{1}{10}, one answered \frac{1}{4}, and the other answered \frac{4}{25}. What percentage of the questions did they answer altogether?
Answer: 71%
10. Ancient problem solving – mixed
Type: Fractions, Reasoning, Problem Solving
Ancient Egyptians only used unit fractions (like \frac{1}{2}, \frac{1}{3} or \frac{1}{4}). For \frac{2}{3}, they’d write \frac{1}{3} + \frac{1}{3}. How might they write \frac{5}{8}?
Answer: \frac{1}{8} + \frac{1}{8} + \frac{1}{8} + \frac{1}{8} + \frac{1}{8} is correct. So is \frac{1}{2} + \frac{1}{8}.
Bonus: Which solution is better? Why? Can you find any more? What if subtractions are allowed?
Learn more about unit fractions here
11. Everybody wants a pizza the action – hard
Type: Fractions, Reasoning, Problem Solving
An infinite number of mathematicians buy pizza. The first wants \frac{1}{2} pizza. The second wants \frac{1}{4} pizza. The third & fourth want \frac{1}{8} and \frac{1}{16} each, and so on. How many pizzas should they order?
Answer: 1
Each successive mathematician wants a slice that is exactly half of what is left:
12. Shade it black – hard
Type: Fractions, Reasoning, Problem Solving
What fraction of this image is shaded black?
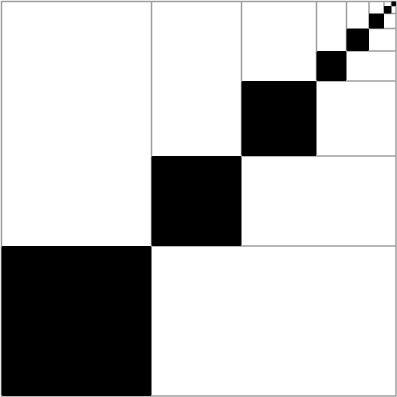
Answer: \frac{1}{3}
Look at the L-shaped part made up of two white and one black squares: \frac{1}{3} of this part is shaded. Zoom in on the top-right quarter of the image, which looks exactly the same as the whole image, and use the same reasoning to find what fraction of its L-shaped portion is shaded. Imagine zooming in to do the same thing again and again…
Multiplication and division problems
13. Giving is receiving – easy
Type: Number, Reasoning, Problem Solving
5 people give each other a present. How many presents are given altogether?
Answer: 20
14. Sharing is caring – mixed
Type: Number, Reasoning, Problem Solving
I have 20 sweets. If I share them equally with my friends, there are 2 left over. If one more person joins us, there are 6 sweets left. How many friends am I with?
Answer: 6 people altogether (so 5 friends!)
15. Times tables secrets – mixed
Type: Area, 2D Shape, Rectangles
Here are 77 letters:
BYHRCGNGNEOEAAHGHGCURPUTSTSASHHSBOBOREOPEEMEMEELATPEPEFADPHLTLTUT
IEEOHOHLENRYTITIIAGBMTNTNFCGEIIGIG
How many different rectangular grids could you arrange all 77 letters into?
Answer: Four: 1⨉77, 77⨉1, 11⨉7 & 7⨉11. If the letters are arranged into one of these, a message appears, reading down each column starting from the top left.
Bonus: Can you find any more integers with the same number of factors as 77? What do you notice about these factors (think about prime numbers)? Can you use this system to hide your own messages?
16. Laugh it up – hard
Type: Multiples, Lowest Common Multiple, Times Tables, Division, Time
One friend jumps every \frac{1}{3} of a minute. Another jumps every 31 seconds. When will they jump together?
Answer: After 620 seconds
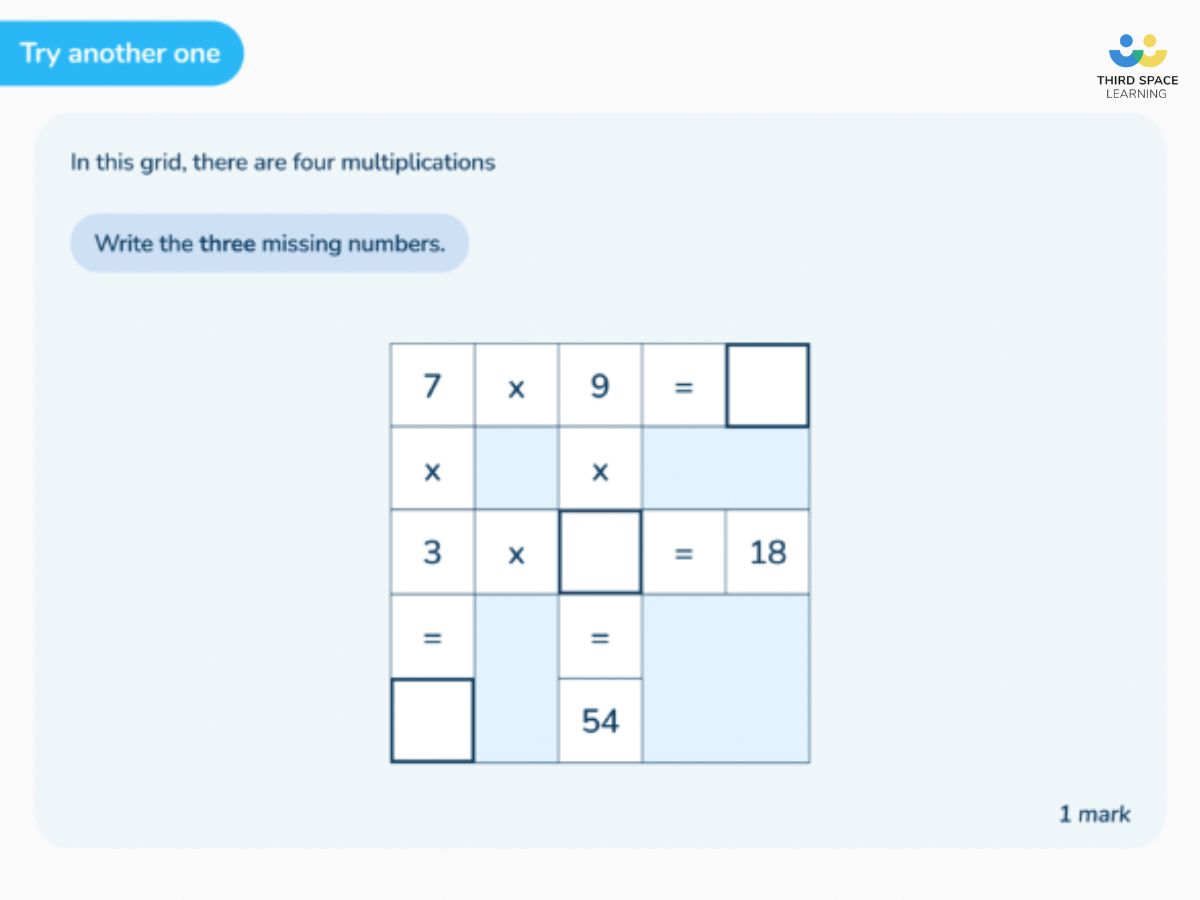
such as in this maths problem taken from our one to one, online maths tuition (SATs programme).
Geometry problems
17. Pictures of matchstick triangles – easy
Type: 2D Shapes, Equilateral Triangles, Problem Solving, Reasoning
Look at the matchsticks arranged below. How many equilateral triangles are there?
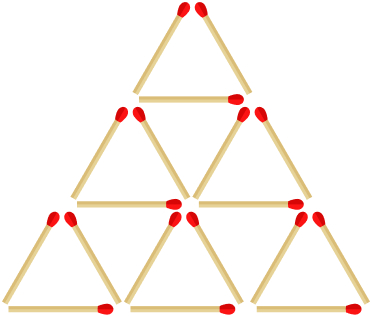
Answer: 13 (9 small, 3 medium, 1 large)
Bonus: What if the biggest triangle only had two matchsticks on each side? What if it had four?
18. Dissecting squares – mixed
Type: Reasoning, Problem Solving
What’s the smallest number of straight lines you could draw on this grid such that each square has a line going through it?
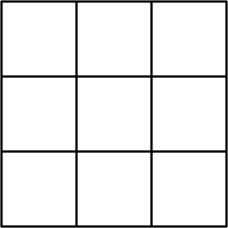
Answer: 2
e.g.

19. Make it right – mixed
Type: Pythagoras’ theorem
This triangle does not agree with Pythagoras’ theorem.
Adding, subtracting, multiplying or dividing each of the side lengths by the same integer can fix it. What is the integer?
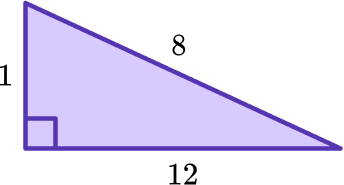
Answer: 3
3 x 1 = 3
8 – 3 = 5
12 ÷ 3 = 4
The new side lengths are 3, 4 and 5 and 32 + 42 = 52.
20. A most regular maths question – hard
Type: Polygons, 2D Shapes, tessellation, reasoning, problem-solving, patterns
What is the regular polygon with the largest number of sides that will self-tessellate?
Answer: Hexagon.
Regular polygons tessellate if one interior angle is a factor of 360°. The interior angle of a hexagon is 120°. This is the largest factor less than 180°.
Problem solving questions
21. Pleased to meet you – easy
Type: Number Problem, Reasoning, Problem-Solving
5 people meet; each shakes everyone else’s hand once. How many handshakes take place?
Answer: 10
Person A shakes 4 people’s hands. Person B has already shaken Person A’s hand, so only needs to shake 3 more, and so on.
Bonus: How many handshakes would there be if you did this with your class?
22. All relative – easy
Type: Number, Reasoning, Problem-Solving
When I was twelve my brother was half my age. I’m 40 now, so how old is he?
Answer: 34
23. It’s about time – mixed
Type: Time, Reasoning, Problem-Solving
When is “8 + 10 = 6” true?
Answer: When you’re telling the time (8am + 10 hours = 6pm)
24. More than a match – mixed
Type: Reasoning, Problem-Solving, Roman Numerals, Numerical Notation
Here are three matches:

How can you add two more matches, but get eight?
Answer: Put the extra two matches in a V shape to make 8 in Roman Numerals:
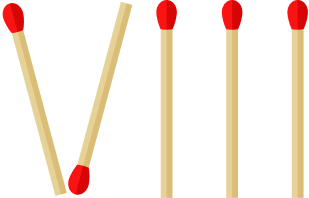
25. Leonhard’s graph – hard
Type: Reasoning, Problem-Solving, Logic
Leonhard’s town has seven bridges as shown below. Can you find a route around the town that crosses every bridge exactly once?

Answer: No!
This is a classic real life historical maths problem solved by mathematician Leonhard Euler (rhymes with “boiler”). The city was Konigsberg in Prussia (now Kaliningrad, Russia). Not being able to find a solution is different to proving that there aren’t any! Euler managed to do this in 1736, practically inventing graph theory in the process.
Maths puzzles are everywhere!
Many of these 25 maths problems are rooted in real life, from everyday occurrences to historical events. Others are just questions that might arise if you say “what if…?”. The point is that although there are many lists of such problem solving maths questions that you can make use of, with a little bit of experience and inspiration you could create your own on almost any topic – and so could your students.
For a kick-starter on creating your own maths problems, read our article on KS3 maths problem solving.
Looking for additional support and resources at KS3?
You are welcome to download any of the secondary maths resources from Third Space Learning’s resource library for free. There is a section devoted to GCSE maths revision with plenty of maths worksheets and GCSE maths questions. There are also maths tests for KS3, including a Year 7 maths test, a Year 8 maths test and a Year 9 maths test
Other valuable maths practice and ideas particularly around reasoning and problem solving at secondary can be found in our KS3 and KS4 maths blog articles. Try these fun maths problems for KS2 and KS3, SSDD problems, KS3 maths games and 30 problem solving maths questions.
For children who need more support, our maths intervention programmes for KS3 achieve outstanding results through a personalised one to one tuition approach.
DO YOU HAVE STUDENTS WHO NEED MORE SUPPORT IN MATHS?
Every week Third Space Learning’s specialist online maths tutors support thousands of students across hundreds of schools with weekly online 1 to 1 maths lessons designed to plug gaps and boost progress.
Since 2013 these personalised one to 1 lessons have helped over 150,000 primary and secondary students become more confident, able mathematicians.
Learn how the programmes are aligned to maths mastery teaching or request a personalised quote for your school to speak to us about your school’s needs and how we can help.