Volume of a cone

GCSE Tutoring Programme

Our chosen students improved 1.19 of a grade on average - 0.45 more than those who didn't have the tutoring.

Teacher-trusted tutoring

In order to access this I need to be confident with:

Multiplying fractions Substitution Perimeter Area Rounding

This topic is relevant for:

GCSE Maths Geometry and Measure 3D Shapes Cone

Volume Of A Cone

Here we will learn about the volume of a cone, including how to calculate the volume of a cone given its radius and perpendicular height and how to calculate a missing length within a cone, given its volume.

There are also cone worksheets based on Edexcel, AQA and OCR exam questions, along with further guidance on where to go next if you’re still stuck.

What is the volume of a cone?

The volume of a cone is the amount of space inside a cone.

The volume of a cone is one third of the volume of a cylinder.

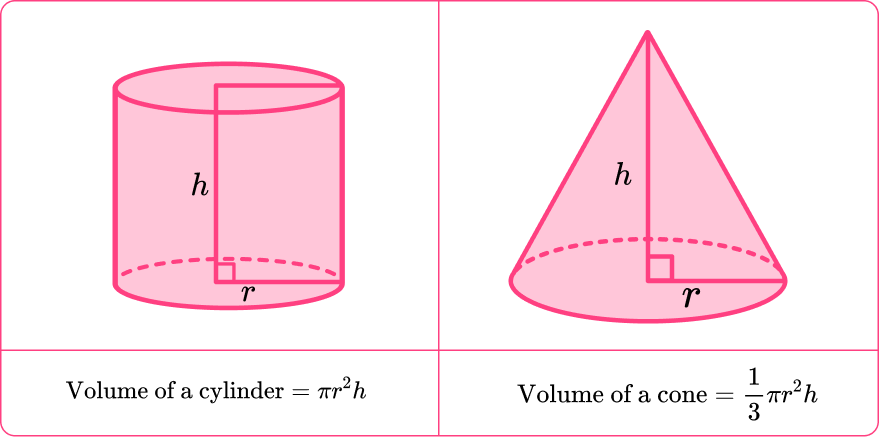
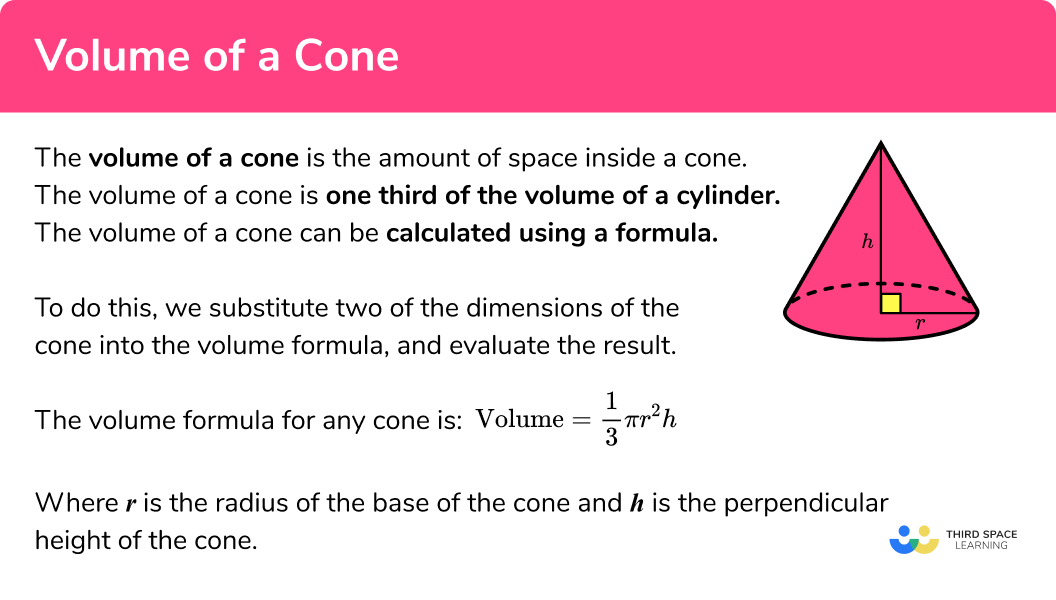
The volume of a cone can be calculated using a formula.

To do this, we substitute two of the dimensions of the cone into the volume formula and evaluate the result.

The volume formula for any cone is

$\text{Volume}=\frac{1}{3} \pi r^2 h$

where $r$ is the radius of the base of the cone and $h$ is the perpendicular height of the cone.

What is the volume of a cone?

Example

Find the volume of this cone where the radius of the base of the cone is $5cm$ and the perpendicular height of the cone is $8cm$ .

We need to find the area of the base of a cone using $A=\pi r^{2}$ (the area of a circle).

We then multiply it by the vertical height $h$ , and find one third of the answer.

\[\text{Volume}=\frac{1}{3} \pi r^2 h\\ = \frac{1}{3} \times \pi \times 5^2 \times 8\\ =\frac{200}{3}\pi\\ =209.4 \ cm^3 \ \text{(1 dp)}\]

Types of cones

A cone is a three dimensional object with a circular base that tapers to a point (a vertex). This vertex is also known as the apex.

If the apex is directly above the centre of the base, the cone is referred to as a right circular cone. If the apex is not above the centre of the base, this is referred to as an oblique cone.

Note: The slant height of the cone l is not needed to calculate the volume of a cone, but it is used to calculate the surface area of a cone.

Step-by-step guide: Surface area of a cone (coming soon)

The volume of a cone stays the same if the apex moves parallel to the base but the surface area of the cone changes. This is because the slant heights are not equal for an oblique cone.

How to calculate the volume of a cone

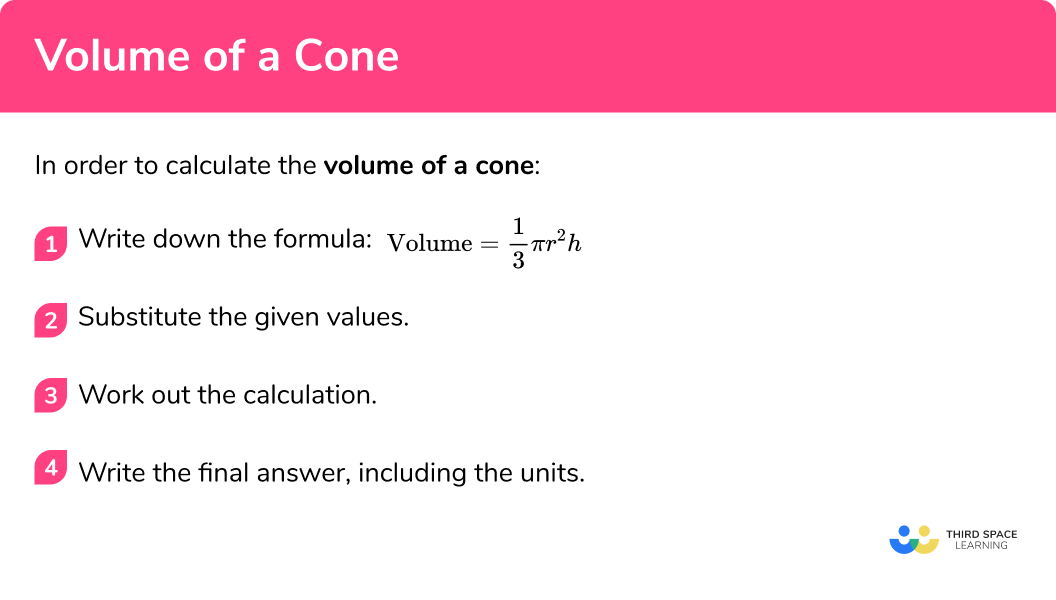
In order to calculate the volume of a cone:

Write down the formula, $\text{volume}=\frac{1}{3} \pi r^2 h$ .
Substitute the given values.
Work out the calculation.
Write the final answer, including the units.

How to calculate the volume of a cone

Volume of a cone worksheet

Get your free volume of a cone worksheet of 20+ questions and answers. Includes reasoning and applied questions.

DOWNLOAD FREE

Volume of a cone worksheet

Get your free volume of a cone worksheet of 20+ questions and answers. Includes reasoning and applied questions.

DOWNLOAD FREE

Related lessons on cones

Volume of a cone is part of our series of lessons to support revision on cone. You may find it helpful to start with the main cone lesson for a summary of what to expect, or use the step by step guides below for further detail on individual topics. Other lessons in this series include:

Volume of a cone examples

Example 1: integer dimensions

Find the volume of the cone with radius $4cm$ and perpendicular height $7cm$ .

Give your answer to $3$ significant figures.

Write down the formula, $\text{volume}=\frac{1}{3} \pi r^2 h$ .

The formula for the volume of a cone is $V=\frac{1}{3} \pi r^2 h$ .

2Substitute the given values.

We need to substitute the value of the radius $r$ and the perpendicular height $h$ into the formula.

V= \frac{1}{3}\times\pi\times{4^2}\times{7}

3Work out the calculation.

Use a calculator to work out the volume.

V=\frac{112}{3}\pi = 117.286…

4Write the final answer, including the units.

Check what form the final answer needs to be. Here we are asked to give the answer to $3$ significant figures.

V=117.286\dots=117 cm^3 (3sf)

The volume of the cone is $117cm^3 (3sf)$ .

Example 2: decimal dimensions

Find the volume of the cone with radius $2.1cm$ and perpendicular height $4.3cm$ .

Give your answer to $3$ significant figures.

Write down the formula, $\text{volume}=\frac{1}{3} \pi r^2 h$ .

The volume formula of a cone is $V=\frac{1}{3} \pi r^2 h$ .

Substitute the given values.

We need to substitute the value of the radius $r$ and the perpendicular height $h$ into the formula.

V= \frac{1}{3} \times \pi \times 2.1^2 \times 4.3

Work out the calculation.

Use a calculator to work out the volume.

V= 19.8580\dots

Write the final answer, including the units.

Check what form the final answer needs to be. Here we are asked to give the answer to $3$ significant figures.

V=19.8580\dots=19.9 cm^3 (3sf)

The volume of the cone is $19.9 cm^3 (3sf)$ .

Example 3: calculate the volume in terms of pi

Calculate the volume of a cone with radius $3cm$ and perpendicular height $5cm$ .

Write your answer in terms of $\pi$ .

Write down the formula, $\text{volume}=\frac{1}{3} \pi r^2 h$ .

The formula for the volume of a cone is $V=\frac{1}{3} \pi r^2 h$ .

Substitute the given values.

We need to substitute the value of the radius $r$ and the perpendicular height $h$ into the formula.

V= \frac{1}{3} \times \pi \times 3^2 \times 5

Work out the calculation.

As we can change the order of a multiplication and the solution stays the same, we have $V=\frac{1}{3}\times\pi\times{3^{2}}\times{5}=\frac{1}{3}\times{3^{2}}\times{5}\times{\pi}$ .

Simplifying this, we get $V=15\pi$ .

Write the final answer, including the units.

Check what form the final answer needs to be. Here we need to leave the answer in terms of $\pi$ .

V=15\pi\text{cm}^3

The volume of the cone is $15\pi cm^3$ .

Example 4: calculate the volume given the diameter

Find the volume of the cone with the diameter of $4cm$ and perpendicular height $6cm$ .

Write your answer in terms of $\pi$ .

Write down the formula, $\text{volume}=\frac{1}{3} \pi r^2 h$ .

The formula for the volume of a cone is $V=\frac{1}{3} \pi r^2 h$ .

Substitute the given values.

As the diameter is twice the length of the radius, the radius is equal to $4\div2=2cm$ .

Now we can substitute the values of $r$ and $h$ into the formula to get $V= \frac{1}{3} \times \pi \times 2^2 \times 6\$ .

Work out the calculation.

As we can change the order of a multiplication and the solution stays the same, we have $V=\frac{1}{3}\times\pi\times{2^{2}}\times{6}=\frac{1}{3}\times{2^{2}}\times{6}\times{\pi}$ .

Simplifying this, we get $V= 8\pi$ .

Write the final answer, including the units.

Check what form the final answer needs to be. Here we are asked to leave the answer in terms of $\pi$ .

V=8\pi\text{cm}^3

The volume of the cone is $8\pi cm^3$ .

Example 5: calculate a length given the volume

A cone has a volume of $500cm^3$ and a radius of $5cm$ . Calculate the perpendicular height of the cone, correct to $1$ decimal place.

Write down the formula, $\text{volume}=\frac{1}{3} \pi r^2 h$ .

The volume formula of a cone is $V=\frac{1}{3} \pi r^2 h$ .

Substitute the given values.

We are given the volume and the radius, so we can substitute these into the formula.

500=\frac{1}{3} \times \pi \times 5^2 \times h

As $5^2=25$ , we have $500=\frac{1}{3} \times \pi \times{25}\times h$ .

Work out the calculation.

We need to solve the equation for $h$ ,

Write the final answer, including the units.

Check what form the final answer needs to be. Here we are asked to give the answer to $1$ decimal place.

h = \frac{60}{\pi} = 19.1\text{cm\quad(1dp)}

The perpendicular height of the cone is $19.1 cm (1dp)$ .

Example 6: calculate the radius given the volume

A cone has a volume of $800cm^3$ and a perpendicular height of $15cm$ . Calculate the radius of the cone. Give your answer to $2$ decimal places.

Write down the formula, $\text{volume}=\frac{1}{3} \pi r^2 h$ .

The question involves the volume of a cone, so we need the formula for the volume of a cone.

V=\frac{1}{3} \pi r^2 h

Substitute the given values.

We are given the volume and the perpendicular height, so we can substitute these into the formula.

800=\frac{1}{3} \times \pi \times r^2 \times 15

Work out the calculation.

As $\frac{1}{3} \times15=5$ , we can simplify the right hand side of the equation, before we find the value of $r$ .

We now have $r^2={\frac{160}{\pi}}$ . To find the value of $r$ , we must square root both sides of the equation. By doing this we get $r=\sqrt{\frac{160}{\pi}}=7.136…$

Write the final answer, including the units.

Check what form the final answer needs to be. Here we are asked to give the answer to $2$ decimal places.

r=7.136\dots=7.14 cm (2dp)

The radius of the cone is $7.14cm (2dp)$ .

Common misconceptions

Using the correct formula

There are several formulas that can be used, so we need to match the correct formula to the correct context.

Rounding

It is important to not round the answer until the end of the calculation. This will mean your final answer is accurate.

Using the radius or the diameter

It is a common error to mix up radius and diameter. Remember the radius is half of the diameter.

Make sure you have the correct units
- For area we use square units such as $cm^2$ .
- For volume we use cube units such as $cm^3$ .

Practice volume of a cone questions

541 cm^3

542 cm^3

719 cm^3

718 cm^3

Substitute the values of $r$ and $h$ into the formula.

V=\frac{1}{3}\pi r^2 h

V=\frac{1}{3}\times \pi \times 7.3^2\times 9.7

V=541.310\dots

V=541 \ cm^3 \ \text{(3sf)}

562 cm^3

563 cm^3

611 cm^3

612 cm^3

Substitute the values of $r$ and $h$ into the formula.

V=\frac{1}{3}\pi r^2 h

V=\frac{1}{3}\times \pi \times 8.6^2 \times 7.9

V=611.860\dots

V=612 \ cm^3 \ \text{(3sf)}

42\pi cm^3

84\pi cm^3

126\pi cm^3

252\pi cm^3

We are finding the volume of a cone so we substitute the values of $r$ and $h$ into the formula.

$V=\frac{1}{3}\pi r^2 h\\$
$V=\frac{1}{3}\times \pi \times 6^2 \times 7\\$
$V=84\pi\\$
$V=84\pi \ cm^{3}$

12\pi cm^3

32\pi cm^3

48\pi cm^3

96\pi cm^3

Calculate the value of the radius by dividing the diameter by $2$ , then substitute the values of $r$ and $h$ into the formula and solve.

r=8 \div 2= 4cm

V=\frac{1}{3} \pi r^2 h

V=\frac{1}{3}\times \pi \times 4^2 \times 6

V=32\pi

V=32\pi \ cm^3

3.5 cm

8.2 cm

10.6 cm

11.1 cm

Substitute the volume and the radius into the volume formula and then rearrange to find the perpendicular height, $h$ .

V=\frac{1}{3} \pi r^2 h

400=\frac{1}{3}\times \pi \times 6^2 \times h

1200=\pi \times 36 \times h

\frac{1200}{\pi \times 36}=h

h=10.610\dots

h=10.6 \ cm \ \text{(1dp)}

5.40 cm

5.72 cm

9.14 cm

9.28 cm

Substitute the values of the volume and the perpendicular height into the formula, and rearrange to find the radius $r$ .

V=\frac{1}{3} \pi r^2 h

700=\frac{1}{3}\times \pi \times r^2 \times 8

2100=\pi \times r^2 \times 8

\frac{1200}{\pi \times 8}=r^2

r=\sqrt{\frac{2100}{\pi \times 8}}

r=9.1409\dots

r=9.14 \ cm \ \text{(to 2 dp)}

Volume of a cone GCSE questions

$\text{Volume of a cone}=\frac{1}{3} \pi r^2 h$

1. Below is a cone with radius $4.1 cm$ and perpendicular height $5.8 cm$ .

Calculate the volume of the cone.

Give your answer to $3$ significant figures.

(2 marks)

Show answer

V=\frac{1}{3} \times \pi \times 4.1^2 \times 5.8

(1)

102.099\dots=102

(1)

2. Use the information in the diagram below to calculate the volume of the 3D shape.

Write your answer in terms of $\pi$ .

(2 marks)

Show answer

V=\frac{1}{3} \times \pi \times 8^2 \times 12

(1)

256\pi

(1)

3. A container is a cone of radius $30 cm$ and perpendicular height $50 cm$ .

Water fills the container at a rate of $3000 cm^3$ per minute.

How long does it take to fill the container?

Give your answer to the nearest minute.

(4 marks)

Show answer

\frac{1}{3} \times \pi \times 30^2 \times 50

(1)

= 47 123.8898\dots

(1)

47 123.8898… \div 3000=15.707…

(1)

$15.707\dots = 16$ minutes

(1)

Learning checklist

You have now learned how to:

Calculate the volume of a cone

The next lessons are

Still stuck?

Prepare your KS4 students for maths GCSEs success with Third Space Learning. Weekly online one to one GCSE maths revision lessons delivered by expert maths tutors.