Rounding decimals

One to one maths interventions built for KS4 success

Weekly online one to one GCSE maths revision lessons now available

Learn more

In order to access this I need to be confident with:

Place value Decimal places Rounding to the nearest 10 100 and 1000

This topic is relevant for:

GCSE Maths Number Rounding Numbers

Rounding Decimals

Here we will learn about rounding decimals, including how to round to one, two and three decimal places.

There are also rounding decimals worksheets based on Edexcel, AQA and OCR exam questions, along with further guidance on where to go next if you’re still stuck.

What is rounding decimals?

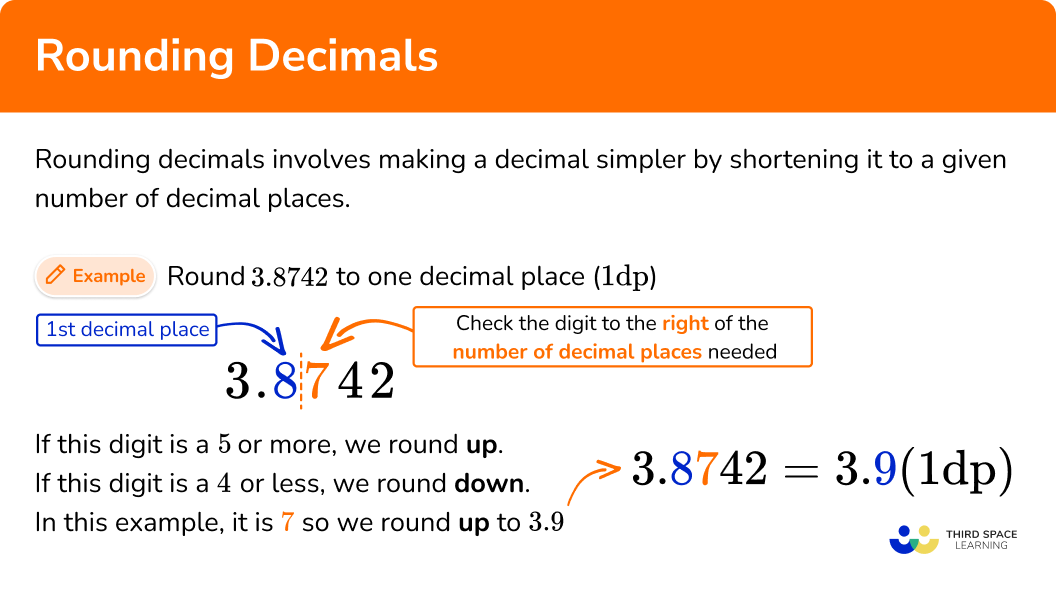
Rounding decimals involves making a decimal simpler by shortening it to a given number of decimal places.

To do this we consider the digit to the right of the number of decimal places required and round it as follows,

If the digit is a $\bf{5}$ or more, we round up.

If the digit is a $\bf{4}$ or less, we round down.

Let’s look at rounding $3.8742$ to one decimal place. This means we need one decimal place only in our answer, so our answer will be either rounded down to $3.8$ or rounded up to $3.9.$ We use rounding rules to help us to decide whether $3.8742$ is closer to $3.8$ or $3.9.$

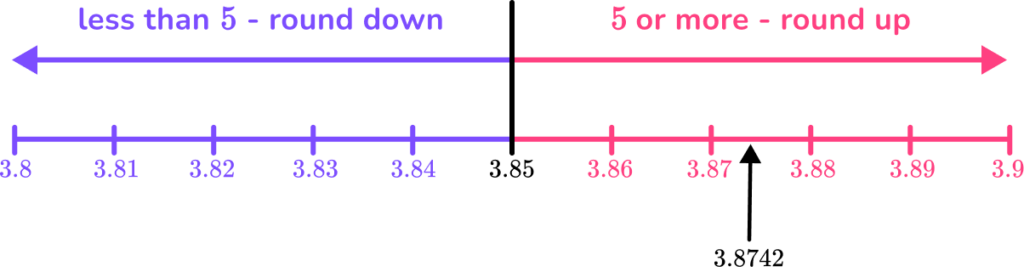
In this case, we look at the $7$ to help us to decide.

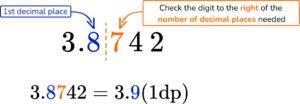
$7$ is more than $\bf{5},$ so we round up to $3.9.$

Now let’s look at rounding $3.8742$ to two decimal places.

We need two decimal places in our answer, so our answer will either be rounded down to $3.87$ or rounded up to $3.88$ .

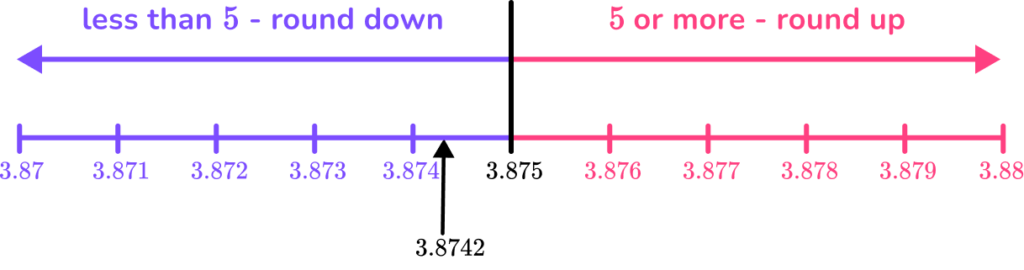
In this case, we look at the $4$ to help us to decide.

$4$ is less than $\bf{5},$ so we round down to $3.87.$

By rounding a number, we lose the accuracy of the number. Rounding can therefore be used to estimate calculations where rounding to the nearest whole number can make mental calculations easier to compute.

Step-by-step guide: Estimation

What is rounding decimals?

How to round decimals

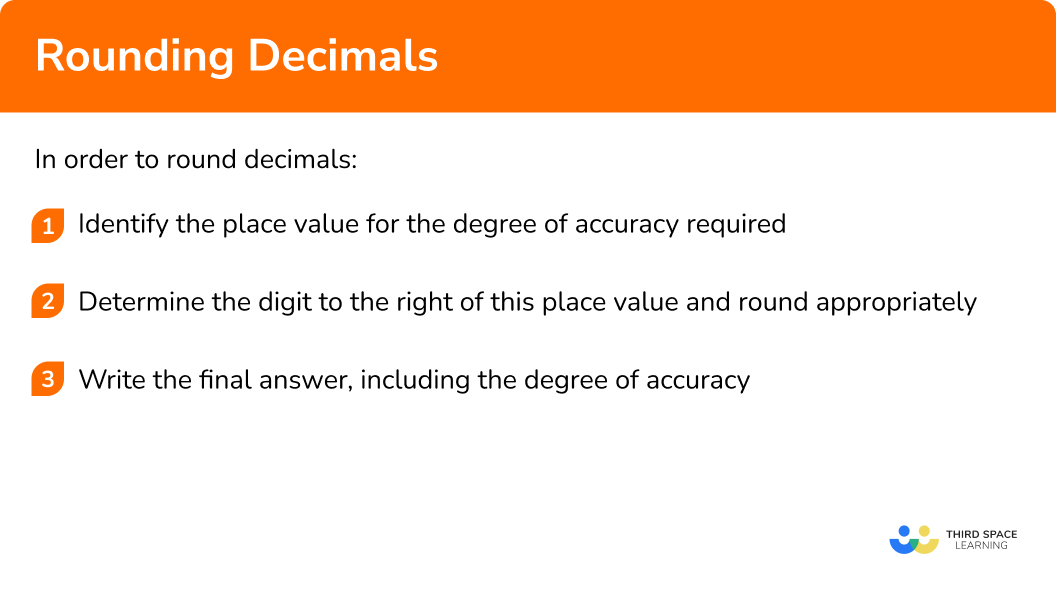
In order to round decimals:

Identify the place value for the degree of accuracy required.
Determine the digit to the right of this place value and round appropriately.
Write the final answer, including the degree of accuracy.

Explain how to round decimals

Rounding decimals worksheet

Get your free rounding decimals worksheet of 20+ questions and answers. Includes reasoning and applied questions.

DOWNLOAD FREE

Rounding decimals worksheet

Get your free rounding decimals worksheet of 20+ questions and answers. Includes reasoning and applied questions.

DOWNLOAD FREE

Rounding decimals examples

Example 1: round to 1 decimal place

Round $9.37$ to $1$ decimal place.

Identify the place value for the degree of accuracy required.

Here we need to round to $1$ decimal place.

2Determine the digit to the right of this place value and round appropriately.

The number to the right of the $1$ st decimal place is $7.$

$7$ is greater than $5$ so we need to round up by changing the $3$ to a $4$ and discarding any subsequent values.

3Write the final answer, including the degree of accuracy.

$9.37 = 9.4$ to $1$ decimal place.

We can also write this as $9.4 \ (1dp).$

Example 2: round to 1 decimal place (nearest tenth)

Round $14.4293$ to the nearest tenth.

Identify the place value for the degree of accuracy required.

The tenths place value is the first digit after the decimal point so we are being asked to round to $1$ decimal place.

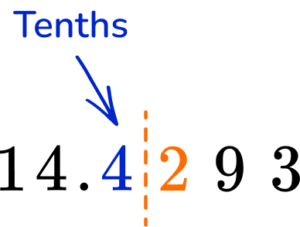
Determine the digit to the right of this place value and round appropriately.

The digit in the second decimal place is a $2. \ 2$ is less than $5$ so we need to round down. This will mean that the value $4$ will stay the same and all subsequent values are discarded.

Write the final answer, including the degree of accuracy.

$14.4293 = 14.4$ to the nearest tenth.

Example 3: round to 2 decimal places

Round $13.612$ to $2$ decimal places.

Identify the place value for the degree of accuracy required.

Here we need to round to $2$ decimal places.

Determine the digit to the right of this place value and round appropriately.

The digit to the right of the second decimal place is a $2. \ 2$ is less than $5$ so we need to round down by keeping the digit in the second decimal place $1$ the same and discarding the subsequent values.

Write the final answer, including the degree of accuracy.

$13.612 = 13.61$ to $2$ decimal places.

We can also write this as $13.61 \ (2dp).$

Example 4: round to 2 decimal places

Round $84.59821$ to the nearest hundredth.

Identify the place value for the degree of accuracy required.

The hundredths place is the second digit after the decimal point so we are being asked to round to $2$ decimal places.

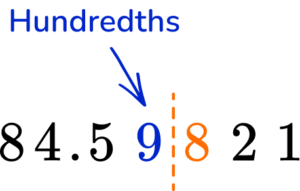
Determine the digit to the right of this place value and round appropriately.

The digit to the right is $8$ so we need to round up. The number that we need to increase is $9$ so increasing it gives us $10.$ The $1$ is carried over to the tenths and the hundredths value becomes zero.

Write the final answer, including the degree of accuracy.

$84.59821 = 84.60$ to the nearest hundredth.

Notice that we need to include the zero in the second decimal place so that the answer is given to $2$ decimal places.

Example 5: round to 3 decimal places

Round $23.5325$ to $3$ decimal places.

Identify the place value for the degree of accuracy required.

Here we need to round to $3$ decimal places.

Determine the digit to the right of this place value and round appropriately.

The digit to the right is a $5.$ We therefore have to round up by increasing the $2$ to $3$ and discarding subsequent values.

Write the final answer, including the degree of accuracy.

23.5325 = 23.533 \ (3dp)

Example 6: round to 3 decimal places

Round $132.6794215$ to the nearest thousandth.

Identify the place value for the degree of accuracy required.

The thousandths place is the third digit after the decimal point so we are being asked to round to $3$ decimal places.

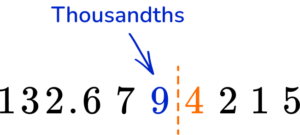
Determine the digit to the right of this place value and round appropriately.

The digit to the right is a $4. \ 4$ is less than $5$ so we need to round down by leaving the $9$ the same and discarding subsequent values.

Write the final answer, including the degree of accuracy.

$132.6794215 = 132.679$ to the nearest thousandth.

Common misconceptions

Decreasing the value of the decimal place when rounding down

When rounding down, the value of the decimal place stays the same, it doesn’t decrease. For example, when rounding $4.62$ to one decimal place, the $2$ indicates that we need to round down. The correct answer is $4.6$ not $4.5.$

Leaving out zeros

When a $9$ is increased to $10$ and the one rolls over, it is important to include the zeros so that you are giving the answer to the correct number of decimal places. For example, $4.597$ to two decimal places is $4.60$ rather than $4.6.$

Changing extra digits

It is only the last digit that should be changed when rounding up. For example, $4.318$ to two decimal places is $4.32,$ not $4.42.$

Not discarding subsequent values

Once we have decided whether to round up or down we can discard all subsequent values. For example, $7.693012$ to $1$ decimal place is $7.7,$ not $7.793012$ or $7.700000.$

Rounding decimals is part of our series of lessons to support revision on rounding numbers, and upper and lower bounds. You may find it helpful to start with the main rounding numbers lesson for a summary of what to expect, or use the step by step guides below for further detail on individual topics. Other lessons in this series include:

Practice rounding decimals questions

8.4

8.5

8.50

9.5

We need to round to $1$ decimal place. The digit to the right is $9$ so we round up by increasing the $4$ to a $5$ and discarding subsequent values.

$8.49 = 8.5$ to $1$ decimal place.

15.7

15.74

15.70000

15.8

The tenths digit is the first digit to the right of the decimal point so we need to round to $1$ decimal place. The next digit to the right is $1$ so we round down by leaving the $7$ the same and discarding subsequent values.

$15.74103 = 15.7$ to the nearest tenth.

7.57

7.68

7.58

7.60

We need to round to $2$ decimal places. The digit to the right is $9$ so we need to round up by increasing the $7$ to an $8$ and discarding subsequent values.

7.579 = 7.58 \ (2dp)

32.82

32.83

32.82000

32.81

The hundredths digit is the second digit after the decimal point so we need to round to $2$ decimal places. The digit to the right is $0$ so we need to round down by leaving the $2$ the same and discarding subsequent values.

$32.82049 = 32.82$ to the nearest hundredth.

0.065

0.063

0.0641

0.064

The thousandths digit is the third digit after the decimal point so we need to round to $3$ decimal places. The digit to the right is $1$ so we need to round down by leaving the $4$ the same and discarding subsequent values.

0.0641 = 0.064 \ (3dp)

14.750

14.749

14.75

14.800

We need to round to $3$ decimal places. The digit to the right is an $8$ which means we need to round up by increasing the $9$ to a $10,$ carrying the $1,$ and discarding subsequent values.

$14.7498123 = 14.750$ to the nearest thousandth.

Rounding decimals GCSE questions

1. Round the number $132.4672$ to one decimal place.

(1 mark)

Show answer

132.5

(1)

2. (a) Calculate $\sqrt{47.5}.$ Write down all the digits on your calculator display.

(b) Round your answer to two decimal places.

(2 marks)

Show answer

(a) $6.892024376$

(1)

(b) $6.89$

(1)

3. Use the formula $C=\pi{d}$ to find the circumference $C$ of a circle with a diameter $d = 10 \ cm.$

Round your answer to $2$ decimal places.

(3 marks)

Show answer

C=\pi\times{10}

(1)

=31.41592654

(1)

=31.42 \ cm \ (2dp)

(1)

Learning checklist

You have now learned how to:

Round numbers and measures to an appropriate degree of accuracy, for example, to a number of decimal places

The next lessons are

Still stuck?

Prepare your KS4 students for maths GCSEs success with Third Space Learning. Weekly online one to one GCSE maths revision lessons delivered by expert maths tutors.