One to one maths interventions built for KS4 success
Weekly online one to one GCSE maths revision lessons now available
In order to access this I need to be confident with:
Decimals Rounding numbers to one or more significant figures BIDMASThis topic is relevant for:

Estimation
Here we will learn about estimation in maths including its definition and how to estimate a calculation. You’ll also learn about the differences between decimals and significant figures and how to round numbers to one significant figure.
There are estimation worksheets based on Edexcel, AQA and OCR exam questions, along with further guidance on where to go next if you’re still stuck.
What is estimation in maths?
Estimation is when we use approximate values in a calculation to give an approximate, predicted answer rather than an exact answer.
Estimating calculations makes them more manageable by using round numbers.
E.g.
If we wanted to estimate the answer to 46.75 x 3.218 we could round both these numbers and use an estimated calculation of 50 x 3 = 150
Estimation from sample
A population includes everyone within a large group, for example, the population of a country includes everyone that lives within that country.
A sample is a smaller group, taken from the population, for example a sample of 100 people could be taken from the total population.
Samples are used when researching populations because it is usually too costly or too time consuming to carry out research on the entire population. This means that the sample must be selected carefully so that it represents the population as closely as possible.
One of the best methods of selecting a sample is using a stratified sampling technique. This allows the researcher to determine the sample based on the proportional size of different subcategories within the population and then randomly select individuals for participation in the research.
Step-by-step guide: Stratified sampling
Once the individuals within the sample have been selected, we can carry out statistical analysis on the sample such as the mean. The sample mean is the average of the sample, whilst the population mean is the average of the population. If the sample is selected carefully, we can estimate the population mean by calculating the sample mean. Including more individuals within the sample will provide a more accurate value for an estimate of the population mean. This is because the value of the sample mean value tends towards the population mean as the sample size increases.
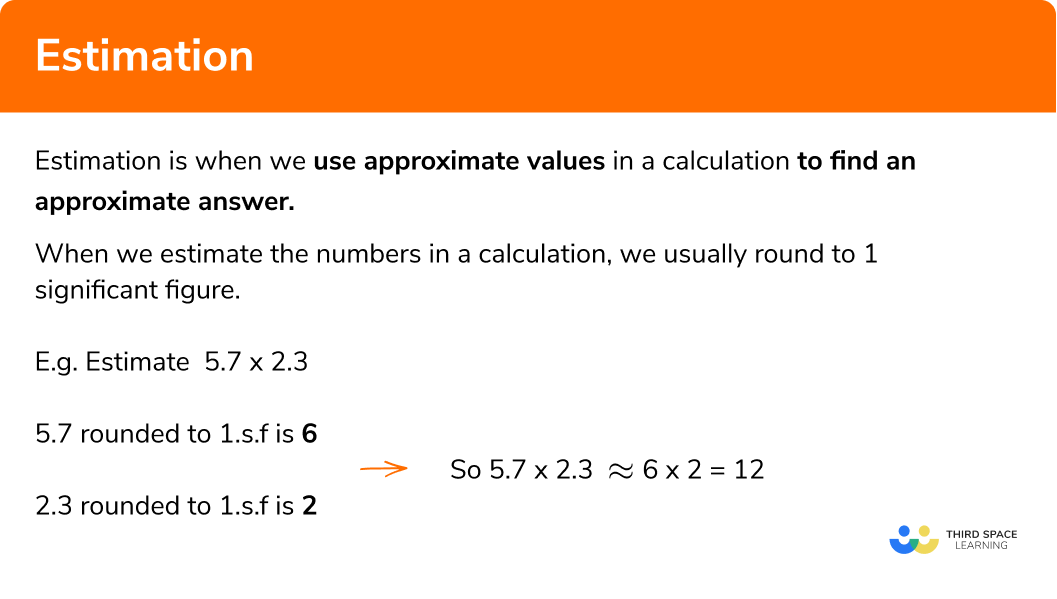
Estimating calculations
When we estimate the numbers in a calculation, we usually round the numbers to
E.g.
Let’s estimate 5.7 ÷ 1.8
First we estimate the value of
We can see that the first significant figure is
This means we need to use the digit to the right of the
So
Then we follow the same process to estimate the value of 1.8 which rounded to 1 significant figure is 2.
Once we have estimated all the values in a calculation we can work out the estimated answer so
5.7 ÷ 1.8 ≈ 6 ÷ 2 = 3
Estimation is used in lots of different areas of maths in order to give an approximate answer to a question, such as estimating the perimeter of a shape or estimating the size of a number written in standard form. We also see examples of estimation all around us, such as estimating the number of bricks will be needed to build a garden wall, or working out if you have enough leftover from £5 in a supermarket to buy a bar of chocolate at the till.
What is estimation?
Key points about estimating calculations
- An estimate is an approximate answer; not the exact answer
- Estimation relies on using easier numbers that are near to the actual numbers involved
- To estimate we usually round to 1 significant figure
- We use estimation in many aspects of life and work – for a building job or planning a budget
- When we are estimating calculations we still have to consider using the order of operations or BIDMAS principles
- ≈ means approximately equal to; = means exactly equal to
How to estimate
In order to estimate a number:
- Round each number to
1 significant figure. - Construct the new estimated calculation using the ‘approximately equal to’
symbol≈ . - Calculate using the rounded numbers to give an approximate answer and consider units in the answer.
Explain how to estimate a calculation in 3 steps


Estimation worksheet

Get your free estimation worksheet of 20+ questions and answers. Includes reasoning and applied questions.
DOWNLOAD FREE
Estimation worksheet

Get your free estimation worksheet of 20+ questions and answers. Includes reasoning and applied questions.
DOWNLOAD FREEEstimation examples
Example 1: (typical foundation GCSE question using the GCSE command word language and no units involved)
Estimate the value of:
- Round numbers to one significant figure
2 Write out the new estimated calculation
3 Complete the calculation using estimation
If we compare this to the exact answer which is
Example 2: worded question
Brian buys 197 packets of crisps for a party. Each bag costs 45p. Use estimation to find the approximate cost in pounds £.
Round numbers to one significant figure
Write out the new estimated calculation
Complete the calculation using estimation
In this case we have to consider the units used
If we compare this to the exact answer which is
Example 3: multi-step calculations
Round numbers to one significant figure
Write out the new estimated calculation
Complete the calculation using estimation
If we compare this to the exact answer which is
Example 4: estimate using BIDMAS principles
Estimate the value of
You must show your working.
Round numbers to one significant figure
Consider the order of operations BIDMAS
Multiply 20 and 3 together first
Then follow the calculation from left to right, as it doesn’t matter which operation you do first with addition and subtraction.
Complete the calculation using estimation
If we compare this to the exact answer which is
Example 5: worded problem requiring reasoning
Estimate how many toys costing
Round numbers to one significant figure
Construct the estimated calculation
Complete the calculation
Consider units within a worded problem in context
The question considered both numbers in pounds but the answer should be a whole number of toys.
The estimate number of toys that could be bought is
If we compare this to the exact answer which is
Example 6: more complex calculation
Write down an estimate for:
Decide which square numbers are closest to 70
Decide which two squares 70 lies between and which one it is closest to
lies between
And
So,
is just under
Complete the calculation using estimation
Example 7: dividing by decimals
Estimate the value of:
Round numbers to one significant figure
Construct the estimated calculation
Complete the calculation using estimation
If we compare this to the exact answer which is
Common misconceptions
- It is common to find the actual calculation rather than use estimation
Estimation relies on rounding to significant figures to simplify the calculation.
An estimate of
- The first significant figure is the first non-zero digit
E.g.
The first significant figure of
E.g.
The first significant figure of
E.g.
The first significant figure of
- Dividing by a decimal gives a larger number
E.g.
- Significant figures and decimal places
Rounding to one decimal place relies on what happens after the first digit after the decimal point, whereas the first significant figure is the
E.g.
Although
- Estimate with fractions
We can also estimate with fractions by first converting the fraction to a decimal and then rounding the numbers to 1 significant figure.
- Estimation with graphs
We can use graphs to estimate values by using a line of best fit and extrapolating.
Did you know?
The word estimation comes from the Middle English word aestimatio which means a valuation.
Related lessons on estimation
Estimation is part of our series of lessons to support revision on rounding numbers. You may find it helpful to start with the main rounding numbers lesson for a summary of what to expect, or use the step by step guides below for further detail on individual topics. Other lessons in this series include:
Practice estimation questions
1. Estimate:
7.2 \times 98
720

700

705.6

706

Remember to round each number to one significant figure, then calculate
\begin{aligned} 7.2 \times 98 &\approx 7 \times 100\\\\ 7 \times 100 &= 700 \end{aligned}
2. Estimate the value of:
\frac{907}{3.02}
300.33

305

300

302

Remember to round each number to one significant figure, then calculate
\begin{aligned} \frac{907}{3.02} & \approx \frac{900}{3} \\\\ \frac{900}{3} &=300 \end{aligned}
3. Estimate the cost of 7.4 litres of petrol costing 123p per litre
\pounds 8.00

\pounds 7.00

\pounds 9.10

\pounds 7.40

We round the number of litres to one significant figure, and the price per litre to one significant figure.
\begin{aligned} 7.4 \times 123 &\approx 7 \times 100 \\ 7 \times 100&=700 p \text { or } \pounds 7.00 \end{aligned}
4. Estimate the value of:
\frac{23 \times 494}{54}
210.407

200

225.4

210

Remember to round each number to one significant figure, then calculate
\begin{aligned} \frac{23 \times 494}{54} & \approx \frac{20 \times 500}{50} \\\\ \frac{20 \times 500}{50} &=\frac{10000}{50} \\\\ \frac{10000}{50} &=200 \end{aligned}
5. Estimate how many packets of seeds costing \pounds 1.84 each can be bought for \pounds 20 ?
11

10

9

10.87

Remember to round each number to one significant figure, then calculate
\begin{array}{l} 1.84 \approx 2\\20 \div 2 = 10 \text{ packets} \end{array}
6. Estimate:
\frac{609 \times 4.63}{0.51}
6000

3000

5529

5528

Remember to round each number to one significant figure, then calculate
\begin{aligned} \frac{600 \times 5}{0.5}&=\frac{3000}{0.5} \\\\ \frac{3000}{0.5}&=6000 \end{aligned}
7. Estimate:
\sqrt{30}




The nearest square numbers are 25 and 36
\sqrt{25}=5 \text { and } \sqrt{36}=6
so the estimate lies between both.
30 is slightly closer to 25 than 36 so an estimate will be 5.4 .
Estimation GCSE questions
1. Anna says that:
472 \div 5 = 9.44
Use estimation to show that Anna cannot be correct.
(3 Marks)
(1)
500 \div 5 = 100(1)
The answer should be near 100
(1)
2. Oliver wants to buy the following items for his garden:
5 packs of turf at \pounds 91 per pack
30 paving slabs at \pounds 5.81 each
4 fruit trees at \pounds 16.50 each
Estimate the total cost.
(3 Marks)
(1)
\begin{aligned} 5 \times \pounds 90 &= \pounds 450 \\30 \times \pounds 6 &= \pounds 180 \\4 \times \pounds 20 &= \pounds 80 \end{aligned}(1)
\pounds 450 + \pounds 180 + \pounds 80 = £710(1)
3. (a) Estimate:
\frac{31 \times 3.96}{0.47}
(b) Estimate:
\sqrt{5.3 \times 8.9}
(6 Marks)
(a)
\frac{31 \times 3.96}{0.47} \approx \frac{30 \times 4}{0.5}
(1)
\frac{30 \times 4}{0.5} = \frac{120}{0.5}(1)
\frac{120}{0.5} = 240(1)
(b)
\sqrt{5.3 \times 8.9} \approx \sqrt{5 \times 9}
(1)
\sqrt{5 \times 9} = \sqrt{45}(1)
\begin{array}{l} \sqrt{36} = 6, \sqrt{49} = 7\\\\ \sqrt{45} \approx 6.7 \end{array}
(accept 6.6-6.8)
(1)
Learning checklist
You have now learned how to:
- Estimate by rounding using significant figures
- Estimate within the context of a worded problem, considering units
- Estimate using multiple steps
- Estimate considering BIDMAS principles
- Estimate using square roots
The next lessons are
Still stuck?
Prepare your KS4 students for maths GCSEs success with Third Space Learning. Weekly online one to one GCSE maths revision lessons delivered by expert maths tutors.

Find out more about our GCSE maths tuition programme.