What Does Greater Depth Look Like In KS2 Maths? Tips On How To Bring Greater Depth Learning Into The Primary Classroom
Figuring out what greater depth looks like in maths can often prove difficult, but the power of teaching in depth should certainly not be underestimated. This blog will discuss why greater depth teaching is important, and give you some tips as to how you can introduce it into your classroom, and provide you with a free Year 6 Greater Depth Maths resource!
Depth has become a word commonly associated with Teaching and Learning for Mastery (TLfM) in maths, yet it is something every maths teacher has always valued, prized and promoted.
But what does depth actually mean?
Just how deep is deep?
We might associate depth in maths along the same lines as diving into the ocean, like some sort of Neptune submersible where we can explore new worlds and uncover hidden treasures.
In a way this is true, but the shallow end might still be considered deep for some learners still building their confidence.
However, shallow learning definitely isn’t surface learning.
The shallow end can be pretty deep when you are learning to swim.
Something that is important to remember is that depth then is relative to your maths experience and confidence.
If you are a non-swimmer then the shallow end is deep, and this is certainly the case in the world of maths.
108 Free Always, Sometimes, Never Greater Depth Questions For KS2
Looking for a quick and easy way to introduce greater depth learning into the classroom? These 108 free questions are a simple way to open a classroom discussion amongst your pupils!
When we think about depth from a learner’s perspective rather than a teacher’s one, we begin to appreciate that it is not necessarily about diving – it might mean just putting your face in the water.
So What Does Greater Depth Look Like In Maths?
Any discussions about what greater depth looks like in maths has to take into account the three R’s of maths: reflecting, representing and reporting, and they are:
- Reflecting: Children need the breathing space to reflect on an experience when deepening their knowledge and understanding. Pausing, probing and pondering all happen when children are not rushed or pressured. Slowing down allows children to take control of their learning and become aware of their own learning too.
- Representing: Children need plenty of opportunities to represent their learning in an active way so deepening becomes memorable. This might be constructing a model, drawing a mathematical picture, using maths manipulatives or writing down their thoughts.
- Reporting: Children have to engage in meaningful maths talk with others – maths has to involve lots of verbal back and forth. Pupils refine, consolidate and develop their understanding by entering into learning conversations with their peers and teacher(s). This type of reporting helps children to realise that their thoughts are valuable and by talking together they can clarify their understanding.
These 3 R’s feed into depth and understanding and classroom activities must integrate them.
How Does Understanding Come Into Greater Depth?
A word about understanding: the goal of learning more about something in greater depth is to gain a greater understanding. But ‘understanding’ can express a fixed sense of closure and completeness which is not always the case in maths.
When we teach for mastery we ‘work towards a fuller understanding’ rather than a complete one, because there are always layers of complexity that feed into our learning.
The maths journey is never complete.
Greater Depth Is Different For Everybody
The good news is that no one agrees what greater depth looks like. However, that’s also the bad news.
Teachers long for a recipe or a book that tells them exactly what needs to be done but that doesn’t exist.
Greater depth is what works for your children, and as their teacher you know them best!
Exemplification materials might be available, however these do not dictate a particular method of teaching and what depth should look like.
Confusingly, greater depth is a hazy concept and should not be seen as a replacement for high attainers because it isn’t.
The language of judgment might link HA with greater depth, but greater depth applies to all children.
If there is one thing we have learned from the mastery maths approach, it is that mastery is an ill-defined approach with many levels of interpretation as to what depth looks like.
What we do know though is that all lessons are deep lessons if we push the boundaries for every pupil.
Greater Depth Through Problems Requiring Multiple Content Domains
When planning a sequence of lessons – or indeed a single lesson – to encourage mastery with greater depth, it is worthwhile to include a range of problems that touch upon content domains other than the content domain that particular lesson or sequence of lessons focuses upon.
It is worth remembering that the NCETM’s definition of mastery includes having a “sufficient depth of knowledge and understanding to reason and explain mathematical concepts and procedures and use them to solve a variety of problems” and that greater depth means to then go on and “independently explore and investigate mathematical contexts and structures, communicate results clearly and systematically explain and generalise the mathematics.”
The surface structure remains the same, but the deeper structure alternates
Craig Barton – when considering how to interweave multiple content domains into a lesson or lesson sequence with a given content domain focus – suggests keeping the surface structure the same whilst altering the deep structure of questions. So – continuing the example Craig uses in his podcast discussion – if the learning objective (and content domain focus) was calculating a missing angle in a triangle, you could keep the surface structure the same – triangles – but present a variety of problems which included measuring angles in triangles (drawn to scale), calculating the area and/or perimeter of triangles, as well as problems that would encourage revision or retrieval of defining facts used to classify triangles.
Equally – it could mean figuring out ways of writing single questions which touch upon multiple content domains.
For example – if we continue to think about triangles but now focusing on calculating the area of a triangle – we could first present a problem-solving question that requires pupils calculating the area of a triangle, and then figuring out what its area would be following a percentage increase or decrease. That question could be followed by a question requiring the calculation of a triangle’s area when it has sides of one or two decimal places. Pupils could then be asked a further question – they could be presented with a parallelogram with missing but calculable dimensions with the task of calculating its area too.
Here, we would be allowing children ample opportunity to practice the focus content domain – calculating the area of triangles – whilst touching upon multiplying numbers up to two decimal places, percentage increases/decreases, calculating missing sides in a shape, and moving onto calculating the area of a parallelogram (seeing whether children can devise the most efficient strategy for doing so).
By choosing to vary the deeper structure of the questions or activities our pupils encounter in their maths lessons, we are giving them the opportunity solve a variety of problems, allowing them further opportunities to revisit topics they have covered earlier in the year or earlier in their learning journey – key to helping them on their pathway to mathematical mastery by ensuring advantageous spacing and interleaving of topics in our maths schemes of learning. Furthermore, by presenting problems touching upon multiple content domains within one lesson or sequence of lessons, pupils have the opportunity to “independently explore and investigate mathematical contexts and structures” thereby pushing beyond mastery and into greater depth.
How To Make Greater Depth Learning Inclusive For All
It is often said that mastery and greater depth in mathematics is not practising the same concept with bigger numbers. That is true, numbers don’t have to be big to be challenging.
One thing I have always tried to do to help children develop greater depth is to get them to ‘milk the maths’.
‘Milking the maths’ is a term I used to help children see there was always more maths to ‘drain’ or ‘tap’ than first appeared.
I’ve heard it referred to as simple complexity i.e. taking something simple but developing it, but ‘milking the maths’ seems to garner the most attention in the classroom!
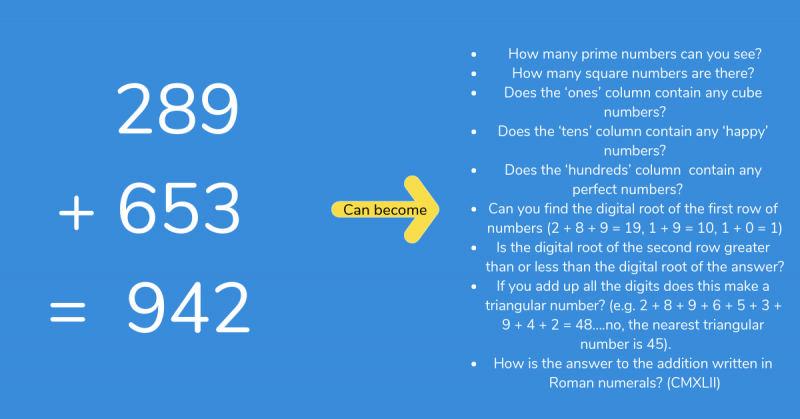
We can look at an addition calculation as an example: 289 + 653
289
+ 653
= 942
Give children this to do and it is just bog-standard stuff. There is nothing spectacular about it and life moves on to the next addition problem.
Press pause.
There is a fair bit you can do with the numbers here to explore the depths.
How You Can Dive Into This Seemingly Simple Question In More Detail
If all children can ‘do’ the addition, have they mastered it?
On the surface, it would appear so, but it tells you absolutely nothing about their knowledge and understanding of the numbers involved.
Greater depth in maths in this instance means interacting with the numbers and getting to know them. You could ask a whole range of questions:
- How many prime numbers can you see?
- How many square numbers are there?
- Does the ‘ones’ column contain any cube numbers?
- Does the ‘tens’ column contain any ‘happy’ numbers?
- Does the ‘hundreds’ column contain any perfect numbers?
- Can you find the digital root of the first row of numbers (2 + 8 + 9 = 19, 1 + 9 = 10, 1 + 0 = 1)
- Is the digital root of the second row greater than or less than the digital root of the answer?
- If you add up all the digits does this make a triangular number? (e.g. 2 + 8 + 9 + 6 + 5 + 3 + 9 + 4 + 2 = 48….no, the nearest triangular number is 45).
- How is the answer to the addition written in Roman numerals? (CMXLII)
Alternatively, going deeper might involve just one question. For example,
- Is the answer to the addition sum a composite number? Explain your answer as fully as you can.
This type of question obviously places more demand on children and so, if they did not know already, they would need to find out about composite numbers and explain further.
A possible answer might be:
A composite number is a number that can be divided evenly by more numbers than 1 and itself.
The number 942 can be evenly divided by 1, 2, 3, 6, 157, 314, 471 and 942 with no remainder.
This might be too deep for some children so another question could be:
- Add up the digits in each corner of the sum (2,9,9,2) and tell me whether this is a composite number? Does it have any other names?
A possible answer for this question might be:
2 + 9 + 9 + 2 = 22
A composite number is not a prime number. This type of number has more than two factors.
Its factors are 1, 2, 11 and 22.
22 is also a palindrome. A palindromic number is number that remains the same when its digits are reversed.
I am a big believer in children ‘knowing their numbers’ even if this means stepping outside of the curriculum to include number types that they probably will not ‘meet’ until further into their maths careers.
Why restrict children to knowing just a few types?
Children gain number confidence by being given opportunities to learn more about the different names we give to numbers, so greater depth in maths for me involves meeting numbers and getting to know them.
It is your decision how far to take number introductions, but I have had Year 6 classes learn about the following: natural, integers, prime, composite, square, triangular, cube, Fibonacci, pentagonal, happy, frugal, equidigital, lucky, deficient, perfect, abundant, palindromic, etc.
Children love learning about numbers and they need to know that some numbers may be more than one type. This can then lead into true-false statements about number types themselves, e.g.
True or False: An integer is also a rational number.
Answer: Since any integer can be formatted as a fraction by putting it over 1, then this statement is true.
For anyone who says this cannot be done, you should let them know that actually, it can!
Achieving Greater Depth In Maths Using True-False Statements
True-False statements are a very powerful way of opening up deeper learning and can be applied to any area of the maths curriculum.
The reason they are powerful is because they ‘push and pull’ by getting children to explain their understanding to others with plenty of reflecting, representing and reporting.
They are also useful for promoting greater independence and fluency as well as helping children synthesise, revisit ideas and apply ideas.
Why True-False Statements Are A Great Way To Help Children Reach Greater Depth
In the same way that concept cartoons can kill three birds with one stone, true-false statements can address reasoning, deduction and complexity all at once.
True-false statements are used to actively assess a learner’s ability to identify the accuracy of a declarative statement.
They are sometimes presented as Always-Sometimes-Never-Not Sure.
True-False statements help learners learn what they know about a concept, idea or topic in greater depth.
Don’t Be Scared To Open True-False Statements Up To The Class
When discussed collectively, True-False statements help learners to build on their own knowledge base and help build-up each other’s ideas.
As children discuss concepts in more depth they are driven to explore and find out more. If children universally reach an agreement this might be taken that they ‘understand’ the answer but this isn’t always the case.
Children might be universally wrong and share the same misconception.
Teachers therefore need to be on top of any discussions, weaving in and out of groups with devil’s advocate comments and challenges.
Any areas of disagreement can be used as the building blocks for further work and often prove to be a fantastic way of getting a classroom discussion up and running.
Make Sure The Burden Of Proof Remains On Your Pupils!
The focus of True-False statements is on evidence and proof so we need to remember that when we assess responses, we give credit for the explanations.
Merely saying whether something is true or false is pretty meaningless without the depth of an explanation.
Well chosen true-false statements are excellent training devices to develop mathematical thinking at a higher level and a deeper level. Their purpose is to challenge maths stereotypes and achieve mathematical insights based on conjecture and proof.
Some True-False statements I have planted into my lessons have yielded excellent results in terms of discussion, argument and enlightened thinking. For example:
True-False Questions I’ve Presented To My Class

Did you find some statements more challenging than others?
See if you can find out more about triangles by searching online and making up some of your own statements for others to try.
Groups could then take individual statements and analyse them in depth to feed back to the rest of the class. Any areas of disagreement can be used as the building blocks for further work.
Use True-False Statements Carefully Though, As They Have The Potential To Overwhelm
Rather than discuss a number of statements, one statement on its own may well be sufficient to promote fruitful discussion.
In fact, too many statements can be confusing and muddy the waters.
These statements all have the potential to cause trouble and that’s a good thing.
It’s good because the statements are deliberately out to provoke disagreements by way of their ambiguity and vagueness.
They are a rich source of challenge that promote thinking, disagreement and dollops of discussion when facilitated by expert teacher scaffolding.
They also raise the status of mathematical talk whereby talk is seen as work in its own right.
For more examples of True-False (Always, Sometimes, Never) statements see the download that comes with this blog post which will provide you with free greater depth maths worksheets for Year 6.
Tried And Tested Practical Recommendations When Using True-False Statements To Learn At Greater Depth
- Allow learners sufficient talk time.
- Statements should be relatively short and simple.
- Use simpler statements first and build up the difficulty levels.
- Display statements on IWB but use ones that can be manipulated physically.
- Learners can respond orally, on paper or use mini-whiteboards.
- True statements should be about the same length as false statements.
- Arrange the statements so that there is no discernible pattern of answers (such as T, F, T, F, T, F and T, T, F, F, T, T, F, F).
- Ensure there is a mixture of categorically true or false statements and some that could be either or offer a degree of ambiguity.
You can create your own True-False statements and adapt them to any part of the mathematics curriculum. For example, you might choose as your focus: triangles, fractions, symmetry, trigonometry, coordinates, negative numbers, and so on.
Ensure there is a mixture of categorically true or false statements and some that could be either or offer a degree of ambiguity, and when it comes time for pupils to create their own statements, they can use your ideas as a starting point for their own creations.
A Simple Greater Depth Activity Idea
Getting the children in your class to generate their own true-false statements is working at greater depth.
This is an extremely simple activity for you as a teacher to set up, but it is also an extremely worthwhile one too because it helps learners focus on wording individual concepts which helps to build their understanding.
For example, they can learn to make a distinction between absolute words such as; always, all, every, etc and qualifying words such as most, sometimes and usually.
The True-False format can easily be adapted into a quiz based activity too!
Exploring Misconceptions Is Another Key Part Of Learning At Greater Depth
For me, going deeper into maths is about exploring misconceptions and that’s where we should be focusing our efforts with children.
We can do this using a number of strategies and True-False statements is just one of them.
If we want to avoid misconceptions then we need to set up opportunities to teach them, make them explicit or at least provide opportunities for children to discuss them for self-discovery.
There are so many misconceptions to discuss and make a meal of that we should devote time to them so that we can explore the thinking around erroneous beliefs or incomplete knowledge.
How To Remove A Fear Of Mistakes From The Classroom
A classroom culture focused on misconceptions sounds like a recipe for confusion, but this culture sits very comfortably within a ‘we welcome all responses’ atmosphere. A fear of mistakes is what can hold children back but going deeper means making mistakes and plenty of them.
By adopting a ‘mistakes welcome’ culture we promote misconceptions as learning curves and opportunities to grow. It’s what Lemov (2015) calls a ‘Culture of Error’ and is an essential thing to ensure is in every maths classroom, mastery or otherwise.
A ‘greater depth’ classroom is a classroom where mistake-making is considered healthy and misconceptions are chances to really grow.
Children need to know that they don’t hide their fears, mistakes, errors and misconceptions, rather they plonk them on the table, they air ‘em and they share ‘em.
Misconceptions In Action – A Classroom Example
Consider the following:
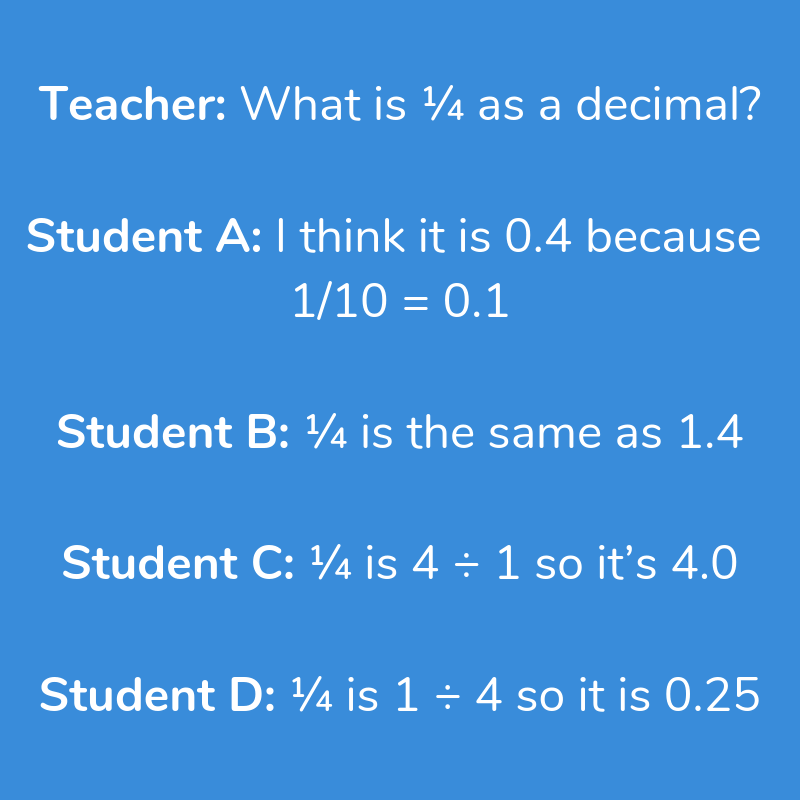
These are all plausible and realistic responses that need unpacking, and this is why it is very important that when bringing greater depth learning into the classroom, that you accept all answers to the problems you are posing and work with your class to break them down.
Bringing A Culture Of Change To Your Classroom
To bring about this culture of discovery and delving deeper then we need to use simple and effective context rich strategies that focus on alternative ways of thinking.
Craig Barton’s Diagnostic Questions and the diagnostic assessments produced by Third Space for their maths hub are very good for promoting depth of thought and ideal for getting misconceptions out into the open.
Try one of the diagnostic quizzes for free here.
Diagnostic assessments operate in a similar way to concept cartoons (minus the visual conversation) by presenting a question/problem and some alternative multiple-choice answers.
The clever part is that “their strength is their weakness” (or weaknesses).
As Craig says in his book How I Wish I’d Taught Maths:
“Diagnostic questions are designed to help identify, and crucially understand students’ mistakes and misconceptions in an efficient and accurate manner.”
(p348, How I Wish I’d Taught Maths, 2018)
If you are interested in learning more about Craig Barton’s book How I Wish I’d Taught Maths take a look at our series of blogs that Clare Sealy has adapted for a primary school audience!
- How I Wish I’d Taught (Primary) Maths: Introduction To Cognitive Load
- How I Wish I’d Taught Primary Maths: Explicit Instruction And Worked Examples
- How I Wish I’d Taught Primary Maths: Focusing Thinking and Goal Free Questions
- How I Wish I’d Taught Maths: The 5 Stages Of Deliberate Practice
- How I Wish I’d Taught Maths: Critical Thinking Skills And Problem Solving Activities In KS2
- How I Wish I’d Taught Primary Maths: How Retrieval Practice Helps Long-Term Maths Skills
Questions like the following can reveal plenty including “dodgy definitions of factors such as a number that goes into another number a whole number of times”.
Which of these is a factor of 27?
- 7
- 13.5
- 54
- 3
What If You Want To Create Your Own Diagnostic Questions?
You can also write your own diagnostic questions which as Craig admits is “flipping hard” but he gives us five golden rules to follow:
- They should be clear and unambiguous
- They should test a single skill or concept
- Students should be able to answer in less than ten seconds
- You should learn something from each incorrect response without the student needing to explain
- It is not possible to answer the question correctly whilst still holding a key misconception
So, whilst it is possible to create your own greater depth diagnostic questions, it is a tricky task!
If you’re looking for more maths resources that help develop maths problem solving techniques, all the White Rose Maths lesson slides contain a mix of fluency, reasoning and problem solving work; and on the Third Space Maths Hub you’ll find resources like Rapid Reasoning (daily word problems), and plenty of worked examples.
Why It Is Important To Teach To Greater Depth In Primary School
Using diagnostic questions or visual disagreements serve the same purpose – to enable deeper learning and to upgrade knowledge and understanding.
Surely we should be asking ourselves as teachers: “What misconceptions are going to stop children from achieving a fuller understanding,” and then once the answers are clear, purposefully planning for them as a daily activity.
Yes, teachers should co-create their own diagnostic questions and cognitive conflict disagreements but children should too – they get to appreciate far more maths when they are under the bonnet and tinkering with the bits and pieces themselves.
Just remember, start simple and build up, sideways, down and diagonally. Depth doesn’t always involve going down!
When we are looking to increase depth, in whichever direction, increasing the amount of discourse in a maths classroom will increase the learning depth so activities need to be maths talk rich.
Supportive and inclusive assessment for learning activities that can fuel maths talk help children to discuss their ideas, negotiate meanings, share strategies and make maths language their own.
Lots of children hold the idea that they have a predetermined and fixed level of ability, but by providing depth opportunities for all you’ll be able to stamp on this entity theory of learning and lets children see that learning is incremental.
Asking children to articulate their ideas, justify their thinking and share ideas forces meta-cognitive activity and this improves the clarity of their thinking and the depth of their understanding.
If we can establish and cultivate a discourse community in our classrooms then greater depth in maths is easily achievable for all!
Read more
- 5 Mastery Essentials for Greater Depth in Maths [KS2]
- Maths Mastery Toolkit: A Practical Guide To Mastery Teaching And Learning
- Why You Should Follow Mastery In Maths: 6 Real Benefits
How Third Space Learning Helps Pupils Learn At Greater Depth
With each child supported by their own 1-to-1 specialist maths tutor, this means that every Third Space pupil has an opportunity to access greater depth at their own pace. The sessions allow pupils the opportunity to reflect, 1-to-1 instruction allows for pausing, probing and pondering, without the pressure of keeping up with class mates. Our online classroom comes complete with a full range of tools and virtual manipulatives allowing children to represent their learning in various active and pictorial ways.
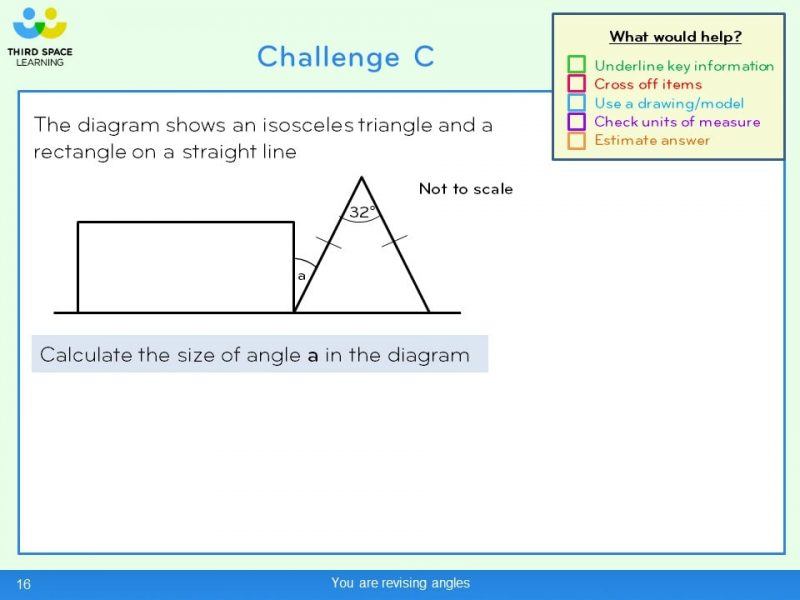
Given that we are a conversation-led intervention, children have plenty of time to engage in meaningful mathematical discussion with their tutor – allowing them the back and forth required to refine, consolidate and deepen their mathematical understanding. Furthermore, each lesson within Third Space Learning’s intervention programmes comes complete with a number of Challenge slides which encourage pupils to engage with the content covered in greater depth.
DO YOU HAVE STUDENTS WHO NEED MORE SUPPORT IN MATHS?
Every week Third Space Learning’s specialist online maths tutors support thousands of students across hundreds of schools with weekly online 1 to 1 maths lessons designed to plug gaps and boost progress.
Since 2013 these personalised one to one lessons have helped over 150,000 primary and secondary students become more confident, able mathematicians.
Learn how the programmes are aligned to maths mastery teaching or request a personalised quote for your school to speak to us about your school’s needs and how we can help.




