Adding and subtracting surds

One to one maths interventions built for KS4 success

Weekly online one to one GCSE maths revision lessons now available

Learn more

In order to access this I need to be confident with:

Squares and square roots Simplifying surds Multiplying and dividing surds

This topic is relevant for:

GCSE Maths Number Surds

Adding And Subtracting Surds

Here we will learn about adding and subtracting surds including when surd expressions can be added or subtracted, and how to carry out these calculations.

There are also adding and subtracting surds worksheets based on Edexcel, AQA and OCR exam questions, along with further guidance on where to go next if you’re still stuck.

What is adding and subtracting surds?

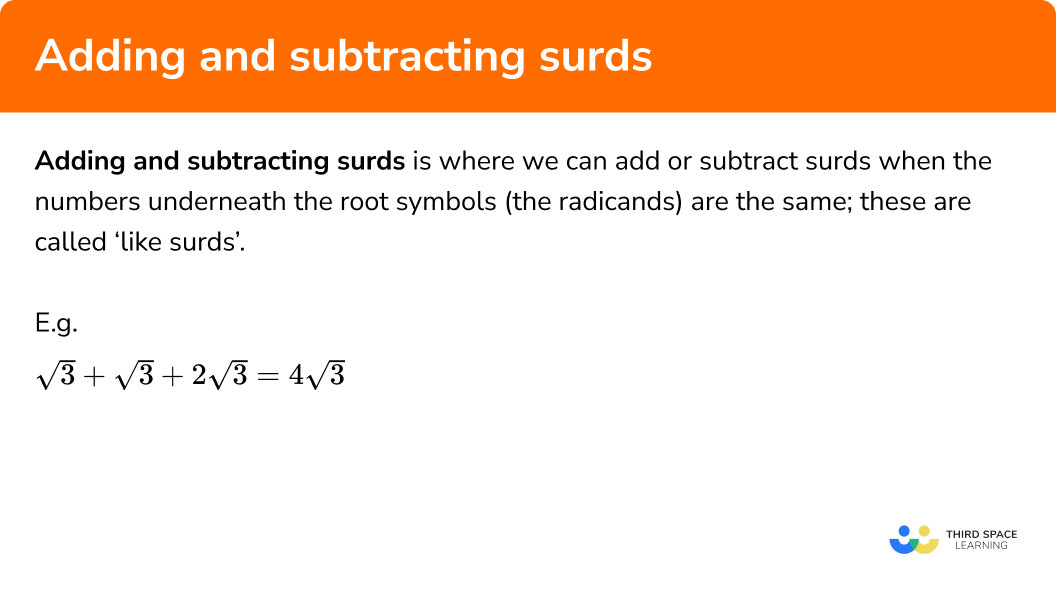
Adding and subtracting surds is where we can add or subtract surds when the numbers underneath the root symbols (the radicands) are the same; these are called ‘like surds’.

This is similar to collecting like terms in algebra:

E.g.

a + a + 2a simplifies to 4a.

So when we do a similar thing with surds:

E.g.

\[\sqrt{3}+\sqrt{3}+2\sqrt{3}=4\sqrt{3}\]

And just as 2a+3b cannot be simplified, because a and b are not like terms:

$2\sqrt{3}+3\sqrt{7}$ cannot be simplified because the numbers underneath the square root symbols (radicands) are different. $\sqrt{3}$ and $\sqrt{7}$ are not ‘like surds’.

If surds can be simplified so that they are ‘like surds’, then they can be added or subtracted.

You may be asked to apply these skills at GCSE maths to give answers to problems in geometry, such as Pythagoras or trigonometry, as exact values, rather than as decimals. Before calculators were invented, surds were the standard form for stating answers which were irrational numbers.

The formula for solving quadratics also uses a square root sign, so you may need to apply your knowledge of surds here as well.

What is adding and subtracting surds?

How to add and subtract surds

In order to add and subtract surds:

Check whether the terms are ‘like surds’.
If they aren’t like surds, simplify each surd as far as possible.
Combine the like surd terms by adding or subtracting.

How to add and subtract surds

Adding and subtracting surds worksheet

Get your free adding and subtracting surds worksheet of 20+ questions and answers. Includes reasoning and applied questions.

DOWNLOAD FREE

Adding and subtracting surds worksheet

Get your free adding and subtracting surds worksheet of 20+ questions and answers. Includes reasoning and applied questions.

DOWNLOAD FREE

Adding and subtracting surds examples

Example 1: like surds, simple addition

Simplify

\[2\sqrt{3}+5\sqrt{3}\]

Check whether the terms are ‘like surds’.

The number under the root sign is already 3 in both terms, so they have a common radicand and are like surds.

2If they aren’t like surds, simplify each surd as far as possible.

We don’t need to change the surd terms in this question.

3Combine the like surd terms by adding or subtracting.

\[2+5=7\]

so,

\[2\sqrt{3}+5\sqrt{3}=7\sqrt{3}\]

Example 2: like surds, simple subtractions

Simplify

\[7\sqrt{5}-9\sqrt{5}+6\sqrt{5}\]

Check whether the terms are ‘like surds’.

The number under the root sign is already 5 in both terms, so they have a common radicand and are like surds.

If they aren’t like surds, simplify each surd as far as possible.

We don’t need to change the surd terms in this question.

Combine the like surd terms by adding or subtracting.

\[7-9+6=4\]

so,

\[7\sqrt{5}-9\sqrt{5}+6\sqrt{5}=4\sqrt{5}\]

Example 3: unlike surds

Simplify

\[10 \sqrt{2}+4 \sqrt{11}\]

Check whether the terms are ‘like surds’.

The numbers under the root signs are 2 and 11; these are not like surds.

If they aren’t like surds, simplify each surd as far as possible.

These surds cannot be simplified further. There are no square factors of either 2 or 11.

Combine the like surd terms by adding or subtracting.

In this case, the surds are not like, so they cannot be combined.

We state the answer as:

\[10 \sqrt{2}+4 \sqrt{11}\]

Example 4: sums containing non-surds

Simplify

\[4+6\sqrt{2}-3\sqrt{2}-\sqrt{25}\]

Check whether the terms are ‘like surds’.

All the surd terms are like surds – they are all root 2. The square root of 25 is not a surd, because 25 is a square number.

If they aren’t like surds, simplify each surd as far as possible.

We don’t need to simplify the surds.

However, the square root of 25 is 5 (this is not a surd), so we simplify this.

\[4+6\sqrt{2}-3\sqrt{2}-5\]

Combine the like surd terms by adding or subtracting.

Combining the surds:

\[6\sqrt{2}-3\sqrt{2}=3\sqrt{2}\]

We also combine the other like terms – in this case, the integers:

\[ 4-5=-1\]

So the final answer is:

\[3\sqrt{2}-1\]

Note that it’s always best to start an answer with a positive term, but:

\[-1+3\sqrt{2}\]

is also correct.

Example 5: one surd needs to be simplified

Simplify

\[\sqrt{7}+\sqrt{28}\]

Check whether the terms are ‘like surds’.

The numbers under the root signs are 7 and 28; these are not like surds.

If they aren’t like surds, simplify each surd as far as possible.

\[\sqrt{7}\]

is already fully simplified; there are no square factors of 7.

\[\sqrt{28}\]

can be simplified, because 4 is a square factor of 28.

\[\begin{aligned} \sqrt{28} &=\sqrt{4 \times 7} \\ &=\sqrt{4} \times \sqrt{7} \\ &=2 \times \sqrt{7} \\ &=2 \sqrt{7} \end{aligned}\]

Combine the like surd terms by adding or subtracting.

\[\begin{aligned} \sqrt{7}+\sqrt{28} &=\sqrt{7}+2 \sqrt{7} \\ &=3 \sqrt{7} \end{aligned}\]

Example 6: both surds need to be simplified

Simplify

\[\sqrt{8}+\sqrt{72}\]

Check whether the terms are ‘like surds’.

The numbers under the root signs are 8 and 72; these are not like surds.

If they aren’t like surds, simplify each surd as far as possible.

\[\sqrt{8}\]

can be simplified, because 4 is a square factor of 8.

\[\begin{aligned} \sqrt{8} &=\sqrt{4 \times 2} \\ &=\sqrt{4} \times \sqrt{2} \\ &=2 \times \sqrt{2} \\ &=2 \sqrt{2} \end{aligned}\]

\[\sqrt{72}\]

can be simplified, because 36 is a square factor of 72.

\[\begin{aligned} \sqrt{72} &=\sqrt{36 \times 2} \\ &=\sqrt{36} \times \sqrt{2} \\ &=6 \times \sqrt{2} \\ &=6 \sqrt{2} \end{aligned}\]

Note that you could have simplified in stages, using the square factors 4, then 9. However, you should always make sure the surd is simplified fully – if you had left your simplification as:

\[\begin{aligned} \sqrt{72} &=\sqrt{4} \times \sqrt{18} \\ &=2 \sqrt{18} \end{aligned}\]

Then the surds would not be ‘like’ and you would not be able to combine by addition or subtraction.

Combine the like surd terms by adding or subtracting.

\[\begin{aligned} \sqrt{8}+\sqrt{72} &=2\sqrt{2}+6 \sqrt{2} \\ &=8 \sqrt{2} \end{aligned}\]

Example 7: two surds need to be simplified

Simplify

\[\sqrt{75}+\sqrt{50}\]

Check whether the terms are ‘like surds’.

The numbers under the root signs are 75 and 50; these are not like surds.

If they aren’t like surds, simplify each surd as far as possible.

\[\sqrt{75}\]

can be simplified, because 25 is a square factor of 75.

\[\begin{aligned} \sqrt{75} &=\sqrt{25 \times 3} \\ &=\sqrt{25} \times \sqrt{3} \\ &=5 \times \sqrt{3} \\ &=5 \sqrt{3} \end{aligned}\]

\[\sqrt{50}\]

can be simplified, because 25 is a square factor of 50.

\[\begin{aligned} \sqrt{50} &=\sqrt{25 \times 2} \\ &=\sqrt{25} \times \sqrt{2} \\ &=5 \times \sqrt{2} \\ &=5 \sqrt{2} \end{aligned}\]

Combine the like surd terms by adding or subtracting.

Even when simplified fully, the surds are not like, so they cannot be combined.

We state the answer as:

\[5\sqrt{3}+5\sqrt{2}\]

Example 8: a sum containing non-surds

Simplify

\[\sqrt{90}+\sqrt{64}-\sqrt{40}\]

Check whether the terms are ‘like surds’.

The numbers under the root signs are 90, 64 and 40; these are not like surds.
64 is a square number, so this isn’t actually a surd.

If they aren’t like surds, simplify each surd as far as possible.

\[\sqrt{90}\]

can be simplified, because 9 is a square factor of 90.

\[\begin{aligned} \sqrt{90} &=\sqrt{9 \times 10} \\ &=\sqrt{9} \times \sqrt{10} \\ &=3 \times \sqrt{10} \\ &=3 \sqrt{10} \end{aligned}\]

\[\sqrt{64}=8\]

\[\begin{aligned} \sqrt{40} &=\sqrt{4 \times 10} \\ &=\sqrt{4} \times \sqrt{10} \\ &=2 \times \sqrt{10} \\ &=2 \sqrt{10} \end{aligned}\]

Combine the like surd terms by adding or subtracting.

\[\begin{aligned} \sqrt{90}+\sqrt{64}-\sqrt{40} &=3 \sqrt{10}+8-2 \sqrt{10} \\ &=8+\sqrt{10} \end{aligned}\]

Common misconceptions

Remember that a root with no integer coefficient is ‘1 lot’ of that surd

As in algebra, we understand that a actually means 1a.

Don’t mix up addition and multiplication laws

\[\sqrt{3}+\sqrt{3}=2 \sqrt{3}\]

\[\sqrt{3} \times \sqrt{3}=\sqrt{9}=3\]

Refer back to knowledge of algebra to help:

a + a = 2a and a × a = a².

Not simplifying each surd fully

As in example 6 above, if we don’t simplify each surd fully, it may look like the surds cannot be simplified to give ‘like surds’.

Trying to combine unlike surds

It’s OK to leave an answer with more than one surd in it if it will not simplify further.

Adding and subtracting surds is part of our series of lessons to support revision on surds. You may find it helpful to start with the main surds lesson for a summary of what to expect, or use the step by step guides below for further detail on individual topics. Other lessons in this series include:

Practice adding and subtracting surds questions

6 \sqrt{11}

4 \sqrt{11}

7 \sqrt{11}

5 \sqrt{11}

Already in like surd form; $3+2-1=4$ .

3 \sqrt{5}+2 \sqrt{3}

5 \sqrt{15}

-24 \sqrt{15}

3 \sqrt{5}-2 \sqrt{3}

We can only collect the ‘like’ root $3$ s; $4-2=2$ .

5\sqrt{11}

\sqrt{55}

3\sqrt{11}

11 \sqrt{5}

$4$ is a square factor of $44$ , so use this to simplify root $44$ . There’s then a like surd of root $11$ .

\sqrt{30}

6\sqrt{30}

6\sqrt{6}

\sqrt{6}

$9$ is a square factor of $54$ and $4$ is a square factor of $24$ . When you simplify both roots, there’s a like surd of root $6$ .

3\sqrt{1000}

6-\sqrt{10} \sqrt{5}

6\sqrt{5}-5\sqrt{2}

6\sqrt{10} \sqrt{5}

When both surds are fully simplified, they are not like surds. We just write the answer as the subtraction with simplified surds.

12+5 \sqrt{10}-2 \sqrt{2}

12+3 \sqrt{2}

\sqrt{2}(3+3 \sqrt{8})

12-3 \sqrt{2}

Root 16 is not a surd, so we work out $3\times4=12$ . Then, looking at the surds, when both are simplified, there is a like surd of root $2$ . We combine these two surds.

Adding and subtracting surds GCSE questions

1. Express $\sqrt{6}+\sqrt{54}$ in the form $a \sqrt{6}$ where $a$ is an integer.

(2 marks)

Show answer

$\sqrt{54}=\sqrt{9} \times \sqrt{6}$

(1)

$\sqrt{6} + \sqrt{54} =4\sqrt{6} (a=4)$

(1)

2. Simplify fully $\sqrt{32}+\sqrt{2}$

(2 marks)

Show answer

$\sqrt{32}=\sqrt{16} \times \sqrt{2}$

(1)

$5 \sqrt{2}$

(1)

3. Write $\sqrt{40}+\sqrt{160}$ in the form $a \sqrt{10}$

(3 marks)

Show answer

$\sqrt{40}=\sqrt{4} \times \sqrt{10}$

(1)

$\sqrt{160}=\sqrt{16} \times \sqrt{10}$

(1)

$\sqrt{40} + \sqrt{160} =6\sqrt{10} (a=6)$

(1)

Learning checklist

You have now learned how to:

Add and subtract surds

The next lessons are

Still stuck?

Prepare your KS4 students for maths GCSEs success with Third Space Learning. Weekly online one to one GCSE maths revision lessons delivered by expert maths tutors.