FREE DOWNLOAD
Inverse Proportion Worksheet
Help your students prepare for their Maths GCSE with this free inverse proportion worksheet of 38 questions and answers
- Section 1 of the inverse proportion worksheet contains 30 skills-based inverse proportion questions, in 3 groups to support differentiation
- Section 2 contains 3 applied inverse proportion questions with a mix of worded problems and deeper problem solving questions
- Section 3 contains 4 foundation and higher level GCSE exam style inverse proportion questions
- Answers and a mark scheme for all inverse proportion questions are provided
- Questions follow variation theory with plenty of opportunities for students to work independently at their own level
- All questions created by fully qualified expert secondary maths teachers
- Suitable for GCSE maths revision for AQA, OCR and Edexcel exam boards
Unlock access to download your free resource
You can unsubscribe at any time (each email we send will contain an easy way to unsubscribe). To find out more about how we use your data, see our privacy policy.
Raise maths attainment across your school with hundreds of flexible and easy to use GCSE maths worksheets and lessons designed by teachers for teachers.
Inverse proportion at a glance
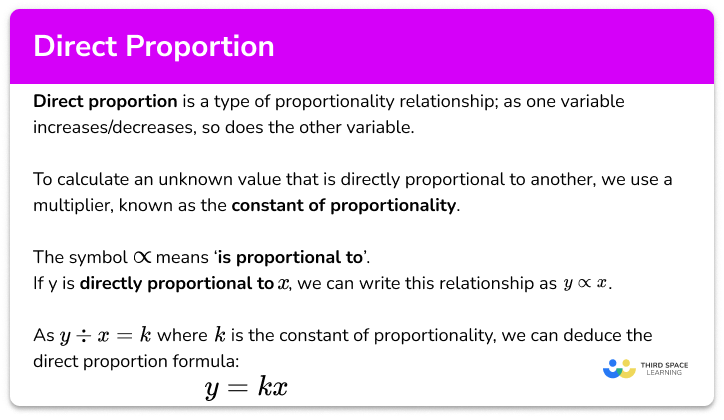
When two amounts are inversely proportional to each other, one amount increases as the other decreases. Another way of looking at this is if x is inversely proportional to y then x is proportional to 1 divided by y.
We see inversely proportional relationships in everyday life. For example, the amount of time it takes to travel somewhere is inversely proportional to the speed at which we are travelling or the amount of time taken to complete a job is inversely proportional to the number of workers.
For more complicated relationships involving inverse proportion, we can find the constant of proportionality, k, and use this to write a formula connecting the two amounts. With y being inversely proportional to x, the value of k is equal to the product of x and y.
Inverse proportion is also known as indirect proportion, or inverse variation.
Looking forward, students can then progress to additional ratio and proportion worksheets, for example a ratio worksheet or a simplifying and equivalent ratios worksheet.
For more teaching and learning support on Ratio and Proportion our GCSE maths lessons provide step by step support for all GCSE maths concepts.
Do you have students who need additional support?
With Third Space Learning's secondary maths tutoring programmes, students in Year 7-11 receive regular one to one maths tutoring to address gaps, build confidence and boost progress.
"My confidence in the tutoring is high. We've had some phenomenal results. I even had one girl get a Grade 8 this year; she came to every tutoring session."
Stacey Atkins, Maths Director, Outwood Grange Academies Trust