GCSE Tutoring Programme

Our chosen students improved 1.19 of a grade on average - 0.45 more than those who didn't have the tutoring.

Teacher-trusted tutoring

In order to access this I need to be confident with:

Quartile Percentage of an amount Fractions of amounts

This topic is relevant for:

GCSE Maths Statistics Cumulative Frequency

Interquartile Range

Interquartile range

Here we will learn about interquartile range, including finding the interquartile range from the quartiles for a set of data, comparing data sets using the median and the interquartile range and analysing data using quartiles and the interquartile range.

There are also interquartile range worksheets based on Edexcel, AQA and OCR exam questions, along with further guidance on where to go next if you’re still stuck.

What is interquartile range?

Interquartile range is the difference between the upper quartile (or third quartile) and the lower quartile (or first quartile) in an ordered data set.

Interquartile range $\bf{=}$ upper quartile $\bf{-}$ lower quartile

IQR=UQ-LQ\text{ or }IQR=Q_{3}-Q_{1}

The data must be in order (smallest to largest).

Remember,

The first quartile, $Q_{1}$ is $\frac{1}{4}$ of the way through the data – the lower quartile.
The second quartile, $Q_{2}$ is $\frac{1}{2}$ of the way through the data – the median value.
The third quartile, $Q_{3}$ is $\frac{3}{4}$ of the way through the data – the upper quartile.

We can also consider each quartile as a percentile where,

The lower quartile $LQ$ is the $\bf{25}$ th percentile as $25\%$ of the data lies below this value.
The median $M$ is the $\bf{50}$ th percentile as $50\%$ of the data lies below (or above) this value.
The upper quartile $UQ$ is the $\bf{75}$ th percentile as $75\%$ of the data lies below this value.

Note: the lower quartile is the median of the lower half of the data, the upper quartile is the median of the upper half of the data.

Step-by-step guide: Quartile

For example,

Find the interquartile range of the following data.

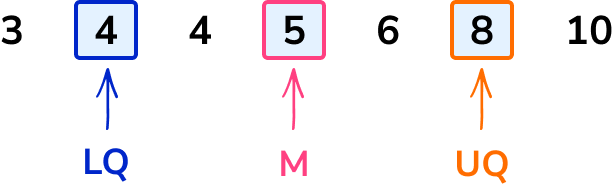
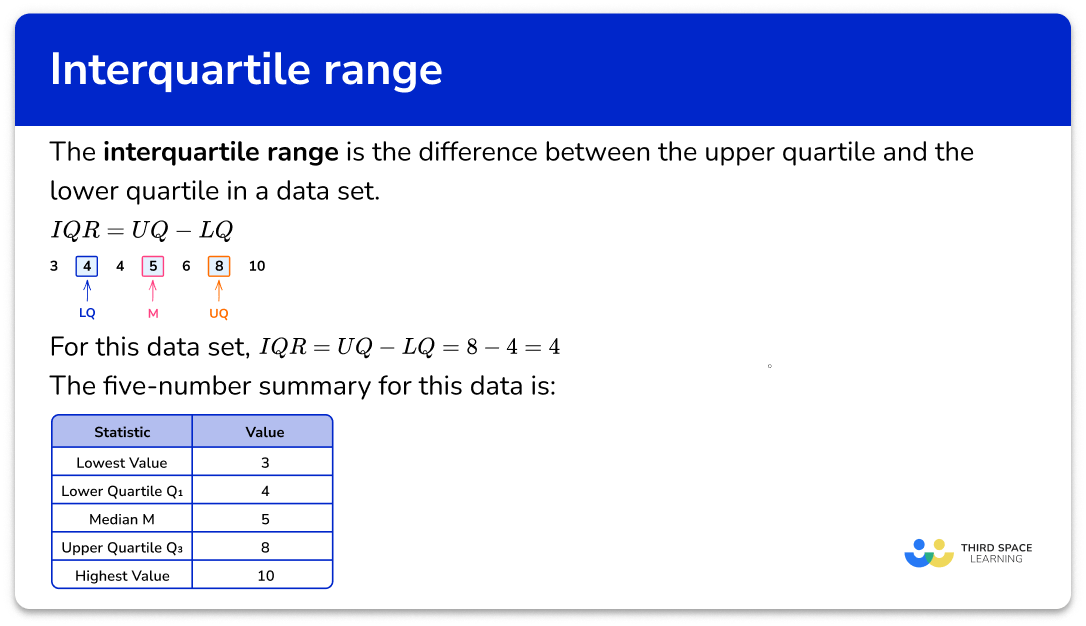
Here, $IQR=UQ-LQ=8-4=4$

The interquartile range $(IQR)$ is a descriptive statistic, and measures the variability or spread of the data.

The larger the interquartile range, the wider the spread of the central $50\%$ of data.

The smaller the value for the interquartile range, the narrower the central $50\%$ of data for the data set.

The $IQR$ is far more representative of the spread of this data set because it is not affected by extreme values.

The median and lower and upper quartiles, along with the minimum value and the maximum value of the data set, form a five-number summary of descriptive statistics for the data set. This information can then be presented in a box plot (box and whisker diagram) making it easy to compare with other sets of data.

The interquartile range is not part of this five-number summary, but is useful alongside it as a measure of dispersion or spread.

What is interquartile range?

How to find the interquartile range

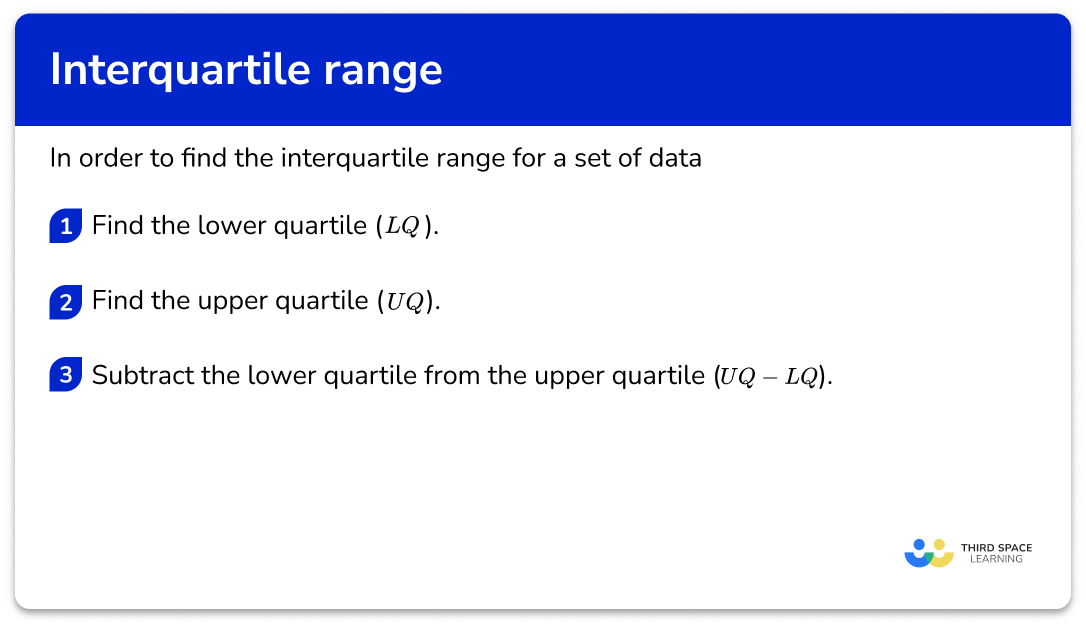
In order to find the interquartile range for a set of data:

Find the lower quartile ( $LQ$ ).
Find the upper quartile ( $UQ$ ).
Subtract the lower quartile from the upper quartile ( $UQ-LQ$ ).

Explain how to find the interquartile range

Interquartile range worksheet

Get your free interquartile range worksheet of 20+ questions and answers. Includes reasoning and applied questions.

DOWNLOAD FREE

Interquartile range worksheet

Get your free interquartile range worksheet of 20+ questions and answers. Includes reasoning and applied questions.

DOWNLOAD FREE

Related lessons on cumulative frequency

Interquartile range is part of our series of lessons to support revision on cumulative frequency. You may find it helpful to start with the main cumulative frequency lesson for a summary of what to expect, or use the step by step guides below for further detail on individual topics. Other lessons in this series include:

Interquartile range examples

Example 1: odd number of ordered data values

Find the interquartile range for the following set of data.

1, 1, 2, 3, 5, 7, 7, 8, 10, 12, 15

Step-by-step guide: Quartile (Example 1)

Find the lower quartile ( $LQ$ ).

The median is the middle value, $7$ , and so the lower quartile is the middle number in the lower half of the data, excluding the median.

LQ = 2

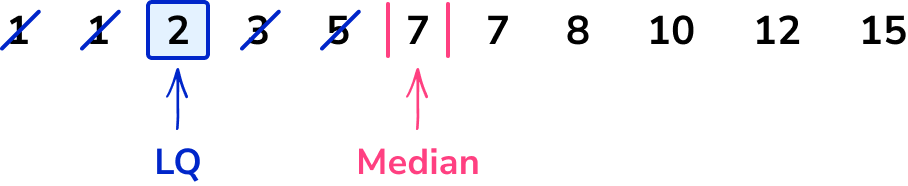
2Find the upper quartile ( $UQ$ ).

The upper quartile is the middle value in the upper half of the data, excluding the median value $(7)$ .

UQ = 10

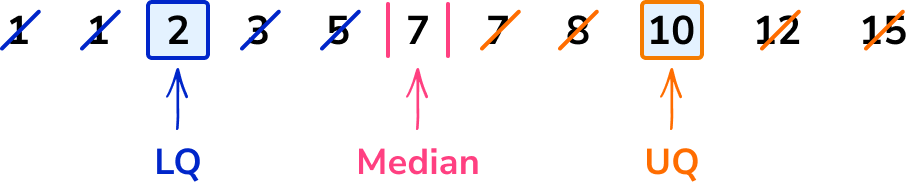
3Subtract the lower quartile from the upper quartile ( $UQ-LQ$ ).

As $LQ=2$ and $UQ=10$

IQR=UQ-LQ=10-2=8

Example 2: small continuous data set (even amount of data values)

The data below shows birth weights of $10$ babies in kilograms.

\begin{aligned} &3.3 \quad 3.7 \quad 2.5 \quad 3.5 \quad 3.0 \\ &4.3 \quad 3.1 \quad 4.1 \quad 1.9 \quad 3.6 \end{aligned}

Calculate the interquartile range for the data set.

Step-by-step guide: Quartile (Example 2)

Find the lower quartile ( $LQ$ ).

The median for an even number of data values in a data set is the average of these two middle values. Here, the median is $3.4$ . The lower quartile is the middle of the lower half of the data.

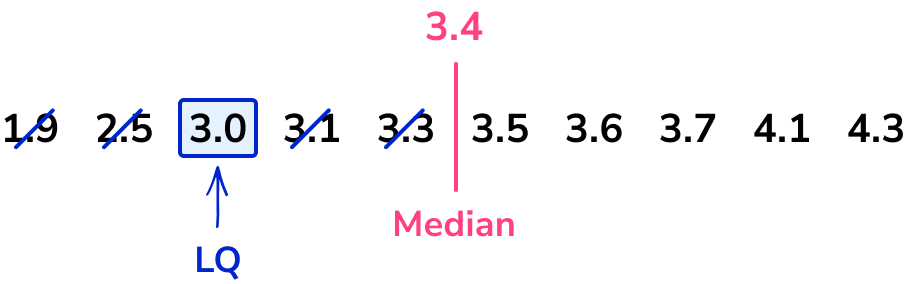
$LQ = 3.0$

Find the upper quartile ( $UQ$ ).

The upper quartile is the middle value of the upper half of the data set.

$UQ = 3.7$

Subtract the lower quartile from the upper quartile ( $UQ-LQ$ ).

As $LQ=3.0$ and $UQ=3.7,$

$IQR=UQ-LQ=3.7-3.0=0.7kg$

Example 3: odd number of unordered data values

Find the interquartile range.

13, 8, 20, 16, 3, 5, 40, 14, 34

Step-by-step guide: Quartile (Example 3)

Find the lower quartile ( $LQ$ ).

Using the ordered data set, we have

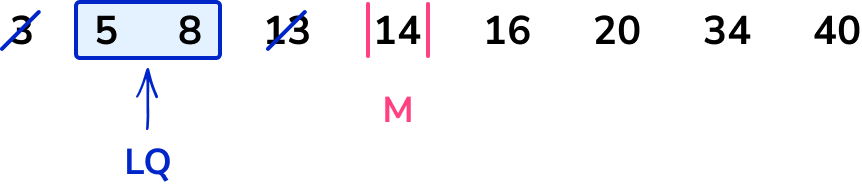
The midpoint of the two data values $5$ and $8$ is $6.5.$

$LQ = 6.5$

Find the upper quartile ( $UQ$ ).

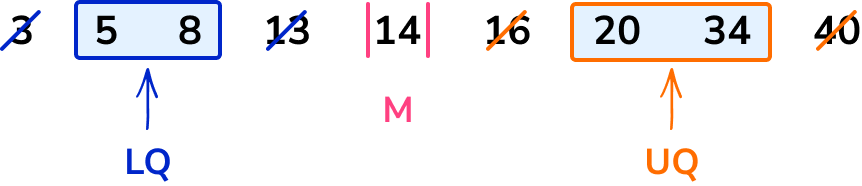
The midpoint of the two data values $20$ and $34$ is $27.$

$UQ = 27$

Subtract the lower quartile from the upper quartile ( $UQ-LQ$ ).

As $LQ=6.5$ and $UQ=27,$

$IQR=UQ-LQ=27-6.5=20.5$

Example 4: IQR from a stem and leaf diagram

Find the interquartile range from the data in the stem and leaf diagram.

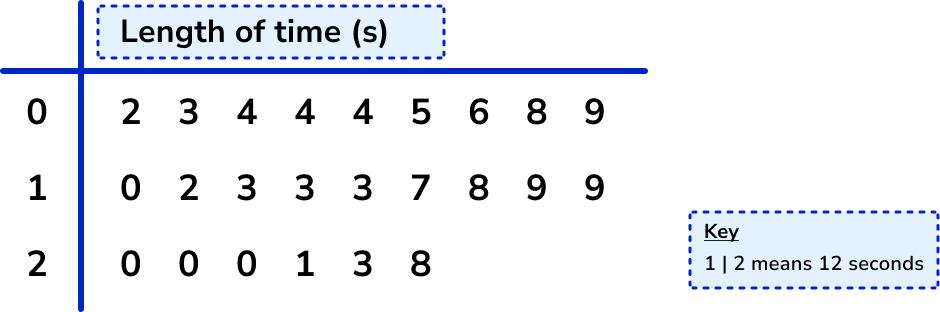
Step-by-step guide: Quartile (Example 4)

Find the lower quartile ( $LQ$ ).

$n$ is the number of values in the data set; here $n=24.$

As we have an even number of items in the data set, we use the formula $\frac{n+1}{4}$ with $n=24$ to give

$\frac{24+1}{4}=\frac{25}{4}=6.25$

So the lower quartile lies between the $6th$ and $7th$ data point. It is sufficient at GCSE just to use the midpoint of these two values.

Using the stem and leaf diagram, we have

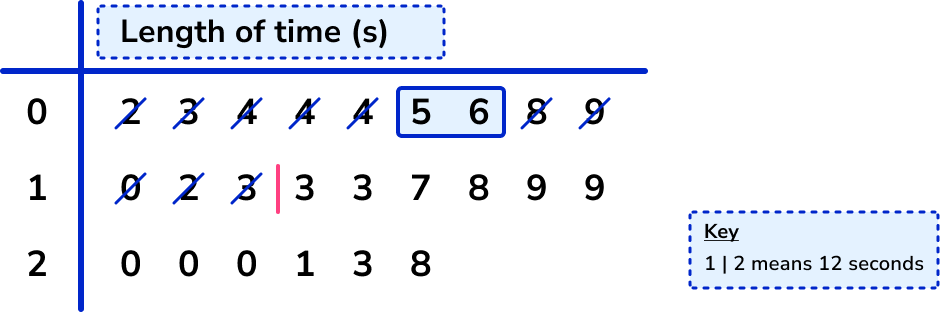
After using the key, the $6th$ and $7th$ of values are $5$ and $6.$ The midpoint of these is $5.5,$ so the lower quartile is $5.5.$

Find the upper quartile ( $UQ$ ).

We use the formula $3\times\frac{n+1}{4}$ with $n=24$ to give

$3 \times \frac{24+1}{4}=3 \times \frac{25}{4}=3 \times 6.25=18.75$

Note that if you’ve already found that the lower quartile is the $6.25th$ value, you can simply multiply $6.25$ by $3$ to get $18.75.$

So the upper quartile lies between the $18th$ and $19th$ data point, and we use the midpoint of these values.

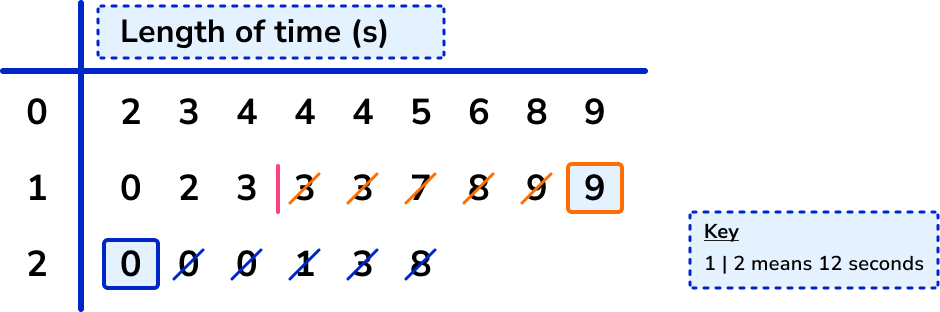
Using the key, the $18th$ and $19th$ values are $19$ and $20$ respectively. The midpoint of these is $19.5,$ so the upper quartile is $19.5.$

Subtract the lower quartile from the upper quartile ( $UQ-LQ$ ).

As $LQ=5.5$ and $UQ=19.5,$

$IQR=UQ-LQ=19.5-5.5=14$

Example 5: IQR from a five-number summary

Below is a table showing a summary of statistical values for a reaction time experiment.

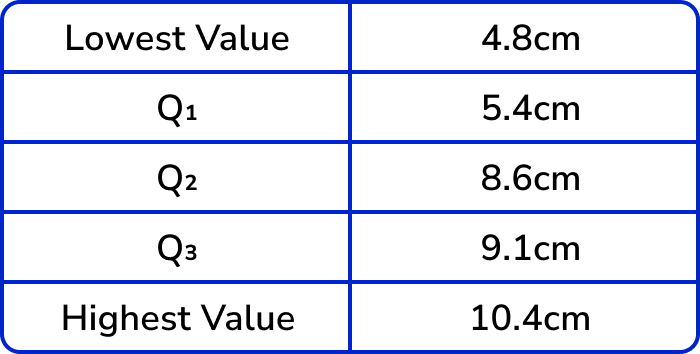
Determine the interquartile range of reaction times.

Find the lower quartile ( $LQ$ ).

The lower quartile is the value $Q_1.$ Here $LQ=5.4cm.$

Find the upper quartile ( $UQ$ ).

The upper quartile is the value $Q_3.$ Here $UQ=9.1cm.$

Subtract the lower quartile from the upper quartile ( $UQ-LQ$ ).

IQR=UQ-LQ=9.1-5.4=3.7cm

Example 6: IQR from a cumulative frequency curve

The cumulative frequency curve below shows the total marks achieved by a sample of students in an exam. Calculate the interquartile range of the data set.

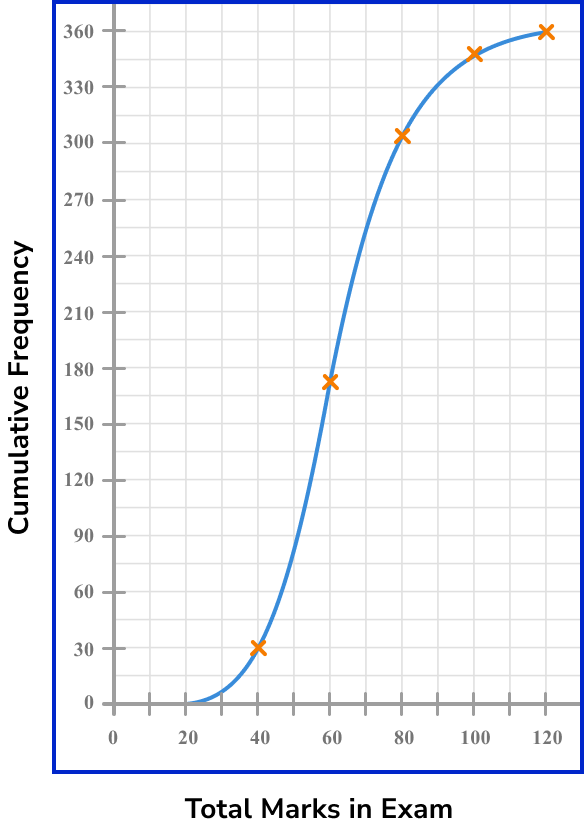
Find the lower quartile ( $LQ$ ).

The sample size is $360$ students. The lower quartile is the value that represents the lowest $25\%$ of the data. To find this, we need to calculate $25\%$ of the total number of students.

$360\times{0.25}=90.$

We then draw a horizontal line from $90$ on the vertical axis to the cumulative frequency curve, and then from the curve to the horizontal axis, and read off the value.

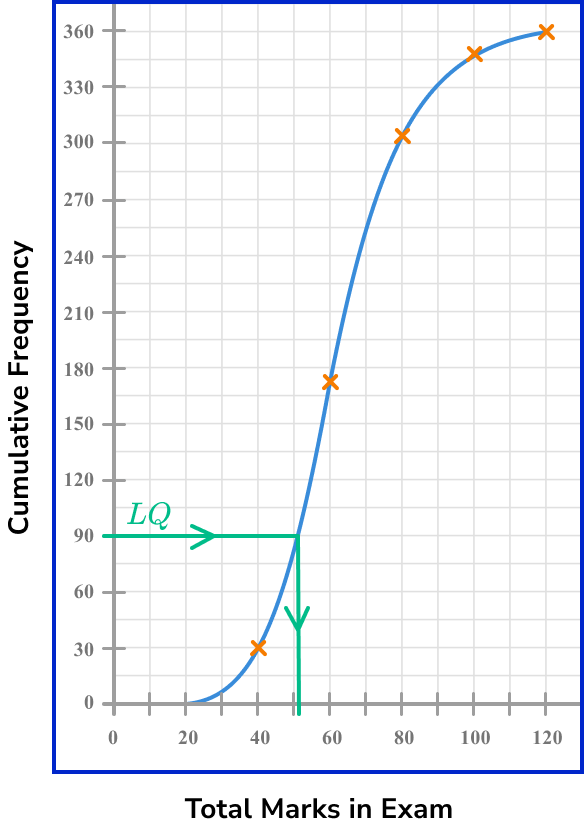
Here, the lower quartile is $51.$

Find the upper quartile ( $UQ$ ).

The upper quartile represents the value where $75\%$ of the data lies below. Calculating $75\%$ of $360,$ we have

$360\times{0.75}=270.$

Using the same approach for the upper quartile as finding the lower quartile, we draw a horizontal line across from $270$ on the vertical axis to the curve, and then down to the horizontal axis, and read off the value.

The upper quartile is therefore $72.$

Subtract the lower quartile from the upper quartile ( $UQ-LQ$ ).

As $UQ=72$ and $LQ=51,$

$IQR=UQ-LQ=72-51=21$

Step-by-step guide: Cumulative frequency (coming soon)

Example 7: IQR from a box plot

The box plot below shows the distribution of the age of trees in a woodland.

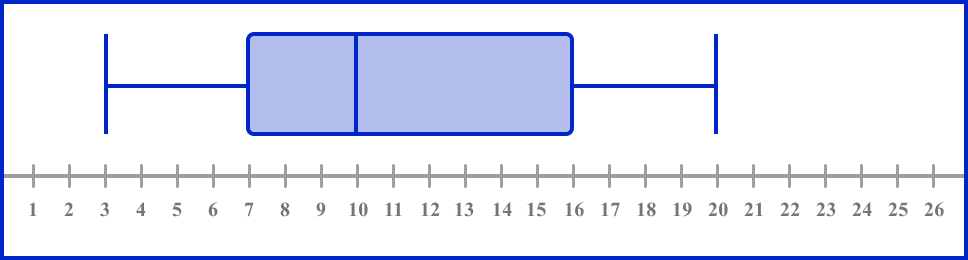
Calculate the interquartile range of ages of trees.

Find the lower quartile ( $LQ$ ).

The lower quartile of a box plot is the left hand edge of the box within the box plot. This line is positioned above the value $7$ on the scale and so

$LQ=7$

Find the upper quartile ( $UQ$ ).

The upper quartile of a box plot is the right hand edge of the box within the box plot. This line is positioned above the value $16$ on the scale and so

$UQ=16$

Subtract the lower quartile from the upper quartile ( $UQ-LQ$ ).

As $UQ=16$ and $LQ=7,$

$IQR=UQ-LQ=16-7=9$

Step-by-step guide: Box plots (coming soon)

Common misconceptions

Forgetting to order the data set before finding the median or quartiles

The list/data set must be in order from smallest to largest before you start finding the key values.

Stating the quartile value as the number given by the formula, rather than counting and finding that value in the data

For example, for the data set $3, 4, 6, 7, 10,$ the formula for the lower quartile gives $\frac{5-1}{4}=\frac{6}{4}=1.5.$ This tells you which data values to select (in this case, the midpoint of the $1$ st and $2$ nd values); do not just write $LQ=1.5.$

The range is calculated instead of the interquartile range

The range is the smallest value subtracted from the largest value whereas the interquartile range is the lower quartile, subtracted from the upper quartile.

Practice interquartile range questions

2.5

8

3

21

\begin{aligned} &LQ=20, \quad UQ=23 \\\\ &IQR=UQ-LQ=23-20=3 \end{aligned}

9.5

11

5.5

13.5

\begin{aligned} &LQ=4, \quad UQ=13.5 \\\\ &IQR=UQ-LQ=13.5-4=9.5 \end{aligned}

15

7.5

9

39

Ordering the data first, we get:

6, 12, 14, 15, 19, 21, 23, 33, 45

\begin{aligned} &LQ=13, \quad UQ=28 \\\\ &IQR=UQ-LQ=28-13=15 \end{aligned}

6

5

5.5

4

\begin{aligned} &LQ=6, \quad UQ=10 \\\\ &IQR=UQ-LQ=10-6=4 \end{aligned}

15

33

19

36

$LQ=15, \ UQ=34$ and then $34-15=19$

x=33

x=2

x=55

x=31

$LQ=12$ and $IQR=43.$

To calculate the $UQ,$ we add the interquartile range to the value for the lower quartile:

x=LQ+IQR=12+43=55.

2cm

3.5cm

1.85cm

2.05cm

There are $25$ apples in the data set.

The median for an odd number of data values is found in the position $\frac{n+1}{2}.$ As $n=25,$ the median is in the $13th$ position and so the value for the median is $29.2cm.$

The lower quartile is the middle value of the lower half of the data, excluding the median value $(29.2cm).$ Here, the lower quartile is between the two values $28.0cm$ and $28.0cm$ and so the lower quartile is $28.0cm.$

interquartile range practice question 7 explanation image 1

The upper quartile is the middle of the upper half of the data, excluding the median. Here the upper quartile is halfway between $30.0cm$ and $30.1cm$ which is equal to $30.05cm.$

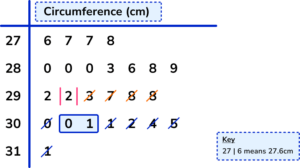
interquartile range practice question 7 explanation image 2

As $IQR=UQ-LQ,$

IQR=30.05-28.0=2.05cm.

Interquartile range GCSE questions

1. The following data shows the maths test results of $15$ students.

12,17,18,19,19,20,23,24,24,25,26,27,28,30,35

Work out the interquartile range.

(2 marks)

Show answer

Either $LQ=19 \;$ or $\; UQ=27$

(1)

IQR=8

(1)

2. (a) This data set shows the yearly salary paid to each worker at a small company.

£23,000, \;\; £25,000, \;\; £25,500, \;\; £27,000, \;\; £32,000, \;\; £60,000

Why should the interquartile range be used to describe the spread of this data?

(b) Calculate the interquartile range.

(c) Each member of staff receives a $1\%$ pay increase. Without any calculation, how will this affect the interquartile range? Tick the correct answer and state your reason why.

Interquartile Range Exam Question 2c

(5 marks)

Show answer

(a)

IQR should be used because the data set contains an extreme value/outlier $(£60,000)$ .

(1)

(b)

Either $LQ=£25,000 \;$ or $\; UQ=£32,000$

(1)

IQR=7

(1)

(c)

The IQR will get larger (with appropriate reason).

(1)

The upper quartile will increase by a larger value than the lower quartile as $1\%$ of a larger quantity is a greater value than $1\%$ of a smaller quantity.

(1)

3. A shopkeeper records the number of people in her shop at half-hour intervals throughout the day:

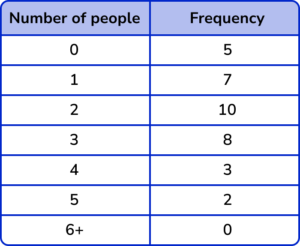
interquartile range GCSE question 3

(a) Find the median number of shoppers.

(b) Calculate the interquartile range.

(6 marks)

Show answer

(a)

Median $18th$ data point so median $=2$ .

(1)

(b)

Lower quartile $9th$ data point so $LQ=1$ .

(1)

Upper quartile $27th$ data point so $UQ=3$ .

(1)

IQR=3-1=2

(1)

(c)

(0 \times 5)+(1 \times 7)+(2 \times 10)+(3 \times 8)+(4 \times 3)+(5 \times 2)+(6 \times 0)

(1)

=73

(1)

Learning checklist

You have now learned how to:

Calculate the interquartile range

(IQR)

for sets of data

The next lessons are

Still stuck?

Prepare your KS4 students for maths GCSEs success with Third Space Learning. Weekly online one to one GCSE maths revision lessons delivered by expert maths tutors.

Find out more about our GCSE maths tuition programme.

Introduction

What is interquartile range?

How to find the interquartile range

Interquartile range worksheets

Interquartile range examples

↓

Example 1: odd number of ordered data values Example 2: small continuous data set (even amount of data values) Example 3: odd number of unordered data values Example 4: IQR from a stem and leaf diagram Example 5: IQR from a five-number summary Example 6: IQR from a cumulative frequency curve Example 7: IQR from a box plot

Common misconceptions

Practice interquartile range questions

Interquartile range GCSE questions

Learning checklist

Next lessons

Still stuck?