FREE DOWNLOAD
Unitary Method Worksheet
Help your students prepare for their Maths GCSE with this free unitary method worksheet of 33 questions and answers
- Section 1 of the unitary method worksheet contains 24 skills-based unitary method questions, in 3 groups to support differentiation
- Section 2 contains 5 applied unitary method questions with a mix of word problems and deeper problem solving questions
- Section 3 contains 4 foundation and higher level GCSE exam style unitary method questions
- An answer key and a mark scheme for all unitary method questions are provided
- Questions follow variation theory with plenty of opportunities for students to work independently at their own level
- All questions created by fully qualified expert secondary maths teachers
- Suitable for GCSE maths revision for AQA, OCR and Edexcel exam boards
Unitary method at a glance
The unitary method is a method that involves finding the value of a single unit and using that to find the value of a different number of units of something. For example, if a factory produces 3000 tubes of toothpaste in 4 days, we can find the number of tubes of toothpaste produced in 5 days using the unitary method. First we calculate the number of tubes of toothpaste in 1 day, 3000 divided by 4 = 750. We can then multiply by 5 to find the number produced in 5 days: 750 times 5 = 3750.
The above example has two variants with direct variation, commonly referred to as direct proportional. The unitary method can also be used for problems involving indirect variation (inverse proportion). For example, if a project is 6 days work for 3 people, we can work out how many days work it would be for 2 people. First, we find the number of days for 1 person, 6 times 3 = 18. We can then divide by 2 to find the number of days for 2 people: 18 divided by 2 = 9.
Looking forward, students can then progress to additional ratio and proportion worksheets, for example a ratio worksheet or a simplifying and equivalent ratios worksheet.

For more teaching and learning support on Ratio and Proportion our GCSE maths lessons provide step by step support for all GCSE maths concepts.
Do you have students who need additional support?
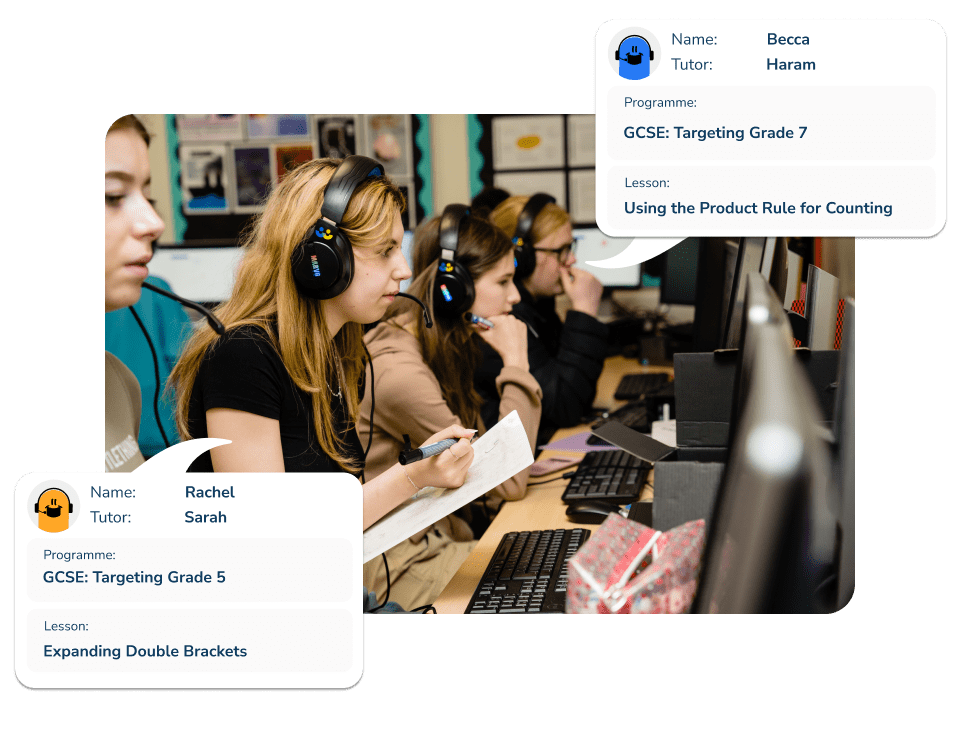
With Third Space Learning's secondary maths tutoring programmes, students in Year 7-11 receive regular one to one maths tutoring to address gaps, build confidence and boost progress.
"My confidence in the tutoring is high. We've had some phenomenal results. I even had one girl get a Grade 8 this year; she came to every tutoring session."
Stacey Atkins, Maths Director, Outwood Grange Academies Trust