GCSE Maths Paper 2 2022: Summary Of Topics, Questions & Planning For Paper 3

Originally published Wednesday 8th June 2022

GCSE maths Paper 2 2022 is done and we are now two papers down in this year’s GCSE mathematics exams – a year that feels quite a bit different due to exam adaptations such as the release of advance information.

Our expert consultant and contributor, Christine Norledge, breaks down the paper and discusses what may be coming on Paper 3.

GCSE Maths 2024: Stay up to date

Join our email list to stay up to date with the latest news, revision lists and resources for GCSE maths 2024. We’ll be bringing in our expert contributors to analyse each paper and identify key topic areas to focus your revision.

GCSE 2024 dates
GCSE 2024 results (when published)
GCSE results 2023
Analysis of GCSE Maths Paper 1 (2023)
Analysis of GCSE Maths Paper 2 (2023)
Analysis of GCSE Maths Paper 3 (2023)
Summary of ALL GCSE Maths Papers (2023)

Here, I go into a bit more detail about how the advance information relates to Paper 2, and make some suggestions for selected revision topics for GCSE Maths Paper 3. This blog focuses on the Edexcel paper, rather than the other exam boards: AQA, OCR or IGCSE.

Be aware that the analysis and recommendations that follow are my own interpretation of GCSE maths Paper 2 2022, and are not endorsed in any way by Edexcel. The question papers, mark schemes and GCSE grade boundaries are yet to be formally released.

See also: Summary of all GCSE maths papers 2022

Paper 2 followed Paper 1 with adherence to advance information

My biggest surprise with GCSE maths Paper 1 2022 was the extent to which the advance information married up with the paper contents. Edexcel’s Maths Emporium originally stated there could be cross-skill assessment of topics not mentioned in the advance information, but there was little evidence of this on Paper 1.

This pattern has continued on GCSE maths Paper 2 2022. Again, the advance information gave a prior warning of every topic on the test, with no significant inclusion of any topic not mentioned in the advance information. As we’ve now had two papers in this style, it’s probably fairly safe to assume that Paper 3 will be similar. 

Read more: GCSE Exams 2022 Advance Information For Maths

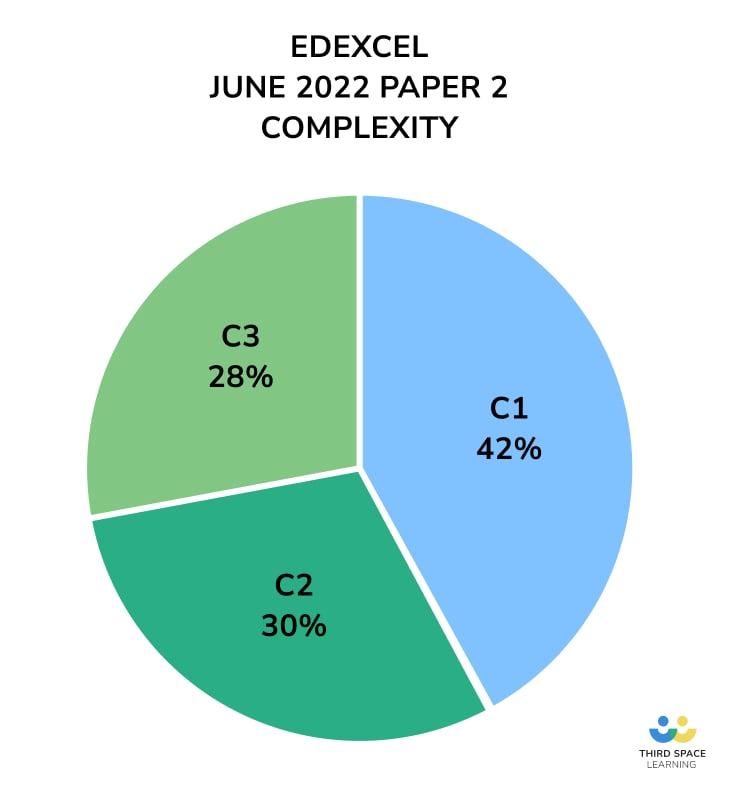
How to understand the charts and graphs that follow

I use the same complexity classifications from my 2017-19 series GCSE Foundation and Higher analysis blogs.

• C1 – standard procedural problems, often worth one or two marks;
• C2 – problems asking candidates to explain their reasoning, interpret information from tables, diagrams or other contexts, or ‘show that’ type questions;
• C3 – non-standard multi-step problems, often requiring the application of skills from a variety of topics, worth three, four, or more marks.

Read more:

• Question Level Analysis Of Edexcel Maths Past Papers (Foundation)
• Question Level Analysis Of Edexcel GCSE Maths Higher Past Papers

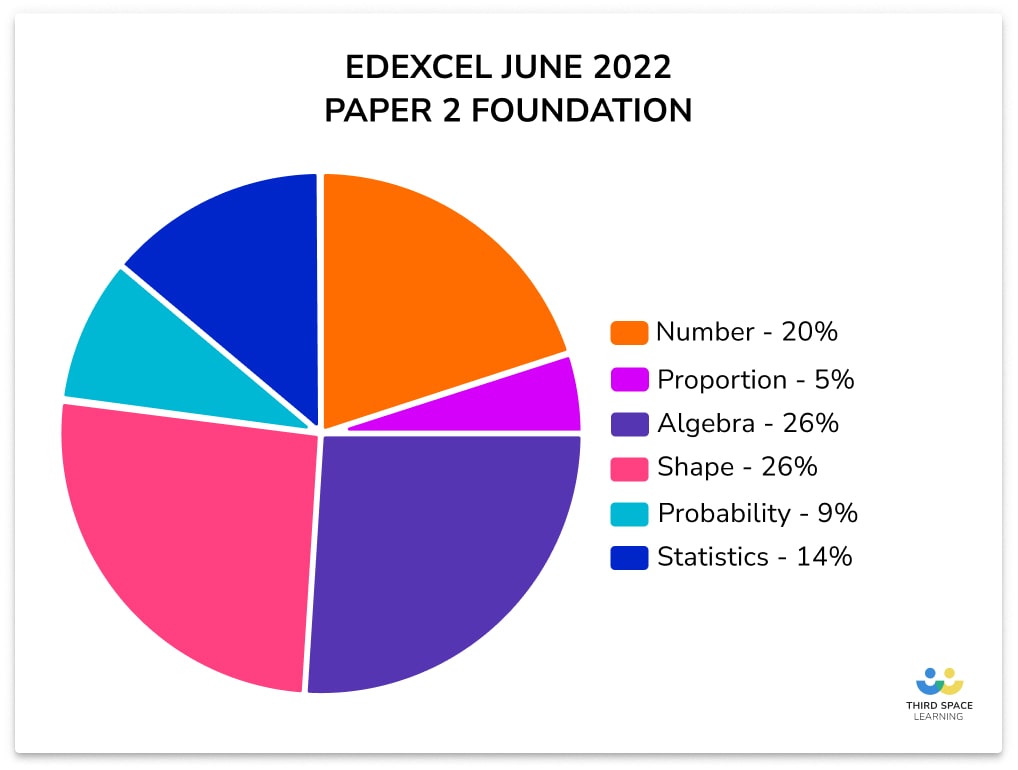
Strand percentages seem to reflect June 2017 (but probably not significant)

Again, I look at the breakdown of questions by strand (Algebra, Number, …) and look at comparisons with previous summer series.

I commented following Paper 1 that the Higher paper, in particular, seemed similarly distributed to June 2017. This trend has continued on Paper 2, with a relatively high proportion of Algebra, Shape and Statistics, and a relatively low proportion of Number and Proportional Reasoning.

However, as before, the variations present aren’t significant when compared to percentage variations in previous series.

Suggestions to support effective Paper 3 revision

Towards the end of the blog, I refine my original suggestions of things to practise with students in the run-up to Paper 3. These aren’t predictions; instead, I’ve offered a few suggestions on how some topics might be combined, based on what we’ve seen in the two papers so far.

Even if these suggestions aren’t accurate, they should provide an opportunity for your students to practise topics in combination with unfamiliar problems.

Paper 3 is likely to follow the advance information (like Paper 1 and 2)

Given that we have now had two papers that match so well with the advance information (and very limited assessment of anything not listed), it is likely that Paper 3 will follow the same pattern.

The advance information lists for Paper 3 are pretty extensive (45 items on both Foundation and Higher). As such, it would be impossible for each item on the advance information to be assessed individually, and it is difficult to see where there is room for assessment of any non-listed topic!

That said, I can offer no guarantees that the situation will be the same for Paper 3, but the advice from my Paper 1 blog still applies; students should only be revising topics off-list if they have completely mastered everything on the advance information list for Paper 3. 

We now take a more detailed look at Paper 2, before thinking ahead to preparations for the final paper. 

Edexcel Paper 2 Foundation more challenging than Paper 1

Paper 2 felt a little more challenging than Paper 1 for Foundation students, particularly with some tricky questions in the crossover content. That said, there were still plenty of opportunities early on in the paper for students to gain some easy marks.

As with Paper 1, topics were generally assessed in a fairly transparent manner. When questions required applications of multiple points from the advance information, this seemed straightforward to spot, such as a frequency table question requiring students to find the median.

There were more C2 questions on this paper than Paper 1. This is partly due to the relatively high proportion of Statistics questions, which frequently ask students to interpret or read information from charts or graphs.

Advance information and Foundation Paper 2 matched well

As with Foundation Paper 1, the advance information matched up almost seamlessly with the questions on the exam paper; it was very easy to spot each bullet point in every question or part question.

For the most part, each topic on the advance information was only assessed once. An interesting exception was coordinates, which appeared at quite a simple procedural level, and again in the crossover content. ‘Money’ appeared a few times on the paper, but this is to be expected as a broad theme at GCSE.

In a few cases, multiple bullet points referred to the same question, such as ‘polygons’ and ‘parallel and perpendicular lines’ referring to a multi-part question about shape properties, and a proportional reasoning question about currency and unit conversions.

The only possible deviation from the advance material was the inclusion of a time series graph. While the advance information mentioned that students would have to interpret a graph, the type of graph was not explicitly stated. However, students could conceivably have answered that question intuitively without much detailed knowledge of time series graphs.

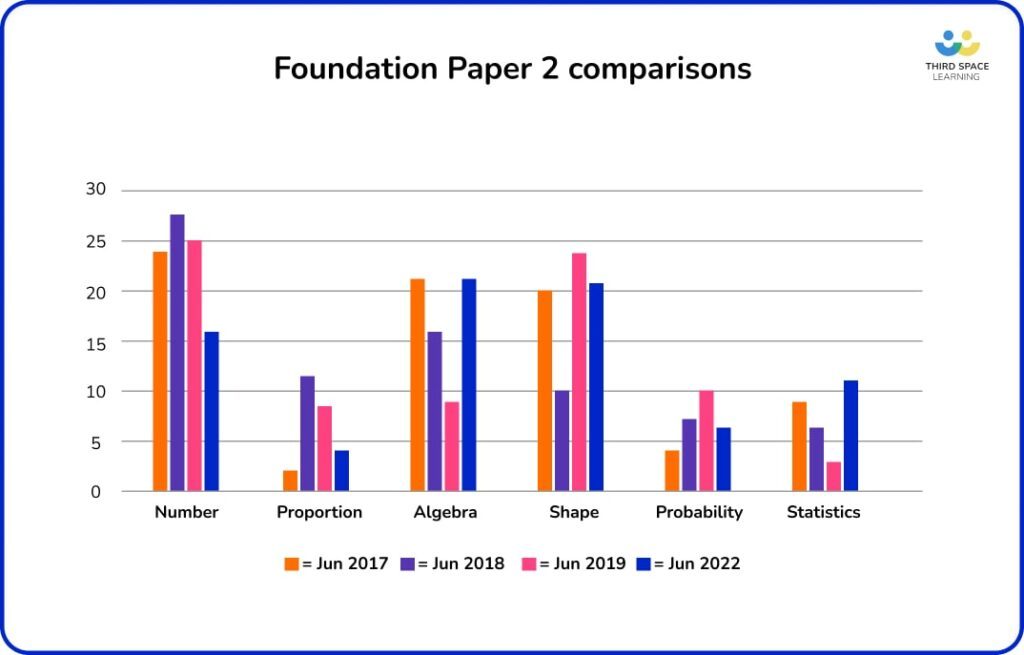
Foundation Paper 2 topics strands less balanced than Paper 1

When compared with previous series, the topic distributions are closest to the first series of exams in June 2017, with a relatively high proportion of Algebra, Shape and Statistics, and relatively little Number work and Proportional Reasoning. 

As we’re expecting more Proportional Reasoning and more Number property work on Paper 3, this is perhaps not especially surprising.

When looking at ‘Shape – Calculations and Measurements’, all of the marks within this strand were for topics involving units, measurements and drawings. In this respect, the paper seemed less balanced than Paper 1.

We might expect Paper 3 to contain quite a bit more work on solving standard linear equations, as we’ve had only two marks so far across both papers. We can also see the topic features twice in the advance information.

Formula sheet redundant for Foundation Paper 2

Students’ reactions indicate that the formula sheet again did not prove useful on GCSE maths Paper 2 2022. In fact, it doesn’t appear that the information provided would be relevant to any question on this paper.

Topics appearing on both lists were all crossover content

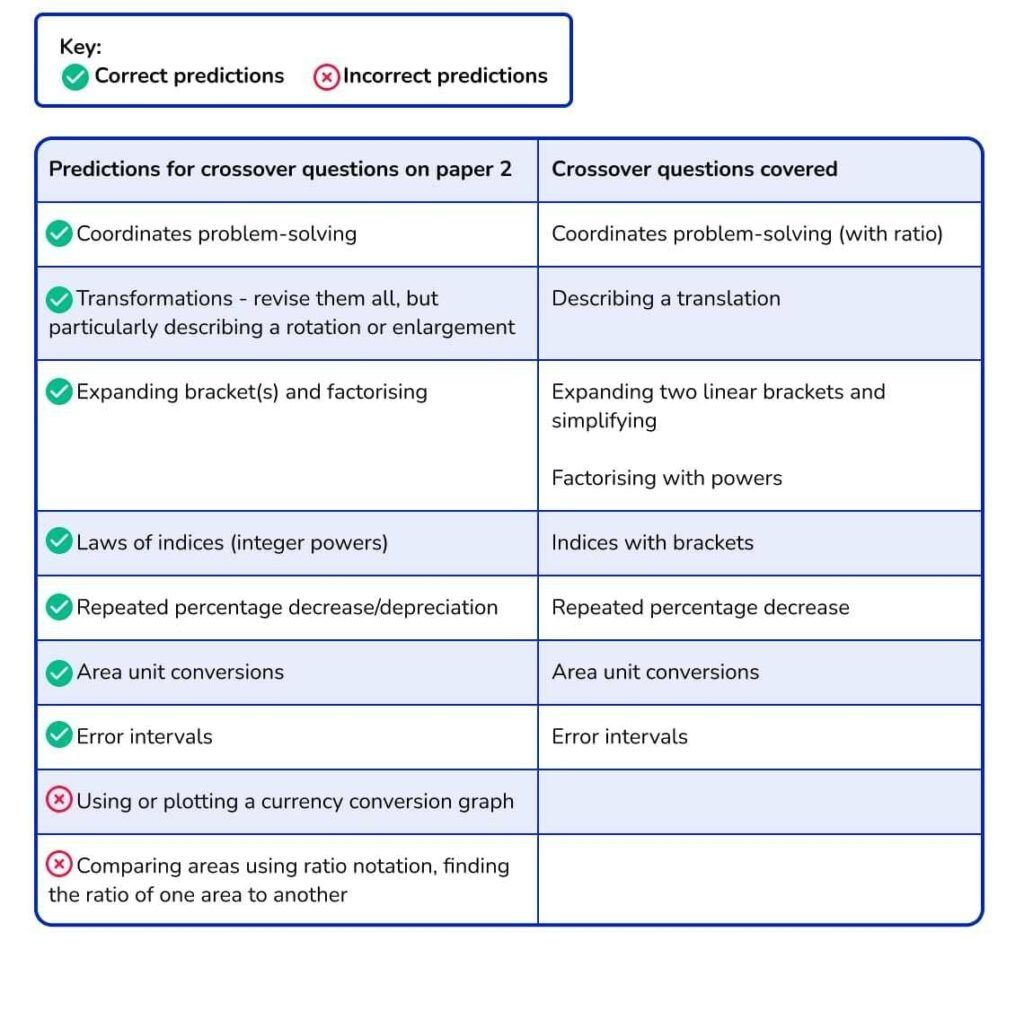
Interestingly for Paper 2, all topics that appeared on both lists did in fact appear in the crossover content, unlike the differing assessment of exact values in trigonometry on Paper 1. 

There were some tricky questions in the crossover content this time, such as coordinates problem-solving. It is also possible that weaker students may have been put off by the non-terminating decimals appearing in the ‘area per person’ question.

When interpreting the lists for Foundation and Higher for Paper 3, remember that a topic appearing on both lists is not a guarantee that it will be assessed in the crossover section, due to some topics (e.g. simplification) being broad-reaching and requiring assessment in different ways on each tier.

Edexcel Higher Paper 2 more positive experience than Paper 1

Students’ initial reactions to Higher Paper 2 seem to be more positive than Paper 1, and the paper itself feels slightly more accessible.

There were a couple of tricky questions – one of my favourites being Marta and Khalid’s wages! The quadratic inequality problem was suitably challenging for the last question on the paper.

As predicted, there was a combined sine/cosine rule question, a fairly standard box plot question requiring students to draw and then interpret, and a transformation of a trigonometric graph (in addition to a simple non-trig transformation).

A big surprise for me was a double inclusion of transformations – the translation in the crossover content, and a negative scale factor enlargement further on in the paper.

As with Foundation, there were more C2 questions on this paper than Paper 1. Again, this was due partly to a higher proportion of Statistics questions, which frequently asked students to interpret or read information from charts or graphs, but also due to the inclusion of Geometry problems involving diagrams, like circle theorems and sine/cosine rule.

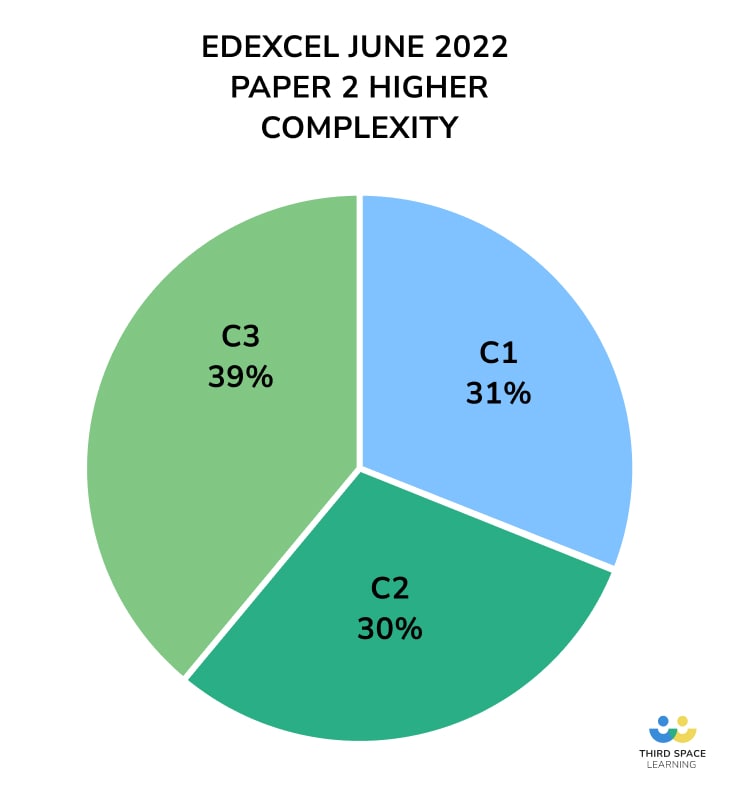
Higher advance information matched Paper 2 more clearly than Paper 1

The Higher advance information again matched well with the questions as they appeared on the paper. Compared to Paper 1, it was easier to match each bullet to a question or part-question.

There also seemed to be fewer topics hidden or embedded in other questions than on Paper 1, with the only notable examples being the linear equation needed to solve the volume of a composite solid problem and the double application of sine/cosine rule. Students could also have used an algebraic method to solve the wages ratio problem.

There were a couple of possible omissions from the advance information this time around – notably the use of difference of two squares, and solving and sketching quadratics in the final question on quadratic inequalities.

However, it’s arguable that solving and sketching quadratics is assumed prior knowledge for tackling quadratic inequality problems. The omission of difference of two squares from the GCSE maths Paper 2 2022 list could indicate that its assessment on Paper 3 will possibly be a more obvious or procedural problem.

We had a few cases where a bulleted topic appeared more than once. For example, there were two questions on transformations; one asking students to describe a translation in the crossover content and one on Higher only regarding negative scale factor enlargement. 

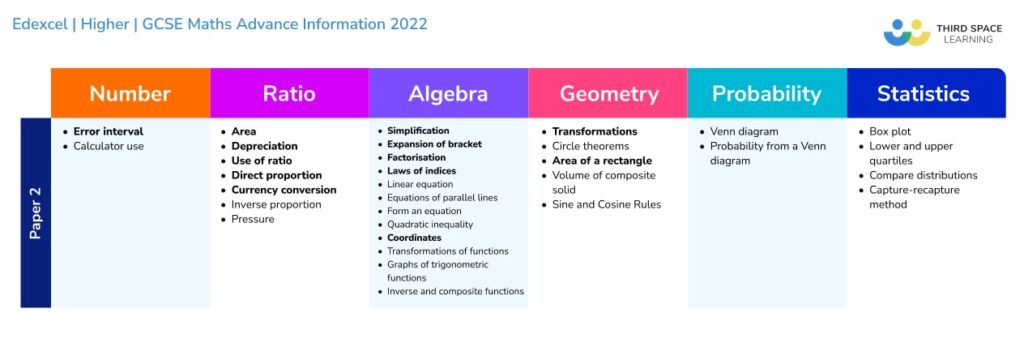
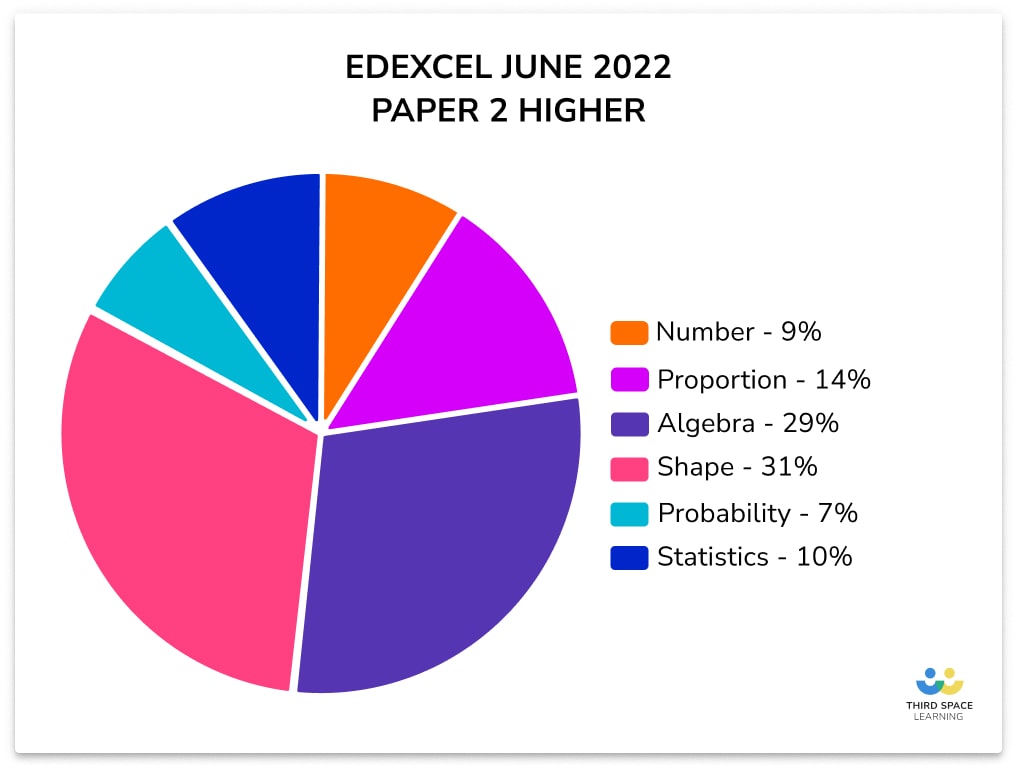
Similar topic strand weighting on Higher Paper 2 as Foundation

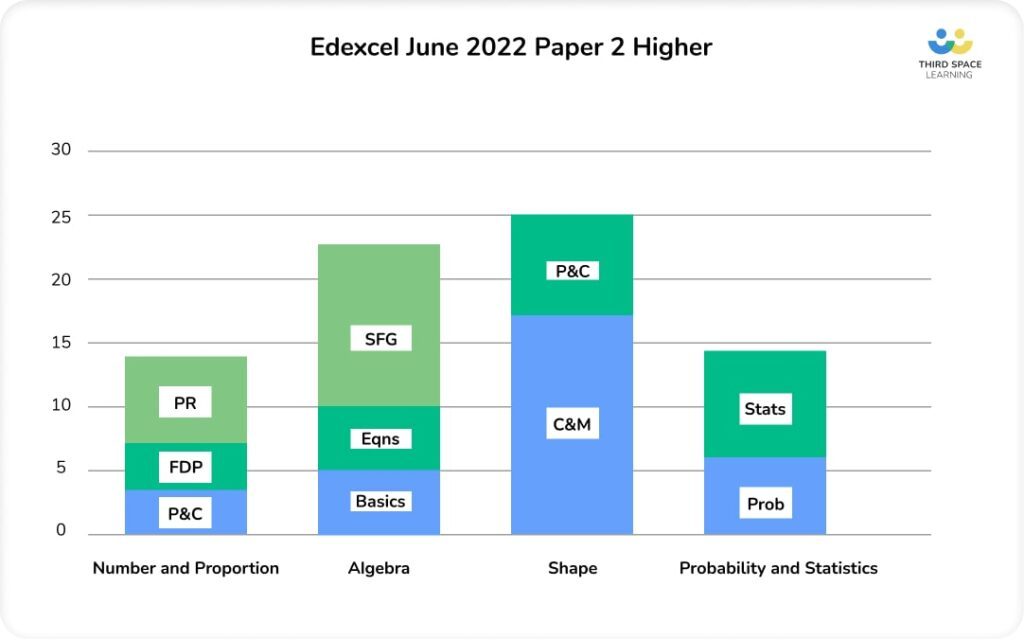
We see a similar strand weighting on Higher Paper 2 to Foundation Paper 2 – relatively fewer marks on Number than on most previous series, and relatively more on Algebra, Shape and Statistics. Again, particularly for Proportion, Shape and Statistics, the distribution most closely models the June 2017 exam papers. It will be interesting to see if this pattern continues in Paper 3.

There were relatively few marks available for procedural algebra basics and algebraic manipulation; the paper instead focused on applications to graphical work and functions, with almost half of the Algebra marks falling within sequences, functions and graphs.

Formula sheet wasn’t useful for Higher Paper 2 either

The formula sheet memes have been popping up all over Twitter again for this exam, and it appears that students haven’t found the sheets particularly useful on Higher.

However, the inclusion of sine and cosine rules on the formula sheet may have removed the obstacle of recalling the formulae and made that question accessible to more students.

Although the second quadratic inequality could be solved by factorisation, it was not a particularly easy factorisation to spot, and therefore students may have referred to the formula sheet for the quadratic formula.

Looking for more GCSE maths practice? Third Space Learning offers a wealth of resources to help prepare students for GCSEs and beyond, from free revision guides and worksheets, to online one-to-one revision sessions. Take a look at our collection of GCSE practice papers!

Using the advance information to direct revision for Paper 3

Now we’re on the home straight with two papers in the bag, we can refine some ideas for Paper 3 preparation. For Papers 1 and 2, the advance information provides a list of the exam content, with no significant inclusion of topics beyond the lists.

It is reasonable to continue with the assumption that any deviations will be very few, and we should use the advance information lists as a guide for revision topics on Paper 3, with students only revising topics from outside these lists if they are fully confident they have mastered the listed content.

Same topic, different paper

Edexcel has previously examined the same topic in multiple papers, and we have seen examples in this series with direct proportion on Foundation. On Paper 1, we had a more straightforward recipe adaptation problem, and on Paper 2 we had a high-tariff currency and unit conversion problem to solve. It appears again on Paper 3 (for both the Foundation and Higher lists), so it’s possible we’ll get a proportion question in the crossover content. 

It’s difficult to predict what this might be; Foundation candidates are only expected to be able to interpret (as opposed to form) proportion equations. We had a clear statement of ‘equations of proportion’ when this topic appeared on Higher. However, Foundation students have not yet been assessed on proportion equations at all, so we may see one of these after all!

Similarly, linear sequences appeared on Paper 1 Foundation as a simple pattern-based question, so Paper 3 could be finding the nth term or showing that a number is or isn’t in a sequence. 

Different topics, same question

In the sections below, I’ve re-examined the lists for Paper 3 Foundation and Higher and tried to piece together topics that may appear in the same question.

Although we can’t guarantee that this will be how the next paper looks, revising topics in various combinations will also help students to apply their skills to unfamiliar contexts in the third and final exam, even if they do not appear in the ways I’ve listed below.

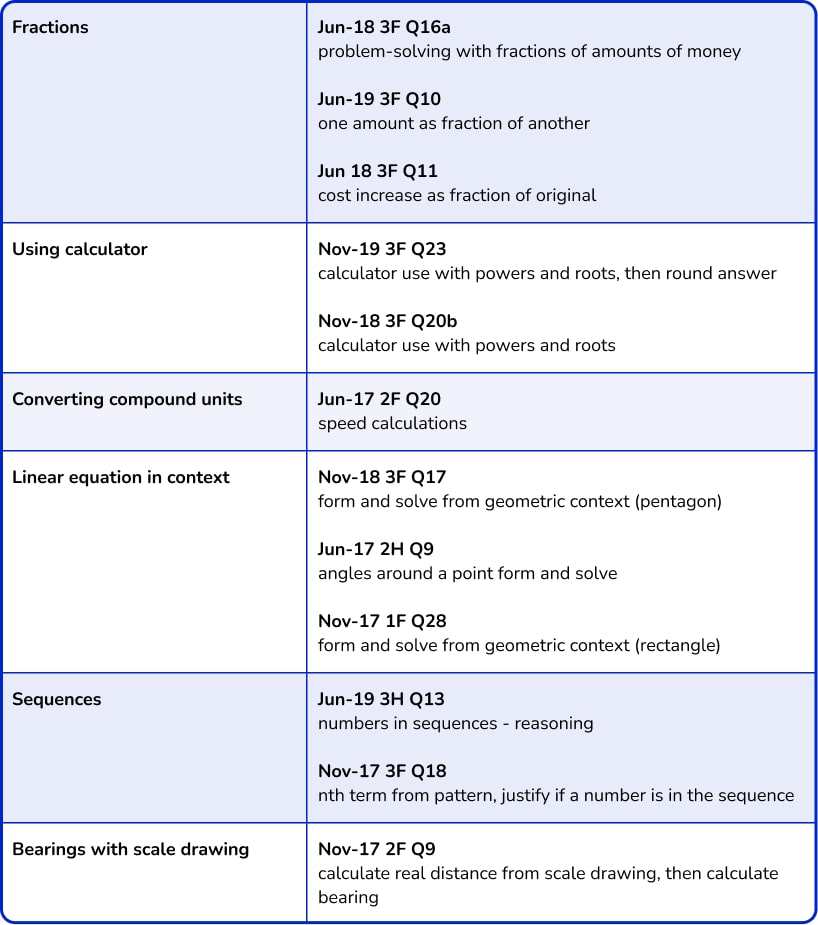
What should students be revising for Paper 3 Foundation?

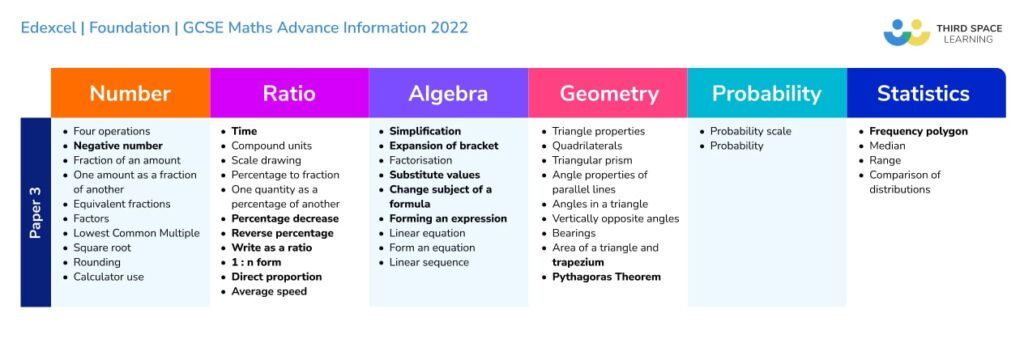
This is not an exhaustive list, but I’d focus on:

• Problem-solving with fractions, possibly including a percentage to convert
• Finding LCM (procedural)
• Using a calculator including square root symbol, rounding answer
• Converting compound units, possibly speed 
• Factorising a simple expression
• Forming a linear equation from a context and solving (simple as not crossover), possibly involving the area of a triangle
• nth term of a linear sequence, or justifying if a term is in a sequence
• Angle properties problem solving* – could include an equilateral or isosceles triangle due to the mention of triangle properties
• Bearings, possibly linked to scale drawing
• Find median and range, and compare distributions
• Simple probability using scale

* We had the assessment of ‘parallel and perpendicular lines’ on Paper 2 as simply labelling on a diagram, so it’s almost certain that ‘angle properties of parallel lines’ will be a pair of lines with a transversal and missing angles to find.

Suggested past paper questions for Paper 3 Foundation

Paper 3 crossover topics to revise

As I’ve mentioned before, not every topic that appears in both Foundation and Higher lists will be crossover content.

However, I have picked out a few ideas to work on with students, with the rationale that, at this stage, any practice on key topics will help, even if the topics don’t appear quite as I’ve listed them.

• A two-part percentage problem, with a decrease and a reverse percentage
• Ratio problem solving, including using 1:n form
• Pythagoras’ theorem – could involve the area of a trapezium
• Changing the subject of a formula
• Frequency polygons
• Direct proportion – possibly a proportion equation

Suggested past paper questions for Paper 3 crossover content

What should students be revising for Paper 3 Higher?

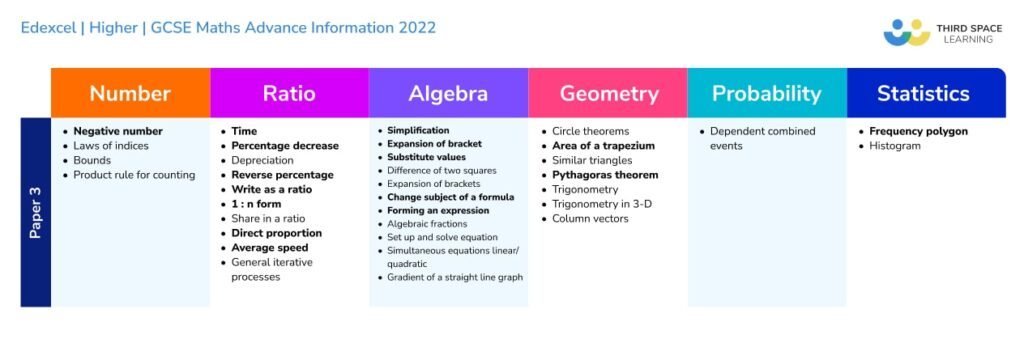
This is not an exhaustive list, but I’d focus on:

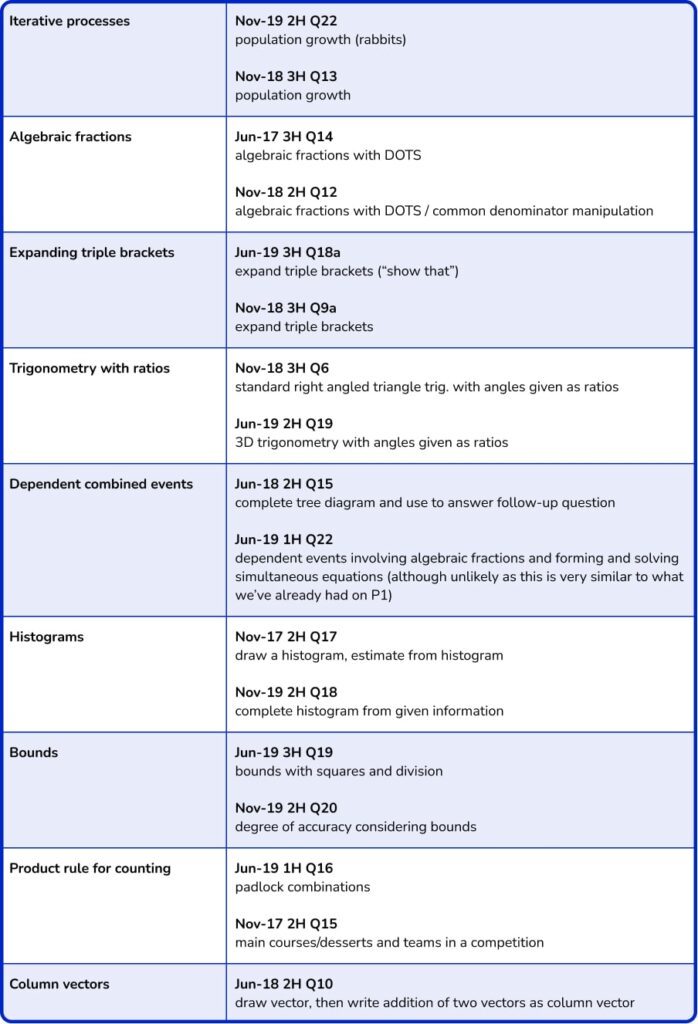
• General iterative processes, referring to population growth/decay (see Nov-19 2H Q22) and could be linked to the depreciation mentioned, particularly as we’ve had a more standard compound decrease on Paper 2
• Algebraic fractions, possibly including the difference of two squares
• Expanding double/triple brackets*
• Something similar to Jun-18 3H Q19, which includes trigonometry, similar triangles, algebraic fractions, setting up and solving a quadratic, and expanding brackets – so this is good practice for lots of advance information skills anyway!
• Trigonometry** (SOHCAHTOA & 3D) where angles are given in a ratio
• Dependent combined events***
• Histograms, bounds, product rule and column vectors, probably as stand-alone questions

* On Paper 2, ‘expansion of bracket’ appeared as an expansion and simplification of two linear brackets. It is mentioned in the singular again in the crossover content. This could be a standalone procedural question or embedded in another problem, such as changing the subject of a formula. ‘Expansion of brackets’ in the plural is Higher only, which makes it reasonable to conclude that this may be triple brackets.

** Trigonometry appears twice on the advance information list for Paper 3, so presumably we’ll have a SOHCAHTOA question and a 3D trigonometry question. The inclusion of both is what has raised my suspicions that the SOHCAHTOA question may involve an element of problem-solving.

*** The question on independent combined events on Paper 1 was a less structured problem-solving question, which was most easily solved by forming and solving an equation. It is possible that the dependent events question on this paper will be a more procedural question with a pre-drawn tree diagram.

Suggested past paper questions for Paper 3 Higher

Top tips for GCSE advance information revision

• Revise the content mentioned in the advance information before you revise other topics.
• If you’re stuck on how to start a question, try and work out what topics listed on the advance information you haven’t seen yet – this might give a clue on how to start.
• There will be combinations of topics within questions – don’t look for one question per topic.
• Use our suggested past paper questions to practise some of the advance information topics.
• If you haven’t already done so, download and work through Third Space Learning’s GCSE revision resources, including free worksheets.

To all GCSE students, whichever your exam board, best of luck on the final GCSE paper!

Free GCSE maths revision resources for schools
As part of the Third Space Learning offer to schools, the personalised online GCSE maths tuition can be supplemented by hundreds of free GCSE maths revision resources from the secondary maths resources library including:
– GCSE maths past papers
– GCSE maths worksheets
– GCSE maths questions
– GCSE maths topic list