Dividing Exponents - Math Steps, Examples & Questions

High Impact Tutoring Built By Math Experts

Personalized standards-aligned one-on-one math tutoring for schools and districts

Request a demo

In order to access this I need to be confident with:

Exponents Simplifying expressions Order of operations

Math resources Algebra Laws of exponents

Dividing exponents

Here you will learn about dividing exponents, including how to use the laws of exponents to divide exponents and how to divide exponents that have different bases.

Students will first learn about dividing exponents as a part of expressions and equations in $8$ th grade math, and will continue to expand on the knowledge throughout high school.

What is dividing exponents?

Dividing exponents is where you divide terms that involve exponents, or powers. You can divide exponents in various forms, including whole numbers, negative numbers, fractions, and decimals.

When dividing numerical or algebraic expressions that have the same base, you can subtract the exponents.

For example,

8^7 \div 8^4=

You can rewrite the division problem in expanded form,

8^7 \div 8^4=\cfrac{8^7}{8^4}=\cfrac{8 \times 8 \times 8 \times 8 \times 8 \times 8 \times 8}{8 \times 8 \times 8 \times 8}

There are seven $8’$ s on the top and four $8’$ s on the bottom. So, the expression can be simplified to be:

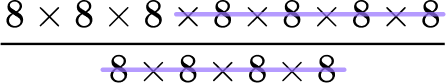
This is equivalent to $\cfrac{8 \times 8 \times 8}{1}=8^3$

Another way to think about it is to subtract exponents.

8^7 \div 8^4=8^{7-4}=8^3=512

What happens if the bases are not the same?

If the bases of the exponential expression are not the same, before calculating an answer, try to rewrite the expression so the bases are the same.

For example,

9^4 \div 3^4=

Let’s try to rewrite $9^4$ so that it has a base of $3.$

$9$ is the same as $3^2$

Replace $9$ with $3^2$ in the original expression.

\left(3^2\right)^4 \div 3^4=

This is the same as,

$3^2 \times 3^2 \times 3^2 \times 3^2,$ which is $3^8 \div 3^4$

Now the bases are the same, so you can subtract exponents.

3^8 \div 3^4=3^{8-4}=3^4=81

Let’s look at one more example.

a^{\frac{3}{4}} \div a^{\frac{1}{2}}

Since the bases are the same, you can subtract exponents.

a^{\frac{3}{4} \, - \, \frac{1}{2}}=a^{\frac{3}{4} \, - \, \frac{2}{4}}=a^{\frac{1}{4}}

[FREE] Algebra Check for Understanding Quiz (Grade 6 to 8)

Use this quiz to check your grade 6 – grade 8 students’ understanding of algebra. 10+ questions with answers covering a range of 6th to 8th grade algebra topics to identify areas of strength and support!

DOWNLOAD FREE

[FREE] Algebra Check for Understanding Quiz (Grade 6 to 8)

DOWNLOAD FREE

The rule for dividing exponential expressions is:

a^m \div a^n=a^{m \, - \, n}

*bases must be the same

What is dividing exponents?

Common Core State Standards

How does this relate to $8$ th grade math – algebra?

Grade 8: Expressions and Equations (8.EE.A.1)
Know and apply the properties of integer exponents to generate equivalent numerical expressions. For example, $32 \times 3 \, – \, 5 = 3 \, – \, 3 = \cfrac{1}{33} = \cfrac{1}{27}.$

High School – The Real Number System (HSN-RN.A.1)
Explain how the definition of the meaning of rational exponents follows from extending the properties of integer exponents to those values, allowing for a notation for radicals in terms of rational exponents.

For example, we define $\cfrac{51}{3}$ to be the cube root of $5$ because we want $(\cfrac{51}{3})^3 = 5(\cfrac{1}{3})^3$ to hold, so $(\cfrac{51}{3})^3$ must equal $5.$

How to divide exponents with the same base

In order to divide exponents with the same base, you will:

Subtract the exponents.
Divide any coefficients of the base variables.

Dividing exponents examples

Example 1: same base with different exponents

Simplify and leave your answer in index form.

a^{5} \div a^{2}

Subtract the exponents.

Subtract the exponents $5$ and $2.$

5 \, - \, 2=3

2Divide any coefficients of the base variables.

Because the coefficients are $1,$

a^{5} \div a^{2}=a^{3}

Example 2: same base with a coefficient in front of base

Simplify and leave your answer in index form.

21 a^{9} \div 7 a^{2}

Subtract the exponents.

Subtract the exponents $9$ and $2.$

$9 \, - \, 2 = 7$

Divide any coefficients of the base variables.

Divide the coefficients $21$ and $7.$

$21 \div 7=3$

So,

$21 a^{9} \div 7 a^{2}=3a^{7}$

Example 3: same base dividing negative exponents

Simplify and leave your answer in index form.

18 a^{-6} \div 9 a^{-4}

Subtract the exponents.

Subtract the powers $-6$ and the $-4.$

$-6-(-4)=-6+4=-2$

Divide any coefficients of the base variables.

Divide the coefficients $18$ and the $9.$

$18 \div 9=2$

So,

$18 a^{-6} \div 9 a^{-4}=2 a^{-2}$

Remember to change negative exponents to positive exponents.

$a^{-2}=\cfrac{1}{a^2}$

So, $2 a^{-2}=2 \times \cfrac{1}{a^2}=\cfrac{2}{a^2}$

Example 4: same base with fractional exponents

Simplify and leave your answer in index form.

x^{\frac{4}{5}} \div x^{\frac{2}{3}}

Subtract the exponents.

Subtract the exponents $\cfrac{4}{5}$ and $\cfrac{2}{3}.$

$\cfrac{4}{5} \, - \, \cfrac{2}{3}$

Remember, to subtract fractions, find a common denominator.

\begin{aligned} \cfrac{4}{5} - \cfrac{2}{3} &= \cfrac{12}{15} - \cfrac{10}{15}\\\\ &= \cfrac{2}{15} \end{aligned}

Divide any coefficients of the base variables.

Because the coefficients are $1,$

$x^{\frac{4}{5}} \div x^{\frac{2}{3}}=x^{\frac{2}{15}}$

How to divide exponents when the bases are different

In order to divide exponents when the bases are different, you will:

Rewrite the expressions that can have matching bases.
Subtract the exponents of the expressions with matching bases.
Work out any of the other calculations and simplify.

Example 5: different base with positive exponents

Evaluate:

4^2 \div 2^3

Rewrite the expressions that can have matching bases.

4^2 \div 2^3

$4$ can be rewritten to be $2^2.$ So, you can replace $4$ with $2^2$

$\left(2^2\right)^2 \div 2^3=2^4 \div 2^3=$

Subtract the exponents of the expressions with matching bases.

Since the bases are the same, you can subtract the exponents.

$2^4 \div 2^2=2^{4-2}=2^2$

Work out any of the other calculations and simplify.

In exponential form, the answer is $2^2.$

The calculation is:

$2^2=4$

Example 6: different base with three terms

Evaluate:

8^2 \div 2^4 \div 3^2

Rewrite the expressions that can have matching bases.

8^2 \div 2^4 \div 3^2

$8$ is the same as $2^3.$ So, you can replace $8$ with $2^3.$

$\left(2^3\right)^2 \div 2^4 \div 3^2$

$2^6 \div 2^4 \div 3^2=$

Subtract the exponents of the expressions with matching bases.

Subtract the exponents of the exponential expressions with the same bases.

\begin{aligned} & 2^6 \div 2^4 \div 3^2= \\\\ & 2^{6-4} \div 3^2= \\\\ & 2^2 \div 3^2= \end{aligned}

Work out any of the other calculations and simplify.

In exponential form, the answer is $2^3 \div 3^2$

The calculation is:

$2^2 \div 3^2=4 \div 9=\cfrac{4}{9}=0 . \overline{4}$

Teaching tips for dividing exponents

Introduce students to real-life scenarios that use dividing exponents, including compound interest and scientific notation.

Provide worksheets with word problems that require students to apply the quotient rule with whole numbers and negative bases.

For students struggling with dividing exponents, provide a step-by-step tutorial that students can access as needed. For example, on an anchor chart or in their individual math journals.

Easy mistakes to make

The dividing exponents law can only be used for terms with the same base
For example,
You cannot simplify
$a^{4} \div b^{3}$
as the bases are different.

Dividing exponents rather than subtracting exponents
Remember to subtract the powers, not divide them.

Forgetting to include the base when writing the answer
For example, simplifying
$6a^{10} \div 2a^{3}$
to
$3^{7}$
when it should be
$3a^{7}$

Practice dividing exponents questions

x^{11}

x^{4.5}

7x

x^{7}

9 \, – \, 2 = 7

x^{9} \div x^{2} = x^{7}

3b^{6}

8b^{6}

3b^{\cfrac{13}{7}}

3^{6}

13 \, – \, 7 = 6

12 \div 4 = 3

12 b^{13} \div 4 b^{7}=3b^{6}

9 a^{\frac{2}{3}}

56a

9a

9 a^{-5}

-2 \, – \, (-3) = -2 \, + \, 3 = 1

63 \div 7=9

63a^{-2} \div 7 a^{-3} = 9a

(When the power is $1,$ you do not need to write it.)

4 x^{\frac{5}{12}}

2 x^{\frac{5}{12}}

0.5 x^{\frac{5}{12}}

2 x^{\frac{9}{4}}

\begin{aligned} \frac{3}{4}-\frac{1}{3} &= \cfrac{9}{12}-\cfrac{4}{12} \\\\ &= \cfrac{5}{12} \end{aligned}

4 \div 2 = 2

4 x^{\frac{3}{4}} \div 2 x^{\frac{1}{3}} = 2 x^{\frac{5}{12}}

3^3

3^{-1}

9

3^{-3}

Rewrite the expression so that the bases are the same.

$27$ is the same as $3^3.$ So, you can replace $27$ with $3^3.$

\begin{aligned} & 27^2 \div 3^3= \\\\ & \left(3^3\right)^2 \div 3^3= \end{aligned}

You can apply the rule and subtract exponents.

\begin{aligned} & 3^6 \div 3^3= \\\\ & 3^{6-3}=3^3 \end{aligned}

20.25

108

\cfrac{81}{\sqrt{2}}

324

There is no possibility to rewrite the terms so they have the same bases. So, perform the calculations.

\begin{aligned} 3^{4}&=3 \times 3 \times 3 \times 3=81\\\\ 2^{-2}&=\cfrac{1}{2^2}=\cfrac{1}{4} \end{aligned}

\begin{aligned} 3^{4} \div 2^{-2} &= 81 \div \cfrac{1}{4}\\\\ &=81 \times 4\\\\ &=324 \end{aligned}

Dividing exponents FAQs

What is an exponent?

An exponent is a small number that is written above and to the right of a number, known as the base number. This indicates how many times a number is multiplied by itself (repeated multiplication).

For example, $2^4, 2$ is the base number and $4$ is the exponent.

What is the negative exponent rule?

The negative exponent rule is, for any nonzero number $a$ and any integer $n, a^{-n}$ is equal to $\cfrac{1}{a^n}.$ Taking a negative exponent is equivalent to finding the reciprocal of the corresponding positive exponent.

Can you divide exponents that are fractions or decimals?

Yes, you can still apply the rules of exponents when dealing with exponential expressions that involve decimal or fractional exponents.

For example, $\cfrac{x^3}{x\frac{1}{2}} =x^3 \div x^{\frac{1}{2}}=x^{3-\frac{1}{2}}=x^{\frac{5}{3}}.$ You will subtract the exponent in the denominator from the exponent in the numerator.

What is the quotient of powers rule?

The quotient of powers rule states that when dividing exponent with the same base, subtract the exponents.

For example, $\cfrac{x^n}{x^m}=x^{n \, - \, m}.$

The next lessons are

Still stuck?

At Third Space Learning, we specialize in helping teachers and school leaders to provide personalized math support for more of their students through high-quality, online one-on-one math tutoring delivered by subject experts.

Each week, our tutors support thousands of students who are at risk of not meeting their grade-level expectations, and help accelerate their progress and boost their confidence.