High Impact Tutoring Built By Math Experts
Personalized standards-aligned one-on-one math tutoring for schools and districts
In order to access this I need to be confident with:
Multiplication and division Order of operations Negative numbers PolynomialDistributing exponents
Here you will learn about distributing exponents, including how to distribute exponents using the laws of exponents.
Students will first learn about distributing exponents as a part of expressions and equations in 8 th grade and will continue expanding on their knowledge in high school algebra.
What is distributing exponents?
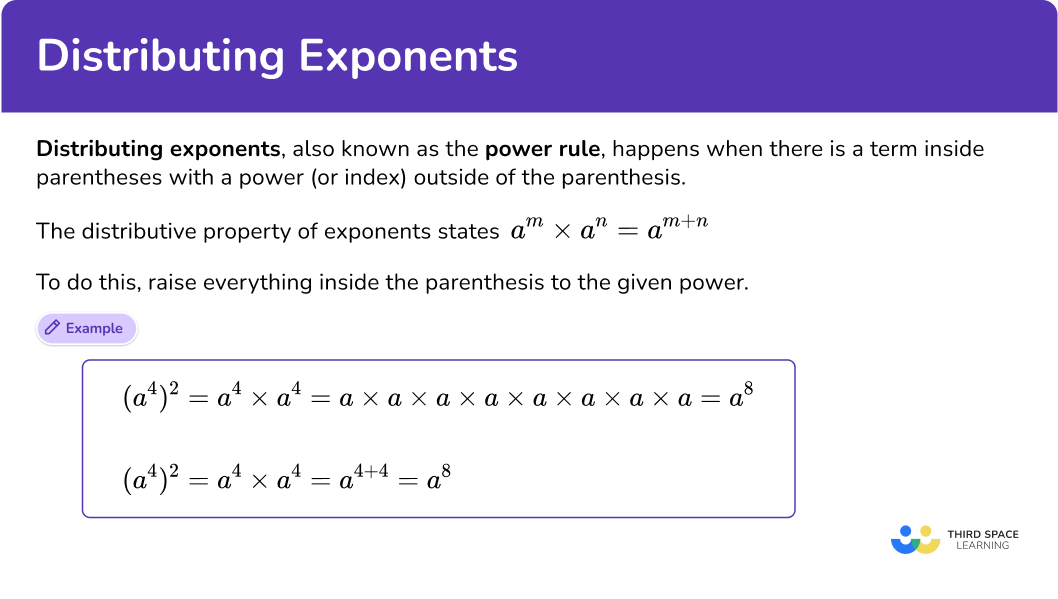
Distributing exponents, also known as the power rule, happens when there is a term inside parentheses with a power (or index) outside of the parenthesis.
It is one of the laws of exponents, or rules of exponents, called the distributive property of exponents.
You can distribute all rational exponents, including positive exponents and negative exponents.
The distributive property of exponents states a^m \times a^n=a^{m+n}
To do this, raise everything inside the parenthesis to the given power.
For example,
\begin{aligned}& \left(a^4\right)^2=a^4 \times a^4=a \times a \times a \times a \times a \times a \times a \times a=a^8 \\\\ & \left(a^4\right)^2=a^4 \times a^4=a^{4+4}=a^8 \end{aligned}Another method would be to multiply the exponents.
For example,
\left(a^4\right)^2=a^{4 \times 2}=a^8In general, when there is an exponent outside the parentheses, multiply the powers. This is known as the power of a power rule.
\left(a^m\right)^n=a^{m \times n}=a^{m n}![[FREE] Distributing Exponents Worksheet (Grade 8)](https://thirdspacelearning.com/wp-content/uploads/2024/04/Distributing-Exponents-Worksheet-listing-image.png)
[FREE] Distributing Exponents Worksheet (Grade 8)
Use this worksheet to check your grade 8 students’ understanding of distributing exponents. 15 questions with answers to identify areas of strength and support!
DOWNLOAD FREE [FREE] Distributing Exponents Worksheet (Grade 8)
Use this worksheet to check your grade 8 students’ understanding of distributing exponents. 15 questions with answers to identify areas of strength and support!
DOWNLOAD FREEDistributing fractional exponents
There may be times where you are expected to distribute an exponent that is a fraction. Some common fractional exponents include,
Square root: a^{\frac{1}{2}}=\sqrt{a}
Cube root: a^{\frac{1}{3}}=\sqrt[3]{a}
Fourth root: a^{\frac{1}{4}}=\sqrt[4]{a}
where the denominator of the fraction signifies the root.
What is distributing exponents?
Common Core State Standards
How does this relate to 8 th grade math?
- Grade 8: Equations and expressions (8.EE.A.1)
Know and apply the properties of integer exponents to generate equivalent numerical expressions.
How to distribute exponents
In order to distribute exponents:
- Raise the term inside the parentheses by the power outside the parentheses.
- Make sure you have considered the coefficient.
- Write the final answer.
Distributing exponents examples
Example 1: power of a power
Write as a single power of 5.
(5^3)^2- Raise the term inside the parentheses by the power outside the parentheses.
It is quicker to multiply the exponents together.
(5^3)^2=5^{3\times2}=5^62Make sure you have considered the coefficient.
There is no coefficient to consider.
3Write the final answer.
The question asked for the answer to be as a single power, so the final answer is 5^6.
Example 2: fraction number base
Simplify the following expression.
\left(\cfrac{2}{3}\right)^4Raise the term inside the parentheses by the power outside the parentheses.
Distribute the exponent 4 to both the numerator and denominator of the fraction.
\begin{aligned}& \cfrac{2^4}{3^4} \\\\
& \cfrac{2 \times 2 \times 2 \times 2}{3 \times 3 \times 3 \times 3} \\\\
& \cfrac{16}{81} \end{aligned}
Make sure you have considered the coefficient.
There is no coefficient to consider.
Write the final answer.
The fraction is in simplest form, so the answer is \cfrac{16}{81}.
Example 3: algebraic base with coefficient of 1
Write as a single power.
(x^3)^4Raise the term inside the parentheses by the power outside the parentheses.
It is quicker to multiply the exponents together.
(x^3)^4=x^{3\times4}=x^{12}
Make sure you have considered the coefficient.
The coefficient is 1 and does not need special consideration.
Write the final answer.
The question asked for the answer to be as a single power, so the final answer is x^{12}.
Example 4: algebraic base with coefficient of 1
Write as a single power.
(y^4)^5Raise the term inside the parentheses by the power outside the parentheses.
It is quicker to multiply the exponents together.
(y^4)^5=y^{4\times5}=y^{20}
Make sure you have considered the coefficient.
The coefficient is 1 and does not need special consideration.
Write the final answer.
The question asked for the answer to be as a single power, so the final answer is y^{20}.
Example 5: algebraic base with a coefficient greater than 1
Simplify (4y^2)^3 .
Raise the term inside the parentheses by the power outside the parentheses.
You can split the term inside the bracket into the coefficient and the base with its power.
The base and its index is y^2
This is being raised to the power 3.
It is quicker to multiply the indices exponents together.
(y^2)^3=y^{2\times3}=y^6
Make sure you have considered the coefficient.
The coefficient is 4. This also needs raising to the power of 3.
4^3 = 4\times4\times4=64
Altogether it would be,
(4y^2)^3=4^3 \times y^{2\times3}=64y^6
Write the final answer.
The question asked for the answer to be as a single power, so the final answer is 64y^6.
Example 6: algebraic base with a coefficient greater than 1
Simplify (3a^5)^2 .
Raise the term inside the parentheses by the power outside the parentheses.
You can split the term inside the bracket into the coefficient and the base with its power.
The base and its power is a^5
This is being raised to the power 2.
It is quicker to multiply the exponents together.
(a^5)^2=a^{5\times2}=a^{10}
Make sure you have considered the coefficient.
The coefficient is 3. This also needs raising to the power of 2.
3^2 = 3\times3=9
Altogether it would be,
(3a^5)^2=3^2 \times a^{5\times2}=9a^{10}
Write the final answer.
The question asked for the answer to be as a single power, so the final answer is 9a^{10}.
Teaching tips for distributing exponents
- Make sure students are familiar with the basic properties of exponents, including power of a power, power of a product and power of a quotient.
- Provide students with step-by-step instruction, starting with simple problems and gradually increasing the complexity of them. This is also a good time to emphasize common mistakes students may see.
- Allow students the opportunity to explain their thinking when distributing exponents. Struggling students can benefit from hearing explanations from fellow students.
Easy mistakes to make
- Multiplication sign between parts of a term when simplifying
You do not need a multiplication sign between the coefficient and the algebraic letter. For example, with (5x^3)^2=5^2\times x^{3\times2}=25\times x^6 the final answer would be 25x^6.
- Not raising everything inside the brackets to the power outside the bracket
It is a common error to forget to raise the coefficient (the whole number multiplying the algebraic term) to the power outside of the fraction. In the example below, it is easy to forget to square the coefficient 4.
(4a^6)^2=4^2\times a^{6\times2}=16\times a^{12}
Related laws of exponents lessons
Practice distributing exponents questions
1. Write as a number to a single power: (2^3)^4
2. Write as a number to a single power: (7^2)^3
3. Write as a single power: (x^4)^2
4. Write as a single power: (h^9)^7
5. Simplify: (2d^3)^2
6. Simplify: (5e^4)^3
Distributing exponents FAQs
The properties of exponents are a set of rules that are fundamental for working with powers and the simplification of expressions in algebra. These include the product of powers, quotient of powers, power of a power, power of a product and power of a quotient.
Yes, you can distribute negative powers. You would following the negative exponent basics which states that a^{- \, n}=\cfrac{1}{a^n}.
The zero exponent property explains that any non-zero number raised to the zero power is equal to one. For example, 12^0=1.
The next lessons are
Still stuck?
At Third Space Learning, we specialize in helping teachers and school leaders to provide personalized math support for more of their students through high-quality, online one-on-one math tutoring delivered by subject experts.
Each week, our tutors support thousands of students who are at risk of not meeting their grade-level expectations, and help accelerate their progress and boost their confidence.
Find out how we can help your students achieve success with our math tutoring programs.
[FREE] Common Core Practice Tests (3rd to 8th Grade)
Prepare for math tests in your state with these 3rd Grade to 8th Grade practice assessments for Common Core and state equivalents.
Get your 6 multiple choice practice tests with detailed answers to support test prep, created by US math teachers for US math teachers!