FREE DOWNLOAD
Mutually Exclusive Events Worksheet
Help your students prepare for their Maths GCSE with this free mutually exclusive events worksheet of 20+ questions and answers
- Section 1 of the mutually exclusive events worksheet contains 20+ skills-based mutually exclusive events questions, in 3 groups to support differentiation
- Section 2 contains 4 applied mutually exclusive events questions with a mix of worded problems and deeper problem solving questions
- Section 3 contains 3 foundation and higher level GCSE exam style mutually exclusive events questions
- Answers and a mark scheme for all mutually exclusive events questions are provided
- Questions follow variation theory with plenty of opportunities for students to work independently at their own level
- All questions created by fully qualified expert secondary maths teachers
Suitable for GCSE maths revision for AQA, OCR and Edexcel exam boards
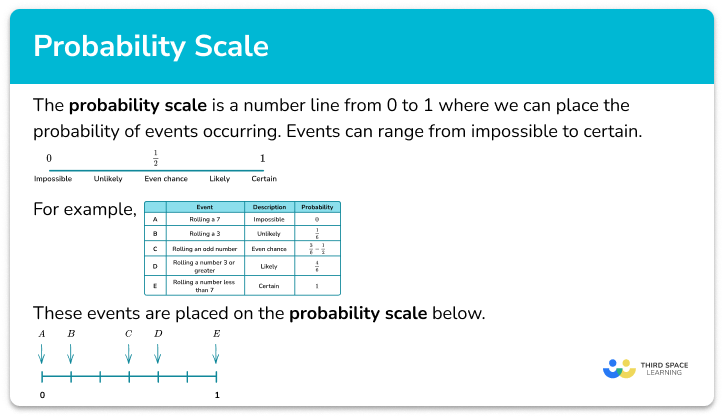
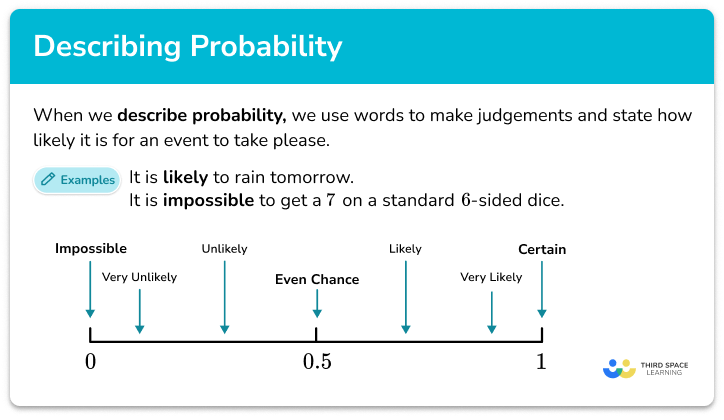
Mutually exclusive events at a glance
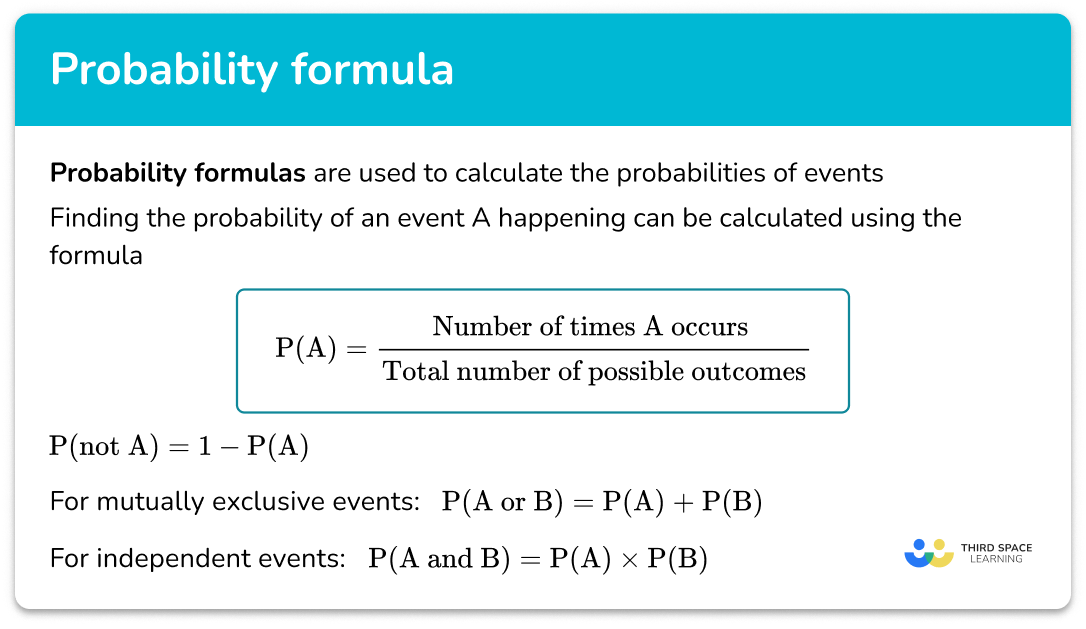
Mutually exclusive events are events that cannot occur at the same time, for example rolling a 1 and a 2 on a dice. For two mutually exclusive events A and B, the probability of an event A happening OR an event B happening, known as P(A or B), is equal to the sum of the probability of event A happening P(A), added to the probability of event B happening (P(B).
In mathematical notation: P(A or B) = P(A) + P(B).
Let’s consider a pack of playing cards. The probability of getting a face card is 12 out of 52, and the probability of getting a card showing a prime number is 16 out of 52. The probability of getting a face card or a prime number is the sum of these two probabilities, which is 28 out of 52. The sum of the probabilities of an exhaustive set of mutually exclusive events is 1.
An example of a non-mutually exclusive event would be choosing a face card or a red card. Half of the face cards are red cards and so we add an extra condition by removing any overlapping outcomes (the probability of a face card AND a red card) from the probability of the two events happening. As 12 cards are face cards, 26 cards are red, and 6 cards are a face card and a red card, the number of cards that are face cards or red cards is 12 + 26 – 6 = 32, so P(face card or red) = 32 out of 52.
In mathematical notation: P(A or B) = P(A) + P(B) – P(A and B)
To find the probability of an event not happening, we subtract the probability of it happening from 1. If the probability of getting on odd number when rolling a biased dice is 3 out of 4 or 0.75, then the probability of getting an even number (i.e. not getting an odd number) is 1 – 0.75 = 0.25.
Independent events are events where the outcome of one event does not affect the outcome of the other. For independent events P(A and B)=P(A) x P(B). When considering compound events, we often represent the different outcomes on a probability tree diagram to help us visualise the options. This is also useful when we find probabilities of dependent events.
Looking forward, students can then progress to additional probability worksheets, for example the combined events probability worksheet, the conditional probability worksheet, or the describing probabilities worksheet.

For more teaching and learning support on probability our GCSE maths lessons provide step by step support for all GCSE maths concepts.
Do you have students who need additional support?
With Third Space Learning's secondary maths tutoring programmes, students in Year 7-11 receive regular one to one maths tutoring to address gaps, build confidence and boost progress.
"My confidence in the tutoring is high. We've had some phenomenal results. I even had one girl get a Grade 8 this year; she came to every tutoring session."
Stacey Atkins, Maths Director, Outwood Grange Academies Trust