High Impact Tutoring Built By Math Experts
Personalized standards-aligned one-on-one math tutoring for schools and districts
In order to access this I need to be confident with:
Percent to decimal Substitution Percent change Simple interest Calculator skillsCompound interest formula
Here you will learn about the compound interest formula, including what it is and how to use it.
Students will first learn about the compound interest formula as part of algebra in high school.
What is the compound interest formula?
The compound interest formula is calculated on the principal (original) amount and the interest already accumulated on previous periods.
Take, for example, the amount of money in a savings account.
If you put \$ 100 in an account with an annual interest rate of 10 \%, the value of the money in the account will increase by 10 \% in year one; the new amount will be \$ 110 or 110 \% of the original.
If a further 10 \% was taken for year two, the new amount would be \$ 121 or 121 \% of the original value, since you are finding 10 \% of \$ 110.
Compound interest formula
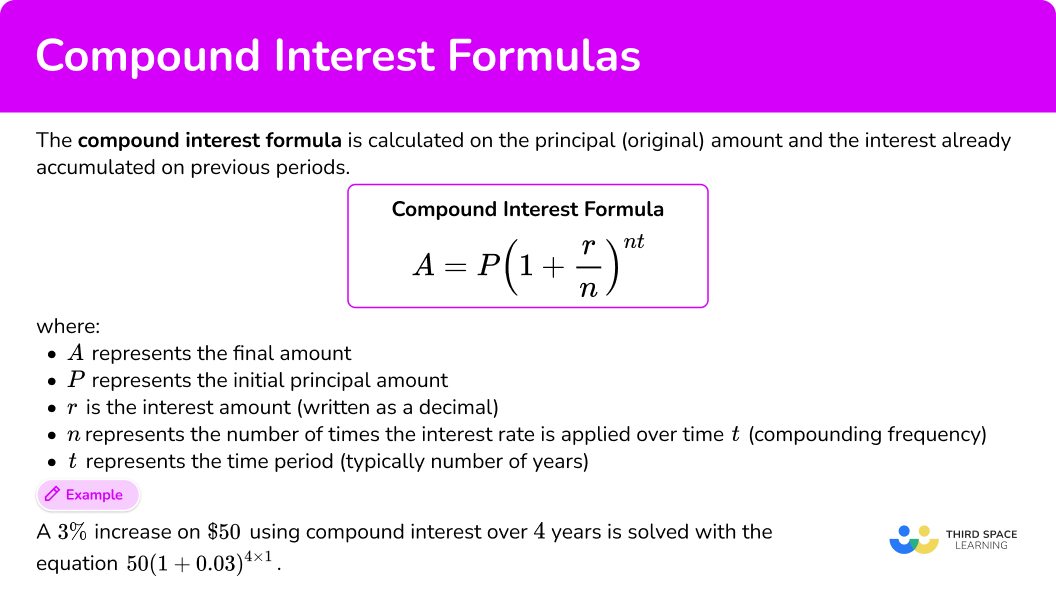
Compound interest is interest calculated on top of the original amount including any interest accumulated so far. The compound interest formula is:
A=P\left(1+\cfrac{r}{n}\right)^{nt}
where:
- A represents the final amount
- P represents the initial principal amount
- r is the interest amount (written as a decimal)
- n represents the number of times the interest rate is applied over time t (compounding frequency)
- t represents the time period (typically number of years)
For example,
Let’s calculate a 3 \% increase on an amount P using compound interest over 4 years. The interest amount written as a decimal is 0.03.
So, P \times 0.03 is the value of the interest only. Add this value to P to find the new amount after the first interval.
This is written as:
\begin{aligned}A&=P+P\times{0.03} \\\\ &=P(1+0.03) \\\\ &=P\times{1.03} \end{aligned}This is the value after the first interval.
The second interval is the new amount, multiplied by a further 1.03 and so on for the amount of intervals. This can be expressed using a table.
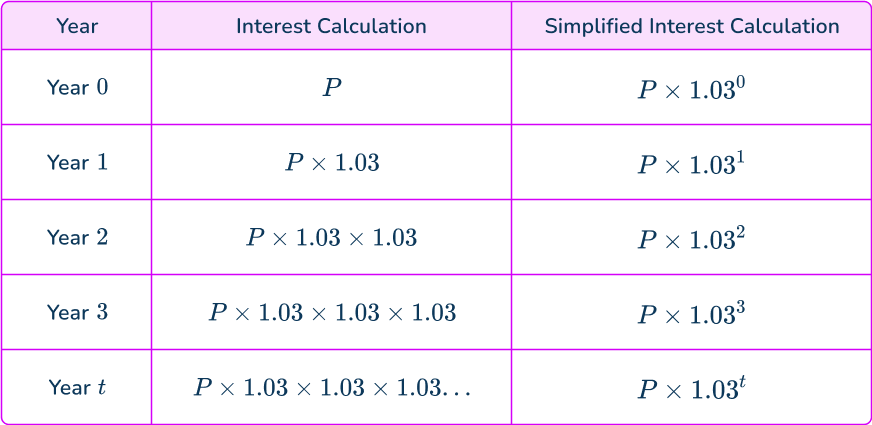
After t years, the principal amount P is multiplied by the percentage change for t times. This is why the power is expressed as t.
So for this example, multiplying P by 1.03^{4} would calculate an increase of 3 \% compound interest for 4 years.
What is the compound interest formula?
Common Core State Standards
How does this relate to high school math?
- Algebra – Seeing Structure in Expressions (HS.A.SSE.B.3c)
Use the properties of exponents to transform expressions for exponential functions. For example, the expression 1.151t can be rewritten as (1.15/12)12t\approx{1.01212t} to reveal the approximate equivalent monthly interest rate if the annual rate is 15 \%.
![[FREE] Percents Check for Understanding (Grade 6 to 7)](https://thirdspacelearning.com/wp-content/uploads/2023/07/Percents-check-for-understanding-quiz-listing-image-.png)
[FREE] Percents Check for Understanding (Grade 6 to 7)
![[FREE] Percents Check for Understanding (Grade 6 to 7)](https://thirdspacelearning.com/wp-content/uploads/2023/07/Percents-check-for-understanding-quiz-listing-image-.png)
Use this quiz to check your grade 6 to 7 students’ understanding of percents. 10+ questions with answers covering a range of 6th and 7th grade percent topics to identify areas of strength and support!
DOWNLOAD FREE![[FREE] Percents Check for Understanding (Grade 6 to 7)](https://thirdspacelearning.com/wp-content/uploads/2023/07/Percents-check-for-understanding-quiz-listing-image-.png)
[FREE] Percents Check for Understanding (Grade 6 to 7)
![[FREE] Percents Check for Understanding (Grade 6 to 7)](https://thirdspacelearning.com/wp-content/uploads/2023/07/Percents-check-for-understanding-quiz-listing-image-.png)
Use this quiz to check your grade 6 to 7 students’ understanding of percents. 10+ questions with answers covering a range of 6th and 7th grade percent topics to identify areas of strength and support!
DOWNLOAD FREEHow to use the compound interest formula
In order to use the compound interest formula:
- State the formula and the value of each variable.
- Substitute the values into the formula.
- Solve the equation.
Compound interest examples
Example 1: compound interest – percentage increase
\$ 8,500 is invested for 5 years at 0.3 \% per year compound interest. What is the value of the investment after this time?
- State the formula and the value of each variable.
Here we use the formula A=P\left(1+\cfrac{r}{n}\right)^{nt} with:
- P=8,500
- r=0.003
- n=1
- t=5
2Substitute the values into the formula.
Substituting these values into the compound interest formula A=P\left(1+\cfrac{r}{n}\right)^{nt}, you get:
A=8500(1+\cfrac{0.003}{1})^{1\times{5}}3Solve the equation.
\begin{aligned}A&=8500(1+0.003)^5 \\\\ &=8500\times1.003^5 \\\\ &=8500\times1.01509027 \\\\ &=\$8628.27\text{ (2dp)} \end{aligned}Example 2: compound interest – percentage decrease
A vacuum cleaner is bought for \$ 399 and loses 22 \% of its value per year compound interest. What is the value of the vacuum cleaner after 2 years?
State the formula and the value of each variable.
Here we use the formula A=P\left(1+\cfrac{r}{n}\right)^{nt} with:
- P=399
- r=- \, 0.22
- n=1
- t=2
Substitute the values into the formula.
Substituting these values into the compound interest formula A=P\left(1+\cfrac{r}{n}\right)^{nt}, you get:
A=399\left(1-\cfrac{0.22}{1}\right)^{1\times{2}}
Solve the equation.
Example 3: compound interest – compounding periods
A house is valued at \$ 150,000. On average, the house price increases by 2.4 \% monthly compounding over 2.5 years. What is the future value of the house after this time?
State the formula and the value of each variable.
Here we use the formula A=P\left(1+\cfrac{r}{n}\right)^{nt} with:
- P=150,000
- r=0.024
- n=12
- t=2.5
Substitute the values into the formula.
Substituting these values into the compound interest formula A=P\left(1+\cfrac{r}{n}\right)^{nt}, you get:
A=150000\left(1+\cfrac{0.024}{12}\right)^{12\times{2.5}}
Notice that the exponent is now 12 \times 2.5 as the number of months over 2.5 years =12\times{2.5}.
Solve the equation.
Example 4: compound interest – different percentages
A painting is valued at \$ 2950. On average, the value increases by 0.8 \% per year for 7 years compound interest. After this time, the rate of interest is 0.3 \% for a further 3 years. What is the value of the antique after this time?
State the formula and the value of each variable.
Here we use the formula A=P\left(1+r_{1}\right)^{t_{1}}\left(1+r_{2}\right)^{t_{2}} as the time scale n=1 with:
- P=2950
- r_{1}=0.008
- t_{1}=7
- r_{2}=0.003
- t_{2}=3
Substitute the values into the formula.
Substituting these values into the compound interest formula A=P(1+r_1)^{t_1}(1+r_2)^{t_2}, you get:
A=2,950(1+0.008)^{7}(1+0.003)^{3}
Solve the equation.
Example 5: compound interest – writing an answer in standard form
Bacteria reproduce at a rate of 3 \% per hour compound interest. If there are 1.2\times{10^7} bacteria in a petri dish at hour 0, how many bacteria are in the petri dish after 4 hours?
State the formula and the value of each variable.
Use the formula A=P(1+r)^{t} as the time scale n=1 with:
- P=1.2\times{10^7}
- r=0.03
- t=4
Substitute the values into the formula.
Substituting these values into the compound interest formula A=P(1+r)^{t}, you get:
A=(1.2\times{10}^{7})(1+0.03)^{4}
Solve the equation.
Example 6: compound interest – best buy
Two offers exist for the same car.
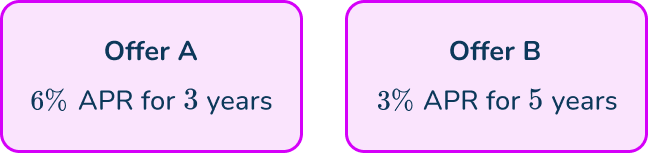
A car has a value of \$ 7999. Which offers a better value over the period of time stated?
State the formula and the value of each variable.
Here you are calculating compound interest with the formula A=P(1+r)^{t} twice to compare each offer:

Substitute the values into the formula.
Substituting these values into the simple interest formula A=P(1+r)^{t}, you get:
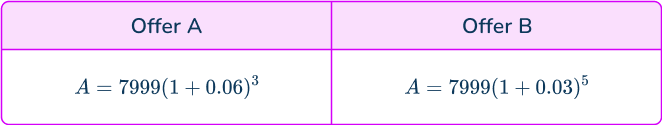
Solve the equation.

The best offer is Offer B, because the total amount paid is less.
Teaching tips for compound interest formula
- Introduce the topic through real-world examples, including examples that do not involve money. Start off with examples that have compounding frequency of 1 and then work up to more complex problems.
- Be mindful of specific vocabulary words that could keep students from understanding the context, such as ‘semi-annually’, ‘withdrawls’, ‘principal investment’, ‘time interval’ or others that often appear on worksheets.
Easy mistakes to make
- Applying the incorrect formula to the question
This is a very common mistake where the simple interest on an amount is calculated instead of using the compound interest formula.
- Incorrect percent change due to different time scales
Here is example 3 with this misconception:
A house is valued at \$ 150,000. On average, the house price increases by 2.4 \% per month over a period of 2.5 years compound interest. What is the value of the house after this time?
Here we use the formula A=P(1+\cfrac{r}{n})^{nt} with:
- P=150,000
- r=0.024
- n=1
- t=2.5
\begin{aligned} A&=150000(1.024)^{2.5} \\\\ &=\$159,162.65 \end{aligned}
Although the answer here looks reasonable, the 2.4 \% interest is per month not per year, which is what has been calculated.
- Using the percent as the value for \textbf{r}
The value for r is the percent divided by 100, so it is in decimal form.
Forgetting to convert to a decimal, leads to the incorrect answer.
For example,
When using compound interest to increase \$ 100 by 2 \% for 5 years, this calculation is made:
\begin{aligned}A&=100(1+2)^{5} \\\\ &=100\times243 \\\\ &=\$24,300 \end{aligned}
- Not considering if the value is increasing or decreasing
If a value is depreciating (going down), the value of r is negative. If a value is increasing (going up), the value of r is positive. Confusing this leads to an incorrect answer.
Related percent lessons
Compound interest practice questions
1. A bar of soap decreases in weight every time it is used by 3 \%. If the bar of soap weighs 50 \, g at the start of the week, and it is used 45 times, how much does it now weigh?




You can use the compound interest formula, since the soap always loses 3 \% of its weight, which is the compound interest rate.
50\times(1-0.03)^{45}=12.7 (rounded to the nearest tenth)
2. The Amazon rainforest covers an area of 5.5\mathrm{~million~km}^2 in 2021. If the rate of deforestation is 0.2 \%, what is the expected area of the rainforest in 2030?




You can use the compound interest formula, since the rate of deforestation is always 0.2 \%. Deforestation is the loss of trees, so r is negative and from 2021 to 2030 is 9 years.
5,500,000\times(1-0.002)^{9}=5,401,788 (rounded to the nearest whole)
3. Account A has an annual percentage yield of 2.5 \% compound interest. Account B has an interest rate of 0.2 \% per month compound interest.
If the initial investment is \$ 3,000, how much more money has Account B accumulated than Account A in interest after 40 months?




40 months =3 years and 4 months
3,000 \times(1+0.025)^{3}-3000=230.67 (rounded to the nearest hundredth)
Account A has accumulated \$ 230.67 in interest after 3 years ( 4 months not included as paid annually).
3,000\times(1+0.002)^{40}-3000=249.60 (rounded to the nearest hundredth)
After 40 months Account B has accumulated \$ 249.60 in interest.
\$ 249.60-\$ 230.67=\$ 18.93
4. A bucket is leaking water at a rate of 17 \% per hour, compound interest. How many hours would it take for the bucket to be less than half full?
2 hours

3 hours

4 hours

5 hours

Let’s use b for the amount of water in the bucket.
After 1 hour the bucket is 83 \% full.
b\times(1-0.17)^{1}=0.83b
After 2 hours the bucket is 68.89 \% full.
b\times(1-0.17)^{2}=0.6889b
After 3 hours the bucket is 57.18 \% full.
b\times(1-0.17)^{3}=0.5718b\text{ (4dp)}
After 4 hours the bucket is 47.46 \% full. This is less than half.
b\times(1-0.17)^{4}=0.4746b
5. A battery loses its charge at a rate of 5 \% every 2 hours, compound interest. If a battery starts fully charged, what percentage charge is left after 8 hours? Write your answer to the nearest hundredth of a percent.




Let’s use c for the amount of charge in the battery.
After 2 hours the battery is 95 \% full. In this case t=1, factoring in that each time period is 2 hours.
c\times(1-0.05)^{1}=0.95c
After 4 hours, the battery is 90.25 \% full. In this case t=2, because 4 is 2 time periods of 2 hours.
c\times(1-0.05)^{2}=0.9025c
After 6 hours, the battery is 85.74 \% full. In this case t=3, because 6 is 3 time periods of 2 hours.
c\times(1-0.05)^{3}=0.8574c\text{ (4dp)}
After 8 hours the battery is 81.45 \% full. In this case t=4, because 8 is 4 time periods of 2 hours.
c\times(1-0.05)^{4}=0.8145c\text{ (4dp)}
6. The amount of water in a pond changes throughout the year. For 6 months of the year, the water level increases by an average of 1 \% per month.
For the next 6 months of the year, the water level drops by 0.7 \% per month. If the pond contains 400 \, L at the beginning of the year, how much water is in the pond at the end of the first year?




The pond starts with 400 \, L of water. We are dealing with two 6 month time periods with a different multiplier for each one.
The amount of water is given by 400\times(1+0.01)^{6}\times(1-0.007)^{6}=407.08 \, L
Compound interest formula FAQs
A similar formula that calculates the total monthly interest paid, given the principal amount, interest rate and time period. The value for n is 12, since there are 12 months in a year.
P \cdot\left(1+\cfrac{r}{12}\right)^{12 t}-P
A similar formula that calculates the total daily interest paid, given the principal amount, interest rate and time period. The value for n is 365, since there are 365 days in a year.
P\cdot\left(1+\cfrac{r}{365}\right)^{365t}-P
The next lessons are
- Compound measures
- Types of data
- Averages and ranges
Still stuck?
At Third Space Learning, we specialize in helping teachers and school leaders to provide personalized math support for more of their students through high-quality, online one-on-one math tutoring delivered by subject experts.
Each week, our tutors support thousands of students who are at risk of not meeting their grade-level expectations, and help accelerate their progress and boost their confidence.

Find out how we can help your students achieve success with our math tutoring programs.
[FREE] Common Core Practice Tests (3rd to 8th Grade)
Prepare for math tests in your state with these 3rd Grade to 8th Grade practice assessments for Common Core and state equivalents.
Get your 6 multiple choice practice tests with detailed answers to support test prep, created by US math teachers for US math teachers!