Percent Increase - Math Steps, Examples & Questions

High Impact Tutoring Built By Math Experts

Personalized standards-aligned one-on-one math tutoring for schools and districts

Request a demo

In order to access this I need to be confident with:

Simplifying fractions Percent to decimal Percent to fraction

Math resources Ratio and proportion Percent

Percent increase

Here you will learn about percent increase, including how to increase a value by a given percentage, use multipliers to calculate percentage increase and work out percentage change.

Students will first learn about percent increase as part of ratios and proportions in the 7th grade.

What is percent increase?

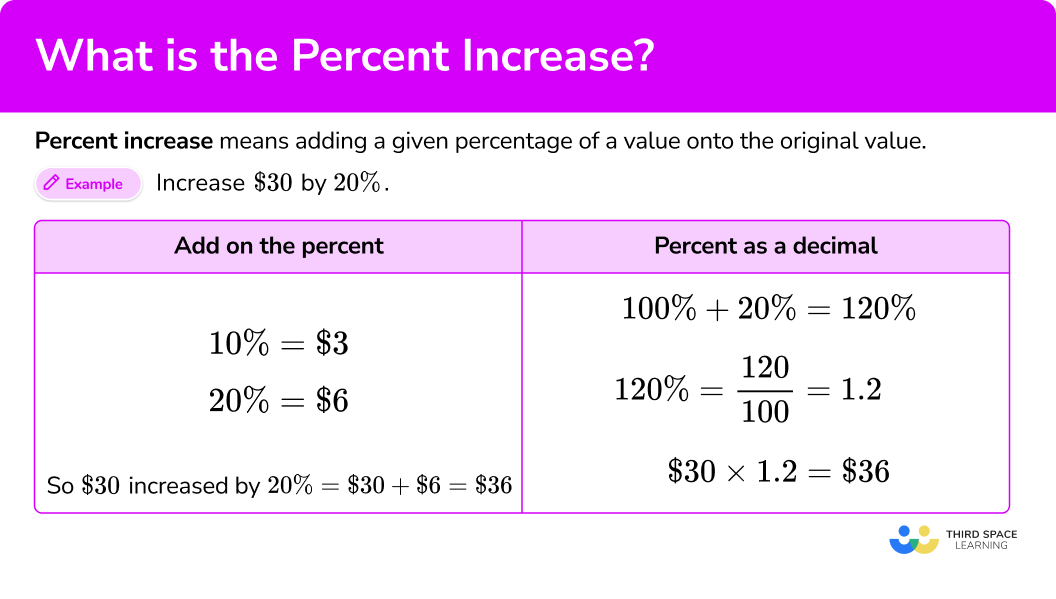
Percent increase means adding a given percentage of a value onto the original value. To do this, you can either calculate the given percentage of the value and then add it on to the original value or use a percent as a decimal.

For example,

Increase $\$30$ by $20\%.$

Add on the percent	Percent as a decimal
$\begin{aligned} & 10 \%=\$ 3 \\\\ & 20 \%=\$6 \end{aligned}$ So $\$30$ increased by $20\% = \$ 30+\$ 6=\$ 36.$	$\begin{aligned} & 100 \%+20 \%=120 \% \\\\ & 120 \%=\frac{120}{100}=1.2 \\\\ & \$ 30 \times 1.2=\$36 \end{aligned}$

When given two values, you can calculate the percentage difference or percentage change. When the value goes up, this may also be called percent gain, percent profit, or a markup.

You can calculate percent change using the percentage change formula:

$\text { Percentage change }=\frac{\text {amount of change }}{\text { original }} \times 100$

Percent gain

Percent gain is when the percent increases or gets bigger, between a starting percent and the final percent.

For example,

At the beginning of the month, you had $\$50$ in your savings account, and by the end of the month, you had $\$75.$ Work out the percent increase.

$\text { Percent increase }=\cfrac{75-50}{50} \times 100=50 \%$

Percent Profit

Percent profit is when a store or person selling a good or a service wants to calculate the difference between the cost price and the selling price and represent it as a percent.

For example,

A school store buys pencils for $\$0.80$ and sells them to the students for $\$1.00.$ Work out the percent profit.

$\text { Percent profit }=\cfrac{1-0.80}{0.80} \times 100=25 \%$

Markups

Markups are the increased price of a good. A company may markup an item that is in high demand and people are willing to pay more money for it.

For example, if a new makeup item is in high demand, a store may markup the price by a given percent due to the shortage of the item, or overall high demand.

What is percent increase?

[FREE] Percents Worksheet (Grade 6 to 7)

Use this quiz to check your grade 6 to 7 students’ understanding of percents. 10+ questions with answers covering a range of 6th and 7th grade percent topics to identify areas of strength and support!

DOWNLOAD FREE

[FREE] Percents Worksheet (Grade 6 to 7)

DOWNLOAD FREE

Common Core State Standards

How does this relate to 7th grade math?

Grade 7: Ratios and Proportional Relationships (7.RP.3)
Use proportional relationships to solve multistep ratio and percent problems. Examples: simple interest, tax, markups and markdowns, gratuities and commissions, fees, percent increase and decrease, percent error.

How to increase a value by a percent

In order to increase a value by a given percent:

Calculate the given percent of the value.
Add the calculated percent to the original number.

Percent increase examples

Example 1: percent increase in value

Kalli purchased a collector’s baseball card for $\$50$ in $2021.$ In $2022,$ the value increased by $40\%.$ What is the value of the baseball card now?

Calculate the given percent of the value.

You will first need to solve for $40\%$ of $\$50.$

The easiest way is to calculate $10\%$ (by dividing by $10$ ) and then multiply by $4$ to get $40\%.$

$10\%$ of $\$50 = \$5.$

$5 \times 4=20$

$40\%$ of $\$50 = \$20.$

2Add the calculated percent to the original number.

Add $\$20$ on to the original value of $\$50.$

$\$ 50+\$ 20=\$ 70$

The final answer is $\$70.$

The value of the baseball card increased to $\$70.$

Example 2: percent increase

Increase $400$ by $60\%.$

Calculate the given percent of the value.

You will first calculate $60\%$ of $400.$

The easiest way is to calculate $10\%$ (by dividing by $10$ ) and then multiply by $6$ to get $60\%.$

$10\%$ of $400 = 40.$

$40 \times 6=240$

$60\%$ of $400 = 240.$

Add the calculated percent to the original number.

Add $240$ on to the original value of $400.$

$400 + 240 = 640$

The final answer is $640.$

$400$ increased by $60\%$ is $640.$

Example 3: percent increase

Increase $350 \, m$ by $30\%.$

Calculate the given percent of the value.

You will first calculate $30\%$ of $350.$

The easiest way to calculate $30\%$ is to calculate $10\%$ (by dividing by $10$ ) and then multiply by $3$ to get $30\%.$

$10\%$ of $350 = 35.$

$35 \times 3=105$

$30\%$ of $350 = 105.$

Add the calculated percent to the original number.

Add $105 \, m$ on to the original value.

$350 \, m+105 \, m=455 \, m$

The final answer is $455 \, m.$

$350 \, m$ increased by $30\%$ is $455 \, m.$

Example 4: percent increase in value

The price of Katrina’s train ticket last year was $\$75.$ This year it has increased by $15\%.$ Find the price of Katrina’s train ticket this year.

Calculate the given percent of the value.

You will first need to find $15\%$ of $\$75.$

$10\%$ of $\$75 = \$7.50.$

$7.50 \div 2=3.75$

$5\%$ of $\$75 = \$3.75.$

$\$ 7.50+3.75=\$ 11.25$

$15\%$ of $\$75$ is $\$11.25.$

Add the calculated percent to the original number.

Add on $\$11.25$ to the original value.

$\$ 75+\$ 11.25=\$ 86.25$

The final answer is $\$86.25.$

Katrina’s train ticket increased by $15\%$ is $\$86.25.$

Percent increase using a percent as a decimal

In order to increase a value by using a percent as a decimal:

Add the percent you are increasing by and $\bf{100\%}$ .
Convert the percent to a decimal.
Multiply the original amount by the decimal.

Example 5: percent as a decimal

Increase $3,200 \, ml$ by $24\%.$

Add the percentage you are increasing by and $\bf{100\%}$ .

Here you are calculating a $24$ percent increase, so add $24\%$ on to $100\%.$

$100 \%+24 \%=124 \%$

Convert the percentage to a decimal.

To do this, you divide the percentage by $100.$

$124 \% \div 100=1.24$

$124\% = 1.24$

Multiply the original amount by the decimal.

Multiply $3,200$ by $1.24.$

$3,200\times 1.24=3,968 \, ml$

The final answer is $3,968 \, ml.$

$3,200 \, ml$ increased by $24\%$ is $3,968 \, ml.$

Example 6: percent as a decimal

In $2015,$ Rachel’s monthly rent was $\$620.$ Over the course of five years, Rachel’s monthly rent increased by $12.5\%.$ Find Rachel’s monthly rent at the end of the five years.

Add the percentage you are increasing by and $\bf{100\%}$ .

This is a $12.5$ percent increase, so you add $12.5\%$ onto $100\%.$

$100 \%+12.5 \%=112.5 \%$

Convert the percentage to a decimal.

$112.5 \div 100=1.125$

$112.5\% = 1.125$

Multiply the original amount by the decimal.

Multiply $\$620$ by $1.125.$

$620\times 1.125=\$697.50$

Remember to always write money using two decimal places.

Rachel’s rent increased from $\$620$ to $\$697.50$ over $5$ years.

Calculating percent increase

In order to calculate the percent increase after a percent change:

Find the amount of change by subtracting the original number from the new number.
Plug numbers into the percent change formula.
$\text { Percentage change }=\cfrac{\text { Change }}{\text { Original }} \times 100$
Simplify the fraction, if necessary, using equivalent fractions.
Convert the fraction to a decimal.
Calculate percent change.

Example 7: calculating percent change

The weight of a lamb has increased from $7 \, kg$ to $13 \, kg.$ Find the percent increase in the lamb’s weight.

Find the amount of change by subtracting the original number from the new number.

The weight of the lamb has changed from $7 \, kg$ to $13 \, kg.$ You will subtract the original weight from the new weight.

$13-7=6 \mathrm{~kg}$

Plug numbers into the percent change formula.

Apply the percent change formula. The change is $6 \, kg$ and the original amount is $7 \, kg.$

$\begin{aligned} & \text { Percent change }=\cfrac{\text { Change }}{\text { Original }} \times 100 \\\\ & \text { Percent change }=\cfrac{6}{7} \times 100 \end{aligned}$

Simplify the fraction, if necessary, using equivalent fractions.

$\cfrac{6}{7} \,$ does not need to be simplified.

$\text { Percent change }=\cfrac{6}{7} \times 100$

Convert the fraction to a decimal.

$\cfrac{6}{7}=85.71$

$\text{Percent change } =0.86 \times 100$

You can round this decimal to $0.86$ to solve.

Calculate percent change.

$0.86 \times 100=86$

$\text{Percent change } = 86\%$

The lamb’s weight has increased by $86\%.$

Example 8: calculating percentage profit

Lucy buys an antique table for $\$150$ and sells it for $\$185.$ Calculate Lucy’s percent profit.

Find the amount of change by subtracting the original number from the new number.

Work out the change in value by subtracting the original price from the new price:

$\$ 185-\$ 150=\$ 35$

Plug numbers into the percent change formula.

Apply the percent change formula. The change is $\$35$ and the original price is $\$150.$

$\text { Percent change }=\cfrac{35}{150} \times 100$

Simplify the fraction, if necessary, using equivalent fractions.

$\cfrac{35}{150} \,$ can be simplified to $\, \cfrac{7}{30}.$

$\text { Percent change }=\cfrac{7}{30} \times 100$

Convert the fraction to a decimal.

$\cfrac{7}{30}=23.333\ldots$

$\text { Percent change }=0.23 \times 100$

This is a repeating decimal, but you can round this decimal to $0.23$ to solve.

Calculate percent change.

$0.23 \times 100=23$

$\text{Percent change }=23 \%$

Lucy’s percentage profit is $23\%.$

Teaching tips for percent increase

When calculating percent change, sometimes the numbers can be quite large. Students may use a calculator, or a percentage change calculator, to assist them with solving these problems.

Percent change can be calculated using Microsoft Excel. For students who are proficient using Excel, this could be an additional teaching point while discussing percent change.

Easy mistakes to make

Believing that percentages cannot be greater than $\bf{100\%}$
For percent increase using a percent as a decimal, you encounter a lot of percentages greater than $100\%$ as you are adding on to the original $100\%.$

Incorrectly converting percentages to decimals
The most common mistakes are with single digit percentages (for example, $5\%$ ), multiples of $10$ (for example, $40\%$ ), and decimal percentages (for example, $3.2\%$ ).
Remember to divide the percentage by $100$ to find the decimal.
For example, $5\%=0.05, \, 40\%=0.4, \, 3.2\%=0.032, \, 106.5\%=1.065$

Using an incorrect value for the denominator in the percentage increase formula
Using the new value instead of the original value for the denominator when calculating percentage change.

Percent increase practice questions

Calculate the percent increase that has occurred in each of these questions.

\$540

\$104

\$624

\$640

You will first calculate $20\%$ of $\$520.$

$10\%$ of $\$520 = \$52.$

$52 \times 2=104$

$20\%$ of $\$520 = \$104.$

$20\%$ of $520$ is $104.$

This can be added to the original amount to find the quantity after the increase.

$\$ 104+\$ 520=\$ 624$

3,463 \, m

4,760 \, m

1,470.6 \, m

3,563 \, m

You will first calculate $40\%$ of $3,400.$

$10\%$ of $3,400 = 340.$

$340 \times 4=1,360$

$40\%$ of $3,400 = 1,360$

$40\%$ of $3,400$ is $1,360.$

This can be added to the original amount to find the quantity after the increase.

$3,400+1,360=4,760$

$3,400 \, m$ increased by $40\%$ is $4,760.$

5.32

43.32

42

33.3

Add the percentage you are increasing by, $14\%,$ and $100.$

$100+14 =114$

Next, you will convert the percentage to a decimal by dividing.

$114 \div 100=1.14$

Then multiply the original amount by the decimal.

$38 \times 1.14=43.32$

$38$ increased by $14\%$ is $43.32.$

172.25 \, kg

819 \, kg

513.83 \, kg

676 \, kg

Add the percentage you are increasing by, $26\%,$ and $100.$

$100+26 =126$

Next, you will convert the percentage to a decimal by dividing.

$126 \div 100=1.26$

Then multiply the original amount by the decimal.

$650 \times 1.26=819$

$650 \, kg$ increased by $26\%$ is $819 \, kg.$

55\%

155 \%

220\%

110\%

First, you will calculate the change from $400 \, ml$ to $620 \, ml.$

$620-400=220$

The change is $220 \, ml,$ so you can now plug the numbers into the percent change formula.

$\text {Percent change }=\cfrac{220}{400} \times 100$

After simplifying the fraction to $\, \cfrac{55}{100} \, ,$ you would convert it to the decimal $0.55.$

$0.55 \times 100=55$

$\text {Percentage increase }=55 \%$

111\%

89 \%

11\%

136.4\%

First, you will calculate the change from $\$124,000$ to $\$137,640.$

$137,640-124,000=13,640$

The change is $\$13,640,$ so you can now plug the numbers into the percent change formula.

$\text {Percent change }=\cfrac{13,640}{124,000} \times 100$

After simplifying the fraction to $\, \cfrac{11}{100} \, ,$ you would convert it to the decimal $0.11.$

$0.11 \times 100=11$

$\text {Percentage increase }=11 \%$

Percent increase FAQs

Can finding the percent change give you a negative number for a percent?

If you are finding a percent decrease, or any percentage where the value is decreasing, the initial percent change calculation will result in a negative number. However, you will use the absolute value of the difference to calculate the overall percent change, which will result in a positive number.

What is a percent increase calculator?

It is an automated way for you to calculate the percentage increase of a given value. It is recommended that you use the calculator when dealing with large numbers and decimals.

The next lessons are

Compound measures
Converting fractions, decimals, and percentages
Ratio
Algebraic expressions
Simplifying fractions

Still stuck?

At Third Space Learning, we specialize in helping teachers and school leaders to provide personalized math support for more of their students through high-quality, online one-on-one math tutoring delivered by subject experts.

Each week, our tutors support thousands of students who are at risk of not meeting their grade-level expectations, and help accelerate their progress and boost their confidence.