Fluency, Reasoning and Problem Solving: What This Looks Like In Every Math Lesson

Fluency, reasoning and problem solving are central strands of mathematical competency, as recognized by the National Council of Teachers of Mathematics (NCTM) and the National Research Council’s report ‘Adding It Up’.

They are key components to the Standards of Mathematical Practice, standards that are interwoven into every mathematics lesson. Here we look at how these three approaches or elements of math can be interwoven in a child’s math education through elementary and middle school.

We look at what fluency, reasoning and problem solving are, how to teach them, and how to know how a child is progressing in each – as well as what to do when they’re not, and what to avoid.

The hope is that this blog will help elementary and middle school teachers think carefully about their practice and the pedagogical choices they make around the teaching of what the common core refers to as ‘mathematical practices’, and reasoning and problem solving in particular.

Before we can think about what this would look like in Common Core math examples and other state-specific math frameworks, we need to understand the background to these terms.

What is fluency in math?

Fluency in math is a fairly broad concept. The basics of mathematical fluency – as defined by the Common Core State Standards for math – involve knowing key mathematical skills and being able to carry them out flexibly, accurately and efficiently.

But true fluency in math (at least up to middle school) means being able to apply the same skill to multiple contexts, and being able to choose the most appropriate method for a particular task.

Fluency in math lessons means we teach the content using a range of representations, to ensure that all students understand and have sufficient time to practice what is taught.

Read more: How the best schools develop math fluency

What is reasoning in math?

Reasoning in math is the process of applying logical thinking to a situation to derive the correct math strategy for problem solving for a question, and using this method to develop and describe a solution.

Put more simply, mathematical reasoning is the bridge between fluency and problem solving. It allows students to use the former to accurately carry out the latter.

Read more: Developing math reasoning: the mathematical skills required and how to teach them.

What is problem solving in math?

It’s sometimes easier to start off with what problem solving is not. Problem solving is not necessarily just about answering word problems in math. If a child already has a readily available method to solve this sort of problem, problem solving has not occurred. Problem solving in math is finding a way to apply knowledge and skills you have to answer unfamiliar types of problems.

Read more:

• Math problem solving: strategies and resources for primary school teachers
• Effective Instructional Strategies

We are all problem solvers

First off, problem solving should not be seen as something that some students can do and some cannot. Every single person is born with an innate level of problem-solving ability.

Early on as a species on this planet, we solved problems like recognizing faces we know, protecting ourselves against other species, and as babies the problem of getting food (by crying relentlessly until we were fed).

All these scenarios are a form of what the evolutionary psychologist David Geary (1995) calls biologically primary knowledge. We have been solving these problems for millennia and they are so ingrained in our DNA that we learn them without any specific instruction.

Why then, if we have this innate ability, does actually teaching problem solving seem so hard?

Mathematical problem solving is a learned skill

As you might have guessed, the domain of mathematics is far from innate. Math doesn’t just happen to us; we need to learn it. It needs to be passed down from experts that have the knowledge to novices who do not.

This is what Geary calls biologically secondary knowledge. Solving problems (within the domain of math) is a mixture of both primary and secondary knowledge.

The issue is that problem solving in domains that are classified as biologically secondary knowledge (like math) can only be improved by practicing elements of that domain.

So there is no generic problem-solving skill that can be taught in isolation and transferred to other areas.

This will have important ramifications for pedagogical choices, which I will go into more detail about later on in this blog.

The educationalist Dylan Wiliam had this to say on the matter: ‘for…problem solving, the idea that students can learn these skills in one context and apply them in another is essentially wrong.’ (Wiliam, 2018) So what is the best method of teaching problem solving to elementary and middle school math students?

The answer is that we teach them plenty of domain specific biological secondary knowledge – in this case, math. Our ability to successfully problem solve requires us to have a deep understanding of content and fluency of facts and mathematical procedures.

Here is what cognitive psychologist Daniel Willingham (2010) has to say:

‘Data from the last thirty years leads to a conclusion that is not scientifically challengeable: thinking well requires knowing facts, and that’s true not simply because you need something to think about.

The very processes that teachers care about most—critical thinking processes such as reasoning and problem solving—are intimately intertwined with factual knowledge that is stored in long-term memory (not just found in the environment).’

Colin Foster (2019), a reader in Mathematics Education in the Mathematics Education Center at Loughborough University, UK, says, ‘I think of fluency and mathematical reasoning, not as ends in themselves, but as means to support students in the most important goal of all: solving problems.’

In that paper he produces this pyramid:

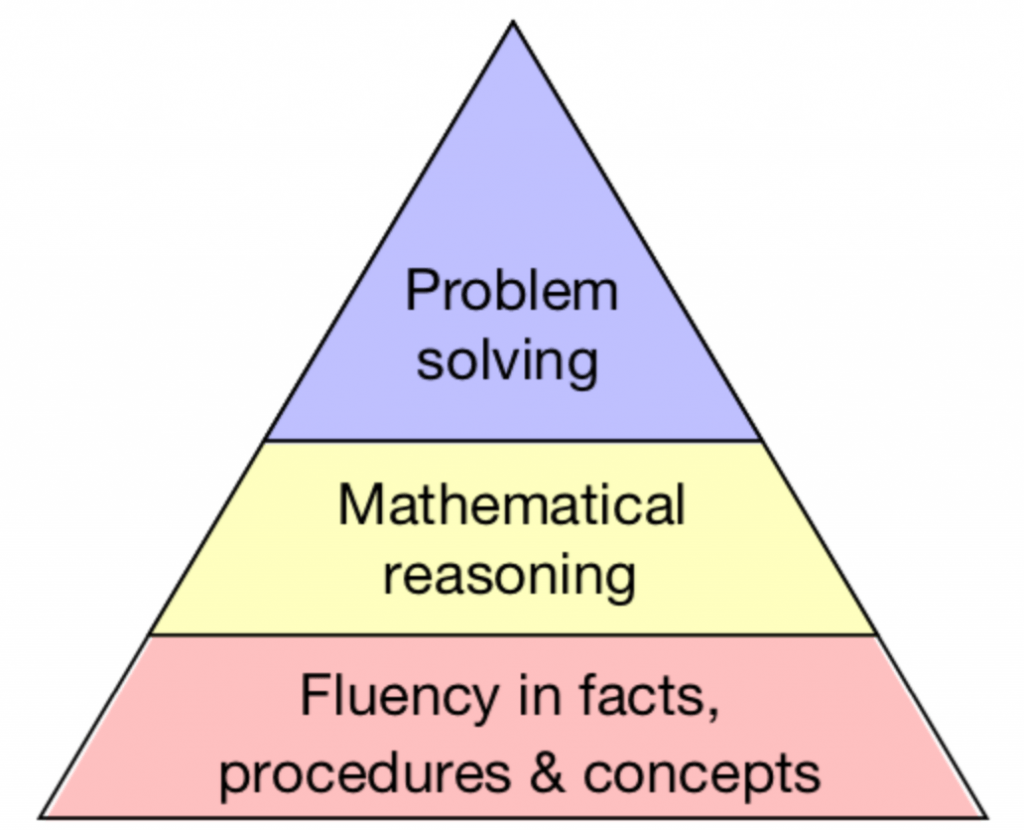
This is important for two reasons:

1)    It splits up reasoning skills and problem solving into two different entities

2)    It demonstrates that fluency is not something to be rushed through to get to the ‘problem solving’ stage but is rather the foundation of problem solving.

In my own work I adapt this model and turn it into a cone shape, as education seems to have a problem with pyramids and gross misinterpretation of them (think Bloom’s taxonomy).

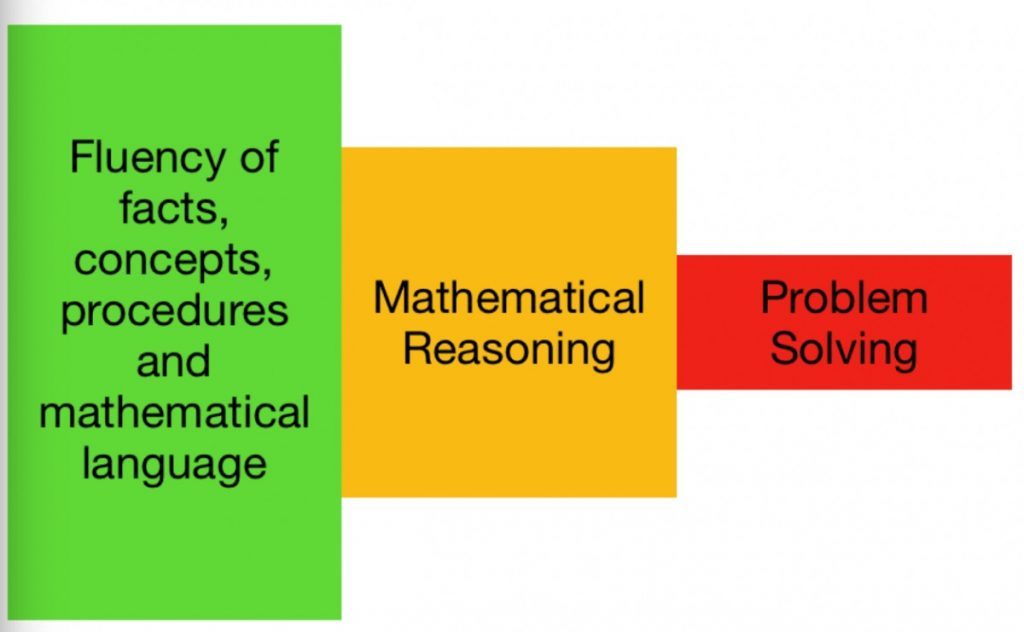
Notice how we need plenty of fluency of facts, concepts, procedures and mathematical language.

Having this fluency will help with improving logical reasoning skills, which will then lend themselves to solving mathematical problems – but only if it is truly learnt and there is systematic retrieval of this information carefully planned across the curriculum.

Performance vs learning: what to avoid when teaching fluency, reasoning, and problem solving

I mean to make no sweeping generalization here; this was my experience both at university when training and from working in schools.

At some point, schools become obsessed with the ridiculous notion of moving students through content at an accelerated rate. I have heard it used in all manner of educational contexts while training and being a teacher. ‘You will need to show ‘accelerated progress in math’ in this lesson,’ ‘School officials will be looking for ‘accelerated progress’ etc.

I have no doubt that all of this came from a good place and from those wanting the best possible outcomes – but it is misguided.

I remember being told that we needed to get students onto the problem solving questions as soon as possible to demonstrate this mystical ‘accelerated progress’.

This makes sense; you have a group of students and you have taken them from not knowing something to working out pretty sophisticated 2-step or multi-step word problems within an hour. How is that not ‘accelerated progress?’

This was a frequent feature of my lessons up until last academic year: teach a mathematical procedure; get the students to do about 10 of them in their books; mark these and if the majority were correct, model some reasoning/problem solving questions from the same content as the fluency content; give the students some reasoning and word problem questions and that was it.

I wondered if I was the only one who had been taught this while at university so I did a quick poll on Twitter and found that was not the case.

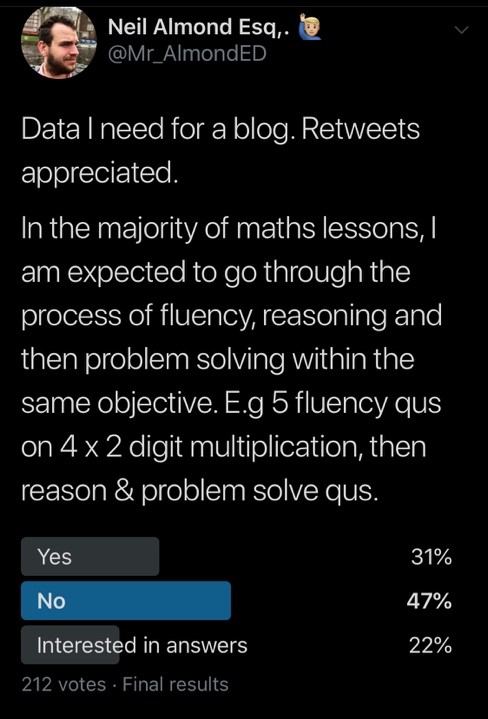
I know these numbers won’t be big enough for a representative sample but it still shows that others are familiar with this approach.

The issue with the lesson framework I mentioned above is that it does not take into account ‘performance vs learning.’

What IS ‘performance vs learning’?

The premise is that performance in a lesson is not a good proxy for learning.

Yes, those students were performing well after I had modeled a mathematical procedure for them, and managed to get questions correct.

But if problem solving depends on a deep knowledge of mathematics, this approach to lesson structure is going to be very ineffective.

As mentioned earlier, the reasoning and problem solving questions were based on the same math content as the fluency exercises, making it more likely that students would solve problems correctly whether they fully understood them or not.

Chances are that all they’d need to do is find the numbers in the questions and use the same method they used in the fluency section to get their answers (a process referred to as “number plucking”) – not exactly high level problem solving skills.

Teaching to “cover the curriculum” hinders development of strong problem solving skills.

This is one of my worries with ‘math mastery schemes’ that block content so that, in some circumstances, it is not looked at again until the following year (and with new objectives).

The pressure for teachers to ‘get through the curriculum’ results in many opportunities to revisit content being missed in the classroom.

Students are unintentionally forced to skip ahead in the fluency, reasoning, problem solving chain without proper consolidation of the earlier processes.

As David Didau (2019) puts it, ‘When novices face a problem for which they do not have a conveniently stored solution, they have to rely on the costlier means-end analysis.

This is likely to lead to cognitive overload because it involves trying to work through and hold in mind multiple possible solutions.

It’s a bit like trying to juggle five objects at once without previous practice. Solving problems is an inefficient way to get better at problem solving.’

Fluency and reasoning – Best practice in a lesson, a unit, and a semester

By now I hope you have realized that when it comes to problem solving, fluency is king. As such we should look to mastery math based teaching to ensure that the fluency that students need is there.

The answer to what fluency looks like will obviously depend on many factors, including the content being taught and the grade you find yourself teaching.

But we should not consider rushing them on to problem solving or logical reasoning in the early stages of this new content as it has not been learnt, only performed.

I would say that in the early stages of learning, content that requires the end goal of being fluent should take up the majority of lesson time – approximately 60%. The rest of the time should be spent rehearsing and retrieving other knowledge that is at risk of being forgotten about.

This blog on mental math strategies students should learn at each grade level is a good place to start when thinking about the core aspects of fluency that students should achieve.

Little and often is a good mantra when we think about fluency, particularly when revisiting the key mathematical skills of number bond fluency or multiplication fluency. So when it comes to what fluency could look like throughout the day, consider all the opportunities to get students practicing.

They could chant multiplication facts when transitioning. If a lesson in another subject has finished earlier than expected, use that time to quiz students on number bonds. Have fluency exercises as part of the morning work.

Read more: How to teach multiplication for instant recall

What about best practice over a longer period?

Thinking about what fluency could look like across a unit of work would again depend on the unit itself.

Look at this unit below from a popular scheme of work.

They recommend 20 days to cover 9 objectives. One of these specifically mentions problem solving so I will forget about that one at the moment – so that gives 8 objectives.

I would recommend that the fluency of this unit look something like this:

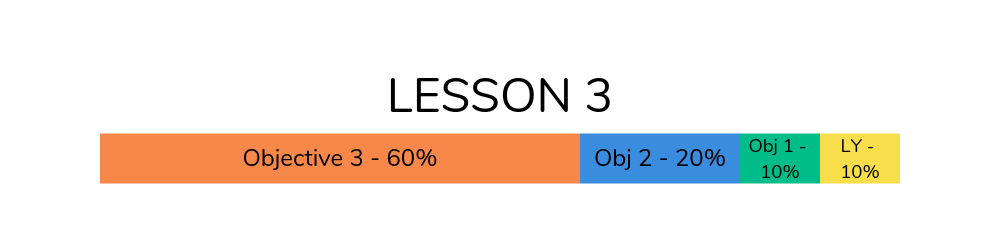
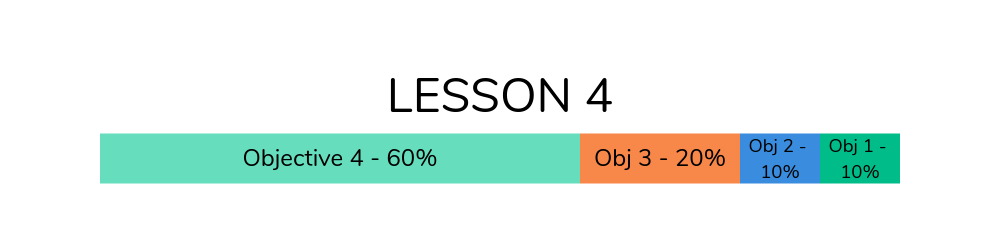
This type of structure is heavily borrowed from Mark McCourt’s phased learning idea from his book ‘Teaching for Mastery.’

This should not be seen as something set in stone; it would greatly depend on the needs of the class in front of you. But it gives an idea of what fluency could look like across a unit of lessons – though not necessarily all math lessons.

When we think about a semester, we can draw on similar ideas to the one above except that your lessons could also pull on content from previous units from that semester.

So lesson one may focus 60% on the new unit and 40% on what was learnt in the previous unit.

The structure could then follow a similar pattern to the one above.

Best practice for problem solving in a lesson, a unit, and a semester 

When an adult first learns something new, we cannot solve a problem with it straight away. We need to become familiar with the idea and practice before we can make connections, reason and problem solve with it.

The same is true for students. Indeed, it could take up to two years ‘between the mathematics a student can use in imitative exercises and that they have sufficiently absorbed and connected to use autonomously in non-routine problem solving.’ (Burkhardt, 2017).

Practice with facts that are secure

So when we plan for reasoning and problem solving, we need to be looking at content from 2 years ago to base these questions on.

You could get students in 3rd grade to solve complicated place value problems with the numbers they should know from 1st or 2nd grade. This would lessen the cognitive load, freeing up valuable working memory so they can actually focus on solving the problems using content they are familiar with.

Increase complexity gradually

Once they practice solving these types of problems, they can draw on this knowledge later when solving problems with more difficult numbers.

This is what Mark McCourt calls the ‘Behave’ phase. In his book he writes:

‘Many teachers find it an uncomfortable – perhaps even illogical – process to plan the ‘Behave’ phase as one that relates to much earlier learning rather than the new idea, but it is crucial to do so if we want to bring about optimal gains in learning, understanding and long term recall.’  (Mark McCourt, 2019)

This just shows the fallacy of ‘accelerated progress’; in the space of 20 minutes some teachers are taught to move students from fluency through to non-routine problem solving, or we are somehow not catering to the needs of the child.

When considering what problem solving lessons could look like, here’s an example structure based on the objectives above.

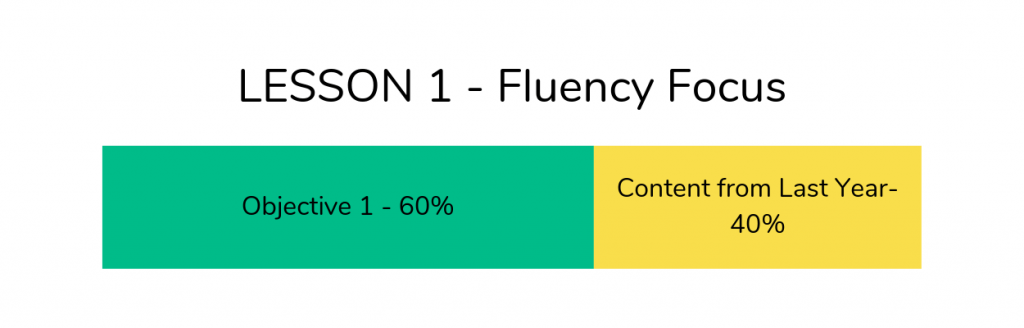
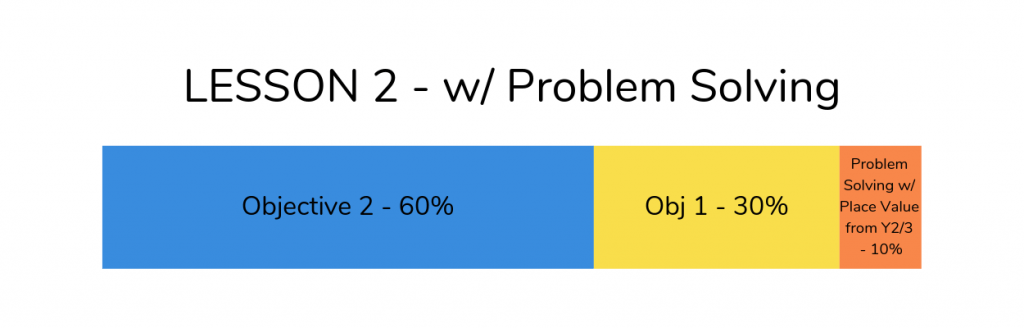
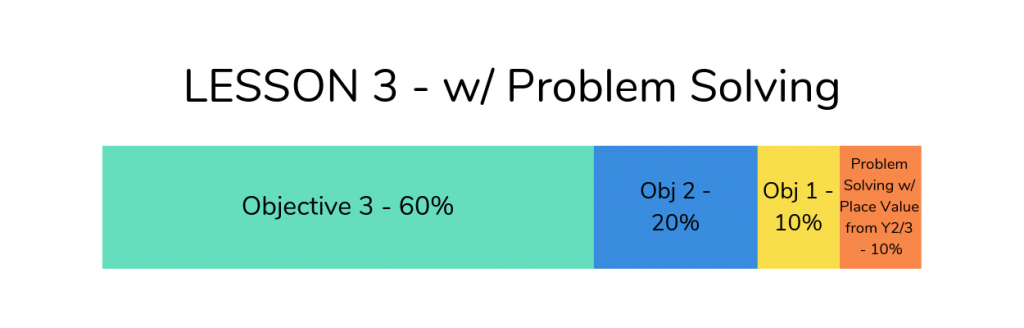
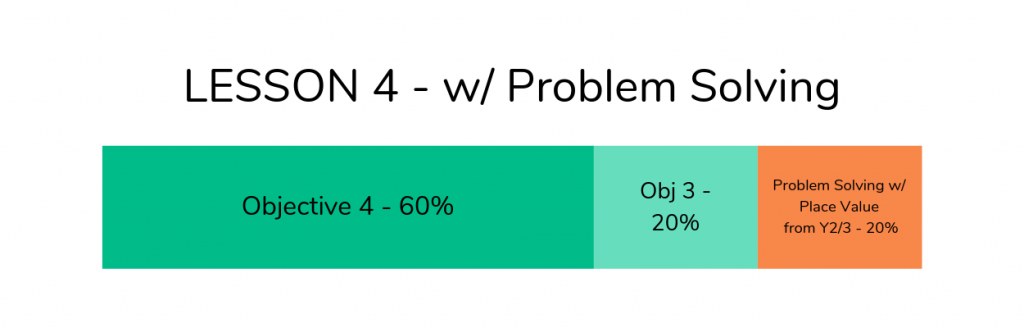
Fluency, Reasoning and Problem Solving should NOT be taught by rote 

It is important to reiterate that this is not something that should be set in stone. Key to getting the most out of this teaching for mastery approach is ensuring your students (across abilities) are interested and engaged in their work.

Depending on the previous attainment and abilities of the children in your class, you may find that a few have come across some of the mathematical ideas you have been teaching, and so they are able to problem solve effectively with these ideas.

Equally likely is encountering students on the opposite side of the spectrum, who may not have fully grasped the concept of place value and will need to go further back than 2 years and solve even simpler problems.

In order to have the greatest impact on class performance, you will have to account for these varying experiences in your lessons.

Read more: 

• Math Mastery Toolkit: A Practical Guide To Mastery Teaching And Learning
• Problem Solving and Reasoning Questions and Answers
• Get to Grips with Math Problem Solving For Elementary Students
• Mixed Ability Teaching for Mastery: Classroom How To
• 21 Math Challenges To Really Stretch Your More Able Students
• Why You Should Be Incorporating Stem Sentences Into Your Elementary Math Teaching

Do you have students who need extra support in math?
Give your students more opportunities to consolidate learning and practice skills through personalized math tutoring with their own dedicated online math tutor.

Each student receives differentiated instruction designed to close their individual learning gaps, and scaffolded learning ensures every student learns at the right pace. Lessons are aligned with your state’s standards and assessments, plus you’ll receive regular reports every step of the way.

Personalized one-on-one math tutoring programs are available for:
– 2nd grade tutoring
– 3rd grade tutoring
– 4th grade tutoring
– 5th grade tutoring
– 6th grade tutoring
– 7th grade tutoring
– 8th grade tutoring

Why not learn more about how it works?

The content in this article was originally written by primary school lead teacher Neil Almond and has since been revised and adapted for US schools by elementary math teacher Jaclyn Wassell.