How To Learn Math: 9 Strategies For Introducing New Concepts To Students

How to learn mathematics? I see the phrase ‘teaching for understanding’ quite a lot, and it always confuses me slightly.

For knowledge and skills gained in the math classroom to persist beyond high school (and to be useful in the future), students need to have a deep understanding of the concepts involved. This should be beyond just being able to replicate a method on an exam.

Obviously, within the current education system, there are constraints and external pressures to take into account.

Here, I take you through 9 key teaching strategies for introducing new concepts. These will enhance student understanding while increasing their ability to learn math in a way, so that the knowledge and skills gained persist beyond the next assessment!

Mathematics is a hierarchical subject, in that understanding new concepts depends on other underlying fundamental concepts. For example, in order to understand how multiplication works, students need to have a well-developed concept of addition and be able to appreciate the concept of repeated addition and the links to multiplication. 

The more complex the level of math, the more foundational blocks of understanding are needed. For example, fraction addition requires an understanding of:

• What a fraction is and what it represents;
• Equivalent fractions;
• Improper fractions and mixed numbers;
• General integer multiplication and number sense;
• Identifying multiples, including the lowest common multiples.

If one of these underlying concepts is underdeveloped (or missing), learners struggle far more to assimilate the new concept of fraction arithmetic with their existing knowledge; they are attempting to build the new concept up on wobbly foundations.  

If misconceptions exist in the foundation blocks, it is likely that these misconceptions will be compounded as students attempt to understand new concepts.

By the time our students reach middle school and high school level, there are many more of these interlinked concepts and many more opportunities for baked-in misconceptions.

Clearly, there is not enough syllabus time to re-teach every math topic from scratch, as if students have never experienced it before, so we need to find a happy medium that gives our students the opportunity to explore their prior math skills and knowledge and highlight issues.

---

2. Assess a starting point to establish realistic next steps

When beginning a new unit of work or topic, it can be useful to give students a quick, low-stakes assessment to judge their prior knowledge and work out a starting point.

Fraction addition example: establishing misconceptions

Taking my example of fraction addition, I might give them a few questions targeting some of the concepts above.

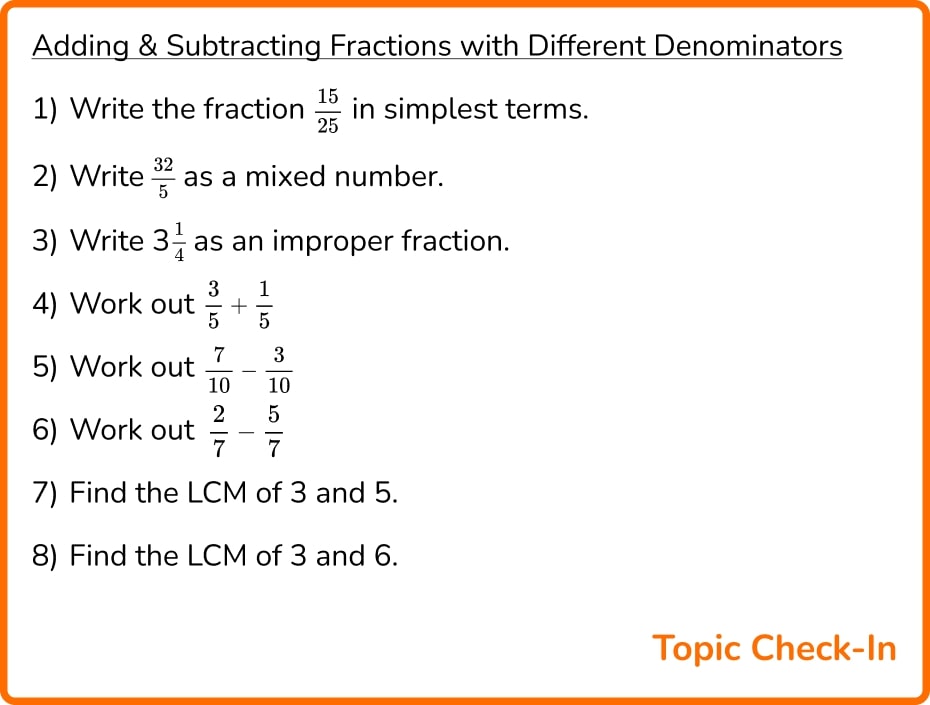
This does not have to be done as a written assessment; a mini-whiteboard quiz is just as valuable and is often a better way to get glimpses of general patterns.

If I was planning a unit on fraction addition, I’d be looking for things like:

• Are students using bar modeling or diagrams?
• Are they using a written (algorithmic) method?
• If they are, is it correct?
• Are errors popping up with basic arithmetic – does multiplication facts recall need work? 
• Are they simplifying their answers? (And doing it correctly!)
• Can they tackle mixed number problems using their preferred method – are there issues with improper and mixed number conversion that need to be tackled first?

Before teaching any new content, I’d then plan to go back and address any large-scale misconceptions or stumbling blocks.

In some circumstances, it may be more appropriate to target individual students or small groups with a very brief intervention. For example, by directing them to a personalized learning program or video tutorials to review the key concepts.

Third Space Learning’s one-to-one, online math tutoring uses diagnostic testing to gauge students’ understanding and identify gaps in learning. Using this information, the program is then personalized to address each individual student’s needs.

Sometimes, with the amount of curriculum content to get through, it can be difficult to feel confident about spending lesson time reviewing content they ‘should already know’, rather than teaching the new material.

However, ensuring that new concepts are built on more solid foundations using a spiral curriculum has two big advantages.

1. The new concept will often be learned more quickly, as students are able to slot the new ideas into a clear existing framework;
2. If the new concept is clearly understood, rather than surface-learned, it is more likely to move from the student’s short-term to long-term memory and stay there. This means that less re-teaching will be needed in the future.

---

3. Work within the constraints of working memory

Research from cognitive science suggests that direct instruction is generally more effective than discovery-based learning (see Kirschner, Sweller and Clark 2006 for a great discussion of these ideas). We need to be aware of cognitive overload and the relationships between working memory and long-term learning and memory. 

Fraction addition example: comparing working memory

This is one reason why good prior knowledge is important. Returning again to adding fractions, let’s look at two different students.

Student A

Student B

They understood equivalent fractions when it was taught the first time around. They have a good concept of multiples. They have good times table recall. They have a good understanding of the underlying mathematical concepts. The procedures for calculating equivalent fractions and simplifying fractions are well-organized in their long-term memory. The relevant multiplication facts are there for rapid recall when needed. When they listen to an explanation on fraction addition, their working memory can concentrate fully on the new bits; why a common denominator is important and how to actually add the two fractions.

They can’t really remember what an equivalent fraction is (and probably didn’t really understand it in the first place). They were absent when multiples were taught. They aren’t particularly secure with 6, 7 or 8 times tables. They have a lot more going on in their working memory. – How has \frac{4}{5} suddenly become \frac{24}{30} ? – What’s 4 × 6? 6, 12, 18, … – How does the teacher just ‘know’ what the denominator is? Why are they talking about multiples?

Poor Student B is disadvantaged from the start because their working memory is dealing with lots of additional tasks alongside the new concept. This means that Student B’s progress will be slower than Student A’s.

The gaps in foundational prior knowledge should ideally be filled in before new concepts are introduced. If this is not possible (due to curriculum design or time constraints), we could support Student B by taking some of the cognitive load off their working memory. In this example, we could provide them with a multiplication chart.

This is a hugely oversimplified model, and I don’t pretend to be a cognitive science expert.

However, we’ve all seen this in the classroom where students seriously struggle to grasp a new concept. It isn’t because the new concept itself is particularly difficult, but because their brains are having to work harder than they should because they’re also juggling several other poorly understood fundamental concepts at the same time.

---

4. Explore links and underlying mathematical structures using models

When introducing new topics, rather than teaching these as distinct pockets of information, we should try (where possible) to incorporate the new material into their existing structure of knowledge and understanding. It can be very useful for students to have existing models or frameworks to ‘hook’ the new information onto.

Visualizing math content through modeling

This is where manipulatives and models can be very useful. I particularly like bar modeling and algebra tiles for diagrammatic representations of a mathematical scenario. There are some great interactive versions available online.

It’s worth highlighting that these models are not generally the end goal. That being said, they can be an appropriate stopping point for some students and are valid methods for solving problems at high school level.

There is sometimes a temptation to cut out modeling, skim over the understanding, and go straight to teaching an algorithm due to time pressures.

However, if students are taught a step-by-step procedure with none of the underlying conceptual ideas, they will be less successful when applying these ideas to non-standard problems. They’ll also be less able to recall the steps when they are needed further down the line.

Fraction addition example: modeling ideas

Returning to our original example of fraction addition, if there were underlying misconceptions relating to how fractions work, I would strip the ideas back down to basics, using partitioned bars to illustrate examples of equivalent fractions.

Ensure students can find equivalent fractions, then build this back up to adding like fractions, and finally, unlike fractions. The speed at which this occurred would depend on the class’s level of understanding.

Another way to explore links is to consider multiple representations of the same topic.

For example, I’ve used Jo Boaler’s suggestions for multiple representations of sequences to explore the links between sequences, functions and graphs. This kind of material is great for encouraging students to reason mathematically.

---

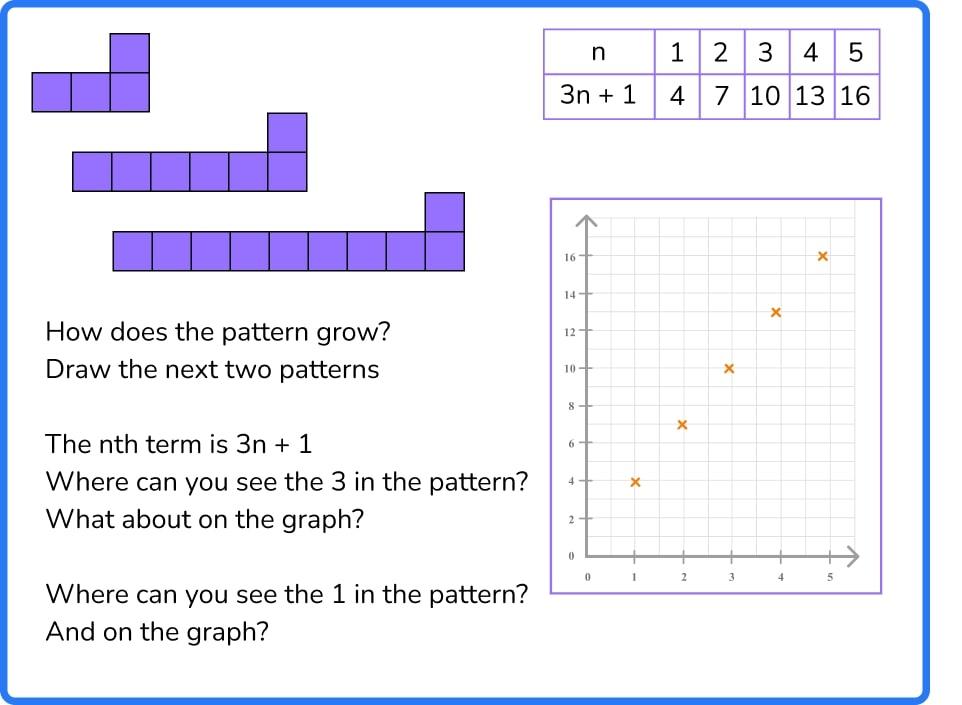
5. Develop familiarity with manipulatives and models

To illustrate this, I discovered algebra tiles when trying to find a way to explain to a group of 9th graders why we half the coefficient of x when completing the square. 

I’d attempted to teach the process algorithmically, which had completely confused the majority of the class, and I realized that I was asking them to do something far too abstract on some pretty shaky foundations.

Following a bit of research, I returned to them with some algebra tiles I’d cut out of cardboard.

We physically moved the x tiles around to convert the rectangular areas into squares; I definitely saw some pennies drop here as to why it was called ‘completing the square’! After a couple of lessons on this, most of them were completing practice problems fairly competently.

However, we had quite a few hang-ups on getting to grips with how the tiles worked; I was simultaneously asking them to become familiar with a new visual representation of algebra, while also asking them to understand quite a complex new concept.

In subsequent years, most of my classes saw algebra tiles long before I asked them to complete the square with them, and this problem was significantly reduced.

---

6. Hook classroom teaching into ‘real-world’ frameworks

I don’t really like the phrase ‘real-world math’. After all, mathematics is the deep underlying structure on which our physical environment is based, but it’s important to try and make connections between classroom mathematics education and everyday life. 

For some topics, such as ratios, this can be easy.

E.g. two people complete a job, one works for three hours, the other only works two, how do they split a $50 payment fairly?

Math students can engage with this and have some pretty good arguments about why it isn’t fair to split the money 50:50 down the middle, particularly if you select a couple of students to play the parts of the workers.

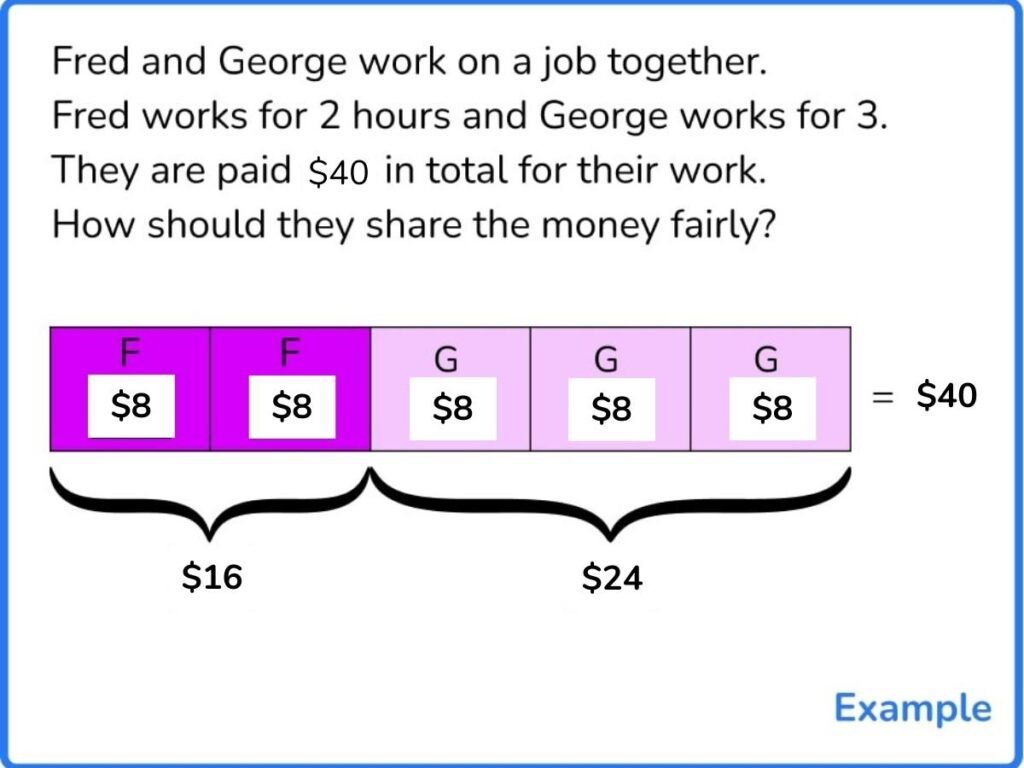
For other topics, we need to be careful that our real-world examples don’t cloud the picture. I’m thinking about things like using trigonometry to calculate the safe angle of a ladder.

While this is a valid real-life application of trigonometry, safe working angles are unlikely to be something that your average 13-year-old needs to think about!

Think again about cognitive overload. Our ratio scenario is going to add a bit of extra processing, but secondary students are likely to have experience with sharing money or at least sharing objects, and the idea of fair and unfair sharing.

These ideas will be easier to think about/process and the scenario becomes useful for enhancing understanding. 

Where there’s a relevant real-world hook that students have direct experience of, or are likely to understand with little-to-no additional information, this can be a nice way into a new concept. I’d recommend saving the applications for more abstract ideas until students are more familiar with the concepts behind them.

---

7. Provide carefully chosen examples to work through with students

The type of examples we provide our students can significantly influence how easily they grasp new concepts. Carefully crafting a hierarchy of worked examples will often result in better understanding.

Fraction addition example: worked example hierarchy

So, for fraction addition, this might go as follows:

• Adding two fractions with unit numerators;
• Adding two fractions where one denominator is a multiple of another;
• Adding two fractions with any two denominators;
• Adding three fractions / subtracting fractions / improper and mixed numbers (depending on prior knowledge).

Working this way removes a lot of initial cognitive load, particularly for those students who have gaps in prior knowledge.

When working with unit fractions, we’re only worrying about sorting denominators. Then, when looking at situations where one denominator is a multiple of the other, it’s easier to ‘spot’ the common denominator to use.

This also means that students whose multiplication recall isn’t great aren’t worrying about multiples, and can attend to the underlying concepts of fraction addition.

I’d judge the number of necessary examples (and the speed at which to work through them) from the student’s prior knowledge and how they did with their starting points assessment.

For some classes, a brief overview would be sufficient. For some, it might be necessary to break it up into chunks over several lessons:

• First covering unit denominators;
• Then giving students an opportunity to practice this;
• Then moving on to denominators with multiples, and so on.

---

8. Incorporate guided practice and faded examples into teaching

When I first started teaching, my process for worked examples was:

• I do one on the board and check for general understanding;
• They’re still confused, so I do another one;
• Some of them are ready to go now, but half of them are still lost, so we do another one as a class;
• Most of them are now getting fidgety, so I set them off on the practice task, then try to rush around to all the students who are still stuck and teach them again individually, inevitably wearing myself out and giving myself a headache!

This process was not particularly effective! I gradually tweaked my process over the years, incorporating a couple of key elements, guided practice and faded examples.

Method 1: guided practice

Guided practice (or ‘I do, we do, you do’ as I call it with students) is a great tool for supporting the learning of any skill, not just learning math skills.

It’s also closely related to how we appear to learn new skills or concepts naturally. Let’s take the example of a baby learning to walk.

1. First, they observe the people around them;
2. Then, they try to copy with support;
3. Next, they take the first few wobbly steps on their own;
4. Finally, they progress to confident walking, then running.

Method 2: faded examples

Faded examples are ones where steps are removed gradually.

1. The first example presented has all steps complete;
2. The next example omits a stage for students to fill in;
3. The next leaves out more steps, until students are completing the task independently.

The stage(s) omitted can be at any point in the process, and will probably depend on the topic being taught. 

A work in progress: using worked example methods in practice

Now, my examples process looks a bit like this:

• I do an example on the board, sometimes in silence, sometimes with an explanation (depending on the topic/class/starting points);
• We discuss the example and pull out key points through effective questioning;
• I work through a second example using the same process, but ask them to join in to complete the final step on their whiteboards (we do);
• We discuss and look for any misconceptions/problems;
• We look at a third example, with minimal guidance from me, except perhaps the starting step or a hint (we do);
• Students complete another couple of practice problems on their whiteboards, showing me after each one. This allows me to pick up on those who still have misconceptions that need addressing (you do);
• Students begin the independent task, while I support those who are still struggling. At this point, there should be less than five of them, so I get them in a small group and re-teach.

---

9. Always master the basics before problem-solving

I’m only going to include a very brief discussion about reasoning and problem solving here due to the scope of this article; this is not to trivialize the very complex issue of ‘teaching problem solving’ (as far as this is possible anyway).

Applying their skills to unfamiliar math problems is something that students really struggle with. Check out Willingham 2009 ‘Why don’t students like school’ (excerpt) for an explanation of why this is a problem inherent to our brain structure and not a deficit particular to teenagers!

My key takeaway for problem-solving is this: don’t ask students to solve problems when they’ve just met a topic for the first time.

They’re unlikely to have well-formed concepts in their long-term memory to draw upon, and the cognitive load of trying to simultaneously process new information and also solve unfamiliar problems using these ideas will be too great.

This often results in very limited success and a spiral of:

• “I don’t get math”
• “I don’t understand any of this”
• “I’m bad at math”

When looking to improve students’ problem-solving skills, I’d try to select a topic I know they’d ideally mastered, or at least mostly understood and were fairly confident with.

This can be a good opportunity to revisit prior knowledge from previous topics; frequently recalling information from long-term memory means it is less likely to be forgotten.

---

And there you have it; 9 essential processes for teachers and for teachers to work through together, whatever stage they’re at!

We’ve only explored how to learn math when teaching new concepts. It’s equally important to plan your teaching so that topics are revisited frequently at the appropriate spacing.

This ensures that all the hard work on developing conceptual understanding isn’t lost because students forget what they’ve learned.

Read more: 13 effective learning strategies

Do you have students who need extra support in math?
Give your students more opportunities to consolidate learning and practice skills through personalized math tutoring with their own dedicated online math tutor.

Each student receives differentiated instruction designed to close their individual learning gaps, and scaffolded learning ensures every student learns at the right pace. Lessons are aligned with your state’s standards and assessments, plus you’ll receive regular reports every step of the way.

Personalized one-on-one math tutoring programs are available for:
– 2nd grade tutoring
– 3rd grade tutoring
– 4th grade tutoring
– 5th grade tutoring
– 6th grade tutoring
– 7th grade tutoring
– 8th grade tutoring

Why not learn more about how it works?

The content in this article was originally written by a former secondary Maths teacher Christine Norledge and has since been revised and adapted for US schools by elementary math teacher Christi Kulesza.