Area Of A Pentagon - Math Steps, Examples & Questions

High Impact Tutoring Built By Math Experts

Personalized standards-aligned one-on-one math tutoring for schools and districts

Request a demo

In order to access this I need to be confident with:

Area of a rectangle Area of a quadrilateral Area of a triangle

Interior angles in polygons

Isosceles triangle Pythagoras theorem

Trigonometry

Math resources Geometry Area

Area of a pentagon

Here you will learn about the area of a pentagon, including how to find the area of a pentagon made from a triangle and a rectangle and how to find the area of a regular pentagon.

Students will first learn about the area of a pentagon as a part of geometry in high school.

What is the area of a pentagon?

The area of a pentagon is the amount of space inside a 2D, five-sided polygon. A regular pentagon is a type of pentagon that has equal sides and all angles have an equal measure.

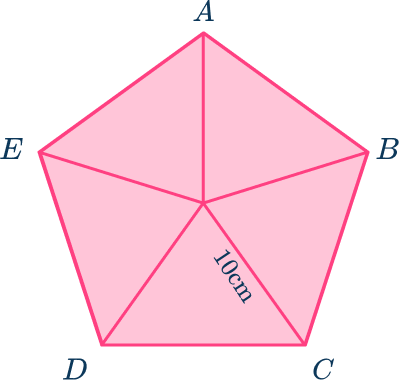
The area of a regular polygon can be found by splitting the shape into congruent isosceles triangles. You can find the area of one of the triangles and then multiply by the number of sides to find the total area of the regular polygon.

To find the area of a regular pentagon, you can use the following formulas,

The area of a regular pentagon can be calculated by:

\text {Area of a regular pentagon }=5 \times \text { area of one triangle. }

The formula for the area of a regular pentagon can also be written as

\text {Area of a regular pentagon }=5 \times \cfrac{1}{2} \, b h.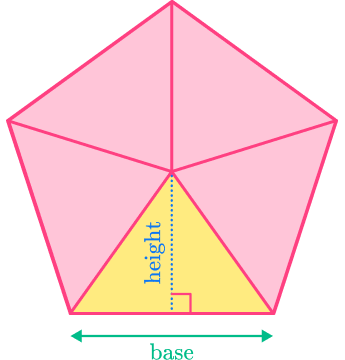

Where $b$ is the base length (or the side of the pentagon) and $h$ is the perpendicular height of the triangle. Sometimes these values will need to be calculated.

For example,

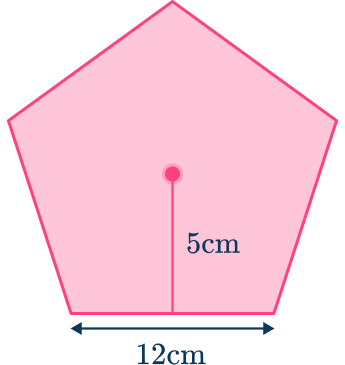
The area of this regular pentagon is

\begin{aligned}& =5 \times \cfrac{1}{2} \times 12 \times 5 \\\\ & =150 \mathrm{~cm}^2 \end{aligned}

[FREE] Area Worksheet (Grade 4 to 6)

Use this quiz to check your grade 4 to 6 students’ understanding of area. 15+ questions with answers covering a range of 4th, 6th and 7th grade area topics to identify areas of strength and support!

DOWNLOAD FREE

[FREE] Area Worksheet (Grade 4 to 6)

DOWNLOAD FREE

Area of an irregular pentagon

An irregular pentagon may have been formed by combining other shapes such as rectangles and triangles. The area of each of these can be found and added together to find the area of the pentagon.

For example,

The area of irregular pentagon $ABCDE$ is

\text{Total area }=(\cfrac{1}{2}\times 10\times 5)+(4\times 10)=25+40=65 \ cm^2.

Angles in a regular pentagon

You can find the angles of a regular polygon to answer a variety of different area questions.

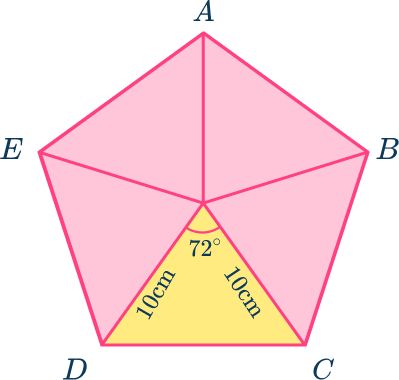
Splitting the regular pentagon into $5$ congruent isosceles triangles, use the angle fact that the sum of internal angles at a point is $360^{\circ}$ to find the angle at the top of the isosceles triangle.

360\div 5=72^{\circ}

The equal base angles can be found by using the angle fact that the sum of angles in a triangle is $180^{\circ}.$

(180-72)\div 2=54^{\circ}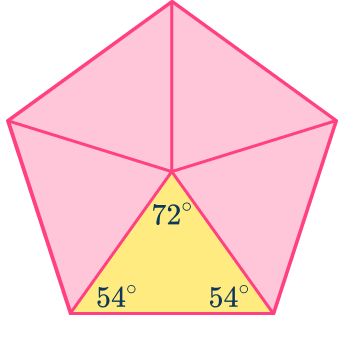

Step-by-step guide: Types of angles

The radii of a regular pentagon

A regular pentagon has five radii. The apothem is the radius of the inscribed circle, $R_i.$

The radius of the inscribed circle is perpendicular to the sides of the regular pentagon and meets the side at the midpoint.

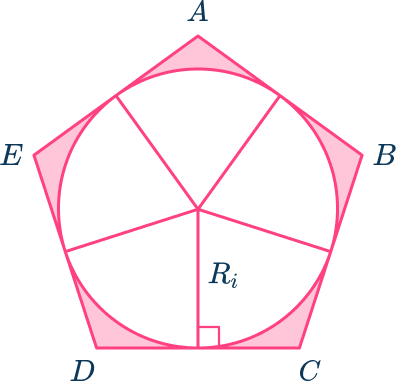
When a regular pentagon is drawn within a circle the circle is known as the circumcircle and the radius is $R_c.$

Area formula using the perimeter and apothem of a pentagon

The height (or inscribed radius) in this regular pentagon is also known as the apothem of the pentagon.

We can use the following formula to find the area of a regular pentagon,

\text{Area of a regular pentagon }= \cfrac{1}{2}\times \text{ perimeter }\times \text{ apothem}.

The perimeter of the pentagon can be found by multiplying the side length by $5.$

What is the area of a pentagon?

Common Core State Standards

How does this relate to high school math?

High School: Geometry (HS.G.SRT.D.11)
Understand and apply the Law of Sines and the Law of Cosines to find unknown measurements in right and non-right triangles (e.g., surveying problems, resultant forces).

How to find the area of a pentagon

In order to find the area of a pentagon, you will:

Consider the pentagon as a compound shape and split it appropriately.
Calculate the area of the parts.
Calculate the total area of the pentagon.
Write the answer, including the correct units.

Area of a pentagon examples

Example 1: area of an irregular pentagon formed with one triangle and a rectangle

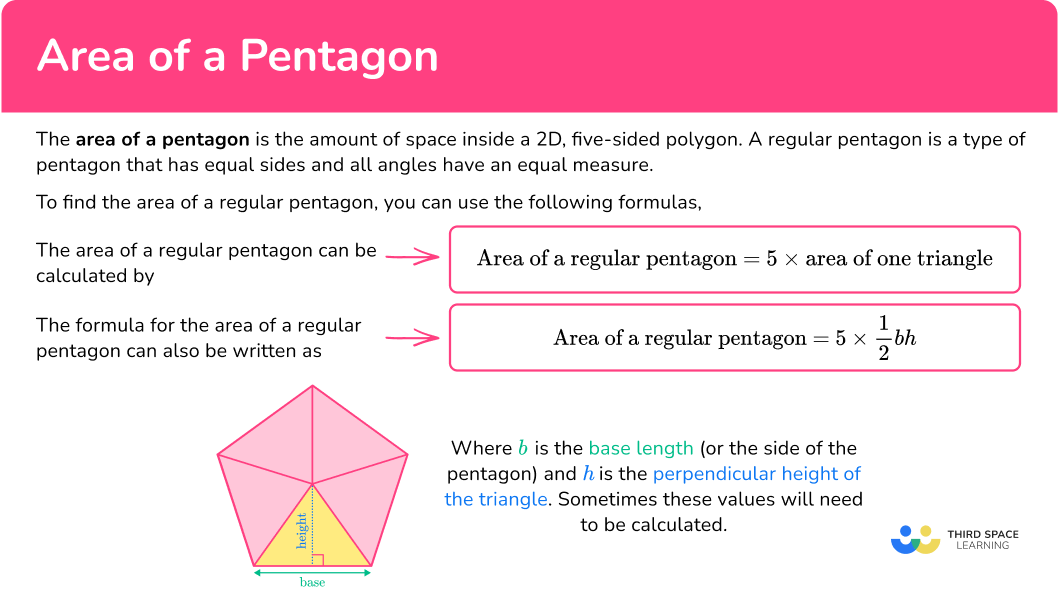
$ABCDE$ is a pentagon.

Find the area of the pentagon.

Consider the pentagon as a compound shape and split it appropriately.

The diagram given in the question is already split into a rectangle and a triangle.

2Calculate the area of the parts.

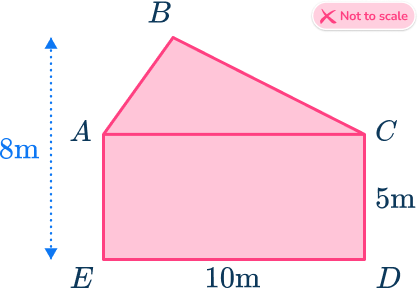
Find the area of the triangle $ABC.$

The height of the triangle, $h,$ is $h=8-5=3.$

Therefore, the area of the triangle is $A=\cfrac{1}{2} \, bh=\cfrac{1}{2}\times 10\times 3=15.$

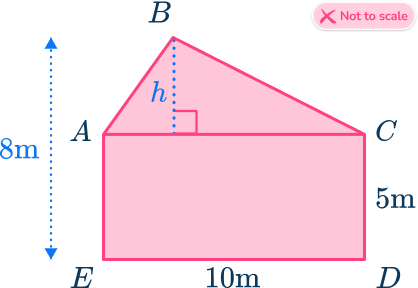
The area of the rectangle $ACDE$ is $A=bh=10\times 5=50.$

3Calculate the total area of the pentagon.

The total area of the pentagon is found by adding the area of the triangle and the area of the rectangle together.

\text{Total Area }=15+50=65

4Write the answer, including the correct units.

The dimensions of the shape are given in meters, so the units of the area will be in square meters. The area of pentagon $ABCDE$ is $65 \, m^2.$

Example 2: area of an irregular pentagon formed with one isosceles triangle and a rectangle

$ABCDE$ is a pentagon.

Calculate the area of the pentagon.

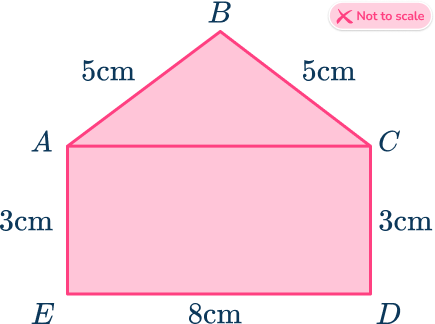
Consider the pentagon as a compound shape and split it appropriately.

The diagram given in the question is already split into a rectangle and a triangle.

Calculate the area of the parts.

Find the area of the triangle $ABC.$

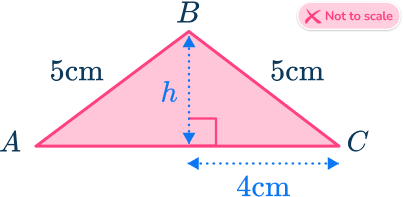
You can find $h$ the height of the triangle by using Pythagoras’ theorem.

$h=\sqrt{5^2-4^2}=3$

Note: The height can also be found as it is part of the $3, 4, 5$ Pythagorean triple.

Therefore, the area of the triangle is $A=\cfrac{1}{2} \, bh=\cfrac{1}{2}\times 8\times 3=12.$

The area of the rectangle $ACDE$ is $A=bh=8\times 3=24.$

Calculate the total area of the pentagon.

The total area of the pentagon is found by adding the area of the triangle and the area of the rectangle together.

$\text{Total Area }=12+24=36$

Write the answer, including the correct units.

The dimensions of the shape are given in centimeters, so the units of the area will be in square centimeters. The area of pentagon $ABCDE$ is $36 \, cm^2.$

Example 3: calculating the area of a regular pentagon given the area of one of the isosceles triangles

Calculate the area of the regular pentagon $ABCDE$ given that the area of one of the congruent triangles is $20 \, cm^2.$

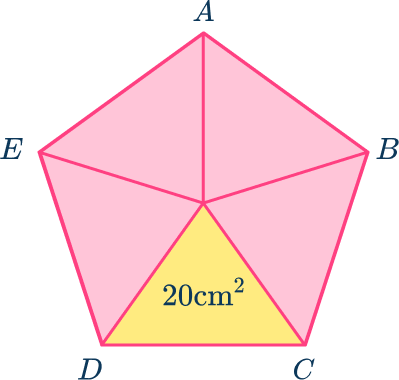
Consider the pentagon as a compound shape and split it appropriately.

The diagram given in the question is already split into five congruent isosceles triangles.

Calculate the area of the parts.

The area of one triangle has been given, $20 \, cm^2.$

Calculate the total area of the pentagon.

The total area of the regular pentagon is

$\text{Area of a regular pentagon }=5\times \text{ Area of one triangle}$

$\begin{aligned}&=5\times 20 \\\\ &=100 \end{aligned}$

Write the answer, including the correct units.

The area of pentagon $ABCDE$ is $100 \, cm^2.$

Example 4: area of a pentagon given the side length

Calculate the area of the regular pentagon $ABCDE$ given that the length of one side of the regular pentagon is $10 \, cm.$

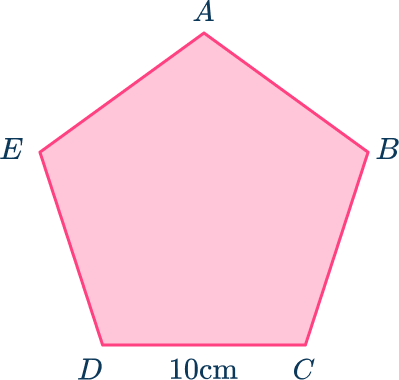
Consider the pentagon as a compound shape and split it appropriately.

Here, the regular pentagon $ABCDE$ has been split into five congruent isosceles triangles.

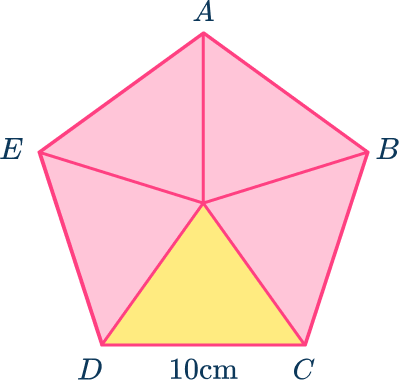
Calculate the area of the parts.

You can find the area of one of the isosceles triangles by using the side length as the base length of the triangle.

You need to work out the height of the triangle. This can be done using trigonometry and the angles in the triangle.

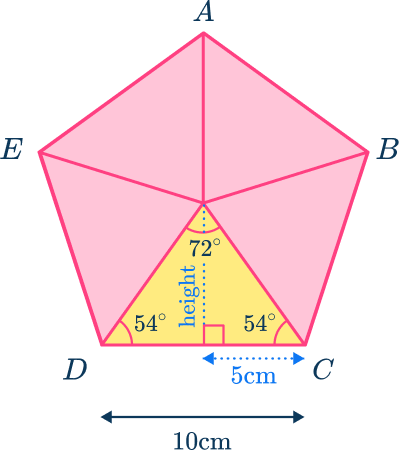
$\text{height}=\tan(54)\times 5=6.8819…$

The area of one of the isosceles triangles is

\begin{aligned} A&=\cfrac{1}{2} \, bh \\\\ &=\cfrac{1}{2}\times 10\times 6.8819… \\\\ &=34.4095… \end{aligned}

Calculate the total area of the pentagon.

The total area of the regular pentagon is

$\text{Area of a regular pentagon }=5\times \text{ area of one triangle}$

\begin {aligned}&=5\times 34.4095… \\\\ &=172.0477… \end {aligned}

Write the answer, including the correct units.

The dimensions of the shape are given in centimeters, so the units of the area will be in square centimeters. The area of pentagon $ABCDE$ is $172 \, cm^2$ (to $3$ significant figures).

Example 5: area of a pentagon given the height (apothem)

Calculate the area of the regular pentagon $ABCDE$ given that the length of the apothem of the regular pentagon is $12 \, cm.$

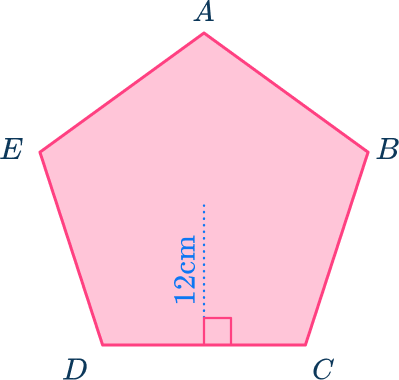
Consider the pentagon as a compound shape and split it appropriately.

Here, the regular pentagon $ABCDE$ has been split into five congruent isosceles triangles.

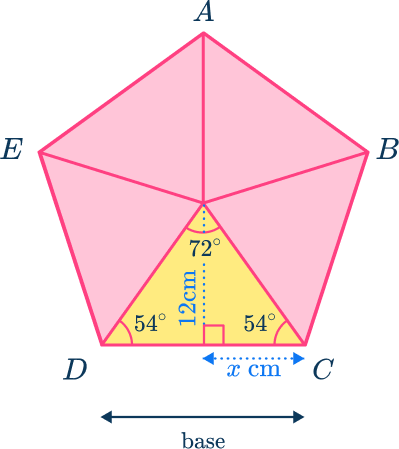
Calculate the area of the parts.

You can find the area of one of the triangles by using the apothem as the perpendicular height of the triangle.

You need to work out the base of the triangle. This can be done using trigonometry and the angles in the isosceles triangle.

$x=12\div \tan(54)=8.7185…$

The base of one of the isosceles triangles is

$\text{base}=2x=2\times 8.7185…=17.4370…$

The area of one of the isosceles triangles is

\begin{aligned}A&=\cfrac{1}{2} \, bh. \\\\ &=\cfrac{1}{2}\times 17.4370…\times 12 \\\\ &=104.6221… \end{aligned}

Calculate the total area of the pentagon.

The total area of the regular pentagon is

$\text{Area of a regular pentagon }=5\times \text{ area of one triangle}$

\begin{aligned}&=5\times 104.6221… \\\\ &=523.1106… \end{aligned}

Write the answer, including the correct units.

The dimensions of the shape are given in centimeters, so the units of the area will be in square centimeters. The area of pentagon $ABCDE$ is $523 \, cm^2$ (to $3$ significant figures).

Example 6: area of a pentagon given the radius of the circumcircle

Calculate the area of the regular pentagon $ABCDE$ given that the length from the center of the pentagon to the vertices is $15 \, cm.$

Consider the pentagon as a compound shape and split it appropriately.

Here is a sketch of the regular pentagon $ABCDE$ showing the five congruent isosceles triangles.

Calculate the area of the parts.

You need to work out the height of the triangle using trigonometry and the angles in the isosceles triangle. The hypotenuse is $15 \, cm.$

$\text{height}=\sin(54)\times 15=12.13525…$

You need to work out the base of the triangle using trigonometry and the angles in the isosceles triangle. The hypotenuse is $15 \, cm.$

$x=\cos(54) \times 15=8.81677…$

The base of one of the isosceles triangles is

$\text{base}=2x=2\times 8.81677…=17.63355…$

The area of one of the isosceles triangles is

\begin{aligned}A&=\cfrac{1}{2} \, bh. \\\\ &=\cfrac{1}{2}\times 17.63355…\times 12.13525… \\\\ &=106.993… \end{aligned}

Calculate the total area of the pentagon.

The total area of the regular pentagon is

$\text{Area of a regular pentagon }=5\times \text{ area of one triangle}$

\begin{aligned}&=5\times 106.993… \\\\ &=534.96… \end{aligned}

Write the answer, including the correct units.

The dimensions of the shape are given in centimeters, so the units of the area will be in square centimeters. The area of pentagon $ABCDE$ is $535 \, cm^2$ (to $3$ significant figures).

Example 7: area of a pentagon given the radius of the circumcircle (diagonals)

Consider the pentagon as a compound shape and split it appropriately.

The diagram given in the question is already split into five congruent isosceles triangles.

Calculate the area of the parts.

You need to work out the area of one of the triangles using trigonometry and the angles in the isosceles triangle. You can use HIGHER trigonometry.

\begin{aligned}A&= \cfrac{1}{2} \, ab \sin C \\\\ &=\cfrac{1}{2} \times 10\times 10\times \sin (72) \\\\ &=47.5528… \end{aligned}

Calculate the total area of the pentagon.

The total area of a regular pentagon is

$\text{Area of a regular pentagon }=5\times \text{ area of one triangle}$

\begin{aligned}&=5\times 47.5528… \\\\ &=237.764… \end{aligned}

Write the answer, including the correct units.

The dimensions of the shape are given in centimeters, so the units of the area will be in square centimeters. The area of pentagon $ABCDE$ is $238 \, cm^2$ (to $3$ significant figures).

Teaching tips for the area of a pentagon

Start by breaking down the formula for the area of a regular pentagon. This isn’t as commonly memorized as some other formulas, so students will benefit from the additional instruction.

Instead of giving students worksheets to work on independently, consider allowing students to work through these practice problems in small groups or in pairs. This allows students to discuss and collaborate on answers, which can lead to students learning from one another.

Use technology, such as an online pentagon calculator or geometry software, which allows learning to be more interactive and engaging for some students.

Easy mistakes to make

Using the wrong units
Remember that area is measured in square units. Square units include square centimeters $(cm^2),$ square meters $(m^2)$ and square millimeters $(mm^2).$

Confusing regular and irregular pentagons
It’s important to identify whether a pentagon is regular or irregular before calculating the area. Remember regular pentagons have all equal sides, while irregular pentagons do not.

Practice area of a pentagon questions

1050 \, m^2

360 \, m^2

255 \, m^2

202.5 \, m^2

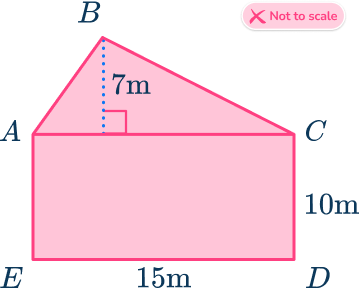
The area of the triangle $ABC$ is $A=\cfrac{1}{2} \, bh=\cfrac{1}{2}\times 15\times 7=52.5.$

The area of the rectangle $ACDE$ is $A=bh=15\times 10=150.$

The total area of the pentagon is found by adding the area of the triangle and the area of the rectangle together.

\text{Total Area}=52.5+150=202.5 \ m^2

1580 \, m^2

1280 \, m^2

1780 \, m^2

1880 \, m^2

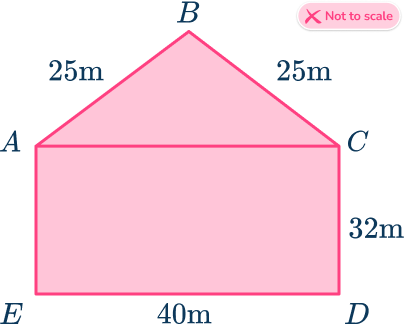
The height of the triangle is $h=\sqrt{25^2-20^2}=15.$

Area of a pentagon 26 US

The area of the triangle $ABC$ is $A=\cfrac{1}{2} \, bh=\cfrac{1}{2}\times 40\times 15=300.$

The area of the rectangle $ACDE$ is $A=bh=40\times 32=1280.$

The total area of the pentagon is found by adding the area of the triangle and the area of the rectangle together.

\text{Total Area}=300+1280=1580 \ m^2

3 \, cm^2

60 \, cm^2

75 \, cm^2

100 \, cm^2

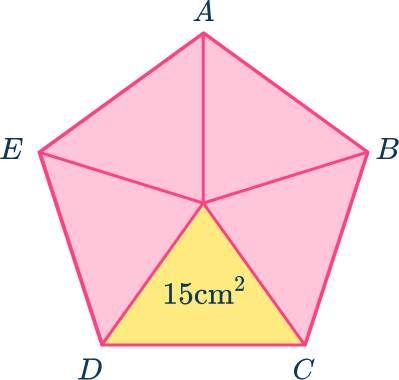
The area of the regular pentagon is

\begin{aligned} & =5 \times \text { area of one triangle } \\\\ & =5 \times 15 \\\\ & =75 \mathrm{~cm}^2 \end{aligned}

1453 \, cm^2

951 \, cm^2

688 \, cm^2

138 \, cm^2

The height of the triangle is

\text{height}=\tan(54)\times 10=13.7638…

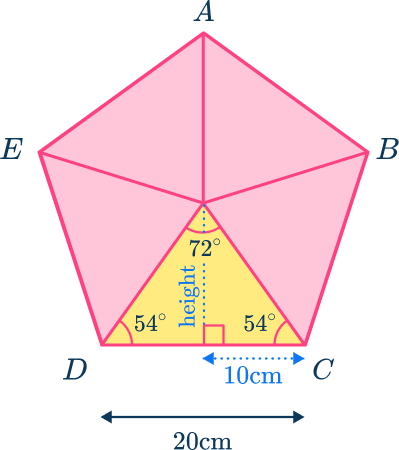
Area of a pentagon 29 US

The area of one of the isosceles triangles is

\begin{aligned}A&=\cfrac{1}{2} \, bh \\\\ &=\cfrac{1}{2}\times 20\times 13.7638… \\\\ &=137.638… \end{aligned}

The area of the regular pentagon is

\begin{aligned} & =5 \times \text { area of one triangle } \\\\ & =5 \times 137.638 \\\\ & =688.190 \ldots \end{aligned}

The area of the regular pentagon is $688 \, cm^2$ to $3$ significant figures.

2750 \, cm^2

5810 \, cm^2

11011 \, cm^2

3804 \, cm^2

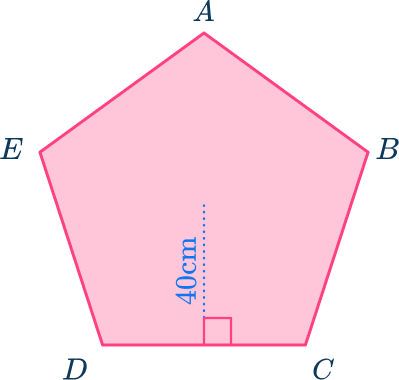
Using trigonometry,

Area of a pentagon 31 US

x=40\div \tan(54)=29.0617…

The base of one of the isosceles triangles is

\text{base}=2x=2\times 29.0617…=58.1234…

The area of one of the isosceles triangles is

\begin{aligned}A&=\cfrac{1}{2}bh. \\\\ &=\cfrac{1}{2}\times 58.1234…\times 40 \\\\ &=1162.468… \end{aligned}

The area of the regular pentagon is

\begin{aligned} & =5 \times \text { area of one triangle } \\\\ & =5 \times 1162.468 \ldots \\\\\ & =5812.34 \ldots \end{aligned}

The area of pentagon $ABCDE$ is $5810 \, cm^2$ (to $3$ significant figures).

497 \, cm^2

1050 \, cm^2

687 \, cm^2

137 \, cm^2

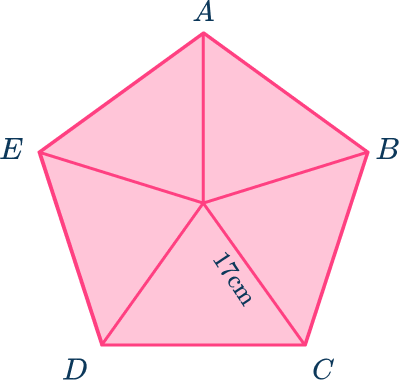
Using trigonometry,

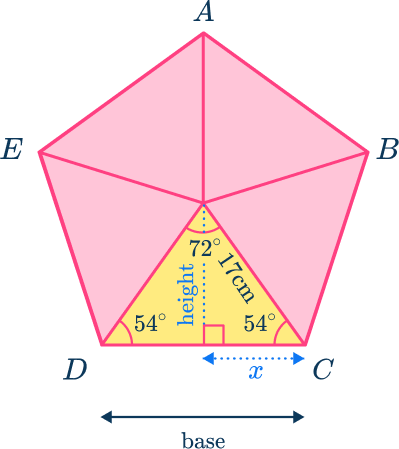
Area of a pentagon 33 US

The height of the triangle can be calculated using trigonometry and the angles in the isosceles triangle. The hypotenuse is $17 \, cm.$

\text{height}=\sin(54)\times 17=13.7532…

You need to work out the base of the triangle using trigonometry.

x=\cos(54) \times 17=9.9923…

The base of one of the isosceles triangles is

\text{base}=2x=2\times 9.9923…=19.9846…

The area of one of the isosceles triangles is

\begin{aligned}A&=\cfrac{1}{2}bh. \\\\ &=\cfrac{1}{2}\times 19.9846…\times 13.7532… \\\\ &=137.427… \end{aligned}

The area of the regular pentagon is

\begin{aligned} & =5 \times \text { area of one triangle } \\\\ & =5 \times 137.427 . . \\\\ & =687.138 \ldots \end{aligned}

The area of pentagon $ABCDE$ is $687 \, cm^2$ (to $3$ significant figures).

Area of a pentagon FAQs

What is the area of a pentagon formula?

A formula that can be used to find the area of a pentagon is

$\text{Area of a regular pentagon } = 5 \, \times \text{ area of one triangle,}$ or

$\text{Area of a regular pentagon } = 5 \times \cfrac{1}{2} \, bh.$

How do you find the measure of the apothem?

The apothem is the distance from the center of a polygon to its sides. To find the apothem, find the center of the shape and then draw a line from the center to the midpoint of one side of a pentagon.

The next lessons are

Still stuck?

At Third Space Learning, we specialize in helping teachers and school leaders to provide personalized math support for more of their students through high-quality, online one-on-one math tutoring delivered by subject experts.

Each week, our tutors support thousands of students who are at risk of not meeting their grade-level expectations, and help accelerate their progress and boost their confidence.