[FREE] Fun Math Games & Activities Packs
Always on the lookout for fun math games and activities in the classroom? Try our ready-to-go printable packs for students to complete independently or with a partner!
In order to access this I need to be confident with:
Addition and subtraction Simplifying fractions Equivalent fractions FactorsHow to write a ratio
Here you will learn about how to write a ratio, including how to write and simplify ratios.
Students will first learn about how to write a ratio as part of ratios and proportions in 6 th grade.
What is writing a ratio?
Writing a ratio is a way of showing the constant relationship between two or more quantities. The quantities can be in the same or different units. Ratios are commonly written in the form a: b.
Example 1
In a class of students, there are 13 boys and 17 girls.
The ratio of boys to girls can be written as 13:17.
The ratio of girls to boys can be written as 17:13.
The ratio of total students to boys can be written as 30:13.
Example 2
In a recipe, there are three eggs, one onion, and two tomatoes. The ratio of eggs to onions is 3:1.
Example 3
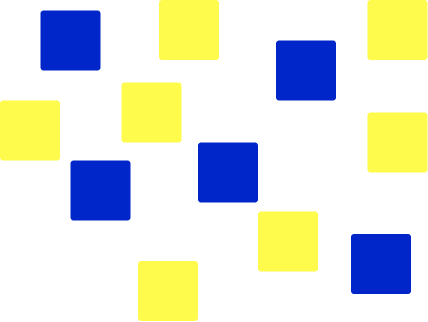
There are 7 yellow squares and 5 blue squares. There are 12 squares in total.
The ratio of yellow to blue squares is 7:5.
The ratio of blue to yellow squares is 5:7.
The ratio of yellow squares to the total number of squares is 7:12.
The ratio of blue squares to the total number of squares is 5:12.
After you have written a ratio, like in the examples above, it is possible that the ratio can be simplified by writing an equivalent ratio. When a ratio is fully simplified, the parts of the ratio are all integers with no common factors.
What is writing a ratio?
Common Core State Standards
How does this relate to 6 th grade math?
- Grade 6 – Ratios and Proportional Relationships (6.RP.A.1)
Understand the concept of a ratio and use ratio language to describe a ratio relationship between two quantities. For example, “The ratio of wings to beaks in the bird house at the zoo was 2:1, because for every 2 wings there was 1 beak.” “For every vote candidate A received, candidate C received nearly three votes.”
- Grade 6 – Ratios and Proportional Relationships (6.RP.A.2)
Understand the concept of a unit rate a/b associated with a ratio a:b with b≠ 0, and use rate language in the context of a ratio relationship.
For example, “This recipe has a ratio of 3 cups of flour to 4 cups of sugar, so there is \cfrac{3}{4} \, cup of flour for each cup of sugar.” “We paid \$75 for 15 hamburgers, which is a rate of \$5 per hamburger.”
How to write a ratio
In order to write a ratio:
- Identify the different quantities being compared and their order.
- Write the ratio using a colon.
- Check if the ratio can be simplified.
![[FREE] Ratio Check for Understanding Quiz (Grade 6 and 7)](https://thirdspacelearning.com/wp-content/uploads/2023/07/Ratio-check-for-understanding-quiz-listing-image-.png)
[FREE] Ratio Check for Understanding Quiz (Grade 6 and 7)
Use this quiz to check your 6th and 7th grade students’ understanding of ratios. 10+ questions with answers covering a range of 6th and 7th grade ratio topics to identify areas of strength and support!
DOWNLOAD FREE [FREE] Ratio Check for Understanding Quiz (Grade 6 and 7)
Use this quiz to check your 6th and 7th grade students’ understanding of ratios. 10+ questions with answers covering a range of 6th and 7th grade ratio topics to identify areas of strength and support!
DOWNLOAD FREEHow to write a ratio examples
Example 1: writing a ratio about an everyday life situation
Ms. Holly is looking after 7 children. She has 5 toys in her nursery. Write the ratio of children to toys.
- Identify the different quantities being compared and their order.
There are 7 children and 5 toys.
The order of the ratio is children to toys.
2Write the ratio using a colon.
Children : Toys
\hspace{0.9cm}7 : 5
3Check if the ratio can be simplified.
7 and 5 only have a common factor of 1, so this ratio is already in its lowest terms (simplest form).
Example 2: writing a ratio about a real life situation
On a farm there are 20 pigs, 8 cows and 124 chickens. Write the ratio of chickens to cows in lowest terms.
Identify the different quantities being compared and their order.
There are 8 cows and 124 chickens.
The order of the ratio is chickens to cows.
Write the ratio using a colon.
Chickens : Cows
\hspace{0.6cm}124 : 8
Check if the ratio can be simplified.
124 and 8 have common factors of 1, 2 and 4 so this ratio can be simplified.
The ratio of chickens to cows in lowest terms is 31:2.
Example 3: writing a ratio from a picture
Use the diagram below to write the ratio of white counters to the total number of counters in lowest terms.
Identify the different quantities being compared and their order.
There are 16 white counters and 24 counters in total.
The order of the ratio is white counters to total number of counters.
Write the ratio using a colon.
White counters : Total counters
\hspace{1.6cm}16 : 24
Check if the ratio can be simplified.
16 and 24 have common factors of 1, 2, 4 and 8 so this ratio can be simplified.
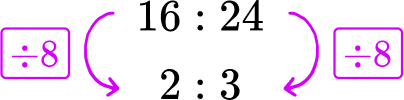
The ratio of white counters to total counters in lowest terms is 2:3.
Example 4: writing a ratio from a graph
The bar chart shows the number of left handed and right handed people in a group.
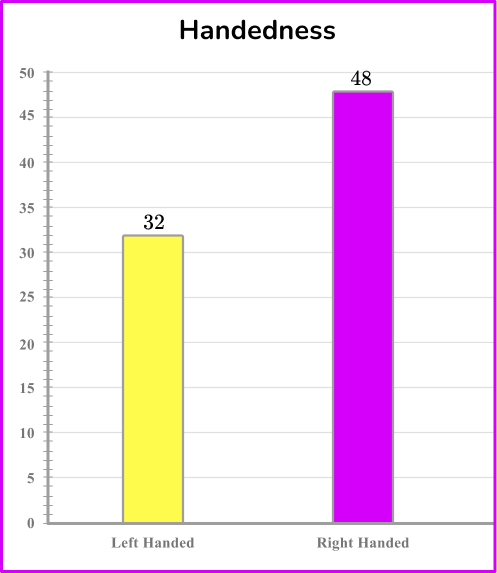
Write the ratio of left handed people to the total number of people in lowest terms.
Identify the different quantities in the question.
There are 32 left handed people.
There are 32 + 48 = 80 people in total.
Identify the order in which the quantities are to be represented.
Left handed people to the total number of people.
Write the ratio using a colon.
Left handed : total
\hspace{1.1cm}32:80
Check if the ratio can be simplified.
32 and 80 have common factors of 1, 2, 4, 8 and 16 so this ratio can be simplified.
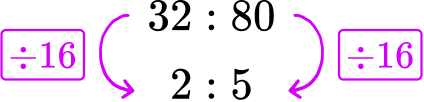
The ratio of left handed people to the total number of people in its lowest terms is 2:5.
Example 5: writing a ratio from a word problem
In a fridge there are three different types of drinks in bottles: water, tea and lemonade. There are 3 bottles of tea and 2 bottles of lemonade. There are 8 bottles in total. What is the ratio of bottles of lemonade to bottles of water?
Identify the different quantities being compared and their order.
3 bottles of tea
2 bottles of lemonade
8 - 2 - 3 = 3 bottles of water
Identify the order in which the quantities are to be represented.
Bottles of lemonade to bottles of water.
Write the ratio using a colon.
lemonade : water
\hspace{1cm}2:3
Check if the ratio can be simplified.
2 and 3 only have a common factor of 1. The ratio cannot be simplified.
The ratio of bottles of lemonade to bottles of water is 2:3.
Example 6: writing an algebraic ratio from a word problem
Amy is a years old. Imran is 12 years older than Amy. Write the ratio of Imran’s age to Amy’s age.
Identify the different quantities in the question.
Amy is a years old
Imran is a+12 years old
Identify the order in which the quantities are to be represented.
Imran’s age to Amy’s age.
Write the ratio using a colon.
Imran : Amy
\hspace{0cm}a+12:a
Check if the ratio can be simplified.
This ratio is already in its lowest terms.
Teaching tips for how to write a ratio
- Worksheets are a good way for students to practice writing ratios, but be sure to include worksheets that show the ratios in a variety of formats. This can include written statements, pictures or graphs. Also choose worksheets that have questions that ask for unsimplified and simplified ratios.
Easy mistakes to make
- Writing the parts of the ratio in the wrong order
When a ratio is written, the order is very important. Remember to read the problem carefully to ensure you get the order right.
For example,
There are 12 dogs and 13 cats. What is the ratio of cats to dogs?
A common mistake would be to write 12:13 because this is the order in which the numbers are presented in the information. However, the question asks for the ratio of ‘cats to dogs’, so the correct answer is 13:12 because there are 13 cats and 12 dogs.
- Confusing wholes and parts
Take the ratio of boys to girls 5:4. This ratio shows two parts. If asked, what is the ratio of boys to total students, the parts need to be added to find the whole. Since 5 + 4 = 9, there are 9 students in all. The ratio of boys to total students is 5:9.
- Confusing fractions and ratios in fraction form
Ratios can also be written as fractions, but this does not typically happen until later to avoid confusion. When students are ready to write a ratio in fraction form, the numerator and the denominator represent the two values being compared. It is important to note that the fraction form of a ratio does NOT always represent a part-whole relationship.
For example,
The ratio of dogs to cats is \cfrac{6}{4} .
The ratio is written the same as an improper fraction, but it is not appropriate to read this ratio as “6 fourths .” The numerator of the fraction represents the dogs 6, and the denominator of the fraction represents the cats 4. This ratio is read as “6 to 4” or “6 dogs to 4 cats.”
Related ratio lessons
- Ratio
- Unit rate math
- Simplifying ratios
- Ratio to percent
- Ratio to fraction
- How to calculate exchange rates
- Ratio problem solving
- Dividing ratios
- How to find the unit rate
- Ratio scale
- Constant of proportionality
Practice how to write a ratio questions
1) In a ball pit, there are 258 red balls, 300 yellow balls, and 546 orange balls. Write the ratio of red balls to yellow balls.
The parts of the ratio should be in order. The first number is red balls. The second number is yellow balls.
There are 258 red balls and 300 yellow balls, so the ratio of red balls to yellow balls is 258:300.
2) A café sells 21 cups of coffee and 14 cups of tea during one lunch break. Write the ratio of coffee to tea sold during the lunch break in lowest terms.
There are 21 cups of coffee and 14 cups of tea.
The ratio of coffee to tea is 21:14.
21 and 14 have common factors of 1 and 7.
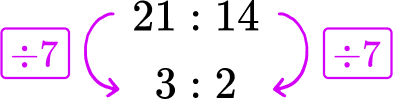
The ratio of coffee to tea sold during the lunch break in lowest terms is 3:2.
3) Mateo is investigating how many people are subscribers of an app. He stands outside a shop and conducts a survey on people he sees using a smartphone. 75 people report that they are not subscribers. 25 people report that they are subscribers. Write the ratio of subscribers to the total number of people surveyed.
The parts of the ratio should be in the order: ‘subscribers’ to ‘total number of people surveyed’.
Calculate the total amount: 75+25 = 100.
Write the ratio 25:100.
25 and 100 have common factors of 1, 5 and 25.
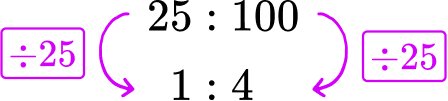
The ratio of people who are subscribers to an app to the total number of people surveyed is 1:4.
4) The bar graph shows the number of fruits. Write the ratio of lemons to blueberries in lowest terms.
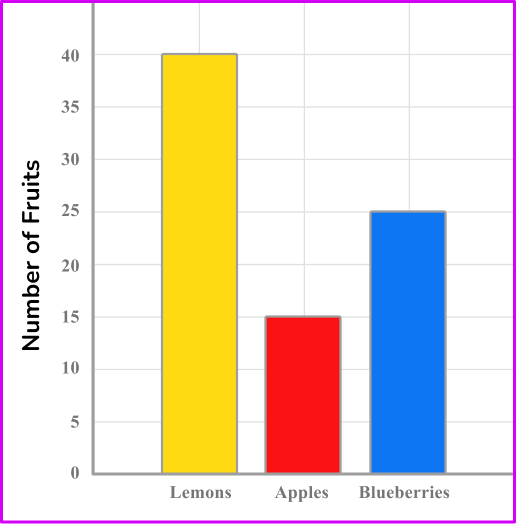
There are 40 lemons and 25 blueberries. The number of apples is not needed.
The ratio of lemons to blueberries is 40:25.
40 and 25 have common factors of 1 and 5.
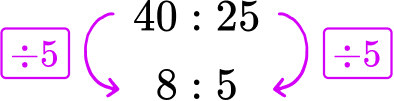
The ratio of lemons to blueberries in lowest terms is 8:5.
5) At a school, 46 students study French and 22 students study Spanish. The rest of the students study German. If there are 98 students at the school, write the ratio of the number of students who study German to the students who study French in lowest terms.
There are 46 students who study French and 22 students who study Spanish. Subtract them from the total to find how many students study German.
98 \, – \, 46 \, – \, 22 = 30 students who study German.
The ratio of German students to French students is 30:46.
30 and 46 have common factors of 1 and 2.
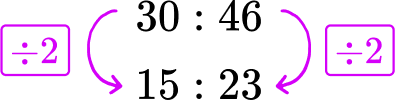
The ratio of German students to French students in lowest terms is 15:23.
6) On a bookcase, there are F number of fiction books and N number of non-fiction books. Write the ratio of fiction books to the total number of books.
There are F+N books in total.
Ratio of fiction books to total number of books is F:F+N.
How to write a ratio FAQs
Yes, ratios can be written with any rational number (including fractions, decimals and negative numbers). This is typically introduced in 7 th grade.
A rate is a special type of ratio. A rate compares two different units of measurement. For example, 3 pounds of apples cost \$9. The pounds are being compared to the dollars – which are different units.
The next lessons are
Still stuck?
At Third Space Learning, we specialize in helping teachers and school leaders to provide personalized math support for more of their students through high-quality, online one-on-one math tutoring delivered by subject experts.
Each week, our tutors support thousands of students who are at risk of not meeting their grade-level expectations, and help accelerate their progress and boost their confidence.
Find out how we can help your students achieve success with our math tutoring programs.
[FREE] Common Core Practice Tests (Grades 3 to 6)
Prepare for math tests in your state with these Grade 3 to Grade 6 practice assessments for Common Core and state equivalents.
40 multiple choice questions and detailed answers to support test prep, created by US math experts covering a range of topics!