Simple interest

One to one maths interventions built for KS4 success

Weekly online one to one GCSE maths revision lessons now available

Learn more

In order to access this I need to be confident with:

Converting percentages to decimals Substitution Percentage change Calculator skills

This topic is relevant for:

GCSE Maths Number Simple Interest And Compound Interest

Simple Interest

Here we will learn about simple interest including how to calculate simple interest for increasing and decreasing values, and how to set up, solve and interpret growth and decay problems.

There are also simple interest worksheets based on Edexcel, AQA and OCR exam questions, along with further guidance on where to go next if you’re still stuck.

What is simple interest?

Simple interest is calculated by finding a percentage of the principal (original) amount and multiplying by the time period of the investment. The final value of the investment can then be found by adding the simple interest to the principal amount.

Simple Interest Formula

Simple interest can be calculated using the following formula:

I=Prt

And we can calculate the value of the investment, $A,$ after the time period with the formula:

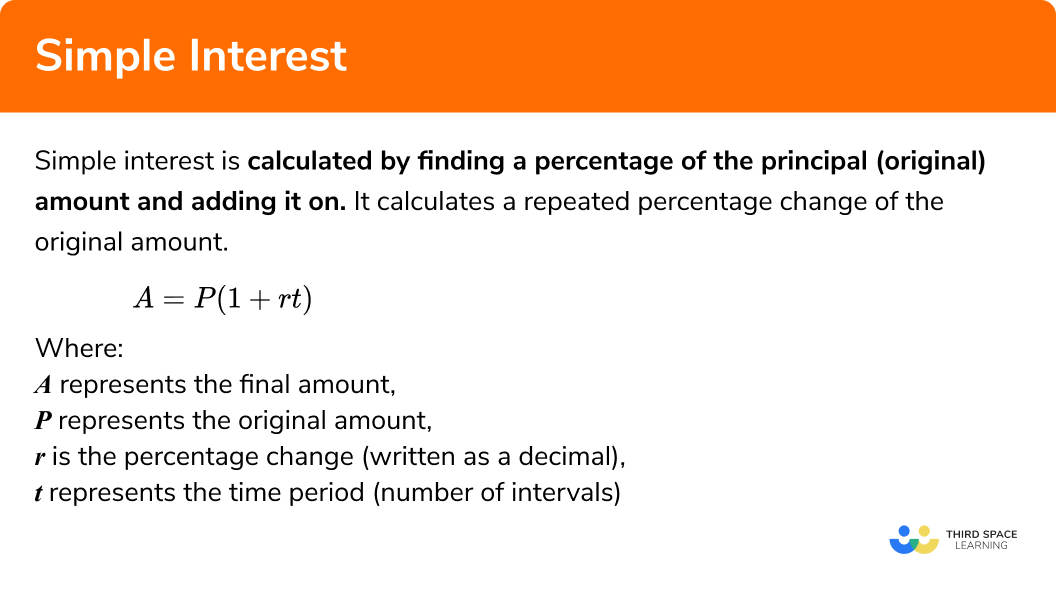
\begin{aligned} A& =P+Prt \\\\ & =P\left( 1+rt \right) \end{aligned}

Where:

$I$ represents the simple interest
$A$ represents the final amount.
$P$ represents the original amount.
$r$ is the percentage rate (written as a decimal).
$t$ represents the time period (number of intervals).

E.g

Lets calculate the interest earned on $£3000$ with a simple interest rate of $5\%$ over $2$ years.

Using the formula $I=Prt,$ remembering that $5\% = 0.05$

\begin{aligned} I&=3000\times 0.05\times 2 \\\\ & =\text{£}300 \end{aligned}

To find the final value of the investment we can now add the interest to the principal amount.

\begin{aligned} A&=3000+300 \\\\ & =\text{£}3300 \end{aligned}

We could have calculated this directly using the formula $A=P\left( 1+rt \right)$

\begin{aligned} A& =3000\left( 1+0.05\times 2 \right) \\\\ & =\text{£}3300 \end{aligned}

What is simple interest?

How to calculate simple interest

In order to calculate simple interest:

State the formula needed and the value of each variable.
Substitute the values into the formula.
Solve the equation.

Explain how to calculate simple interest in 3 steps.

Simple interest worksheet

Get your free simple interest worksheet of 20+ questions and answers. Includes reasoning and applied questions.

DOWNLOAD FREE

Simple interest worksheet

Get your free simple interest worksheet of 20+ questions and answers. Includes reasoning and applied questions.

DOWNLOAD FREE

Simple interest is part of our series of lessons to support revision on simple interest and compound interest. You may find it helpful to start with the main simple interest and compound interest lesson for a summary of what to expect, or use the step by step guides below for further detail on individual topics. Other lessons in this series include:

Simple interest examples

Example 1: Finding the simple interest

$£2100$ is invested for $3$ years at an annual interest rate of $2\%$ per year simple interest. Find the interest earned on the investment in that time?

State the formula needed and the value of each variable

We required the interest so we will use the formula $I=Prt$ with:

$P=2100$
$r=0.02$
$t=3$

2Substitute the values into the formula.

Substituting these values into the simple interest formula $I=Prt,$ we get:

I=2100\times 0.02\times 3

3Solve the equation.

I=£126

$£126$ was earned on the investment.

Example 2: Simple interest – finding the final amount after an increase

$£1500$ is invested for $4$ years at an annual interest rate of $5\%$ per year simple interest. What is the value of the investment after this time?

State the formula needed and the value of each variable

We required final the value of the investment so we will use the formula $A=P(1+rt)$ with:

$P=1500$
$r=0.05$
$t=4$

Substitute the values into the formula.

Substituting these values into the simple interest formula $A=P(1+rt),$ we get:

$A=1500(1+0.05\times{4})$

Solve the equation.

\begin{aligned} &A=1500(1+0.20)\\\\ &A=1500(1.20)\\\\ &A=1500\times{1.2}\\\\ &A=\pounds1800. \end{aligned}

Example 3: Simple interest – finding the final amount after a decrease

A car is bought for $£10,000$ and loses $9\%$ of its value per annum, simple interest. What is the value of the car after $8$ years?

State the formula needed and the value of each variable

Here we use the formula $A=P(1+rt)$ with:

$P=10000$
$r=-0.09$
$t=8$

Substitute the values into the formula.

Substituting these values into the simple interest formula $A=P(1+rt),$ we get:

$A=10000(1-0.09\times{8})$

Solve the equation.

\begin{aligned} &A=10000(1-0.72)\\\\ &A=10000(0.28)\\\\ &A=10000\times{0.28}\\\\ &A=\pounds2800. \end{aligned}

Example 4: Simple interest – different time scale

$£7600$ is borrowed for $2$ years on a credit card. The cost of borrowing is a $1\%$ interest payment per month simple interest for the life of the loan. What is the total cost to pay off after this time?

State the formula needed and the value of each variable

Here we use the formula $A=P(1+rt)$ with:

$P=7600$
$r=0.01$
$t= 2 \times 12 = 24$ (remember there are $12$ months in $1$ year)

Substitute the values into the formula.

Substituting these values into the simple interest formula $A=P(1+rt),$ we get:

$A=7600(1+0.01\times{24})$

Solve the equation.

\begin{aligned} &A=7600(1+0.24)\\\\ &A=7600(1.24)\\\\ &A=7600\times{1.24}\\\\ &A=\pounds{9424}. \end{aligned}

Example 5: Simple interest – different percentages

A house is currently valued at $£175,000.$ For the first $3$ years, the value of the house increases by the rate of simple interest of $0.2\%$ per annum. For the following $4$ years, the value of the house decreases in value by a simple interest rate of $0.18\%$ per annum. Calculate the value of the house after these $7$ years.

State the formula needed and the value of each variable

Here we use the formula

$A=P\left(1+r_{1} t_{1}+r_{2} t_{2}\right)$

$P=175,000$
$r_{1}=0.002$
$t_{1}=3$
$r_{2}=-0.0018$
$t_{2}=4$

Substitute the values into the formula.

Substituting these values into the simple interest formula

$A=P\left(1+r_{1} t_{1}+r_{2} t_{2}\right),$

we get:

$A=175,000(1+0.002\times{3}-0.0018\times{4})$

Solve the equation.

\begin{aligned} &A=175,000(1+0.006-0.0072)\\\\ &A=175,000(0.9988)\\\\ &A=175,000\times{0.9988}\\\\ &A=\pounds{174,790}. \end{aligned}

Common misconceptions

Applying the incorrect formula to the question

This is a very common mistake where the simple interest on an amount is calculated instead of using the compound interest formula.

Incorrect percentage change due to different time scales

In example 4 “ $£7600$ is invested for $2$ years at $1\%$ per month simple interest. What is the value of the investment after this time?”, the value of t is written as $2$ and not $24.$

State the formula needed and the value of each variable

Here we use the formula $A=P(1+rt)$ with:

$P=7600$
$r=0.01$
$t= 2$

Substitute the values into the formula.

Substituting these values into the simple interest formula $A=P(1+rt),$ we get:

$A=7600(1+0.01\times{2})$

Solve the equation.

\begin{aligned} &A=7600(1+0.02)\\\\ &A=7600(1.02)\\\\ &A=7600\times{1.02}\\\\ &A=\pounds{7752}. \end{aligned}

Using the incorrect value for the percentage change

Using the percentage as the value for $r$ (therefore not dividing the percentage by $100$ ). For example when using simple interest to increase $£100$ by $2\%$ for $5$ years, this calculation is made:

\begin{aligned} &A=100(1+2\times{5})\\\\ &A=100\times{11}\\\\ &A=\pounds{1100!} \end{aligned}

Is the value increasing or decreasing?

If a value is depreciating (going down), the value of r is negative whereas it is incorrectly used as a positive and so the answer will be larger than the original amount.

Simple Interest practice questions

£551.20

£485.06

£529.15

£507.10

The original price was $£689$ . The sale price was $80$ % of this. $80$ % of $£689$ is $£551.20$ . The value of the laptop has decreased by $4$ % of $£551.20$ per year for three years. We can work out $4$ % of $551.20$ and multiply by $3$ ; this is $£66.14$ . Subtracting this from the purchase price gives the new value.

£1068.93

£801.70

£44.54

£267.23

The amount to be paid back is given by $1.07\times999=1068.93$
Six months is a quarter of the finance period, so a quarter of the full amount will have been spent on the saxophone, therefore $\frac{1}{4}\times1068.93=267.23$

 $Shop$   $A$

 $Shop$   $B$

Shop A: After a $10$ % reduction, the t-shirt will cost $£14.40$
Shop B: The $8$ % reduction means the t-shirt will be $£14.72$ , with the additional $2$ % reduction making the price $£14.43$

£7459.47

£7694.42

£8751.66

£8046.83

The initial amount is $7342$ , the simple interest rate is $0.4$ % and the number of months is $48$ , which results in $7342(1+0.004\times48)$

£366,413

£376,465

£497,080

£387,430

The initial amount is $365500$ , the simple interest rate is $0.25$ % and the number of years is $12$ , which results in $365500(1+0.0025\times12)$

Simple Interest GCSE Exam Questions:

1.(a) $£850$ is invested for $6$ years at $2\%$ simple interest per year. Work out the total interest earned?

(b) The interest rate changes to $0.15\%$ simple interest per month. How does this affect the final value of the investment over the same time period of $6$ years?

(6 marks)

Show answer

(a)

850 \times 0.02 \times 6

(1)

£102

(1)

(b)

0.15 \times 12 \times 6 = 10.8\%

(1)

850 \times (0.108)

(1)

£91.80

(1)

Decreased by $£10.20$ over $6$ years

(1)

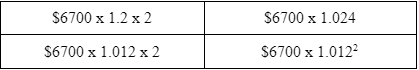
2. (a) Freya invests $\$6700$ for $2$ years. The simple interest rate is $1.2\%$ per year. Which calculation below works out the total value after $2$ years? Circle your answer.

(b) Euan invests $\$6400$ for $2$ years. The simple interest rate is

$1.4\%$ for the first year

$1.1\%$ for the second year.

Whose investment is worth more after $2$ years and by how much?

You must show your working.

(4 marks)

Show answer

(a) $\$6700 \times 1.024$

(1)

(b)

1.4+1.1=2.5\%

(1)

\$6700 \times (0.001)

(1)

\$6.70

(1)

3. (a) Lauren places $£300$ into a new bank account with a simple interest rate of $3\%.$ After the second year, she withdraws $£30.$ How much money would she have after $5$ years?

(b) The interest rate after $5$ years halves. How many years will it now take Lauren to save $£400?$

(5 marks)

Show answer

(a)

300 \times (1+0.03 \times 5)-30

(1)

£315

(1)

(b)

400-315=£85

(1)

85 \div 4.725

(1)

$18$ years

(1)

Learning checklist

You have now learned how to:

Solve problems involving percentage change, including: percentage increase, decrease and original value problems and simple interest in financial mathematics

Set up, solve and interpret the answers in growth and decay problems, including compound interest {and work with general iterative processes}

The next lessons are

Still stuck?

Prepare your KS4 students for maths GCSEs success with Third Space Learning. Weekly online one to one GCSE maths revision lessons delivered by expert maths tutors.