How To Simplify Radicals

[FREE] Fun Math Games & Activities Packs

Always on the lookout for fun math games and activities in the classroom? Try our ready-to-go printable packs for students to complete independently or with a partner!

DOWNLOAD FREE

In order to access this I need to be confident with:

Laws of exponents Factors Factor tree

Math resources Algebra

Radicals

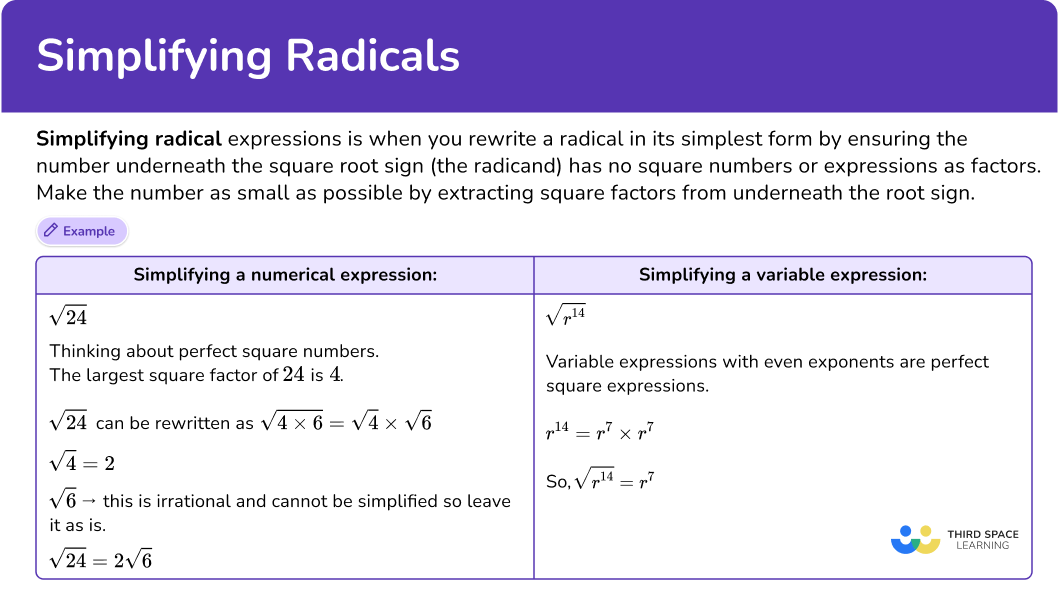
How to simplify radicals

Here you will learn about how to simplify radicals including the definition of a radical, the rules of radicals and how to write radicals in their simplest form.

Students first learn how to work with radicals when they learn about square roots and cube roots in $8$ th grade and expand the knowledge as they progress through high school math.

What is simplifying radicals?

Simplifying radicals or simplifying radical expressions is when you rewrite a radical in its simplest form by ensuring the number underneath the square root sign (the radicand) has no square numbers as factors. Make the number as small as possible by extracting square factors from underneath the root sign.

The radical sign (also known as square root symbol) is $→ \sqrt{\;\;\;}.$

You can use the three properties of radicals, which can be derived from the laws of exponents (powers) to help you to simplify radicals:

See also: Laws of exponents

Multiplying radicals: $\sqrt{m} \times \sqrt{n}=\sqrt{m n}$

Dividing radicals: $\sqrt{m} \div \sqrt{n}=\sqrt{\cfrac{m}{n}}=\cfrac{\sqrt{m}}{\sqrt{n}}$

In the quotient, $\sqrt{m}$ is in the numerator and $\sqrt{n}$ is in the denominator.

Squaring radicals: $\sqrt{m} \times \sqrt{m}=\sqrt{m^2}=m$

For example, let’s simplify $\sqrt{20}.$

$20$ is not a perfect square number, so let’s think about possible perfect square factors of $20.$

$4$ is a factor of $20$ and a perfect square number.

$4$ is a perfect square because $2 \times 2 =4.$

$\sqrt{20}$ can be written as $\sqrt{4 \times 5}.$

\sqrt{4 \times 5}=\sqrt{4} \times \sqrt{5}

\sqrt{4}=2

So, $\sqrt{20}$ is simplified to be $2 \sqrt{5}.$

\sqrt{20}=2 \sqrt{5}

Let’s simplify a radical algebraic expression.

\sqrt{50 x^3}

First, think of the perfect square factors of $50.$

$25$ is a factor of $50$ and it is a perfect square number.

Next, look at the exponent. If it is divisible by $2$ it is considered a perfect square if not, it is not a perfect square. $3$ is the exponent which means it is not a perfect square.

Rewrite $x^3$ where one factor is a perfect square.

In this case, $x^3$ can be rewritten as $x^2 \times x.$

\begin{aligned}&\begin{aligned}& \sqrt{50 x^3}=\sqrt{25 \times 2 \times x^2 \times x} \\\\ & \sqrt{25} \times \sqrt{2} \times \sqrt{x^2} \times \sqrt{x} \\\\ & \sqrt{25}=5\end{aligned}\\\\ &\sqrt{x^2}=x\end{aligned}

$\sqrt{50 x^3}$ simplifies to be $5 x \sqrt{2 x}.$

\sqrt{50 x^3}=5 x \sqrt{2 x}

What is simplifying radicals?

Common Core State Standards

How does this relate to $8$ th grade math and high school math?

Grade 8 – Expressions and Equations (8.EE.A.2)
Use square root and cube root symbols to represent solutions to equations of the form $x^2 = p$ and $x^3 = p,$ where $p$ is a positive rational number. Evaluate square roots of small perfect squares and cube roots of small perfect cubes. Know that $\sqrt{2}$ is irrational.

High School Number and Quantity – The Real Number System (HSN-RN.B.3)
Explain why the sum or product of two rational numbers is rational; that the sum of a rational number and an irrational number is irrational; and that the product of a nonzero rational number and an irrational number is irrational.

[FREE] Algebra Check for Understanding Quiz (Grade 6 to 8)

Use this quiz to check your grade 6 – grade 8 students’ understanding of algebra. 10+ questions with answers covering a range of 6th to 8th grade algebra topics to identify areas of strength and support!

DOWNLOAD FREE

[FREE] Algebra Check for Understanding Quiz (Grade 6 to 8)

DOWNLOAD FREE

In order to simplify a radical:

Find the largest square number that is a factor of the number under the root.
Rewrite the radical as a product of this square number and another number, then evaluate the root of the square number.
Write the simplified answer.

Simplifying radicals examples

Example 1: simple integer

Simplify the radical.

\sqrt{8}

Find the largest square number that is a factor of the number under the root.

Thinking about some of the perfect square numbers.

\begin{aligned} &1 = 1 \times 1 \\ &4 = 2 \times 2 \\ &9 = 3 \times 3 \\ &16 = 4 \times 4 \\ &25 = 5 \times 5 \\ &36 = 6 \times 6\end{aligned}

$4$ is a perfect square number and a factor of $8.$

2 \times 4 = 8

2Rewrite the radical as a product of this square number and another number, then evaluate the root of the square number.

\sqrt{8}=\sqrt{2 \times 4}=\sqrt{2} \times \sqrt{4}

$\sqrt{2} →$ This is irrational and cannot be simplified, so leave it as is.

$\sqrt{4} →$ This expression can be simplified. $\sqrt{4}=2$

3Write the simplified answer.

\sqrt{8}=2 \sqrt{2}

Example 2: simple integer

Simplify the radical.

\sqrt{45}

Find the largest square number that is a factor of the number under the root.

Thinking about some of the perfect square numbers.

$\begin{aligned} &1 = 1 \times 1 \\ &4 = 2 \times 2 \\ &9 = 3 \times 3 \\ &16 = 4 \times 4 \\ &25 = 5 \times 5 \\ &36 = 6 \times 6 \end{aligned}$

$9$ is a perfect square number and a factor of $45.$

Rewrite the radical as a product of this square number and another number, then evaluate the root of the square number.

\sqrt{45}=\sqrt{5 \times 9}=\sqrt{5} \times \sqrt{9}

$\sqrt{5} →$ This is irrational and cannot be simplified, so leave it as is.

$\sqrt{9} →$ This expression can be simplified. $\sqrt{9}=3$

Write the simplified answer.

\sqrt{45}=3 \sqrt{5}

Example 3: larger integer

Simplify the radical.

\sqrt{240}

Find the largest square number that is a factor of the number under the root.

Thinking about some of the perfect square numbers.

$\begin{aligned} &1 = 1 \times 1 \\ &4 = 2 \times 2 \\ &9 = 3 \times 3 \\ &16 = 4 \times 4 \\ &25 = 5 \times 5 \\ &36 = 6 \times 6 \\ &49 = 7 \times 7 \\ &64 = 8 \times 8 \\ &81 = 9 \times 9 \end{aligned}$

$4$ and $16$ are perfect square numbers and factors of $240.$

Use the perfect square factor that is larger. So, in this case use $16.$

$16 \times 15 = 240$

Rewrite the radical as a product of this square number and another number, then evaluate the root of the square number.

\sqrt{240}=\sqrt{15 \times 16}=\sqrt{15} \times \sqrt{16}

$\sqrt{15} →$ This is irrational and cannot be simplified, so leave it as is.

$\sqrt{16} →$ This expression can be simplified. $\sqrt{16}=4$

Write the simplified answer.

\sqrt{240}=4 \sqrt{15}

How to simplify radicals with variables

In order to simplify a radical with a variable:

If the exponent is even, go to step $\bf{3}$ . If the exponent is odd, go to step $\bf{2}$ .
Subtract $\bf{1}$ from the exponent and rewrite the expression as a product.
Even exponents are perfect squares, take half of the exponent.
Write the simplified answer.

Example 4: simplify a variable expression with an even exponent

Simplify the radical.

\sqrt{x^{10}}

If the exponent is even, go to step $\bf{3}$ . If the exponent is odd, go to step $\bf{2}$ .

The exponent is $10$ which is an even number.

Subtract $\bf{1}$ from the exponent and rewrite the expression as a product.

The exponent is even.

Even exponents are perfect squares, take half of the exponent.

Even exponents are perfect squares. In this case, $x^{10}=x^5 \times x^5$

So, to take the square root, you can just divide the exponent by $2.$

Write the simplified answer.

\sqrt{x^{10}}=x^5

Example 5: simplify a variable expression with an odd exponent

Simplify the expression.

\sqrt{y^9}

If the exponent is even, go to step $\bf{3}$ . If the exponent is odd, go to step $\bf{2}$ .

The exponent is $9$ which is an odd number.

Subtract $\bf{1}$ from the exponent and rewrite the expression as a product.

In this case, you can rewrite the expression, $y^9$ to be, $y^1 \times y^8.$

$y^8$ is a perfect square expression because, $y^8=y^4 \times y^4.$

$\sqrt{y^1 \times y^8}=\sqrt{y} \times \sqrt{y^8}$

$\sqrt{y} →$ This expression is considered to be irrational and cannot be simplified so leave as is.

$\sqrt{y^8} →$ This expression can be simplified. $\sqrt{y^8}=y^4$

Even exponents are perfect squares, take half of the exponent.

The original expression had an odd exponent.

Write the simplified answer.

\sqrt{y^9}=y^4 \sqrt{y}

Example 6: simplify an expression with two variables

Simplify the radical.

\sqrt{x^6 y^3}

If the exponent is even, go to step $\bf{3}$ . If the exponent is odd, go to step $\bf{2}$ .

In this case, you have two variables. $x^6$ has an even exponent and $y^3$ has an odd exponent.

Subtract $\bf{1}$ from the exponent and rewrite the expression as a product.

In this case, you can rewrite $y^3$ the expression to be, $y^1 \times y^2.$

$y^2$ is a perfect square expression because, $y^2=y^1 \times y^1.$

$\sqrt{y^1 \times y^2}=\sqrt{y} \times \sqrt{y^2}$

$\sqrt{y} →$ This expression is considered irrational and cannot be simplified so leave as is.

$\sqrt{y^2} →$ This expression can be simplified. $\sqrt{y^2}=y^1$

Even exponents are perfect squares, take half of the exponent.

$x^6$ has an even exponent. So, $x^6$ is a perfect square because $x^3 \times x^3=x^6.$

$\sqrt{x^6}=x^3$

Write the simplified answer.

\sqrt{x^6 y^3}=x^3 y \sqrt{y}

Teaching tips for how to simplify radicals

Be sure to review the concept of perfect square numbers with students before simplifying radicals.

Demonstrate alternate strategies for simplifying the number under the radical, such as prime factorization, for students that struggle with math facts.

Instead of giving students worksheets to practice, have them engage in game playing such as scavenger hunts or using digital platforms.

Easy mistakes to make

Incorrectly rewriting the number under the square root sign (the radicand) as a product of any two factors
One of these factors must be a square number in order for you to be able to simplify the radical. For example, rewriting $\sqrt{48}$ as $\sqrt{6 \times 8}$ instead of $\sqrt{16 \times 3}.$

Not simplifying fully
Always check that there are no square factors of the number under the root. For example, $\sqrt{32}=2 \sqrt{8}$ instead of fully simplifying to be $4 \sqrt{2}.$

Thinking that only variables that have perfect square exponents are perfect squares
For example, thinking that $\sqrt{x^9}=x^3$ instead of $\sqrt{x^9}=\sqrt{x} \times \sqrt{x^8}=x^4 \sqrt{x}.$

Radicals
Adding radicals
Subtracting radicals
Rationalize the denominator
Multiplying radicals
Dividing radicals

Practice how to simplify radicals questions

4

8

256

32

$16$ is a perfect square number because $4 \times 4 = 16.$ So, $\sqrt{16}=4.$

10

5 \sqrt{4}

2 \sqrt{5}

4 \sqrt{5}

Thinking about the perfect square numbers, $4$ is the largest perfect square factor of $20.$ So, rewrite the expression to be, $\sqrt{20}=\sqrt{4 \times 5}=\sqrt{4} \times \sqrt{5}.$

$\sqrt{4} →$ This is a perfect square so it can be simplified to be $\sqrt{4}=2.$

$\sqrt{5} →$ This is irrational and cannot be simplified.

So, $\sqrt{20}=2 \sqrt{5}.$

3 \sqrt{12}

6 \sqrt{3}

3 \sqrt{6}

4 \sqrt{27}

The largest perfect square factor of $108$ is $36.$

So, rewrite the expression to be, $\sqrt{108}=\sqrt{36 \times 3}=\sqrt{36} \times \sqrt{3}.$

$\sqrt{36} →$ This is a perfect square so it simplifies to be $\sqrt{36}=6.$

$\sqrt{3} →$ This is irrational so it cannot be simplified.

\sqrt{108}=6 \sqrt{3}

y^5

y^{12.5}

\sqrt{y^5}

y^{12} \sqrt{y}

$y^{25}$ has an odd exponent so it is not a perfect square expression. Subtracting $1$ from the exponent and rewriting to the expression as a product forces part of the expression to be simplified.

y^{25}=y \times y^{24}

So, $\sqrt{y^{25}}=\sqrt{y \times y^{24}}=\sqrt{y} \times \sqrt{y^{24}}$

$\sqrt{y} →$ This is irrational and cannot be simplified.

$\sqrt{y^{24}} →$ This is a perfect square expression and can be simplified to be $y^{12}.$

The simplified answer is:

\sqrt{y^{25}}=y^{12} \sqrt{y}.

r^3 s \sqrt{r}

r^3 s^2 \sqrt{r}

s \sqrt{r^7}

r s \sqrt{r}

To simplify the expression, $\sqrt{r^7 s^2},$ look at each variable separately.

\begin{aligned}& \sqrt{r^7 s^2}=\sqrt{r^7} \times \sqrt{s^2} \\\\ & \sqrt{r^7}=\sqrt{r \times r^7}=\sqrt{r} \times \sqrt{r^6} \end{aligned}

$\sqrt{r} →$ This is irrational and cannot be simplified.

$\sqrt{r^6} →$ This is a perfect square expression and can be simplified to be $r^3.$

$\sqrt{s^2}$ is a perfect square expression because $s \times s=s^2.$

$\sqrt{s^2} →$ Simplifies to be $s.$

So, $\sqrt{r^7 s^2}=r^3 s \sqrt{r}.$

16 x

4 \sqrt{2 x^8}

4 x^4 \sqrt{2}

2 x^4 \sqrt{4}

Rewrite $\sqrt{32 x^8}$ to be $\sqrt{32} \times \sqrt{x^8}.$

Thinking about the perfect square numbers. $16$ is the largest perfect square factor of $32.$

\sqrt{32}=\sqrt{16} \times \sqrt{2}

$\sqrt{16} →$ This is a perfect square number and simplifies to be 4.

$\sqrt{2} →$ This is irrational and cannot be simplified.

$\sqrt{x^8} →$ The exponent is even so this is a perfect square expression. It simplifies to be $x^4$ (take half of the original exponent).

The simplified answer is: $\sqrt{32 x^8}=4 x^4 \sqrt{2}.$

How to simplify radicals FAQs

Can you simplify radical expressions that have decimals?

There are decimals that are perfect squares such as $0.25.$ So, is a rational expression $\sqrt{0.25}=0.5.$

Can radical expressions have rational exponents?

Rational exponents can be used to rewrite radical expressions. For example $\sqrt{x}=x^{\frac{1}{2}}$ where $\cfrac{1}{2}$ is the fractional exponent representing square root. You will learn how to convert between radical form and exponential form in algebra.

Can you take the square root of polynomials?

Yes, there are polynomials that are perfect square expressions. For example, $\sqrt{(x+3)^2}=x+3.$ You work with simplifying polynomials under radicals in algebra $2$ and precalculus.

Are there radical equations?

Yes, there are radical equations that you will learn how to solve in algebra $1$ and algebra $2$ classes.

Can you take the square root of prime numbers?

Square roots of prime numbers are considered irrational and cannot be simplified.

What is a complex number?

A complex number is in the form of $a+b i$ where $i=\sqrt{-1}.$ You will work with complex numbers and their conjugates in precalculus.

Can you take the square root of negative numbers?

The square root of negative numbers is considered to be imaginary numbers. For example, $\sqrt{-4}=2 i.$

Can you simplify cube roots?

The step-by-step approach to simplifying cube roots is very similar to simplifying square roots. You will look for perfect cube factors of the number instead of perfect square factors of the number.

The next lessons are

Radical functions
Vectors
Trig graphs
Quadratic Equations

Still stuck?

At Third Space Learning, we specialize in helping teachers and school leaders to provide personalized math support for more of their students through high-quality, online one-on-one math tutoring delivered by subject experts.

Each week, our tutors support thousands of students who are at risk of not meeting their grade-level expectations, and help accelerate their progress and boost their confidence.