What Are Angles? Acute, Obtuse And Right Angles: Explained For Teachers, Parents and Kids

Here you can find out about different angles, when they are taught in elementary and middle school, and how you can help children to understand them as part of their math learning at home.

Students informally begin their learning of angles in lower elementary when identifying shapes. While they would not be expected to use the names of the angles at this stage, it does set the foundation for the vocabulary of right angles, for example.

Students will fully explore angles in upper elementary where they will have to draw them accurately with a protractor and identify them from sight. 

What is an angle?

An angle is the space between two intersecting lines where they join at a point. The size of the angle is measured in degrees.

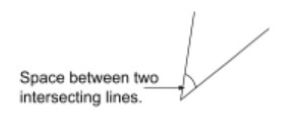
Depending on the numerical range of ‘space’ between the sets of intersecting lines, the angles can be classified into different types of angles: acute, obtuse, or right angles.

Types of angles

What is a right angle?

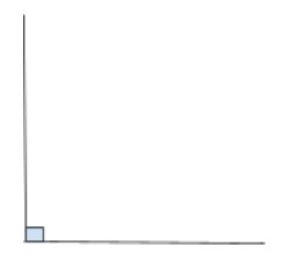
A right angle is created when two intersecting lines meet at exactly 90 degrees. This is often regarded as a quarter turn as four quarter turns would result in a whole turn. A right angle is annotated between two intersecting lines through the use of a square.

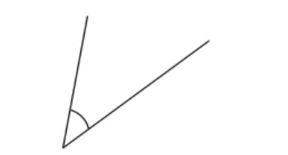
What is an acute angle?

An acute angle is where the space between two intersecting lines range between 1 and 89 degrees.

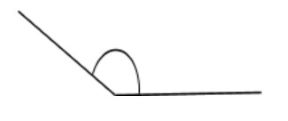
What is an obtuse angle?

An obtuse angle is where the space between two intersecting lines ranges between 91 and 179 degrees.

What is a straight line?

A straight line is an angle that is exactly 180 degrees. It represents a change in the opposite direction. Note that supplementary angles are two angles which add up to 180 degrees.

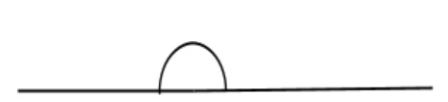
What is a full rotation?

A full rotation represents a 360 degree turn where the space between an intersecting line returns to its original position.

When do children learn about angles in school?

Schools following Common Core:

Informally, students begin learning about angles in 1st grade where they are taught about 2D and 3D shapes. Students are expected to compose 2D shapes, such as half-circles and quarter-circles.

Although the term ‘right angle’ wouldn’t be used to describe the quarter-circle, this would be a student’s first exposure to this angle.

2nd Grade Angles 

This is built on in 2nd grade when students are expected to ‘recognize and draw shapes having specified attributes, such as a given number of angles, or a given number of equal faces.’ 

At this stage, students would not be expected to know the measurement of the angle in degrees or know the formal definition of what an ‘angle is’. 

3rd Grade Angles

In 3rd grade, students apply the learning from the previous year groups to describe properties of shape. They will be expected to:

• Understand that shapes in different categories (e.g., rhombuses, rectangles, and others) may share attributes (e.g., having four sides), and that the shared attributes can define a larger category (e.g., quadrilaterals). 
• Recognize rhombuses, rectangles, and squares as examples of quadrilaterals, and draw examples of quadrilaterals that do not belong to any of these subcategories.

It is at this point that a formal definition of ‘angle’ is likely to be introduced but they will not need to know the technical name of acute or obtuse angles at this time. 

4th Grade Angles

In 4th grade, students will extend their knowledge of angles by learning the terms acute angle and obtuse angle. The expectation in what students must know and be able to do with angles increases greatly in 4th grade where they are expected to know the following:

• Recognize angles as geometric shapes that are formed wherever two rays share a common endpoint, and understand concepts of angle measurement:
• Measure angles in whole-number degrees using a protractor. Sketch angles of specified measure. 
• Draw points, lines, line segments, rays, angles (right, acute, obtuse), and perpendicular and parallel lines. Identify these in two-dimensional figures.
• Classify two-dimensional figures based on the presence or absence of parallel or perpendicular lines, or the presence or absence of angles of a specified size. Recognize right triangles as a category, and identify right triangles.
• Recognize angle measure as additive. When an angle is decomposed into non-overlapping parts, the angle measure of the whole is the sum of the angle measures of the parts. 
• Solve addition and subtraction problems to find unknown angles on a diagram in real world and mathematical problems, e.g., by using an equation with a symbol for the unknown angle measure.

5th grade Angles

Finally, in 5th grade, there is greater emphasis on the application of knowledge of angles to do the following:

• Understand that attributes belonging to a category of two dimensional figures also belong to all subcategories of that category. For example, all rectangles have four right angles and squares are rectangles, so all squares have four right angles.
• Classify two-dimensional figures in a hierarchy based on properties.
• Compare and classify geometric shapes based on their properties and sizes and find unknown angles in any triangles, quadrilaterals, and regular polygons

Other schools:

For school districts and schools that follow standards other than the Common Core Math State Standards, these concepts may be taught in a different order or at different times. Refer to the standards set by your school to know when these concepts are discussed in your child’s school. 

How does this relate to other areas of math?

Angles relate to other areas of Euclidean geometry such as shape. A prerequisite for understanding and calculating angles is to have fluent arithmetic skills. Particularly when subtracting multiple numbers from 90, 180 or 360.

How does this relate to real life?

Knowledge of angles is important as its application has many uses. Much of the construction industry relies on ensuring that buildings are structurally sound using their knowledge of angles. 

Pool players rely on angles to predict how two balls will react. Analog clocks can show every angle up to 360 degrees! Angles are all around us!

Angles worked examples

1) Which angles are less than a right angle?

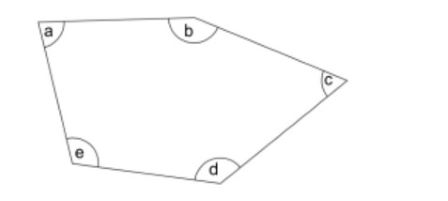
To answer this question students need to know that a right angle is 90 degrees and be able to visualize what this looks like as the picture is not drawn to scale.

By having a strong mental model of what a right angle looks like, students will be able to deduce that angles ‘a’ and ‘c’ are less than a right angle.

2) This is a three-quarter turn. How many degrees is ‘x’?

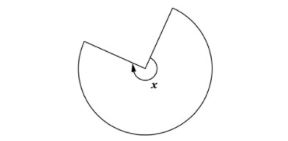
For this question, students would be expected to know that a three-quarter-degree turn is equal to 270 degrees. Some students may know this fact by heart.

Some students may multiply 90 (one-quarter turn) by 3 to get 270 degrees or subtract 90 degrees from 360 (a full rotation or 4 quarters).

3) Find the missing angle ‘y

This question requires students to apply their knowledge and understanding of various aspects of their learning about angles and arithmetic. They must know that angles in a straight line add up to 180 degrees.

They must also deduce that a square at the vertex of two lines represents a right angle and that this means 90 degrees. They will have to use this knowledge to calculate y. They may do this in the following way:

65 + 90 = 155

180 – 155 = 25

y = 25

Angles practice questions

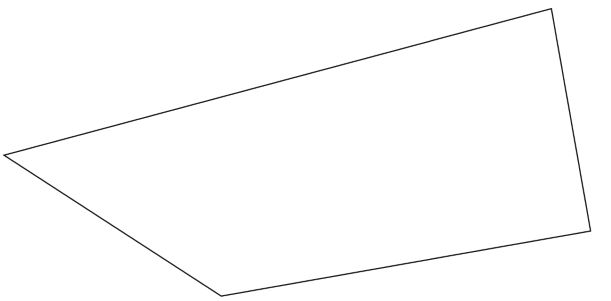
1. Find the obtuse angles.

2. For each shape, write how many right angles it has.

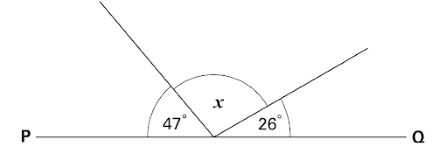
3. Calculate the size of ‘x’.

4. Use a protractor to draw an acute angle.

5. Use a protractor to draw an angle that measures 105 degrees.

Wondering about how to explain other key math vocabulary to your children? Check out our Math Dictionary For Kids, or try these other blogs:

• What is Math Mastery?

Do you have students who need extra support in math?
Give your students more opportunities to consolidate learning and practice skills through personalized math tutoring with their own dedicated online math tutor.

Each student receives differentiated instruction designed to close their individual learning gaps, and scaffolded learning ensures every student learns at the right pace. Lessons are aligned with your state’s standards and assessments, plus you’ll receive regular reports every step of the way.

Personalized one-on-one math tutoring programs are available for:
– 2nd grade tutoring
– 3rd grade tutoring
– 4th grade tutoring
– 5th grade tutoring
– 6th grade tutoring
– 7th grade tutoring
– 8th grade tutoring

Why not learn more about how it works?

The content in this article was originally written by primary school lead teacher Neil Almond and has since been revised and adapted for US schools by elementary math teacher Christi Kulesza.