Common Elementary Math Misconceptions & How To Fix Them
Expert advice on the most common math misconceptions, errors and myths in elementary school mathematics, and how to manage, overcome them and prevent them from spreading into middle school.
If I did a survey about which areas of math children find the trickiest, I bet we’d all pretty much agree there are some mathematical concepts, such as fractions, that some children just seem to find really difficult.
What’s even more perplexing is when others in the same class grasp these math concepts almost effortlessly, and others really struggle.
From a teacher’s point of view, it can be very frustrating. You think you have really planned something well, explained it really clearly, and given children practical materials to make the abstract more concrete…and yet the penny does not seem to drop. Why is this? And what can we do about it?
From my own experience, I bet that, in this imaginary survey, the top three topics to come up would be fractions, place value and decimals. Some children can do addition, but find subtraction really hard.
Money often confuses younger children. For older children, square numbers get confused with multiplying by two.
Overcoming common math misconceptions: What cognitive science can tell us
In order to understand and overcome the math problems these topics present, we need to turn to what cognitive science tells us about how we learn things.
More specifically, we need to understand how students learn the wrong things, the “misconceptions” that can be difficult to eradicate.
Where cognitive science can really help us understand misconceptions is in its account of how we build “schemas”.
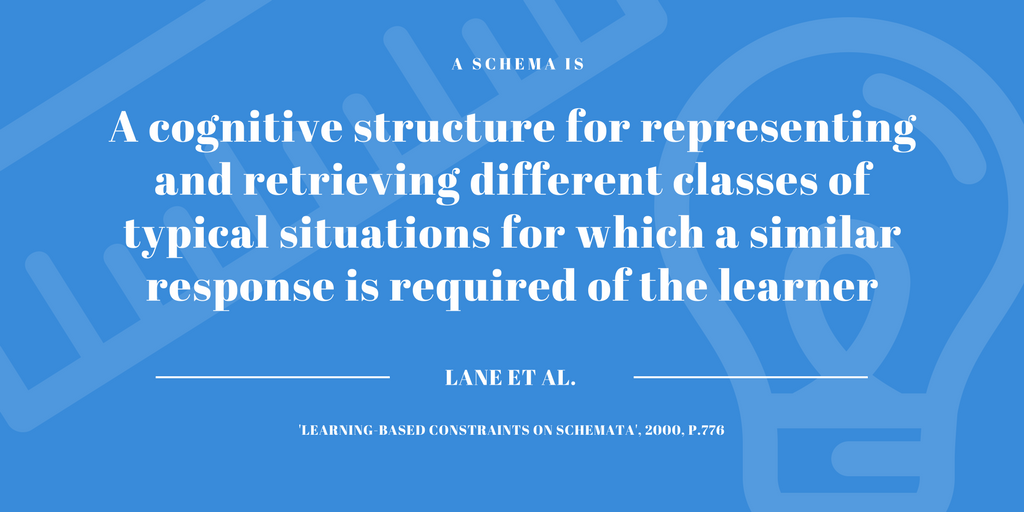
What is a schema?
A schema is “a cognitive structure for representing and retrieving classes of typical situations for which a similar response is required of the learner” (Lane et al., 2000, p. 776). Essentially, a schema is a mental framework learners build as they organize and connect together information which they believe is related.
The obvious benefit to schemas is that they make learning new information much less strenuous. They help us build on what we already know, linking any new information back to the big, connected framework of what we have previously linked together.
A good example in elementary math is how children can understand thousands quite easily, but only after they have understood hundreds, tens, and ones.
This is because the new learning follows the same basic pattern as the old learning. The children have simply added the new information into their “schema” on how the number system operates.
This ability makes humans very successful as a species. It means we can rapidly adapt to new situations by recognising patterns from our existing knowledge.
To top it all off, this ability to slot new information into our existing schema is fairly automatic. We are able to easily do it, without the need for deep, complex thought.
The problem with schemas & the math misconceptions they cause
However, this great strength in how we learn is also our Achilles’ heel. Having carefully crafted our schema, we go to great pains to fit anything new into it, even if it doesn’t really fit. Our minds will happily distort new information to fit our schema, rather than try to create a new schema.
Even if it directly contradicts the assumptions built into our existing schema. And because the process is semi-subconscious, we can be almost unaware that it is happening! Our desire to re-interpret new pieces of information and shoe-horn them into our pre-existing schemas is the stuff misconceptions are made of.
And because operating our schemas happens at a semi-subconscious level, these kinds of misconceptions are highly resistant to new learning. Once we understand this, the startling thing is not why some children have misconceptions but rather, why some children don’t!
Let’s examine a really common example of this.
Common math misconceptions in fractions
Many children find fractions particularly hard. Though frustrating, the reason for this becomes obvious once you consider just how different fractions are from the kinds of numbers children have worked with before.
As “experts” who “get” fractions, we forget quite how different fractions actually are.
Most misconceptions in fractions arise from the fact that fractions are not natural numbers.
Natural numbers are the positive whole numbers that we count with, e.g. 1, 2, 3, 97, 345, 234, 561 etc. These are the kinds of numbers children spend most of their time learning.
Crucially, this means that by the time we start to teach them fractions, they’ll have a highly developed schema about how natural numbers work.
Then we teach them fractions, which are rational numbers (ratio-nal numbers). Fractions work differently to natural numbers, but children will try to shoe-horn fractions into their pre-existing understanding, as they assume numbers all work the same way. But this is simply not the case.
To get to the root of how to fix this, we have to understand how natural and rational numbers differ.
Overcoming fractions misconceptions in math:
Understand number values
First of all, their natural and rational numbers differ with regards to value. With natural numbers one number is always linked to one value. For example, 3 is always 3, 26 is always 26 and so on. In fractions, value is a lot more complicated.
In a fraction, value is governed by the relationship between the two numbers in the fraction and the ‘whole’. The value of 3 in a fraction is not simply linked to the natural number 3.
Its value not only depends upon whether it is the numerator, the denominator or the whole, but also upon what the other numbers in the fraction are. It is the relationship between these three numbers that determines the value.
To make things even more complicated, it is not always obvious what the “whole” is. When we start teaching fractions, quite often we start off with examples where the “whole” is one.
For example, dividing shapes into halves and quarters assumes that the whole is “one shape”. Strictly speaking, we should say that this is half of one circle, not “half a circle” or just “half”.
After all, things are always “half” of something (even 0.5 is half of one) but we often miss that bit out, then wonder why children are confused. When we shift to examples where the example is not one, if we omit to flag that up and make a big deal of it, then children will get confused.
But, alas, it is even more complicated than that. For though the ‘whole’ is not always one, the final answer is almost always expressed with reference to one.
Confused? Let me share this anecdote.
A friend was doing some training about fractions and asked the teachers what 2 pizzas divided between 3 people would be.

There was a big argument. Some people said as you are dividing by 3 then everybody would get a third of the pizza whereas others said no, each person would get two thirds of the pizza.
They had to resort to cutting up paper plates before they were satisfied they understood who was right. Because, of course, both answers are correct.
Each person would get a third of the pizza (all the pizza, both of them) or, alternatively expressed, each person would get 2/3 of one pizza – which is how we usually express fractions.
In this case the “whole” is 2 as there are 2 pizzas, but we express the correct answer with reference to 1 pizza (each person gets 2/3 of a pizza). So you can see how a child’s pre-existing schema about number value struggles, when presented with something quite so different.
Sadly, that’s not the end of it, at all. Natural numbers also differ from rational numbers in other ways.
Overcoming fractions misconceptions in math: Understand number order
The second way natural and rational numbers differ is in terms of order. With natural numbers, order is simply linked to magnitude; each number in the counting sequence is one more than its predecessor. Whereas, ordering fractions does not work like that at all.
For a start, bigger denominators actually mean numbers are getting smaller (schema alert!), and that’s without even considering the added complications of considering the numerator as well.
Really, it’s no wonder so many children struggle to understand that bigger denominators do not mean bigger values. Even when we show them practically that 1/10 of a cake is a lot smaller than 1/3.
Their pre-existing schema can’t cope. Plus, as soon as the cake is no longer physically visible, the old schema reasserts itself and imposes its familiar way of understanding ordering onto fractions.
Of course, that’s before we consider equivalence (who said there wasn’t a reason teaching Math can be so frustrating at times!) With natural numbers, the number label indicates a fixed quantity. 2 is always 2. But with rational numbers, the same number label can represent different quantities.
For example, if I have half of $10 and you have half of $100, we do not have the same amount. Yet, we still talk about ‘a half.’ For some children, they assume that a half is a fixed quantity, because this is what their schema tells them.
Conversely, with fractions, different number labels can equal the same quantity (2/4 being the same as ½). This never happens with natural numbers where different number labels never equal the same quantity, so children struggle with it.
But there is still more! With fractions, in English at any rate, some of the number names are not obviously linked to their natural number counterparts. Half, third and a quarter do not call to mind 2, 3 and 4.
Then we have numbers like ‘fifth’ which might mean the ordinal position ‘fifth’ or might mean the fraction ‘⅕’.
The best ways to avoid common fractions misconceptions

Because of all these substantial differences between natural and rational numbers, to truly overcome fractions misconceptions, teachers need to:
- Resist the temptation to rush through material and just leave it on a knowledge organizer for young mathematicians to look over.
- Bear in mind the cognitive load inherent in what we are teaching (only try and overcome one potential misconception at a time)
- Take small steps – even when it seems easy
- Make sure all children really master these simple steps before moving on
- Use a model you can build on later
To see what this kind of teaching really looks like, watch this clip of a video I produced as part of Third Space Learning’s Math Masterclass series.
In it, I break the initial teaching of fractions down into very small steps, to avoid engendering the kind of misconceptions we are all familiar with.
The principles of how to overcome these misconceptions in fractions can then be applied to most other misconceptions in mathematics.
Common math misconceptions in place value
Naturally, once we appreciate the important role our prior learning plays in understanding new learning, we can identify pitfalls in other areas of math.
Then we can take more care to directly teach children why their previous learning needs adapting in any particular case.
For instance, some children struggle with place value because it contradicts their previously built schema about how the number system works.
They see each number as a number in its own right and not as a partition-able quantity, where the value of each digit is determined by its place.
So the number 10 might be seen not really as a 1 and a 0, but as a distinct sign – as if the two digits were joined up together.
Or maybe their schema is fixed on 1 meaning 1, so the idea that 1 can refer to 1 group of something doesn’t really take root. They might just cope with the idea of 1 group of apples, 2 groups of apples but balk when the groups are groups of a given number.
So the concept of 1 group of 10 can’t quite get traction. How can you have a group of a number? What does that even mean?
We all too readily ask children to understand the idea of groups, and particular groups of a number. If you think about it, the word order doesn’t help.
It would be more straightforward if we thought first about what’s in our group – oh yes, tens – and then thought about how many of these groups we had.
Even when children have incorporated an understanding of countable groups into their schema, there are more icebergs ahead.
Since place isn’t really important when we first teach children numbers, their schemas are resistant to the idea that, suddenly, place has a crucial meaning that alters the value of previously familiar digits.
Overcoming place value misconceptions: Understand ‘lots’ and ‘groups’
Rushing through early place value work without really spending quality time on the vital concept of 1 lot of 10 is a false economy.
Instead, spend time getting children to bundle straws into groups of 10 and draw circles around groups of 10 objects before using more abstract apparatus such as dienes or numicon – where the individual ones comprising the tens are less obvious.
As math teachers, it can be hard to remember this because lots of children grasp this easily. Meaning the important groundwork that many other children really need is not laid.
This is counterproductive and is likely to lead to misconceptions that will be resistant to correction later on. The trick is to get everybody getting it the first time!
Other common math misconceptions in elementary school
Decimals misconceptions in upper elementary math
Children think that 0.10 is more than 0.2 because their schema tells them that 10 is more than 2. The fact that we rarely tell them that 0.2 is also the same as 0.20 doesn’t help.
In fact, our whole teaching as zero as a place holder would be better if we explained that place value columns continue into infinity so in theory, we could write 20 as 020 or 000020 or 20.0000 or even 000000020.0000000.
If children were introduced to the concept of redundant zeros (alongside lots more work on place value) that would help.
Subtraction misconceptions in lower elementary math
We spend a lot of time teaching addition, then after that, teach subtraction, usually spending a lot less time. We are then surprised when children revert to adding when faced with a subtraction.
Overcome this common misconception by teaching the part, part, whole model; where the underlying model of both addition and subtraction are clear.
This is much better than rushing through, doing examples with bigger and bigger numbers. As it means children really understand how subtraction and addition fit together.
It is much better if a child really understands that 5-2=3 and how this relates to 3+2=5 and 5-3=2 and 2+3=5, than if a child can do equations with numbers to 20.
Money misconceptions in lower elementary math
We spent so much time in the early years building one to one correspondence. Then we introduce money, and this concept is comprehensively undermined. Personally I think our curriculum does this far too early and it would be better taught much later.
However, until that happens, we should really explicitly teach why the old rule doesn’t apply. Getting children to see how heavy, awkward and fiddly carrying lots of 1 cent coins around and showing them how coins are a helpful solution to this problem will probably help. So don’t start with 1 cent, start with 10 cent.
Square numbers in upper elementary math
The superscript 2 in square numbers is a recipe for confusion. Address this head on and use cubes so show 3 x 2 as well as 3², and discuss how these are different.
Don’t rush through this. Repeat for lots of different examples. This technique of showing non-examples as well as examples is a really good way of building deep conceptual understanding within a new schema.
Ultimately, course-correcting common math misconceptions can be a real uphill battle. But with a bit of time, patience, and understanding of what lies behind misconceptions, it is possible! Even better, teach the math conceptually to start with.
Read more
Do you have students who need extra support in math?
Give your students more opportunities to consolidate learning and practice skills through personalized math tutoring with their own dedicated online math tutor.
Each student receives differentiated instruction designed to close their individual learning gaps, and scaffolded learning ensures every student learns at the right pace. Lessons are aligned with your state’s standards and assessments, plus you’ll receive regular reports every step of the way.
Personalized one-on-one math tutoring programs are available for:
– 2nd grade tutoring
– 3rd grade tutoring
– 4th grade tutoring
– 5th grade tutoring
– 6th grade tutoring
– 7th grade tutoring
– 8th grade tutoring
Why not learn more about how it works?
The content in this article was originally written by primary headteacher Clare Sealy and has since been revised and adapted for US schools by elementary math teacher Christi Kulesza.




