7 Methods To Develop Conceptual Understanding in The Classroom
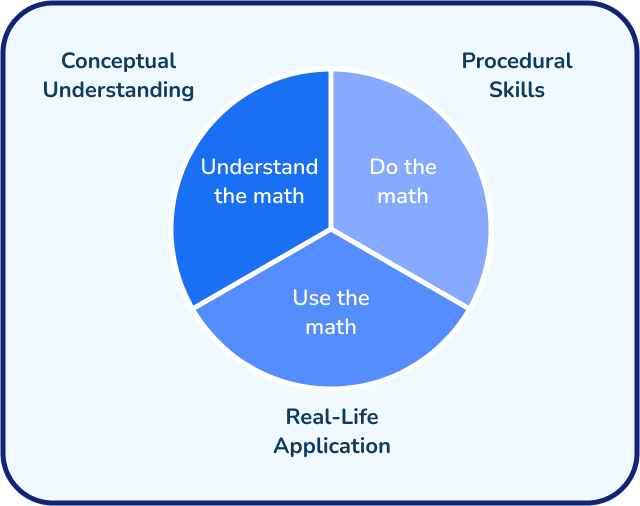
Conceptual understanding should act as the foundation of math instruction rather than rote memorization of procedural skills like algorithms and equations. It helps students move beyond understanding the “what” of mathematical operations to the “why” and “how”.
This article looks in-depth at why deep conceptual understanding is essential to ensuring students can build on and apply mathematical processes to more complex math problems later in their academic careers. It will also explore how educators can promote conceptual understanding of mathematical concepts in students through everyday classroom instruction.
What is conceptual understanding in math?
Conceptual understanding is a student’s ability to understand how to learn math and how math works. It allows students to apply learned concepts and procedures to new situations.
This level of deeper understanding extends beyond student understanding of procedural fluency – the process of following the steps of a mathematical equation.
While students may be able to arrive at the correct answer, procedural fluency requires strategic competence to understand each step of the process and how they are connected.
Encourage these skills early on in math learning so students can apply this problem-solving way of thinking as they learn more complex mathematical concepts.
The Ultimate Guide To Metacognition
Everything teachers need to know about math and metacognition, including practical tips and ideas to encourage students to think about their learning more explicitly and accelerate math growth.
Download Free Now!Productive disposition leads to conceptual understanding
To help students build confidence in conceptual understanding, teachers must model and encourage the tendency toward a productive disposition. This tendency occurs when students see mastering the root of mathematical concepts as worthwhile rather than moving through the motions of using equations and formulas without a more profound understanding.
Students can also demonstrate understanding through strategic competence
As students build mathematical confidence, they start to demonstrate their understanding through strategic competence. This is the ability to explain how they solved a math problem.
With strategic competence, students can demonstrate that they understand the concepts behind a mathematical idea through critical thinking, not just produce the correct answer.
Why do students need conceptual understanding in math?
Conceptual understanding in math is essential because it encourages a lasting and adaptable understanding of general math concepts. Students can build on these math concepts in novel situations to solve new problems.
Helps to apply procedural knowledge.
Through critical thinking, students can use their procedural knowledge of different mathematical ideas to solve more complex problems later in life. Flexible thinking skills also benefit students in other academic areas, such as English, supporting their growth as learners far beyond the math classroom.
Moves knowledge from working to long-term memory.
Memory and learning go hand-in-hand, especially when teaching students more complex mathematical skills. A strong memory allows students to retain the necssary foundational concepts, such as addition or subtraction, to build more advanced mathematical skills.
Students must be able to recall these concepts efficiently to solve more complex math problems. This knowledge is pulled from long-term memory rather than working memory.
Working memory is the part of a learner’s brain where they temporarily hold and manipulate information. The working memory actively processes information, responsible for tasks such as reasoning and problem-solving. This type of memory differs from long-term memory, where knowledge is stored and retrieved later after repetitive practice and heightened comprehension.
Cognitive load theory is the idea that the working memory can only hold onto a limited amount of information. This means that students must be able to access both the working and long-term memory to solve more complex math problems, through synthesis, application, and other higher-order thinking.
When students learn the “why” of a mathematical procedure, they are more equipped to process additional tasks, going from being able to solve a single math problem to long-term mathematical understanding.
Benefits of Conceptual understanding in math
When students demonstrate conceptual understanding in math, it can lead to a deeper sense of overall learning that can be applied to other contexts. Both in and out of the classroom.
Conceptual understanding builds problem-solving skills.
Conceptual understanding improves students’ problem-solving skills. Having a deep conceptual understanding helps them to apply the relevant problem-solving skills to new and novel situations.
For example, a student might stay up memorizing how to solve a specific mathematical expression for a test. If they don’t understand how they got to the answer, it is unlikely they will be able to answer the question or apply the mathematical expression if the test question is not formatted the same way as the practice problems.
Having conceptual understanding encourages the student and builds confidence. It is a consistent way to ensure students retain their learning and apply it elsewhere in their math education and the real world.
Students become more engaged and motivated about learning math.
When students can understand the learning in the math classroom, they are far more likely to be engaged and motivated about learning math.
Conceptual understanding helps students with more advanced problem-solving skills. Students feel much more prepared when they have a deeper understanding of how and why something works than if they can only solve a problem if it’s presented exactly the way they were taught.
What influences students’ conceptual understanding?
Many factors influence how well students grasp a conceptual understanding of mathematical concepts. Below are three factors that impact a student’s conceptual understanding and tips to overcome them.
High-quality instruction and the mathematical proficiency of teachers
Building conceptual understanding requires high-quality instruction from teachers proficient in the appropriate mathematical concepts. Use a range of teaching strategies to develop understanding and address individual student needs.
Teachers can’t effectively guide students in building conceptual knowledge if they don’t have the understanding themselves. School districts and administration should ensure teachers receive appropriate professional development so they are up-to-date with the relevant teaching standards.
Students’ prior knowledge and mathematical skills
While conceptual understanding is the end goal for learning a math concept, students also need prior knowledge of the mathematical skills needed to understand the new skill.
Students can’t truly understand more complex math problems if they don’t already have a strong understanding of any prerequisite math skills.
Teachers throughout the school, at all grade levels, must ensure students understand the taught mathematical concepts before moving on to new learning.
Third Space Learning’s one-on-one math tutoring sessions afford struggling students the opportunity to cover math concepts they haven’t fully grasped. Extra math instruction with highly-trained and experienced STEM tutors means the students who need extra help to catch up receive it without adding extra work for teachers.
Math sessions take place at a time suited to school schedules and are personalized to each student. Educators know their students best, so schools can request the topics they know their students need to catch up with, or our highly qualified tutors can choose the lessons based on assessment for learning to accelerate math learning quickly.
Effective instructional strategies
Students can’t build a conceptual understanding of math without effective learning design and instructional strategies from the math teacher.
Teaching math effectively requires teachers to understand how to guide students from turning procedural knowledge into conceptual understanding.
Educators need to understand the student needs in their class and use a range of learning strategies to build conceptual understanding for all students.
7 teaching strategies to develop conceptual understanding in math
Teaching conceptual understanding in math requires teachers to use instructional strategies that encourage higher-order thinking skills.
Here are 7 strategies elementary, middle and high school math teachers can incorporate into their math classes at all grade levels to build conceptual understanding.
1. Spiral curriculum
A spiral curriculum revisits mathematical process over time. Topics are introduced in smaller chunks and educators build students knowledge on them over time.
For example, a teacher is teaching fractions to students. First, they would want to introduce the concept of fractions through a real-life example such as cutting a cake.
The first spiral might compare fractions. This could be done using the cake as a visual representation to demonstrate the concept of parts of a whole. Real-life examples help students understand that they would rather have \frac{1}{2} of a cake than \frac{1}{4} because there are fewer parts of the whole.
Once students demonstrate mastery of this concept, teachers can continue to scaffold knowledge and ask students to apply these skills to more complex tasks.
2. Higher-order tasks to build critical thinking skills
Higher-order tasks are essential to building the critical thinking skills that lead to conceptual understanding. This type of thinking includes connecting ideas and expressing abstract thoughts.
A great way to teach this is through fun “brain-teasers” that your students can complete after finishing any assignment.
Create math problems that are an extension of the lesson and require students to apply their learning in different scenarios. Whether it’s tarsia puzzles or code crackers, through these tasks students should understand how math concepts can be used for various problem-solving tasks.
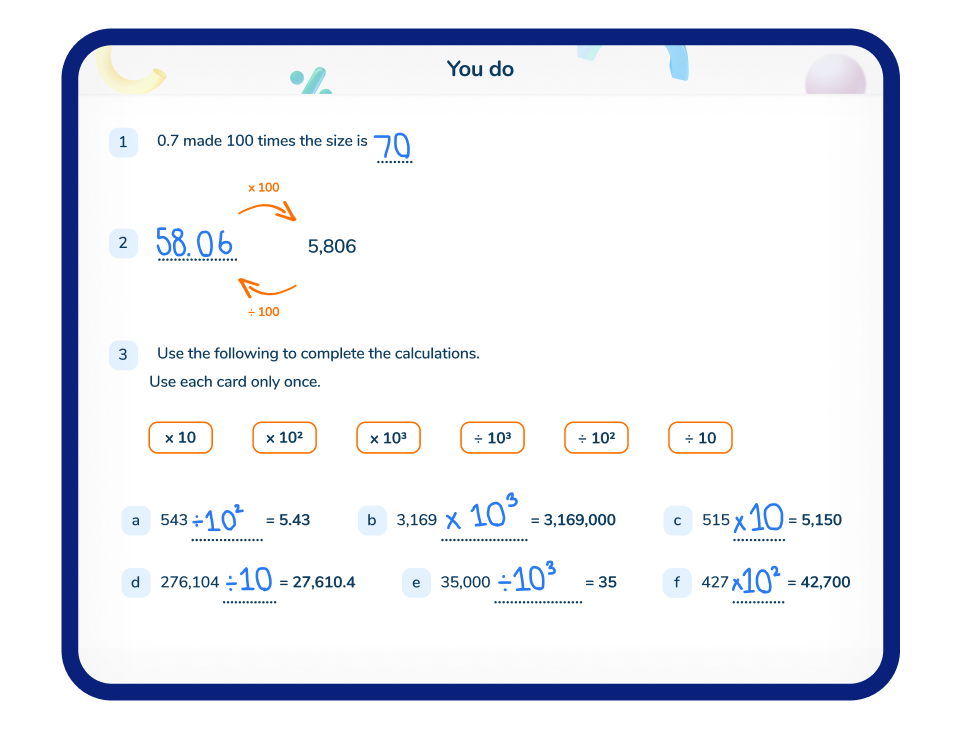
3. Visual representations to improve retrieval
Using visual representations for math concepts helps students apply their math knowledge without creating cognitive overload.
For example, visuals are an excellent tool for teaching geometry to middle school students. They help students conceptualise the abstractness of basic geometric properties, apply definitions, and understand relationships between concepts.
4. Manipulatives and hands-on learning
Manipulatives and hands-on learning help students visualize and relate concepts to their own experience rather than trying to imagine them abstractly.
For example, an elementary math teacher may ask students to practice single-digit multiplication with manipulatives like counting cubes at the start a multiplication lesson
This type of hands-on manipulation helps students gain a deeper conceptual understanding of multiplication as opposed to rote memorizing of multiplication facts without context.
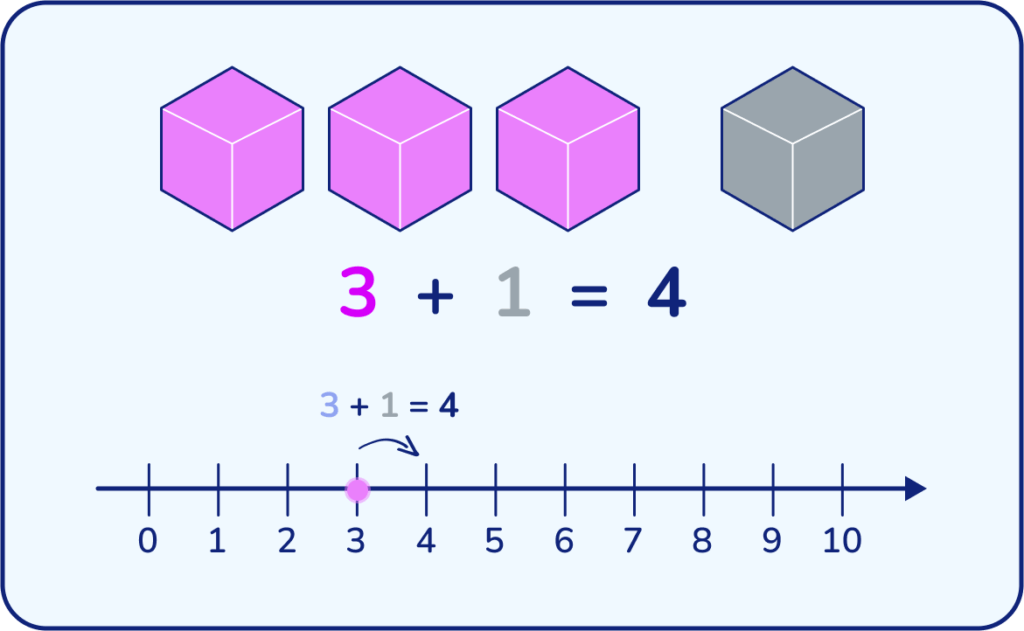
5. Connect concepts
Making connections between mathematical concepts with students promotes conceptual understanding. When students can demonstrate how ideas are related, they can make connections between procedural skills that build on each other.
To help make connections, you can use manipulatives and concrete representations to master addition and subtraction before progressing to a number line.
Start by giving students manipulatives such as blocks to demonstrate their understanding of addition. Once they have demonstrated mastery of that skill, students will be ready to begin learning the more abstract concept of a number line.
6. Make real world connections
When students can see the relevance of what is taught to the real world, they are much more likely to engage in the lesson.
Making connections to the real world takes considerable planning, but embeds conceptual understanding.
For example, when teaching your middle school students about percentages, create a classroom “store” and give each student a budget and a coupon for a different percentage off.
Differentiate learning by giving some students a percentage off one item, while others get a percentage off their whole purchase. It’s their job to figure out what they can afford. Students can also compare the difference between 20% off a single item versus an entire purchase!
7. Collaboration
Allowing students to collaborate is the perfect opportunity to demonstrate conceptual understanding with peers.
Metacognition occurs when students can understand their thought processes, a skill needed to share that understanding with others. Collaboration is a great way to encourage metacognition in the classroom and help students learn to share their thinking with others.
One way you can do this is by having students solve a more complex problem together that would be challenging individually. By communicating, sharing knowledge, and confirming understanding, students can solve a math problem that would be more difficult on their own.
Conclusion
Incorporating lessons and activities that encourage conceptual understanding into your school’s math curriculum helps students apply higher-order thinking skills to more complex mathematical concepts.
When students demonstrate conceptual understanding, they show they understand “why” and “how” they got to the answer. This thinking will transfer outside the classroom to the real world.
Conceptual understanding encourages students to tackle more complex mathematical problems, including those they have not encountered before.
FAQs
An example of conceptual understanding in math is if a student understands that equivalent fractions have the same value and represent the same number of parts of a whole, even though they have different numerators and denominators.
Conceptual understanding is a student’s ability to understand how math works rather than following rote memorization of rules.
Conceptual understanding helps students move beyond understanding the “what” of mathematical operations to communicating the “why” of how they got there, a skill they can apply to more complex math problems.
