Dividing Radicals - Math Steps, Examples & Questions

High Impact Tutoring Built By Math Experts

Personalized standards-aligned one-on-one math tutoring for schools and districts

Request a demo

In order to access this I need to be confident with:

Simplifying surds

Collecting like terms

Expanding brackets

Math resources Algebra Radicals

Dividing radicals

Here you will learn about dividing radicals, including when radical expressions can be divided, how to simplify expressions using division and how to do the calculations.

Students first learn how to work with radicals in pre-algebra and expand this knowledge as they move into an algebra $1$ and algebra $2$ class.

What is dividing radicals?

Dividing radicals is where radicals are combined using the division rule to be written as a rationalized radical expression. To divide radicals, use the quotient rule:

$\sqrt{\cfrac{m}{n}}=\cfrac{\sqrt{m}}{\sqrt{n}}=\sqrt{m}\div\sqrt{n}$

Once the radical expression is written in the form of a numerator divided by a denominator, you must determine if they divide evenly.

If the radicands in the numerator and denominator do not divide, you cannot leave the expression with a radical in the denominator. If there is a radical in the denominator, you must simplify by rationalizing it.

Examples

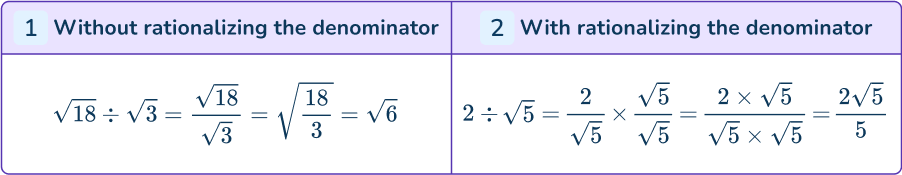
In the second example, you cannot divide the numerator and the denominator of the expression for two reasons.

The first is that both expressions are not radical expressions. In order to divide radicals, both expressions have to be radicals.

The second reason is that $2$ is not a factor of $5.$ So, $\cfrac{2}{\sqrt{5}}$ cannot be divided, but notice that the denominator has a radical expression which means that the expression needs to be rationalized.

In order to rationalize the denominator, multiply the numerator and the denominator by the radical in the denominator as shown above.

Step-by-step guide: Rationalize the denominator

Remember that surds can be further simplified using the product rule

\sqrt{a\times{b}}=\sqrt{a}\times\sqrt{b}

What is dividing radicals?

Common Core State Standards

How does this relate to high school math?

Number and Quantity – High School: (HSN-RN.B.3)
Explain why the sum or product of two rational numbers is rational; that the sum of a rational number and an irrational number is irrational; and that the product of a nonzero rational number and an irrational number is irrational.

[FREE] Algebra Check for Understanding Quiz (Grade 6 to 8)

Use this quiz to check your grade 6 to 8 students’ understanding of algebra. 10+ questions with answers covering a range of 6th and 8th grade algebra topics to identify areas of strength and support!

DOWNLOAD FREE

[FREE] Algebra Check for Understanding Quiz (Grade 6 to 8)

DOWNLOAD FREE

How to divide radicals

In order to divide radicals:

Substitute known values into the quotient rule.
Simplify the expression.

Dividing radicals examples

Example 1: simplifying radical expressions

Simplify the expression $\cfrac{\sqrt{24}}{\sqrt{8}}.$

Substitute known values into the quotient rule.

\cfrac{24}{8}=\sqrt{\cfrac{24}{8}}

2Simplify the expression.

\sqrt{\cfrac{24}{8}}=\sqrt{3}.

So $\cfrac{\sqrt{24}}{\sqrt{8}}$ simplified fully is $\sqrt{3}.$

Example 2: simplifying radical expressions

Simplify the expression $\cfrac{4\sqrt{15}}{2\sqrt{5}}$ fully.

Substitute known values into the quotient rule.

The coefficients and the radicals of the numerator and denominator divide each other; $2$ is a factor of $4$ and $5$ is a factor of $15.$

$4\div{2}=2$

$\sqrt{15}\div\sqrt{5}=\cfrac{\sqrt{15}}{\sqrt{5}}=\sqrt{\cfrac{15}{5}}=\sqrt{3}$

Simplify the expression.

\cfrac{4\sqrt{15}}{2\sqrt{5}}=2\sqrt{3}

Example 3: simplifying radical expressions

Simplify fully the radical expression $\cfrac{\sqrt{27}}{3\sqrt{3}}.$

Substitute known values into the quotient rule.

The radical in the numerator and the radical in the denominator divide evenly, $3$ is a factor of $27.$

$1$ is the coefficient of the numerator and $3$ is the coefficient of the denominator. $3$ is not a factor of $1.$

$\sqrt{27}\div\sqrt{3}=\cfrac{\sqrt{27}}{\sqrt{3}}=\sqrt{\cfrac{27}{3}}=\sqrt{9}$

$1\div{3}=\cfrac{1}{3}$

Simplify the expression.

$9$ is a perfect square so $\sqrt{9}$ can be simplified further.

$\sqrt{9}=\sqrt{3\times{3}}=\sqrt{3}\times\sqrt{3}=3$

So $\cfrac{\sqrt{27}}{3\sqrt{3}}=\cfrac{\sqrt{9}}{3}=\cfrac{3}{3}=1$

Example 4: rationalize denominator

Simplify the radical expression $\cfrac{5\sqrt{2}}{\sqrt{3}}$ fully.

Substitute known values into the quotient rule.

$\sqrt{2}$ is the radical in the numerator and $\sqrt{3}$ is the radical in the denominator. $3$ is not a factor of $2$ so they do not divide evenly. The coefficient of the numerator is $5$ and the coefficient of the denominator is $1, \, 5$ divided by $1$ is $5.$

The numerator and denominator do not divide each other evenly.

In this case, you have to rationalize the denominator to get rid of the radical expression in the denominator.

Simplify the expression.

\cfrac{5\sqrt{2}}{\sqrt{3}}

Expressions that have radicals in the denominator are considered irrational.

Rationalize the expression by multiplying the numerator and the denominator by $\sqrt{3}.$

$\cfrac{5\sqrt{2}}{\sqrt{3}}\times\cfrac{\sqrt{3}}{\sqrt{3}}=\cfrac{5\sqrt{6}}{\sqrt{9}}=\cfrac{5\sqrt{6}}{3}$

$\cfrac{5\sqrt{2}}{\sqrt{3}}$ simplified fully is $\cfrac{5\sqrt{6}}{3}.$

Example 5: dividing radical expressions

Simplify the expression $\cfrac{\sqrt{40}}{\sqrt{5}}.$

Substitute known values into the quotient rule.

\cfrac{\sqrt{40}}{\sqrt{5}}=\sqrt{\cfrac{40}{5}}

Simplify the expression.

\sqrt{\cfrac{40}{5}}=\sqrt{8}.

$\sqrt{8}$ can be simplified further using the product rule.

$\sqrt{8}=\sqrt{4\times{2}}=\sqrt{4}\times\sqrt{2}=2\sqrt{2}$

So $\cfrac{\sqrt{40}}{\sqrt{5}}$ simplified fully is $2\sqrt{2}.$

Example 6: dividing radical expressions

Simplify the expression $\cfrac{4\sqrt{15}+\sqrt{60}}{\sqrt{5}}.$

Substitute known values into the quotient rule.

Before substituting into the quotient rule, the numerator can be simplified first.

In this case, you can use factoring skills to determine if the radical in the denominator divides the numerator. The expression can be rewritten as:

$\cfrac{4\sqrt{15}+\sqrt{60}}{\sqrt{5}}=\cfrac{4\times\sqrt{5\times{3}}+\sqrt{5\times{12}}}{\sqrt{5}}=\cfrac{4\times\sqrt{5}\times\sqrt{3}+\sqrt{5}\times\sqrt{12}}{\sqrt{5}}$

Notice how all of the terms in the fraction contain a common factor of $\sqrt{5}.$

You can factor the numerator to be:

$\cfrac{4\times\sqrt{5}\times\sqrt{3}+\sqrt{5}\times\sqrt{12}}{\sqrt{5}}=\cfrac{\sqrt{5}(4 \sqrt{3}+\sqrt{12})}{\sqrt{5}}$

The $\sqrt{5}$ in the denominator cancels the $\sqrt{5}$ in the numerator.

$\cfrac{\sqrt{5}(4 \sqrt{3}+\sqrt{12})}{\sqrt{5}}=4 \sqrt{3}+\sqrt{12}$

Simplify the expression.

$4 \sqrt{3}+\sqrt{12}$ can be simplified further because $12$ has a perfect square factor of $4.$

\begin{aligned}\sqrt{12}&=\sqrt{4}\times\sqrt{3} \\\\ &=2\times\sqrt{3} \\\\ &=2\sqrt{3} \end{aligned}

$\sqrt{12}$ can be rewritten as $2\sqrt{3}\text{.}$

Therefore $4\sqrt{3}+\sqrt{12}=4\sqrt{3}+2\sqrt{3}.$

Since the numbers under the radicals are the same, you add them together.

$4\sqrt{3}+2\sqrt{3}=6\sqrt{3}$

So $\cfrac{4\sqrt{15}+\sqrt{60}}{\sqrt{5}}$ fully simplified is $6\sqrt{3}.$

Example 7: using a conjugate to rationalize the denominator

Simplify the radical expression $\cfrac{5}{1+\sqrt{2}}.$

Substitute known values into the quotient rule.

In the expression $\cfrac{5}{1+\sqrt{2}}$ , you cannot divide the numerator by the denominator.

Simplify the expression.

In order to simplify $\cfrac{5}{1+\sqrt{2}},$ you must find the conjugate of the denominator and then multiply the numerator and the denominator by the conjugate.

The conjugate of the binomial in the denominator, $1+\sqrt{2},$ is $1-\sqrt{2}.$ Notice how the operation of $“+”$ changed to $“-”.$

Step-by-step guide: Difference of two squares

When multiplying radical expressions in the denominator, the radical simplifies to a whole number.

$\cfrac{5}{1+\sqrt{2}}\times\cfrac{1-\sqrt{2}}{1-\sqrt{2}}=\cfrac{5-5\sqrt{2}}{1+\sqrt{2}-\sqrt{2}-\sqrt{4}}=\cfrac{5-5\sqrt{2}}{1-2}=\cfrac{5-5\sqrt{2}}{- \, 1}$

$=- \, 5+5\sqrt{2}$

$\cfrac{5}{1+\sqrt{2}}=- \, 5+5 \sqrt{2}$

Teaching tips for dividing radicals

Reinforce perfect square numbers with students.

Review with students how to break numbers down into their factors.

Instead of having students practice skills with worksheets, incorporate game playing.

Easy mistakes to make

Dividing a radical with a coefficient
For example, in the expression $\cfrac{4}{\sqrt{2}}$ thinking that you can divide.
$\cfrac{4}{\sqrt{2}}$ ≠ ${2}$ or $\sqrt{2}$

You can only divide radicals with radicals and coefficients with coefficients.

In this case, you cannot divide, but you can rationalize the denominator.

$\cfrac{4}{\sqrt{2}}\times\cfrac{\sqrt{2}}{\sqrt{2}}=\cfrac{4\sqrt{2}}{\sqrt{4}}=\cfrac{4\sqrt{2}}{2}=2\sqrt{2}$

Forgetting to divide the entire numerator by the denominator
For example, in the expression $\cfrac{2\sqrt{15}-\sqrt{10}}{\sqrt{5}}$ only dividing one part of the numerator by the denominator,

$\cfrac{2\sqrt{15}-\sqrt{10}}{\sqrt{5}}$ ≠ ${2}\sqrt{3}-\sqrt{10}$

In this case, the correct way to divide is to divide $\sqrt{5}$ into both parts of the numerator, $2\sqrt{15}\div\sqrt{5}$ and $\sqrt{10}\div\sqrt{5}.$

So, $\cfrac{2\sqrt{15}-\sqrt{10}}{\sqrt{5}}=2\sqrt{3}-\sqrt{2}$

Practice dividing radicals questions

\sqrt{10}

\sqrt{36}

2\sqrt{5}

\sqrt{160}

For the expression $\cfrac{\sqrt{40}}{\sqrt{4}},$ the radical in the denominator divides into the radical in the numerator because $4$ is a factor of $40.$

\sqrt{40}\div\sqrt{4}=\sqrt{\cfrac{40}{4}}=\sqrt{10}

5 \sqrt{3}

\sqrt{45}

3 \sqrt{5}

\sqrt{88}

For the expression $\cfrac{\sqrt{90}}{\sqrt{2}}$ , the radical in the denominator divides into the radical in the numerator because $2$ is a factor of $90.$

\sqrt{90} \div \sqrt{2}=\sqrt{45}

\cfrac{\sqrt{90}}{\sqrt{2}}=\sqrt{45}

$\sqrt{45}$ can be simplified further because $9$ is the largest square factor of $45.$

\begin{aligned}\sqrt{45}&=\sqrt{9}\times\sqrt{5} \\\\ &=3\times\sqrt{5} \\\\ &=3\sqrt{5} \end{aligned}

7 \sqrt{30}

\cfrac{7 \sqrt{30}}{6}

\cfrac{7 \sqrt{5}}{36}

\cfrac{7 \sqrt{5}}{6}

For the expression $\cfrac{7 \sqrt{5}}{\sqrt{6}},$ the radical in the denominator does not divide into the radical in the numerator because $6$ is not a factor of $5.$

So in order to remove the radical from the denominator, you have to rationalize the denominator by multiplying the numerator and the denominator by $\sqrt{6}.$

\cfrac{7\sqrt{5}}{\sqrt{6}}\times\cfrac{\sqrt{6}}{\sqrt{6}}=\cfrac{7\sqrt{30}}{\sqrt{36}}=\cfrac{7\sqrt{30}}{6}

\cfrac{7\sqrt{30}}{6}

$30$ does not have any square factors except $1$ so this cannot be simplified any further.

\cfrac{12\sqrt{6}}{2}

2\sqrt{6}

\cfrac{6\sqrt{2}}{\sqrt{6}}

6\sqrt{2}

The expression $\cfrac{12\sqrt{12}}{2\sqrt{6}}$ can be divided because the coefficient of the denominator divides the coefficient of the numerator $(2$ is a factor of $12).$

Also, the radical in the denominator divides the radical in the numerator, $(6$ is a factor of $12).$

You can remove the radical from the denominator by dividing.

12\div2=6

\sqrt{12} \div \sqrt{6}=\sqrt{2}

\cfrac{12\sqrt{12}}{2\sqrt{6}}=6\sqrt{2}

\cfrac{\sqrt{22}}{3}

\cfrac{\sqrt{22}}{33}

\sqrt{22}

\cfrac{\sqrt{22}}{11}

For the expression $\cfrac{\sqrt{2}}{3 \sqrt{11}} ,$ the denominator does not divide evenly into the numerator.

So, to remove the radical from the denominator, you have to rationalize the denominator.

To rationalize the denominator, multiply the denominator and the numerator by $\sqrt{11}.$

\cfrac{\sqrt{2}}{3\sqrt{11}}\times\cfrac{\sqrt{11}}{\sqrt{11}}=\cfrac{\sqrt{22}}{3\sqrt{121}}=\cfrac{\sqrt{22}}{3\times11}=\cfrac{\sqrt{22}}{33}

6\sqrt{3}

6\sqrt{5}

2\sqrt{3}

2\sqrt{5}

For the expression $\cfrac{4 \sqrt{15}+2 \sqrt{15}}{3 \sqrt{5}},$ the numerator can be simplified because the numbers under the radicals are the same.

So, the radical in the denominator can be removed by division.

So, $\cfrac{4\sqrt{15}+2\sqrt{15}}{3\sqrt{5}}=\cfrac{6\sqrt{15}}{3\sqrt{5}}$

The numerator and the denominator can divide because $6\div{3}=2$ and $\sqrt{15}\div\sqrt{5}=\sqrt{3}$

So, $\cfrac{6\sqrt{15}}{3\sqrt{5}}=2\sqrt{3}$

Dividing radicals FAQs

Can exponential expressions be represented by radical expressions?

Yes, exponential expressions can be written as exponential expressions using rational exponents.

Can cube root expressions be simplified?

Yes, cube root expressions can be simplified using the same strategies as simplifying square root expressions.

Can there be polynomials under a radical sign?

Yes, there can be polynomials under radical signs such as $\sqrt{x^{2}+2x+1}.$
When solving radical equations, you will see polynomials under the radical.

Can there be radicals in rational expressions?

Yes, there can be radicals in rational expressions, such as in the expressions, $\cfrac{\sqrt{x}}{\sqrt{2x-4}}.$

The next lessons are

Still stuck?

At Third Space Learning, we specialize in helping teachers and school leaders to provide personalized math support for more of their students through high-quality, online one-on-one math tutoring delivered by subject experts.

Each week, our tutors support thousands of students who are at risk of not meeting their grade-level expectations, and help accelerate their progress and boost their confidence.